Enhancing the Low-Frequency Induction Heating Effect of Magnetic Composites for Medical Applications
Abstract
:1. Introduction
2. Material Fabrication
3. Results and Discussions
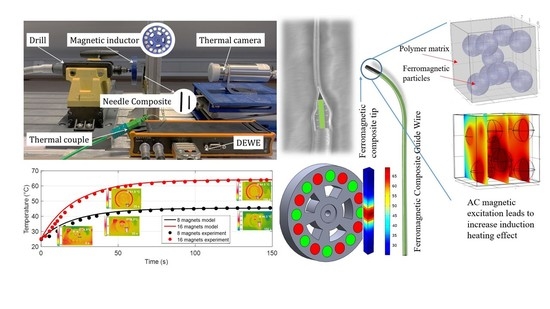
3.1. Experimental Setup
3.2. Experimental Result
4. Ferromagnetic Composite Enhancement: Toward Medical Application
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Rudnev, V.; Loveless, D.; Cook, R.L.; Loveless, D.; Cook, R.L. Handbook of Induction Heating; CRC Press: Boca Raton, FL, USA, 2017; ISBN 978-1-315-11748-5. [Google Scholar]
- Lozinskiĭ, M.G. Industrial Applications of Induction Heating; Pergamon Press: Oxford, UK, 1969; ISBN 978-0080115863. [Google Scholar]
- Moreland, W.C. The Induction Range: Its Performance and Its Development Problems. IEEE Trans. Ind. Appl. 1973, 9, 81–85. [Google Scholar] [CrossRef]
- Stauffer, P.R.; Cetas, T.C.; Jones, R.C. Magnetic Induction Heating of Ferromagnetic Implants for Inducing Localized Hyperthermia in Deep-Seated Tumors. IEEE Trans. Biomed. Eng. 1984, 31, 235–251. [Google Scholar] [CrossRef] [PubMed]
- Lucía, O.; Maussion, P.; Dede, E.J.; Burdío, J.M. Induction Heating Technology and Its Applications: Past Developments, Current Technology, and Future Challenges. IEEE Trans. Ind. Electron. 2014, 61, 2509–2520. [Google Scholar] [CrossRef] [Green Version]
- Soares, P.I.P.; Laia, C.A.T.; Carvalho, A.; Pereira, L.C.J.; Coutinho, J.T.; Ferreira, I.M.M.; Novo, C.M.M.; Borges, J.P. Iron oxide nanoparticles stabilized with a bilayer of oleic acid for magnetic hyperthermia and MRI applications. Appl. Surf. Sci. 2016, 383, 240–247. [Google Scholar] [CrossRef]
- Soares, P.I.P.; Lochte, F.; Echeverria, C.; Pereira, L.C.J.; Coutinho, J.T.; Ferreira, I.M.M.; Novo, C.M.M.; Borges, J.P.M.R. Thermal and magnetic properties of iron oxide colloids: Influence of surfactants. Nanotechnology 2015, 26, 425704. [Google Scholar] [CrossRef]
- Cano, M.E.; Barrera, A.; Estrada, J.C.; Hernandez, A.; Cordova, T. An induction heater device for studies of magnetic hyperthermia and specific absorption ratio measurements. Rev. Sci. Instrum. 2011, 82, 114904. [Google Scholar] [CrossRef]
- Gómez-Polo, C.; Larumbe, S.; Pérez-Landazábal, J.I.; Pastor, J.M. Analysis of heating effects (magnetic hyperthermia) in FeCrSiBCuNb amorphous and nanocrystalline wires. J. Appl. Phys. 2012, 111, 07A314. [Google Scholar] [CrossRef]
- Xiang, Z.; Ducharne, B.; Della Schiava, N.; Capsal, J.-F.; Cottinet, P.-J.; Coativy, G.; Lermusiaux, P.; Le, M.Q. Induction heating-based low-frequency alternating magnetic field: High potential of ferromagnetic composites for medical applications. Mater. Des. 2019, 174, 107804. [Google Scholar] [CrossRef]
- Ito, A.; Shinkai, M.; Honda, H.; Kobayashi, T. Medical application of functionalized magnetic nanoparticles. J. Biosci. Bioeng. 2005, 100, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.; Jiang, M.; Zhou, Z.; Gou, J.; Hui, D. 3D printing of polymer matrix composites: A review and prospective. Compos. Part B Eng. 2017, 110, 442–458. [Google Scholar] [CrossRef]
- Grinberg, D.; Siddique, S.; Le, M.-Q.; Liang, R.; Capsal, J.-F.; Cottinet, P.-J. 4D Printing based piezoelectric composite for medical applications. J. Polym. Sci. Part B Polym. Phys. 2019, 57, 109–115. [Google Scholar] [CrossRef]
- Liu, J.-J.; Fan, L.-H.; Xu, D.-C.; Li, X.; Dong, Z.-H.; Fu, W.-G. The endovenous laser treatment for patients with varicose veins. Pak. J. Med. Sci. 2016, 32, 55–58. [Google Scholar] [PubMed] [Green Version]
- Gupta, A.K.; Gupta, M. Synthesis and surface engineering of iron oxide nanoparticles for biomedical applications. Biomaterials 2005, 26, 3995–4021. [Google Scholar] [CrossRef] [PubMed]
- Yan, A.; Liu, X.; Qiu, G.; Wu, H.; Yi, R.; Zhang, N.; Xu, J. Solvothermal synthesis and characterization of size-controlled Fe3O4 nanoparticles. J. Alloys Compd. 2008, 458, 487–491. [Google Scholar] [CrossRef]
- Wojtyła, S.; Klama, P.; Baran, T. Is 3D printing safe? Analysis of the thermal treatment of thermoplastics: ABS, PLA, PET, and nylon. J. Occup. Environ. Hyg. 2017, 14, D80–D85. [Google Scholar] [CrossRef]
- Xiang, Z.; Le, M.-Q.; Cottinet, P.-J.; Ducharne, B. Induction heating properties of ferromagnetic composite for varicose veins healing. In Proceedings of the 15th International Conference on Magnetic Fluid (ICMF), Paris, France, 8–12 July 2019. [Google Scholar]
- Bayerl, T.; Duhovic, M.; Mitschang, P.; Bhattacharyya, D. The heating of polymer composites by electromagnetic induction—A review. Compos. Part A Appl. Sci. Manuf. 2014, 57, 27–40. [Google Scholar] [CrossRef]
- Xiang, Z.; Gupta, B.; Le, M.Q.; Cottinet, P.J.; Ducharne, B. Hysteresis Model of 3D Printed Magnetic Particles Based Polymer Composite Materials. In Proceedings of the 2018 IEEE International Magnetics Conference (INTERMAG), Singapore, 23–27 April 2018; pp. 1–5. [Google Scholar] [CrossRef]
- García-Madrid, C.; Pastor Manrique, J.Ó.; Gómez Blasco, F.; Sala Planell, E. New Advances in the Treatment of Varicose Veins: Endovenous Radiofrequency VNUS Closure®. Cirugía Española Engl. Ed. 2011, 89, 420–426. [Google Scholar] [CrossRef]
- Yang, Y.; He, J.; Li, Q.; Gao, L.; Hu, J.; Zeng, R.; Qin, J.; Wang, S.X.; Wang, Q. Self-healing of electrical damage in polymers using superparamagnetic nanoparticles. Nat. Nanotechnol. 2019, 14, 151. [Google Scholar] [CrossRef]
- Riviere, L.; Causse, N.; Lonjon, A.; Dantras, E.; Lacabanne, C. Specific heat capacity and thermal conductivity of PEEK/Ag nanoparticles composites determined by Modulated-Temperature Differential Scanning Calorimetry. Polym. Degrad. Stab. 2016, 127, 98–104. [Google Scholar] [CrossRef] [Green Version]
- Budiansky, B. Thermal and thermoelastic properties of isotropic composites. J. Compos. Mater. 1970, 4, 286–295. [Google Scholar] [CrossRef]
- Wang, J.; Carson, J.K.; North, M.F.; Cleland, D.J. A new structural model of effective thermal conductivity for heterogeneous materials with co-continuous phases. Int. J. Heat Mass Transf. 2008, 51, 2389–2397. [Google Scholar] [CrossRef]

















| 8-Magnet Inductor | 16-Magnet Inductor | |||
|---|---|---|---|---|
| Big Needle | 18 °C | 21 °C | 35 °C | 40 °C |
| Small Need | 11 °C | 14 °C | 25 °C | 30 °C |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiang, Z.; Jakkpat, K.-I.; Ducharne, B.; Capsal, J.-F.; Mogniotte, J.-F.; Lermusiaux, P.; Cottinet, P.-J.; Della Schiava, N.; Le, M.Q. Enhancing the Low-Frequency Induction Heating Effect of Magnetic Composites for Medical Applications. Polymers 2020, 12, 386. https://doi.org/10.3390/polym12020386
Xiang Z, Jakkpat K-I, Ducharne B, Capsal J-F, Mogniotte J-F, Lermusiaux P, Cottinet P-J, Della Schiava N, Le MQ. Enhancing the Low-Frequency Induction Heating Effect of Magnetic Composites for Medical Applications. Polymers. 2020; 12(2):386. https://doi.org/10.3390/polym12020386
Chicago/Turabian StyleXiang, Ziyin, Khao-Iam Jakkpat, Benjamin Ducharne, Jean-Fabien Capsal, Jean-François Mogniotte, Patrick Lermusiaux, Pierre-Jean Cottinet, Nellie Della Schiava, and Minh Quyen Le. 2020. "Enhancing the Low-Frequency Induction Heating Effect of Magnetic Composites for Medical Applications" Polymers 12, no. 2: 386. https://doi.org/10.3390/polym12020386
APA StyleXiang, Z., Jakkpat, K. -I., Ducharne, B., Capsal, J. -F., Mogniotte, J. -F., Lermusiaux, P., Cottinet, P. -J., Della Schiava, N., & Le, M. Q. (2020). Enhancing the Low-Frequency Induction Heating Effect of Magnetic Composites for Medical Applications. Polymers, 12(2), 386. https://doi.org/10.3390/polym12020386