Fabrication and Characterization of a Low-Cost Microfluidic System for the Manufacture of Alginate–Lacasse Microcapsules
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Microsystem Design and Multiphysics Simulation
2.3. Fabrication of the Microfluidic Device for Microcapsules Generation
2.4. Fabrication and Characterization of Microcapsules
2.5. Enzyme Encapsulation
2.6. Free and Microencapsulated Laccase Activity
2.7. Encapsulated Laccase Characterization
3. Results
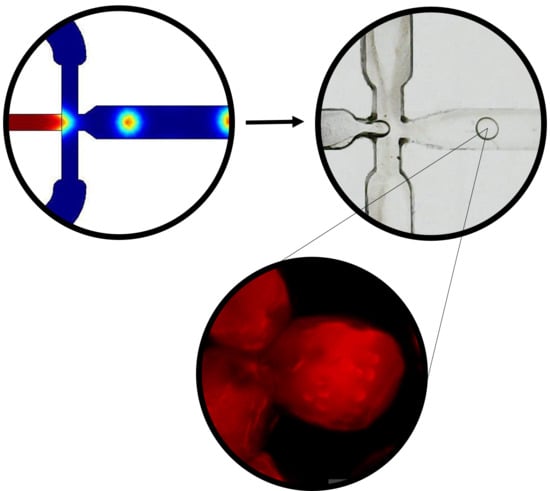
3.1. Multiphysics Simulations
3.2. Microcapsules Size Analysis
3.3. Visualization of Enzyme Encapsulation
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Mesh Convergence Study

References
- Perignon, C.; Ongmayeb, G.; Neufeld, R.; Frere, Y.; Poncelet, D. Microencapsulation by interfacial polymerisation: Membrane formation and structure. J. Microencapsul. 2015, 32, 1–15. [Google Scholar] [CrossRef] [PubMed]
- Fu, F.; Hu, L. Temperature sensitive colour-changed composites. In Advanced High Strength Natural Fibre Composites in Construction; Elsevier: Amsterdam, The Netherlands, 2017; pp. 405–423. [Google Scholar]
- Ozkan, G.; Franco, P.; De Marco, I.; Xiao, J.; Capanoglu, E. A review of microencapsulation methods for food antioxidants: Principles, advantages, drawbacks and applications. Food Chem. 2019, 272, 494–506. [Google Scholar] [CrossRef] [PubMed]
- Yuni Hendrawati, T.; Meta Sari, A.; Iqbal Syauqi Rahman, M.; Ariatmi Nugrahani, R.; Siswahyu, A. Microencapsulation Techniques of Herbal Compounds for Raw Materials in Food Industry, Cosmetics and Pharmaceuticals. In Microencapsulation-Processes, Technologies and Industrial Applications; IntechOpen: London, UK, 2019. [Google Scholar]
- Synowiec, A.; Lenart-Boron, A.; Kalemba, D. Effect of soil application of microencapsulated caraway oil on weed infestation and maize yield. Int. J. Pest Manag. 2018, 64, 315–323. [Google Scholar] [CrossRef]
- Shishir, M.R.I.; Xie, L.; Sun, C.; Zheng, X.; Chen, W. Advances in micro and nano-encapsulation of bioactive compounds using biopolymer and lipid-based transporters. Trends Food Sci. Technol. 2018, 78, 34–60. [Google Scholar] [CrossRef]
- Gonzalez-Pujana, A.; Orive, G.; Pedraz, J.L.; Santos-Vizcaino, E.; Hernandez, R.M. Alginate Microcapsules for Drug Delivery. Adv. Calcium Phosphate Biomater. 2017, 11, 67–100. [Google Scholar]
- Mu, X.T.; Ju, X.J.; Zhang, L.; Huang, X.B.; Faraj, Y.; Liu, Z.; Wang, W.; Xie, R.; Deng, Y.; Chu, L.Y. Chitosan microcapsule membranes with nanoscale thickness for controlled release of drugs. J. Membr. Sci. 2019, 590, 117275. [Google Scholar] [CrossRef]
- Semyonov, D.; Ramon, O.; Kovacs, A.; Friedlander, L.; Shimoni, E. Air-Suspension Fluidized-Bed Microencapsulation of Probiotics. Dry. Technol. 2012, 30, 1918–1930. [Google Scholar] [CrossRef]
- Li, M.; Xu, W.; Hu, D.; Song, B. Preparation and application of pyraclostrobin microcapsule formulations. Colloids Surfaces A: Physicochem. Eng. Asp. 2018, 553, 578–585. [Google Scholar] [CrossRef]
- He, Z.; Jiang, S.; Li, Q.; Wang, J.; Zhao, Y.; Kang, M. Facile and cost-effective synthesis of isocyanate microcapsules via polyvinyl alcohol-mediated interfacial polymerization and their application in self-healing materials. Compos. Sci. Technol. 2017, 138, 15–23. [Google Scholar] [CrossRef]
- Xiao, Y.; Wu, B.; Fu, X.; Wang, R.; Lei, J. Preparation of biodegradable microcapsules through an organic solvent-free interfacial polymerization method. Polym. Adv. Technol. 2019, 30, 483–488. [Google Scholar] [CrossRef]
- Kentepozidou, A.; Kiparissides, C. Production of water-containing polymer microcapsules by the complex emulsion/solvent evaporation technique. Effect of process variables on the microcapsule size distribution. Microencapsul. 1995, 12, 627–638. [Google Scholar] [CrossRef] [PubMed]
- Salaün, F. Microencapsulation technology for smart textile coatings. In Active Coatings for Smart Textiles; Elsevier Inc.: Amsterdam, The Netherlands, 2016; pp. 179–220. [Google Scholar]
- Wang, X.; Yin, H.; Chen, Z.; Xia, L. Epoxy resin/ethyl cellulose microcapsules prepared by solvent evaporation for repairing microcracks: Particle properties and slow-release performance. Mater. Today Commun. 2020, 22, 100854. [Google Scholar] [CrossRef]
- Timilsena, Y.P.; Akanbi, T.O.; Khalid, N.; Adhikari, B.; Barrow, C.J. Complex coacervation: Principles, mechanisms and applications in microencapsulation. Int. J. Boil. Macromol. 2019, 121, 1276–1286. [Google Scholar] [CrossRef] [PubMed]
- Giri, T.K. Alginate Containing Nanoarchitectonics for Improved Cancer Therapy. In Nanoarchitectonics for Smart Delivery and Drug Targeting; Elsevier Inc.: Amsterdam, The Netherlands, 2016; pp. 565–588. [Google Scholar]
- Li, Y.; Wu, C.; Wu, T.; Wang, L.; Chen, S.; Ding, T.; Hu, Y. Preparation and characterization of citrus essential oils loaded in chitosan microcapsules by using different emulsifiers. J. Food Eng. 2018, 217, 108–114. [Google Scholar] [CrossRef]
- Benavides, S.; Cortés, P.; Parada, J.; Franco, W. Development of alginate microspheres containing thyme essential oil using ionic gelation. Food Chem. 2016, 204, 77–83. [Google Scholar] [CrossRef] [PubMed]
- Paula, D.A.; Martins, E.M.F.; Costa, N.A.; de Oliveira, P.M.; de Oliveira, E.B.; Ramos, A.M. Use of gelatin and gum arabic for microencapsulation of probiotic cells from Lactobacillus plantarum by a dual process combining double emulsification followed by complex coacervation. Int. J. Biol. Macromol. 2019, 133, 722–731. [Google Scholar] [CrossRef] [PubMed]
- Seo, M.; Seo, M.; Choi, S.E.; Shin, K.; Lee, J.B.; Yang, D.Y.; Kim, J.W. Cellulose nanofiber-multilayered fruit peel-mimetic gelatin hydrogel microcapsules for micropackaging of bioactive ingredients. Carbohydr. Polym. 2020, 229, 115559. [Google Scholar] [CrossRef] [PubMed]
- Kang, Y.R.; Lee, Y.K.; Kim, Y.J.; Chang, Y.H. Characterization and storage stability of chlorophylls microencapsulated in different combination of gum Arabic and maltodextrin. Food Chem. 2019, 272, 337–346. [Google Scholar] [CrossRef]
- Tohidi, S.D.; Dencheva, N.; Denchev, Z.; Rocha, A.M.; Engesser, B. Development and characterization of single polymer composites prepared by compression molding of polyamide 6 empty microcapsules and novel woven textile structures. Mater. Today Commun. 2020, 23, 100912. [Google Scholar] [CrossRef]
- Pak, A.R.; Park, J.H.; Lee, S.G. Blowing Properties and Functionality of Thermoplastic Polyester Film Using Thermally Expandable Microcapsules. Polymers 2019, 11, 1652. [Google Scholar] [CrossRef] [Green Version]
- Lee, K.Y.; Mooney, D.J. Alginate: Properties and biomedical applications. Prog. Polym. Sci. 2012, 37, 106–126. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ching, S.H.; Bansal, N.; Bhandari, B. Alginate gel particles–A review of production techniques and physical properties. Crit. Rev. Food Sci. Nutr. 2017, 57, 1133–1152. [Google Scholar] [CrossRef] [PubMed]
- Reis, C.P.; Neufeld, R.J.; Vilela, S.; Ribeiro, A.J.; Veiga, F. Review and current status of emulsion/dispersion technology using an internal gelation process for the design of alginate particles. J. Microencapsul. 2006, 23, 245–257. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Rochefort, D. Characterisation and applications of microcapsules obtained by interfacial polycondensation. J. Microencapsul. 2012, 29, 636–649. [Google Scholar] [CrossRef] [PubMed]
- Thorne, M.F.; Simkovic, F.; Slater, A.G. Production of monodisperse polyurea microcapsules using microfluidics. Sci. Rep. 2019, 9, 17983–17987. [Google Scholar] [CrossRef] [PubMed]
- Enck, K.; Rajan, S.P.; Aleman, J.; Castagno, S.; Long, E.; Khalil, F.; Hall, A.R.; Opara, E.C. Design of an Adhesive Film-Based Microfluidic Device for Alginate Hydrogel-Based Cell Encapsulation. Ann. Biomed. Eng. 2020, 48, 1103–1111. [Google Scholar] [CrossRef] [PubMed]
- Yew, M.; Ren, Y.; Koh, K.S.; Sun, C.; Snape, C.; Yan, Y. Synthesis of microcapsules for carbon capture via needle-bassed drplet micofluidics Synthesis of microcapsules for carbon capture via needle-based droplet microfluidics. Energy Procedia 2019, 160, 443–450. [Google Scholar] [CrossRef]
- Serra, C.A.; Khan, I.U.; Cortese, B.; de Croon, M.H.J.M.; Hessel, V.; Ono, T.; Anton, N.; Vandamme, T. Microfluidic Production of Micro- and Nanoparticles. In Encyclopedia of Polymer Science and Technology; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2013. [Google Scholar]
- Shang, L.; Cheng, Y.; Zhao, Y. Emerging Droplet Microfluidics. Chem. Rev. 2017, 117, 7964–8040. [Google Scholar] [CrossRef]
- Elvira, K.S.; I Solvas, X.C.; Wootton, R.C.R.; Demello, A.J. The past, present and potential for microfluidic reactor technology in chemical synthesis. Nat. Chem. 2013, 5, 905–915. [Google Scholar] [CrossRef]
- Holtze, C. Large-scale droplet production in microfluidic devices - An industrial perspective. J. Phys. D: Appl. Phys. 2013, 46, 114008. [Google Scholar] [CrossRef]
- Jeong, H.H.; Issadore, D.; Lee, D. Recent developments in scale-up of microfluidic emulsion generation via parallelization. Korean J. Chem. Eng. 2016, 33, 1757–1766. [Google Scholar] [CrossRef]
- Walsh, D.I.; Kong, D.S.; Murthy, S.K.; Carr, P.A. Enabling Microfluidics: From Clean Rooms to Makerspaces. Trends Biotechnol. 2017, 35, 383–392. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rui Rodrigues, J.; Lagoa, R. Copper ions binding in Cu-alginate gelation. J. Carbohydr. Chem. 2006, 25, 219–232. [Google Scholar] [CrossRef]
- Kaczmarek-Pawelska, A. Alginate-Based Hydrogels in Regenerative Medicine. In Alginates-Recent Uses of This Natural Polymer; IntechOpen: London, UK, 2020. [Google Scholar]
- Wichai, S.; Chuysinuan, P.; Chaiarwut, S.; Ekabutr, P.; Supaphol, P. Development of bacterial cellulose/alginate/chitosan composites incorporating copper (II) sulfate as an antibacterial wound dressing. J. Drug Deliv. Sci. Technol. 2019, 51, 662–671. [Google Scholar] [CrossRef]
- Zhou, Q.; Kang, H.; Bielec, M.; Wu, X.; Cheng, Q.; Wei, W.; Dai, H. Influence of different divalent ions cross-linking sodium alginate-polyacrylamide hydrogels on antibacterial properties and wound healing. Carbohydr. Polym. 2018, 197, 292–304. [Google Scholar] [CrossRef]
- Ramírez-Cavazos, L.I.; Junghanns, C.; Ornelas-Soto, N.; Cárdenas-Chávez, D.L.; Hernández-Luna, C.; Demarche, P.; Enaud, E.; García-Morales, R.; Agathos, S.N.; Parra, R. Purification and characterization of two thermostable laccases from Pycnoporus sanguineus and potential role in degradation of endocrine disrupting chemicals. J. Mol. Catal. B: Enzym. 2014, 108, 32–42. [Google Scholar] [CrossRef] [Green Version]
- Iliev, S.P. Modelling the One-Dimensional Advection- Diffusion Equation in MATLAB Computational Fluid Dynamics Coursework I; Technical Report; South Kensington Campus: London, UK, November 2015; Available online: https://doi.org/10.13140/RG.2.2.31592.26882 (accessed on 15 April 2020).
- Niku-Paavola, M.L.; Raaska, L.; Itävaara, M. Detection of white-rot fungi by a non-toxic stain. Mycol. Res. 1990, 94, 27–31. [Google Scholar] [CrossRef]
- Nunes, J.K.; Tsai, S.S.H.; Wan, J.; Stone, H.A. Dripping and jetting in microfluidic multiphase flows applied to particle and fibre synthesis. J. Mol. Catal. B Enzym. 2013, 46, 114002. [Google Scholar] [CrossRef]
- Yang, T.; Cingolani, A.; Casalini, T.; Aribia, A.; Klaue, A.; Wu, H.; Stavrakis, S.; de Mello, A.; Morbidelli, M. Reactive Gelation Synthesis of Monodisperse Polymeric Capsules Using Droplet-Based Microfluidics. Adv. Mater. Technol. 2019, 4, 1900092. [Google Scholar] [CrossRef]
- Lee, S.W.; Choi, J.S.; Cho, K.Y.; Yim, J.H. Facile fabrication of uniform-sized, magnetic, and electroconductive hybrid microspheres using a microfluidic droplet generator. Eur. Polym. J. 2016, 80, 40–47. [Google Scholar] [CrossRef]
- Moreno Raja, M.; Lim, P.Q.; Wong, Y.S.; Xiong, G.M.; Zhang, Y.; Venkatraman, S.; Huang, Y. Polymeric Nanomaterials. In Nanocarriers for Drug Delivery; Elsevier: Amsterdam, The Netherlands, 2019; pp. 557–653. [Google Scholar]
- Webber, R.E.; Shull, K.R. Strain dependence of the viscoelastic properties of alginate hydrogels. Macromolecules 2004, 37, 6153–6160. [Google Scholar] [CrossRef]
- Rowley, J.A.; Madlambayan, G.; Mooney, D.J. Alginate hydrogels as synthetic extracellular matrix materials. Biomaterials 1999, 20, 45–53. [Google Scholar] [CrossRef]
- Zhang, D.H.; Zhang, Y.F.; Zhi, G.Y.; Xie, Y.L. Effect of hydrophobic/hydrophilic characteristics of magnetic microspheres on the immobilization of BSA. Colloids Surf., B 2011, 82, 302–306. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Zhang, L.; Wei, Y.; Chao, J.; Shuang, S.; Cai, Z.; Dong, C. A selectively rhodamine-based colorimetric probe for detecting copper(II) ion. Spectrochim. Acta Part A: Mol. Biomol. Spectrosc. 2014, 132, 191–197. [Google Scholar] [CrossRef] [PubMed]
- Bhat, S.S.; Kotian, A.; Shaikh, S.; Hegde, P.L.; Pinjari, R.V.; Revankar, V.K. Highly sensitive and selective colorimetric probe for detection of Cu2+ in aqueous medium based on rhodamine B. Chem. Data Collect. 2020, 26, 100359. [Google Scholar] [CrossRef]
- Jiao, Y.; Zhou, L.; He, H.; Yin, J.; Gao, Q.; Wei, J.; Duan, C.; Peng, X. A novel rhodamine B-based “off-on’’ fluorescent sensor for selective recognition of copper (II) ions. Talanta 2018, 184, 143–148. [Google Scholar] [CrossRef]
- Chang, T.M.S. Encapsulation of Enzymes, Cell Contents, Cells, Vaccines, Antigens, Antiserum, Cofactors, Hormones, and Proteins. Biomed. Appl. Immobil. Enzym. Proteins 1977, 69–90. [Google Scholar] [CrossRef]
- Betancor, L.; Luckarift, H.R. Bioinspired enzyme encapsulation for biocatalysis. Trends Biotechnol. 2008, 26, 566–572. [Google Scholar] [CrossRef]
- Caruso, F.; Trau, D.; Möhwald, H.; Renneberg, R. Enzyme encapsulation in layer-by-layer engineered polymer multilayer capsules. Langmuir 2000, 16, 1485–1488. [Google Scholar] [CrossRef]
- Wang, J.; Eijkel, J.C.T.; Jin, M.; Xie, S.; Yuan, D.; Zhou, G.; van den Berg, A.; Shui, L. Microfluidic fabrication of responsive hierarchical microscale particles from macroscale materials and nanoscale particles. Sensors Actuators B Chem. 2017, 247, 78–91. [Google Scholar] [CrossRef]
- Liu, L.; Yang, J.P.; Ju, X.J.; Xie, R.; Liu, Y.M.; Wang, W.; Zhang, J.J.; Niu, C.H.; Chu, L.Y. Monodisperse core-shell chitosan microcapsules for pH-responsive burst release of hydrophobic drugs. Soft Matter 2011, 7, 4821–4827. [Google Scholar] [CrossRef]
- Soon, C.F.; Yap, H.Y.; Ahmad, M.K.; Sahdan, M.Z.; Tee, K.S.; Khagani, S.A.; Youseffi, M. Environmental friendly flow focusing microfluidic device and pump system for microencapsulation of 3D cells. J. Eng. Appl. Sci. 2017, 12, 6928–6933. [Google Scholar]
- Palmieri, G.; Giardina, P.; Bianco, C.; Fontanella, B.; Sannia, G. Copper induction of laccase isoenzymes in the ligninolytic fungus Pleurotus ostreatus. Appl. Environ. Microbiol. 2000, 66, 920–924. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hernández-Monjaraz, W.S.; Caudillo-Pérez, C.; Salazar-Sánchez, P.U.; Macías-Sánchez, K.L. Influence of iron and copper on the activity of laccases in Fusarium oxysporum f. sp. lycopersici. Braz. J. Microbiol. 2018, 49, 269–275. [Google Scholar] [CrossRef] [PubMed]
- Bédard, M.F.; De Geest, B.G.; Skirtach, A.G.; Möhwald, H.; Sukhorukov, G.B. Polymeric microcapsules with light responsive properties for encapsulation and release. Adv. Colloid Interface Sci. 2010, 158, 2–14. [Google Scholar] [CrossRef] [PubMed]
- Chandrasekar, V.; Coupland, J.N.; Anantheswaran, R.C. Release Kinetics of Nisin from Chitosan-Alginate Complex Films. J. Food Sci. 2016, 81, E2503–E2510. [Google Scholar] [CrossRef] [PubMed]
- Polk, A.; Amsden, B.; De Yao, K.; Peng, T.; Goosen, M.F.A. Controlled release of albumin from chitosan—Alginate microcapsules. J. Pharm. Sci. 1994, 83, 178–185. [Google Scholar] [CrossRef] [PubMed]
- Suzuki, Y.; Nishimura, Y.; Tanihara, M.; Suzuki, K.; Nakamura, T.; Shimizu, Y.; Yamawaki, Y.; Kakimaru, Y. Evaluation of a novel alginate gel dressing: Cytotoxicity to fibroblasts in vitro and foreign-body reaction in pig skin in vivo. J. Biomed. Mater. Res. 1998, 39, 317–322. [Google Scholar] [CrossRef]









© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Campaña, A.L.; Sotelo, D.C.; Oliva, H.A.; Aranguren, A.; Ornelas-Soto, N.; Cruz, J.C.; Osma, J.F. Fabrication and Characterization of a Low-Cost Microfluidic System for the Manufacture of Alginate–Lacasse Microcapsules. Polymers 2020, 12, 1158. https://doi.org/10.3390/polym12051158
Campaña AL, Sotelo DC, Oliva HA, Aranguren A, Ornelas-Soto N, Cruz JC, Osma JF. Fabrication and Characterization of a Low-Cost Microfluidic System for the Manufacture of Alginate–Lacasse Microcapsules. Polymers. 2020; 12(5):1158. https://doi.org/10.3390/polym12051158
Chicago/Turabian StyleCampaña, Ana Lucia, Diana Camila Sotelo, Hector Alfonso Oliva, Andres Aranguren, Nancy Ornelas-Soto, Juan C. Cruz, and Johann F. Osma. 2020. "Fabrication and Characterization of a Low-Cost Microfluidic System for the Manufacture of Alginate–Lacasse Microcapsules" Polymers 12, no. 5: 1158. https://doi.org/10.3390/polym12051158
APA StyleCampaña, A. L., Sotelo, D. C., Oliva, H. A., Aranguren, A., Ornelas-Soto, N., Cruz, J. C., & Osma, J. F. (2020). Fabrication and Characterization of a Low-Cost Microfluidic System for the Manufacture of Alginate–Lacasse Microcapsules. Polymers, 12(5), 1158. https://doi.org/10.3390/polym12051158