Self-Reinforced Nylon 6 Composite for Smart Vibration Damping
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Fabrication of Coiled Fibres
2.3. Fabrication of Self-Reinforced Composites
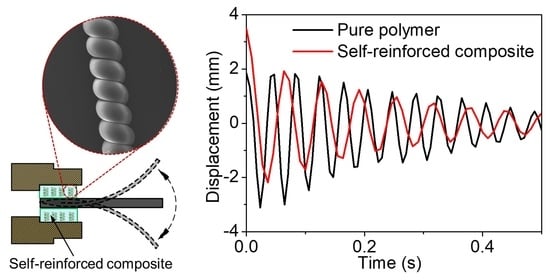
2.4. Vibration Damping Test
3. Results and Discussion
3.1. Properties of Coiled Fibres
3.2. Properties of Self-Reinforced Composites
3.3. Vibration Damping Test Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lu, Y.C.; Shinozaki, D.M. Temperature dependent viscoelastic properties of polymers investigated by small-scale dynamic mechanical analysis. Exp. Mech. 2010, 50, 71–77. [Google Scholar] [CrossRef]
- De Silva, C.W. Vibration: Fundamentals and Practice; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Li, A. Basic principles of energy dissipation and vibration control. In Vibration Control for Building Structures; Springer: Berlin, Germany, 2020; pp. 13–45. [Google Scholar]
- Bhattacharya, B.; Tomlinson, G.J. House, Vibration suppression of structures with viscoelastic inserts, Proceedings of the Institution of Mechanical Engineers. J. Mech. Eng. Sci. 2002, 216, 983–995. [Google Scholar] [CrossRef]
- Chen, J.; Wu, C.; Pu, F.; Chiu, C. Fabrication and mechanical properties of self-reinforced poly (ethylene terephthalate) composites. Express Polym. Lett. 2011, 5, 228–237. [Google Scholar] [CrossRef]
- Deng, M.; Shalaby, S.W. Properties of self-reinforced ultra-high-molecular-weight polyethylene composites. Biomaterials 1997, 18, 645–655. [Google Scholar] [CrossRef]
- Katunin, A. Criticality of the Self-Heating Effect in polymers and polymer matrix composites during fatigue, and their application in non-destructive testing. Polymers 2019, 11, 19. [Google Scholar] [CrossRef] [Green Version]
- de Cazenove, J.; Rade, D.A.; de Lima, A.M.G.; Araújo, C.A. A numerical and experimental investigation on self-heating effects in viscoelastic dampers. Mech. Syst. Signal Process. 2012, 27, 433–445. [Google Scholar] [CrossRef]
- Moisa, S.; Landsberg, G.; Rittel, D.; Halary, J.L. Hysteretic thermal behavior of amorphous semi-aromatic polyamides. Polymer 2005, 46, 11870–11875. [Google Scholar] [CrossRef]
- Chandra, R.; Singh, S.P.; Gupta, K. Damping studies in fiber-reinforced composites—A review. Compos. Struct. 1999, 46, 41–51. [Google Scholar] [CrossRef]
- Chauhan, S.; Karmarkar, A.; Aggarwal, P. Damping behavior of wood filled polypropylene composites. J. Appl. Polym. Sci. 2009, 114, 2421–2426. [Google Scholar] [CrossRef]
- Swolfs, Y.; Crauwels, L.; Gorbatikh, L.; Verpoest, I. The influence of weave architecture on the mechanical properties of self-reinforced polypropylene. Compos. Part A. Appl. Sci. Manuf. 2013, 53, 129–136. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.; Jiang, M.; Zhou, Z.; Gou, D.; Hui, D. 3D printing of polymer matrix composites: A review and prospective. Compos. Part B. Eng. 2017, 110, 442–458. [Google Scholar] [CrossRef]
- Mutlu, R.; Alici, G.; in het Panhuis, M.; Spinks, G.M. 3D Printed flexure hinges for soft monolithic prosthetic fingers. Soft Robot. 2016, 3, 120–133. [Google Scholar] [CrossRef]
- Nikzad, M.; Masood, S.H.; Sbarski, I. Thermo-mechanical properties of a highly filled polymeric composites for Fused Deposition Modeling. Mater. Des. 2011, 32, 3448–3456. [Google Scholar] [CrossRef]
- Masood, S.H. Advances in fused deposition modeling. In Comprehensive Materials Processing; Hashmi, S., Batalha, G.F., van Tyne, C.J., Yilbas, B., Eds.; Elsevier: Oxford, UK, 2014; pp. 69–91. [Google Scholar]
- Dickson, A.N.; Abourayana, H.M.; Dowling, D.P. 3D Printing of fibre-reinforced thermoplastic composites using fused filament fabrication—A review. Polymers 2020, 12, 2188. [Google Scholar] [CrossRef]
- Shofner, M.; Lozano, K.; Rodríguez-Macías, F.J.; Barrera, E.V. Nanofiber-reinforced polymers prepared by fused deposition modeling. J. Appl. Polym. Sci. 2003, 89, 3081–3090. [Google Scholar] [CrossRef]
- Kim, Y.-K.; Bae, H.-I.; Koo, J.-H.; Kim, K.-S.; Kim, S. Note: Real time control of a tunable vibration absorber based on magnetorheological elastomer for suppressing tonal vibrations. Rev. Sci. Instrum. 2012, 83, 046108. [Google Scholar] [CrossRef]
- Haines, C.S.; Lima, M.D.; Li, N.; Spinks, G.M.; Foroughi, J.; Madden, J.D.W.; Kim, S.H.; Fang, S.; Andrade, M.; Göktepe, F.; et al. Artificial muscles from fishing line and sewing thread. Science 2014, 343, 868–872. [Google Scholar] [CrossRef]
- Aziz, S.; Naficy, S.; Foroughi, J.; Brown, H.R.; Spinks, G.M. Controlled and scalable torsional actuation of twisted nylon 6 fiber. J. Polym. Sci. Part B: Polym. Phys. 2016, 54, 1278–1286. [Google Scholar] [CrossRef] [Green Version]
- Aziz, S.; Naficy, S.; Foroughi, J.; Brown, H.R.; Spinks, G.M. Twist–coil coupling fibres for high stroke tensile artificial muscles. Sens. Actuators A. Phys. 2018, 283, 98–106. [Google Scholar] [CrossRef]
- Suzuki, M.; Kamamichi, N. Displacement control of an antagonistic-type twisted and coiled polymer actuator. Smart Mater. Struct. 2018, 27, 035003. [Google Scholar] [CrossRef]
- Aziz, S.; Naficy, S.; Foroughi, J.; Brown, H.R.; Spinks, G.M. Characterisation of torsional actuation in highly twisted yarns and fibres. Polym. Test. 2015, 46, 88–97. [Google Scholar] [CrossRef] [Green Version]
- Khan, S.U.; Li, C.Y.; Siddiqui, N.A.; Kim, J.-K. Vibration damping characteristics of carbon fiber-reinforced composites containing multi-walled carbon nanotubes. Compos. Sci. Technol. 2011, 71, 1486–1494. [Google Scholar] [CrossRef]
- Bendaoud, A.; Pillon, C. Handbook of Thermoplastics; CRC Press: Boca Raton, FL, USA, 2019. [Google Scholar]
- McIntyre, J.E. Synthetic Fibres: Nylon, Polyester, Acrylic, Polyolefin; Elsevier: Amsterdam, The Netherlands, 2005. [Google Scholar]
- Aziz, S.; Naficy, S.; Foroughi, J.; Brown, H.R.; Spinks, G.M. Thermomechanical effects in the torsional actuation of twisted nylon 6 fiber. J. Appl. Polym. Sci. 2017, 134, 45529. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.; Cui, L.; Guan, F.; Gao, Y.; Hedin, N.E.; Zhu, L.; Fong, H. Crystalline morphology and polymorphic phase transitions in electrospun nylon-6 nanofibers. Macromolecules 2007, 40, 6283–6290. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fornes, T.D.; Paul, D.R. Crystallization behavior of nylon 6 nanocomposites. Polymer 2003, 44, 3945–3961. [Google Scholar] [CrossRef]
- Gogolewski, S.; Czerntawska, K.; Gastorek, M. Effect of annealing on thermal properties and crystalline structure of polyamides. Nylon 12 (polylaurolactam). Colloid Polym. Sci. 1980, 258, 1130–1136. [Google Scholar] [CrossRef]
- Murthy, N.S.; Bray, R.G.; Correale, S.T.; Moore, R.A.F. Drawing and annealing of nylon-6 fibres: Studies of crystal growth, orientation of amorphous and crystalline domains and their influence on properties. Polymer 1995, 36, 3863–3873. [Google Scholar] [CrossRef]
- Kousiatza, C.; Chatzidai, N.; Karalekas, D. Temperature mapping of 3D printed polymer plates: Experimental and numerical study. Sensors 2017, 17, 456. [Google Scholar] [CrossRef] [Green Version]
- Sun, Q.; Rizvi, G.M.; Bellehumeur, C.T.; Gu, P. Effect of processing conditions on the bonding quality of FDM polymer filaments. Rapid Prototyp. J. 2008, 14, 72–80. [Google Scholar] [CrossRef]
- Aziz, S.; Spinks, G.M. Torsional artificial muscles. Mater. Horiz. 2020, 7, 667–693. [Google Scholar] [CrossRef]





| Sample | Melting Temperature (°C) | Area of Melting Peak (J/g) | Degree of Crystallinity (%) |
|---|---|---|---|
| As-received | 225.3(±0.08) | 85.23 (±0.21) | 35.7 (±0.05) |
| Coiled: Heat set @ 50 °C | 221.96 (±0.06) | 77.32 (±0.18) | 32.3 (±0.1) |
| Coiled: Heat set @ 100 °C | 221.87 (±0.08) | 78.63 (±0.22) | 32.9 (±0.09) |
| Coiled: Heat set @ 150 °C | 223.47 (±0.1) | 82.43 (±0.27) | 34.5 (±0.1) |
| Coiled: Heat set @ 200 °C | 222.31 (±0.12) | 80.83 (±0.23) | 33.8 (±0.11) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salahuddin, B.; Mutlu, R.; Baigh, T.A.; Alghamdi, M.N.; Aziz, S. Self-Reinforced Nylon 6 Composite for Smart Vibration Damping. Polymers 2021, 13, 1235. https://doi.org/10.3390/polym13081235
Salahuddin B, Mutlu R, Baigh TA, Alghamdi MN, Aziz S. Self-Reinforced Nylon 6 Composite for Smart Vibration Damping. Polymers. 2021; 13(8):1235. https://doi.org/10.3390/polym13081235
Chicago/Turabian StyleSalahuddin, Bidita, Rahim Mutlu, Tajwar A. Baigh, Mohammed N. Alghamdi, and Shazed Aziz. 2021. "Self-Reinforced Nylon 6 Composite for Smart Vibration Damping" Polymers 13, no. 8: 1235. https://doi.org/10.3390/polym13081235
APA StyleSalahuddin, B., Mutlu, R., Baigh, T. A., Alghamdi, M. N., & Aziz, S. (2021). Self-Reinforced Nylon 6 Composite for Smart Vibration Damping. Polymers, 13(8), 1235. https://doi.org/10.3390/polym13081235