A Trefoil Knot Polymer Chain Translocates through a Funnel-like Channel: A Multi-Particle Collision Dynamics Study
Abstract
:1. Introduction
2. Model and Simulation Methods
2.1. Dynamics Behaviors of the Knotted Liner Polymer Chain
2.2. Multi-Particle Collision Dynamics for the Fluid Simulation
2.3. Parameters
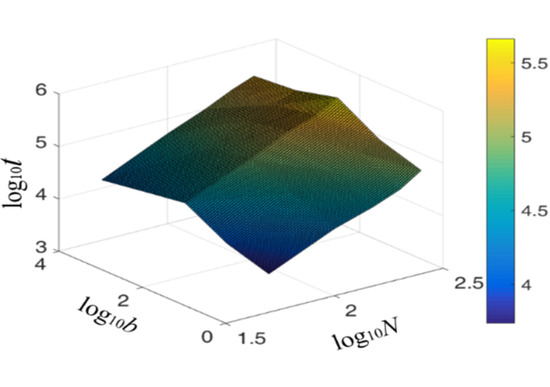
3. Simulation Results and Discussions
3.1. The Poiseuille Flow
3.2. Translocation Conformations of the Polymer Chains
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liebetreu, M.; Ripoll, M.; Likos, C.N. Trefoil Knot Hydrodynamic Delocalization on Sheared Ring Polymers. Acs Macro Lett. 2018, 7, 447–452. [Google Scholar] [CrossRef]
- Gruziel, M.; Thyagarajan, K.; Dietler, G.; Stasiak, A.; Ekiel-Jezewska, M.L.; Szymczak, P. Periodic Motion of Sedimenting Flexible Knots. Phys. Rev. Lett. 2018, 121, 127801. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cardelli, C.; Tubiana, L.; Bianco, V.; Nerattini, F.; Dellago, C.; Coluzza, I. Heteropolymer Design and Folding of Arbitrary Topologies Reveals an Unexpected Role of Alphabet Size on the Knot Population. Macromolecules 2018, 51, 8346–8356. [Google Scholar] [CrossRef]
- Beaton, N.R.; Eng, J.W.; Ishihara, K.; Shimokawa, K.; Soteros, C.E. Characterising knotting properties of polymers in nanochannels. Soft Matter 2018, 14, 5775–5785. [Google Scholar] [CrossRef] [Green Version]
- Richard, D.; Stalter, S.; Siebert, J.T.; Rieger, F.; Trefz, B.; Virnau, P. Entropic Interactions between Two Knots on a Semiflexible Polymer. Polymers 2017, 9, 55. [Google Scholar] [CrossRef] [Green Version]
- Marenz, M.; Janke, W. Knots as a Topological Order Parameter for Semiflexible Polymers. Phys. Rev. Lett. 2016, 116, 128301. [Google Scholar] [CrossRef] [Green Version]
- Soh, B.W.; Klotz, A.R.; Doyle, P.S. Untying of Complex Knots on Stretched Polymers in Elongational Fields. Macromolecules 2018, 51, 9562–9571. [Google Scholar] [CrossRef]
- Klotz, A.R.; Soh, B.W.; Doyle, P.S. Motion of Knots in DNA Stretched by Elongational Fields. Phys. Rev. Lett. 2018, 120, 188003. [Google Scholar] [CrossRef] [Green Version]
- Klotz, A.R.; Narsimhan, V.; Soh, B.W.; Doyle, P.S. Dynamics of DNA Knots during Chain Relaxation. Macromolecules 2017, 50, 4075–4083. [Google Scholar] [CrossRef]
- Michieletto, D.; Marenduzzo, D.; Orlandini, E. Topological patterns in two-dimensional gel electrophoresis of DNA knots. Proc. Natl. Acad. Sci. USA 2015, 112, E5471–E5477. [Google Scholar] [CrossRef] [Green Version]
- Marenda, M.; Orlandini, E.; Micheletti, C. Sorting ring polymers by knot type with modulated nanochannels. Soft Matter 2017, 13, 795–802. [Google Scholar] [CrossRef] [PubMed]
- Zantop, A.W.; Stark, H. Squirmer rods as elongated microswimmers: Flow fields and confinement. Soft Matter 2020, 16, 6400–6412. [Google Scholar] [CrossRef] [PubMed]
- Liebetreu, M.; Likos, C.N. Cluster prevalence in concentrated ring-chain mixtures under shear. Soft Matter 2020, 16, 8710–8719. [Google Scholar] [CrossRef] [PubMed]
- Chakrapani, T.H.; den Otter, W.K. Capillary Imbibition of Binary Fluid Mixtures in Nanochannels. Langmuir 2020, 36, 12712–12722. [Google Scholar] [CrossRef] [PubMed]
- Chelakkot, R.; Winkler, R.G.; Gompper, G. Flow-Induced Helical Coiling of Semiflexible Polymers in Structured Microchannels. Phys. Rev. Lett. 2012, 109, 178101. [Google Scholar] [CrossRef] [Green Version]
- Gompper, G.; Ihle, T.; Kroll, D.M.; Winkler, R.G. Multi-Particle Collision Dynamics: A Particle-Based Mesoscale Simulation Approach to the Hydrodynamics of Complex Fluids. Adv. Polym. Sci. 2008, 221, 1–87. [Google Scholar]
- Malevanets, A.; Kapral, R. Mesoscopic model for solvent dynamics. J. Chem. Phys. 1999, 110, 8605–8613. [Google Scholar] [CrossRef]
- Guo, J.; Li, X.; Liu, Y.; Liang, H. Flow-induced translocation of polymers through a fluidic channel: A dissipative particle dynamics simulation study. J. Chem. Phys. 2011, 134, 134906. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Z.M.; Chien, W.; Henry, E.; Fedosov, D.A.; Gompper, G. Sharp-edged geometric obstacles in microfluidics promote deformability-based sorting of cells. Phys. Rev. Fluids 2019, 4, 024201. [Google Scholar] [CrossRef] [Green Version]
- Singh, S.P.; Gompper, G.; Winkler, R.G. Steady state sedimentation of ultrasoft colloids. J. Chem. Phys. 2018, 148, 084901. [Google Scholar] [CrossRef]
- Das, S.; Riest, J.; Winkler, R.G.; Gompper, G.; Dhont, J.K.G.; Nagele, G. Clustering and dynamics of particles in dispersions with competing interactions: Theory and simulation. Soft Matter 2017, 14, 92–103. [Google Scholar] [CrossRef] [PubMed]
- Lamura, A.; Gompper, G.; Ihle, T.; Kroll, D.M. Multi-particle collision dynamics: Flow around a circular and a square cylinder. Europhys. Lett. 2001, 56, 319–325. [Google Scholar] [CrossRef] [Green Version]
- Huang, C.C.; Chatterji, A.; Sutmann, G.; Gompper, G.; Winkler, R.G. Cell-level canonical sampling by velocity scaling for multiparticle collision dynamics simulations. J. Comput. Phys. 2010, 229, 168–177. [Google Scholar] [CrossRef]
- Huang, C.-C.; Winkler, R.G.; Sutmann, G.; Gompper, G. Semidilute Polymer Solutions at Equilibrium and under Shear Flow. Macromolecules 2010, 43, 10107–10116. [Google Scholar] [CrossRef] [Green Version]







Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wen, X.; Wang, D.; Tang, J.; Yang, Z. A Trefoil Knot Polymer Chain Translocates through a Funnel-like Channel: A Multi-Particle Collision Dynamics Study. Polymers 2022, 14, 1164. https://doi.org/10.3390/polym14061164
Wen X, Wang D, Tang J, Yang Z. A Trefoil Knot Polymer Chain Translocates through a Funnel-like Channel: A Multi-Particle Collision Dynamics Study. Polymers. 2022; 14(6):1164. https://doi.org/10.3390/polym14061164
Chicago/Turabian StyleWen, Xiaohui, Deyin Wang, Jiajun Tang, and Zhiyong Yang. 2022. "A Trefoil Knot Polymer Chain Translocates through a Funnel-like Channel: A Multi-Particle Collision Dynamics Study" Polymers 14, no. 6: 1164. https://doi.org/10.3390/polym14061164
APA StyleWen, X., Wang, D., Tang, J., & Yang, Z. (2022). A Trefoil Knot Polymer Chain Translocates through a Funnel-like Channel: A Multi-Particle Collision Dynamics Study. Polymers, 14(6), 1164. https://doi.org/10.3390/polym14061164