Mechanical Properties, Fractal Dimension, and Texture Analysis of Selected 3D-Printed Resins Used in Dentistry That Underwent the Compression Test
Abstract
:1. Introduction
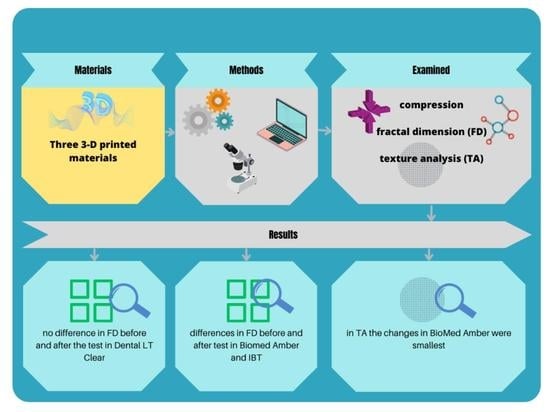
- There are no differences in the mechanical properties of the three 3D-printed materials (BioMed Amber, IBT, and Dental LT Clear).
- All the examined materials react similarly during the compression test.
- There are no differences in FD between each material before the compression test.
- There are no differences in FD between the materials after the compression test.
- There are no differences in FD before and after the compression test.
- There are no differences in TA between the materials after the compression test.
- There are no differences in TA before and after the compression test.
2. Materials and Methods
2.1. Preparation of Samples and the First Test
2.2. Preparation of Photographs
2.3. Fractal Dimension Analysis
2.4. Texture Analysis
2.5. Statistical Analysis
2.5.1. Mechanical Features
2.5.2. FDA
2.5.3. TA
3. Results
3.1. Analysis of Mechanical Properties
3.2. FDA
3.3. Material Surface TA
4. Discussion
5. Limitations
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Małysa, A.; Weżgowiec, J.; Danel, D.; Boening, K.; Walczak, K.; Więckiewicz, M. Bond strength of modern self-adhesive resin cements to human dentin and different CAD/CAM ceramics. Acta Bioeng. Biomech. 2020, 22, 25–34. [Google Scholar] [CrossRef] [PubMed]
- Karatas, O.; Gul, P.; Akgul, N.; Celik, N.; Gundogdu, M.; Duymus, Z.; Seven, N. Effect of staining and bleaching on the microhardness, surface roughness and color of different composite resins. Dent. Med. Probl. 2021, 58, 369–376. [Google Scholar] [CrossRef] [PubMed]
- Skośkiewicz-Malinowska, K.; Mysior, M.; Rusak, A.; Kuropka, P.; Kozakiewicz, M.; Jurczyszyn, K. Application of Texture and Fractal Dimension Analysis to Evaluate Subgingival Cement Surfaces in Terms of Biocompatibility. Materials 2021, 14, 5857. [Google Scholar] [CrossRef] [PubMed]
- Boing, T.F.; De Geus, J.L.; Wambier, L.M.; Loguercio, A.D.; Reis, A.; Gomes, O.M.M. Are Glass-Ionomer Cement Restorations in Cervical Lesions More Long-Lasting than Resin-based Composite Resins? A Systematic Review and Meta-Analysis. J. Adhes Dent. 2018, 20, 435–452. [Google Scholar] [PubMed]
- Kustrzycka, D.; Marschang, T.; Mikulewicz, M.; Grzebieluch, W. Comparison of the Accuracy of 3D Images Obtained fromDif- 474 ferent Types of Scanners: A Systematic Review. J. Health Eng. 2020, 2020, 8854204. [Google Scholar] [CrossRef]
- Wezgowiec, J.; Paradowska-Stolarz, A.; Malysa, A.; Orzeszek, S.; Seweryn, P.; Wieckiewicz, M. Effects of Various Disinfection Methods on the Material Properties of Silicone Dental Impressions of Different Types and Viscosities. Int. J. Mol Sci. 2022, 23, 10859. [Google Scholar] [CrossRef]
- Valenti, C.; Federici, M.I.; Masciotti, F.; Marinucci, L.; Xhimitiku, I.; Cianetti, S.; Pagano, S. Mechanical properties of 3D-printed prosthetic materials compared with milled and conventional processing: A systematic review and meta-analysis of in vitro studies. J. Prosthet. Dent. 2022, 5, S0022-3913(22)00415-2. [Google Scholar] [CrossRef]
- Hamoudi, H.; Berdiyorov, G.R.; Zekri, A.; Tong, Y.; Mansour, S.; Esaulov, V.A.; Youcef-Toumi, K. Building block 3D printing based on molecular self-assembly monolayer with self-healing properties. Sci. Rep. 2022, 12, 6806. [Google Scholar] [CrossRef]
- Pagano, S.; Lombardo, G.; Caponi, S.; Costanzi, E.; Di Michele, A.; Bruscoli, S.; Xhimitiku, I.; Coniglio, M.; Valenti, C.; Mattarelli, M.; et al. Bio-mechanical characterization of a CAD/CAM PMMA resin for digital removable prostheses. Dent. Mater. 2021, 37, e118–e130. [Google Scholar] [CrossRef]
- Miedzińska, D.; Gieleta, R.; Popławski, A. Experimental Study on Influence of Curing Time on Strength Behavior of SLA-Printed Samples Loaded with Different Strain Rates. Materials 2020, 13, 5825. [Google Scholar] [CrossRef]
- Minervino, B.L.; Barriviera, M.; Curado, M.M.; Gandini, L.G. MARPE Guide: A Case Report. J. Contemp. Dent. Pract. 2019, 20, 1102–1107. [Google Scholar] [PubMed]
- Alhroob, K.; Alsabbagh, M.M.; Alsabbagh, A.Y. Effect of the use of a surgical guide on heat generation during implant placement: A comparative in vitro study. Dent. Med. Probl. 2021, 58, 55–59. [Google Scholar] [CrossRef] [PubMed]
- Paradowska-Stolarz, A.; Malysa, A.; Mikulewicz, M. Comparison of the Compression and Tensile Modulus of Two Chosen Resins Used in Dentistry for 3D Printing. Materials 2022, 15, 8956. [Google Scholar] [CrossRef] [PubMed]
- Armi, L.; Fekri-Ershad, S. Texture Analysis and texture classification methods—A review. Int. Online J. Image Process. Pattern Recognit. 2019, 2, 1–29. [Google Scholar]
- Castellano, G.; Bonilha, L.; Li, L.; Cendes, F. Texture analysis of medical images. Clin. Radiol. 2004, 59, 1061–1069. [Google Scholar] [CrossRef] [PubMed]
- Gonçalves, B.C.; De Araújo, E.C.; Nussi, A.D.; Bechara, N.; Sarmento, D.; Oliveira, M.S.; Santamaria, M.P.; Costa, A.L.F.; Lopes, S. Texture analysis of cone-beam computed tomography images assists the detection of furcal lesion. J. Periodontol. 2020, 91, 1159–1166. [Google Scholar] [CrossRef]
- Lee, K.M.; Kim, H.G.; Lee, Y.H.; Kim, E.J. mDixon-based texture analysis of an intraosseous lipoma: A case report and current review for the dental clinician. Oral Surg. Oral Med. Oral Pathol. Oral Radiol. 2018, 125, e67–e71. [Google Scholar] [CrossRef]
- Jatti, A.; Joshi, R. Characterization of dental pathologies using digital panoramic X-ray images based on texture analysis. In Proceedings of the 39th Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC) 2017, Jeju Island, Korea, 11–15 July 2017; pp. 592–595. [Google Scholar]
- Sarul, M.; Kozakiewicz, M.; Jurczyszyn, K. Surface Evaluation of Orthodontic Wires Using Texture and Fractal Dimension Analysis. Materials 2021, 14, 3688. [Google Scholar] [CrossRef]
- Jurczyszyn, K.; Kubasiewicz-Ross, P.; Nawrot-Hadzik, I.; Gedrange, T.; Dominiak, M.; Hadzik, J. Fractal dimension analysis a supplementary mathematical method for bone defect regeneration measurement. Ann. Anat. 2018, 219, 83–88. [Google Scholar] [CrossRef]
- Mishra, S.; Kumar, M.; Mishra, L.; Mohanty, R.; Nayak, R.; Das, A.C.; Mishra, S.; Panda, S.; Lapinska, B. Fractal Dimension as a Tool for Assessment of Dental Implant Stability-A Scoping Review. J. Clin. Med. 2022, 11, 4051. [Google Scholar] [CrossRef]
- Suer, B.T.; Yaman, Z.; Büyüksaraç, B. Correlation of Fractal Dimension Values with Implant Insertion Torque and Resonance Frequency Values at Implant Recipient Sites. Int. J. Oral Maxillofac. Implants 2016, 31, 55–62. [Google Scholar] [CrossRef] [PubMed]
- Trafalski, M.; Kozakiewicz, M.; Jurczyszyn, K. Application of Fractal Dimension and Texture Analysis to Evaluate the Effectiveness of Treatment of a Venous Lake in the Oral Mucosa Using a 980 Nm Diode Laser—A Preliminary Study. Materials 2021, 14, 4140. [Google Scholar] [CrossRef] [PubMed]
- Grzebieluch, W.; Kowalewski, P.; Grygier, D.; Rutkowska-Gorczyca, M.; Kozakiewicz, M.; Jurczyszyn, K. Printable and Machinable Dental Restorative Composites for CAD/CAM Application-Comparison of Mechanical Properties, Fractographic, Texture and Fractal Dimension Analysis. Materials 2021, 14, 4919. [Google Scholar] [CrossRef] [PubMed]
- PN-EN ISO 604:2006; PLASTICS—DETERMINATION OF COMPRESSIVE PROPERTIES. Polish Committee for Standardization: Warsaw, Poland, 2006.
- Szczypiński, P.M.; Strzelecki, M.; Materka, A.; Klepaczko, A. MaZda—A software package for image texture analysis. Comput. Methods Programs Biomed. 2009, 94, 66–76. [Google Scholar] [CrossRef] [PubMed]
- Kozakiewicz, M. Measures of Corticalization. J. Clin. Med. 2022, 11, 5463. [Google Scholar] [CrossRef] [PubMed]
- Kołaciński, M.; Kozakiewicz, M.; Materka, A. Textural Entropy as a Potential Feature for Quantitative Assessment of Jaw Bone Healing Process. Arch. Med. Sci. 2015, 11, 78–84. [Google Scholar] [CrossRef]
- Kozakiewicz, M.; Wach, T. New Oral Surgery Materials for Bone Reconstruction—A Comparison of Five Bone Substitute Materials for Dentoalveolar Augmentation. Materials 2020, 13, 2935. [Google Scholar] [CrossRef]
- Stajcic, I.; Stajcic, A.; Serpa, C.; Vasiljevic-Radovic, D.; Randjelovic, B.; Radojevic, V.; Fecht, H. Microstructure of Epoxy-Based Composites: Fractal Nature Analysis. Fractal Fract. 2022, 6, 741. [Google Scholar] [CrossRef]
- Sabbagh, H.; Khazaei, Y.; Baumert, U.; Hoffmann, L.; Wichelhaus, A.; Rankovic, M.J. Bracket Transfer Accuracy with the Indirect Bonding Technique-A Systematic Review and Meta-Analysis. J. Clin. Med. 2022, 11, 2568. [Google Scholar] [CrossRef]
- Bozhkova, T.; Shopova, D. T-Scan Novus System in the Management of Splints—Pilot Study. Eur. J. Dent. 2022, 16, 454–457. [Google Scholar] [CrossRef]
- Franchi, L.; Vichi, A.; Marti, P.; Lampus, F.; Guercio, S.; Recupero, A.; Giuntini, V.; Goracci, C. 3D Printed Customized Facemask for Maxillary Protraction in the Early Treatment of a Class III Malocclusion: Proof-of-Concept Clinical Case. Materials 2022, 15, 3747. [Google Scholar] [CrossRef] [PubMed]
- Jindal, P.; Juneja, M.; Siena, F.L.; Bajaj, D.; Breedon, P. Mechanical and geometric properties of thermoformed and 3D printed clear dental aligners. Am. J. Orthod. Dentofac. Orthop. 2019, 156, 694–701. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kawala, M.; Smardz, J.; Adamczyk, L.; Grychowska, N.; Więckiewicz, M. Selected Applications for Current Polymers in Prosthetic Dentistry—State of the Art. Curr. Med. Chem. 2018, 25, 6002–6012. [Google Scholar] [CrossRef] [PubMed]
- Layman, B. Digital Bracket Placement for Indirect Bonding. J. Clin. Orthod. 2019, 53, 387–396. [Google Scholar]
- Chen, P.; Nikoyan, L. Guided Implant Surgery: A Technique Whose Time Has Come. Dent. Clin. N. Am. 2021, 65, 67–80. [Google Scholar] [CrossRef]
- Pamula, E.; Cholewa-Kowalska, K.; Szuta, M.; Osyczka, A. Bioactive Glasses as Composite Components: Technological Advantages and Bone Tissue Engineering Applications. In Biomaterials and Stem Cells in Regenerative Medicine, 1st ed.; Ramalingam, M., Rama-Krishna, S., Best, S., Eds.; CRC Press: Boca Raton, FL, USA, 2012; pp. 239–258. ISBN 9780429066658. [Google Scholar] [CrossRef]
- Abdulhameed, E.A.; Al-Rawi, N.H.; Uthman, A.; Samsudin, A.R. Bone Texture Fractal Dimension Analysis of Ultrasound-Treated Bone around Implant Site: A Double-Blind Clinical Trial. Int. J. Dent. 2018, 15, 2672659. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yasar, F.; Akgünlü, F. Fractal dimension and lacunarity analysis of dental radiographs. Dentomaxillofac Radiol. 2005, 34, 261–267. [Google Scholar] [CrossRef]
- Mo, Y.; Yue, S.; Zhou, Q.; Feng, B.; Liu, X. Dynamic Properties and Fractal Characteristics of 3D Printed Cement Mortar in SHPB Test. Materials 2021, 14, 5554. [Google Scholar] [CrossRef]
- Chen, Y.; Li, J.; Lu, J.; Ding, M.; Chen, Y. Synthesis and properties of poly (vinyl alcohol) hydrogels with high strength and toughness. Polym. Test. 2022, 108, 107516. [Google Scholar] [CrossRef]
- Rahmatabadi, D.; Aberoumand, M.; Soltanmohammadi, K.; Soleyman, E.; Ghasemi, I.; Baniassadi, M.; Abrinia, K.; Bodaghi, M.; Baghani, M. 4D Printing Encapsulated Polycaprolactone-Thermoplastic Polyurethane with High Shape Memory Performances. Adv. Eng. Mater. 2022, 25, 2201309. [Google Scholar] [CrossRef]
- Ahmadi, M.; Tabary, S.A.A.B.; Rahmatabadi, D.; Ebrahimi, M.S.; Abrinia, K.; Hashemi, R. Review of selective laser melting of magnesium alloys: Advantages, microstructure and mechanical characterizations, defects, challenges, and applications. J. Mater. Res. Technol. 2022, 19, 1537–1562. [Google Scholar] [CrossRef]
- Rahmatabadi, D.; Aberoumand, M.; Soltanmohammadi, K.; Soleyman, E.; Ghasemi, I.; Baniassadi, M.; Abrinia, K.; Zolfagharian, A.; Bodaghi, M.; Baghani, M. A New Strategy for Achieving Shape Memory Effects in 4D Printed Two-Layer Composite Structures. Polymers 2022, 14, 5446. [Google Scholar] [CrossRef] [PubMed]




| Variable | ||||
|---|---|---|---|---|
| Material | N | W | p | |
| height_mean [mm] | Dental LT Clear | 10 | 0.87 | 0.101598 |
| width_mean [mm] | 0.96 | 0.823717 | ||
| F [N] | 0.91 | 0.286673 | ||
| ΔL [mm] | 0.86 | 0.070812 | ||
| L [mm] | ||||
| nominal strain [%] | 0.86 | 0.070812 | ||
| compression modulus [MPa] | 0.94 | 0.510467 | ||
| height_mean [mm] | BioMed Amber | 10 | 0.92 | 0.345571 |
| width_mean [mm] | 0.95 | 0.621571 | ||
| F [N] | 0.97 | 0.894680 | ||
| Δ L [mm] | 0.97 | 0.869527 | ||
| L [mm] | 0.37 | 0.000000 | ||
| nominal strain [%] | 0.97 | 0.871362 | ||
| compression modulus [MPa] | 0.91 | 0.292340 | ||
| height_mean [mm] | IBT | 10 | 0.96 | 0.762307 |
| width_mean [mm] | 0.87 | 0.098586 | ||
| F [N] | 0.90 | 0.244912 | ||
| ΔL [mm] | 0.97 | 0.882997 | ||
| L [mm] | ||||
| nominal strain [%] | 0.97 | 0.882977 | ||
| compression modulus [MPa] | 0.82 | 0.026361 | ||
| Young’s Modulus (GPa) | Material | p-Value | ||
|---|---|---|---|---|
| Dental LT Clear | BioMed Amber | IBT | ||
| N = 10 | N = 10 | N = 10 | ||
| Compression | <0.001 | |||
| Mean ± SD | 2.46 ± 0.14 | 2.73 ± 0.36 | 0.69 ± 0.021 | |
| Me [Q1; Q3] | 2.44 [2.39; 2.53] | 2.78 [2.60; 2.98] | 0.69 [0.677; 0.706] | |
| Min–Max | 2.26–2.68 | 1.92–3.17 | 0.674–0.744 | |
| Kruskal-Wallis Test: H (2, N = 30) = 22.16516 p = 0.0000 | ||||
|---|---|---|---|---|
| No. | Number of Probes | Sum of Ranks | Mean Rank | |
| Dental LT Clear | 1 | 10 | 172.0000 | 17.20000 |
| BioMed Amber | 2 | 10 | 238.0000 | 23.80000 |
| IBT | 3 | 10 | 55.0000 | 5.50000 |
| Variable | SS Effect | df Effect | MS Effect | SS Error | df Error | MS Error | F | p |
|---|---|---|---|---|---|---|---|---|
| Height mean | 0.000207 | 2 | 0.000104 | 0.002718 | 27 | 0.000101 | 1.029291 | 0.370857 |
| Width mean | 0.005165 | 2 | 0.002583 | 0.017174 | 27 | 0.000636 | 4.060209 | 0.028731 |
| F [N] | 0.768667 | 2 | 0.384333 | 2.166000 | 27 | 0.080222 | 4.790859 | 0.016571 |
| ΔL [mm] | 0.000240 | 2 | 0.000120 | 0.002367 | 27 | 0.000088 | 1.371349 | 0.270882 |
| Nominal strain [%] | 0.116510 | 2 | 0.058255 | 1.238218 | 27 | 0.045860 | 1.270281 | 0.297001 |
| Height Mean | Width Mean | F [N] | ΔL [mm] | Nominal Strain [%] | |
|---|---|---|---|---|---|
| SS effect | 0.1096 | 0.0205 | 0.7247 | 0.4856 | 260.6316 |
| Df effect | 2 | 2 | 2 | 2 | 2 |
| MS effect | 0.0548 | 0.0102 | 0.3623 | 0.2428 | 130.3158 |
| SS error | 0.006262 | 0.042954 | 4.785000 | 0.005867 | 3.049181 |
| df error | 27 | 27 | 27 | 27 | 27 |
| MS error | 0.000232 | 0.001591 | 0.177222 | 0.000217 | 0.112933 |
| F | 236.378 | 6.432 | 2.045 | 1117.478 | 1153.925 |
| p | 0.000000 | 0.005195 | 0.149010 | 0.000000 | 0.000000 |
| df Welch effect | 2 | 2 | 2 | 2 | 2 |
| df Welch error | 16.59621 | 14.81115 | 13.17062 | 16.89735 | 16.95062 |
| F Welch | 260.633 | 9.611 | 6.095 | 1072.963 | 1084.491 |
| p Welch | 0.000000 | 0.002110 | 0.013366 | 0.000000 | 0.000000 |
| Dental LT Clear M = 3.85 mm, SD = 0.01 | BioMed Amber M = 4.00 mm, SD = 0.02 | IBT M = 3.93 mm, SD = 0.01 | |
|---|---|---|---|
| Dental LT Clear | p = 0.000127 | p = 0.000127 | |
| BioMed Amber | p = 0.000127 | p = 0.000127 | |
| IBT | p = 0.000127 | p = 0.000127 |
| Dental LT Clear M = 10.07 mm, SD = 0.03 | BioMed Amber M = 10.11 mm, SD = 0.02 | IBT M = 10.05 mm, SD = 0.06 | |
|---|---|---|---|
| Dental LT Clear | p = 0.104833 | p = 0.332215 | |
| BioMed Amber | p = 0.104822 | p = 0.003916 | |
| IBT | p = 0.332215 | p = 0.003916 |
| Dental LT Clear M = 249.65 N, SD = 0.64 | BioMed Amber M = 249.48 N, SD = 0.33 | IBT M = 249.86 N, SD = 0.10 | |
|---|---|---|---|
| Dental LT Clear | p = 0.643187 | p = 0.513133 | |
| BioMed Amber | p = 0.643187 | p = 0.127154 | |
| IBT | p = 0.513133 | p = 0.127154 |
| Dental LT Clear M = 0.098 mm, SD = 0.010 | BioMed Amber M = 0.05 mm, SD = 0.018 | IBT M = 0.35 mm, SD = 0.015 | |
|---|---|---|---|
| Dental LT Clear | p = 0.000129 | p = 0.000127 | |
| BioMed Amber | p = 0.000129 | p = 0.000127 | |
| IBT | p = 0.000127 | p = 0.000127 |
| Dental LT Clear M = 2.27%, SD = 0.23 | BioMed Amber M = 1.31%, SD = 0.41 | IBT M = 7.98%, SD = 0.35 | |
|---|---|---|---|
| Dental LT Clear | p = 0.000128 | p = 0.000127 | |
| BioMed Amber | p = 0.000128 | p = 0.000127 | |
| IBT | p = 0.000127 | p = 0.000127 |
| Dental LT Clear M = 1.5864, SD = 0.0204 | BioMed Amber M = 1.5689, SD = 0.0169 | IBT M = 1.5766 SD = 0.0168 | |
|---|---|---|---|
| Dental LT Clear | p = 0.003082 | p = 0.070650 | |
| BioMed Amber | p = 0.003082 | p = 0.156513 | |
| IBT | p = 0.070650 | p = 0.156513 |
| Dental LT Clear M = 1.5860, SD = 0.0181 | BioMed Amber M = 1.5570, SD = 0.0169 | IBT M = 1.5515, SD = 0.0220 | |
|---|---|---|---|
| Dental LT Clear | p = 0.000016 | p = 0.000000 | |
| BioMed Amber | p = 0.000016 | p = 0.318011 | |
| IBT | p = 0.000000 | p = 0.318011 |
| Material | FD before Compression | FD after Compression | t | p |
|---|---|---|---|---|
| BioMed Amber | 1.5689 | 1.5570 | 2.22 | 0.0326 |
| Dental LT Clear | 1.5864 | 1.5860 | 0.07 | 0.9409 |
| IBT | 1.5766 | 1.5515 | 4.87 | 0.0000 |
| Material | Average | SD | p < 0.05 |
|---|---|---|---|
| Amber_before | 0.6390 | 0.0410 | 2, 3 |
| Dental LT_before | 0.6824 | 0.0304 | 1 |
| IBT_before | 0.6785 | 0.0676 | 1 |
| Material | Average | SD | p < 0.05 |
|---|---|---|---|
| BioMed Amber_after | 0.6341 | 0.0482 | 3 |
| Dental LT_after | 0.6894 | 0.0418 | 3 |
| IBT_after | 0.4994 | 0.1014 | 1, 2 |
| Material | Before (BI) | After (BI) | t | p |
|---|---|---|---|---|
| BioMed Amber | 0.6390 | 0.6341 | 0.3440 | 0.7227 |
| Dental LT | 0.6824 | 0.6895 | −0.6071 | 0.5474 |
| IBT | 0.6785 | 0.4994 | 7.8289 | 0.0000 |
| Material | BI Before Compression | BI After Compression | U | p |
|---|---|---|---|---|
| BioMed Amber | M = 0.6390 (R = 420) | M = 0.63413 (R = 400) | 190 | 0.7972 |
| Dental LT Clear | M = 0.6825 (R = 365) | M = 0.6895 (R = 455) | 155 | 0.2287 |
| IBT | M = 0.6785 (R = 1216) | M = 0.4994 (R = 737) | 71 | 0.0000 |
| Resin | Application |
|---|---|
| Dental LT Clear |
|
| BioMed Amber |
|
| IBT |
|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Paradowska-Stolarz, A.; Wieckiewicz, M.; Kozakiewicz, M.; Jurczyszyn, K. Mechanical Properties, Fractal Dimension, and Texture Analysis of Selected 3D-Printed Resins Used in Dentistry That Underwent the Compression Test. Polymers 2023, 15, 1772. https://doi.org/10.3390/polym15071772
Paradowska-Stolarz A, Wieckiewicz M, Kozakiewicz M, Jurczyszyn K. Mechanical Properties, Fractal Dimension, and Texture Analysis of Selected 3D-Printed Resins Used in Dentistry That Underwent the Compression Test. Polymers. 2023; 15(7):1772. https://doi.org/10.3390/polym15071772
Chicago/Turabian StyleParadowska-Stolarz, Anna, Mieszko Wieckiewicz, Marcin Kozakiewicz, and Kamil Jurczyszyn. 2023. "Mechanical Properties, Fractal Dimension, and Texture Analysis of Selected 3D-Printed Resins Used in Dentistry That Underwent the Compression Test" Polymers 15, no. 7: 1772. https://doi.org/10.3390/polym15071772
APA StyleParadowska-Stolarz, A., Wieckiewicz, M., Kozakiewicz, M., & Jurczyszyn, K. (2023). Mechanical Properties, Fractal Dimension, and Texture Analysis of Selected 3D-Printed Resins Used in Dentistry That Underwent the Compression Test. Polymers, 15(7), 1772. https://doi.org/10.3390/polym15071772