Formation and Dissolution of γ’ Precipitates in IN792 Superalloy at Elevated Temperatures
Abstract
:1. Introduction
2. Material and Methods
2.1. Specimens and Thermo-Mechanical Treatment
2.2. SANS Technique
3. Results
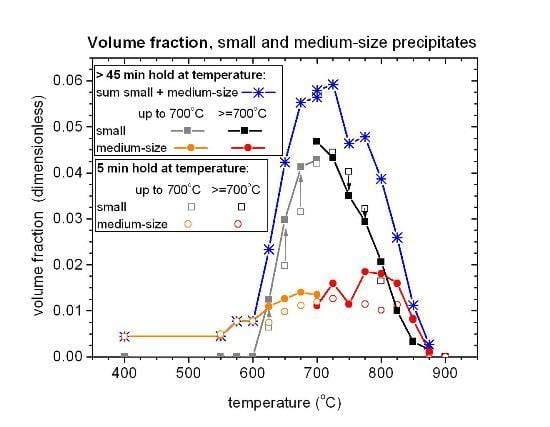
3.1. Formation of Precipitates
3.2. Evolution and Dissolution of Precipitates between 700 and 900 °C
3.3. Dissolution of Precipitates at and Above 900 °C
4. Evaluation and Discussion
4.1. Model for Evaluation
4.2. Volume Fraction
4.3. Formation and Dissolution of the Precipitates
4.4. Strengthening by Tertiary Precipitates
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Ross, E.W.; Sims, C.T. Nickel-Base Alloys. In Superalloys II: High-Temperature Materials for Aerospace and Industrial Power, 2nd ed.; Sims, C.T., Stoloff, N.S., Hagel, W.C., Eds.; John Wiley and Sons: New York, NY, USA, 1987; Part 2, Chapter 4; pp. 97–134. [Google Scholar]
- Reed, R.C. The Superalloys, Fundamentals and Applications; Cambridge University Press: New York, NY, USA, 2006. [Google Scholar]
- Socrate, S.; Parks, D.M. Numerical determination of the elastic driving force for directional coarsening in Ni-superalloys. Acta Metall. 1993, 41, 2185–2209. [Google Scholar] [CrossRef]
- Strunz, P.; Petrenec, M.; Gasser, U.; Tobiáš, J.; Polák, J.; Šaroun, J. Precipitate microstructure evolution in exposed IN738LC superalloy. J. Alloy. Compd. 2014, 589, 462–471. [Google Scholar] [CrossRef]
- Kostorz, G. Small-Angle Scattering and Its Applications to Materials Science. In Neutron Scattering: Treatise on Materials Science and Technology; Kostorz, G., Ed.; Academic Press: New York, NY, USA, 1979; pp. 227–289. [Google Scholar]
- Kozar, R.W.; Suzuki, A.; Milligan, W.W.; Schirra, J.J.; Savage, M.F.; Pollock, T.M. Strengthening mechanisms in polycrystalline multimodal nickel-base superalloys. Metallurgical and Mater. Trans. A 2009, 40, 1588–1603. [Google Scholar] [CrossRef]
- Galindo-Nava, E.I.; Connor, L.D.; Rae, C.M.F. On the prediction of the yield stress of unimodal and multimodal gamma’ Nickel-base superalloys. Acta Mater. 2015, 98, 377–390. [Google Scholar] [CrossRef]
- Rogante, M.; Lebedev, V.T. Small angle neutron scattering comparative investigation of Udimet 520 and Udimet 720 samples submitted to different ageing treatments. J. Alloy. Compd. 2012, 513, 510–517. [Google Scholar] [CrossRef]
- Strunz, P.; Zrník, J.; Epishin, A.; Link, T.; Balog, S. Microstructure of creep-exposed single crystal nickel base superalloy CSMX4. J. Phys.: Conf. Ser. 2010, 247. [Google Scholar] [CrossRef]
- Veron, M.; Bastie, P. Strain induced directional coarsening in nickel based superalloys: Investigation on kinetics using the small angle neutron scattering (SANS) technique. Acta Mater. 1997, 45, 3277–3282. [Google Scholar] [CrossRef]
- Miller, R.J.R.; Messoloras, S.; Stewart, R.J.; Kostorz, G. Small-angle neutron-scattering study of temperature and stress dependence of microstructure of Nimonic alloys. J. Appl. Cryst. 1978, 11, 583–588. [Google Scholar] [CrossRef]
- Mukherji, D.; Del Genovese, D.; Strunz, P.; Gilles, R.; Wiedenmann, A.; Rösler, J. Microstructural characterisation of a Ni-Fe-based superalloy by in situ small-angle neutron scattering measurements. J. Phys.: Condens. Matter. 2008, 20. [Google Scholar] [CrossRef]
- Zickler, G.A.; Schnitzer, R.; Radis, R.; Hochfellner, R.; Schweins, R.; Stockinger, M.; Leitner, H. Microstructure and mechanical properties of the superalloy ATI Allvac® 718Plus™. Mater. Sci. Eng. A 2009, 523, 295–303. [Google Scholar] [CrossRef]
- Strunz, P.; Schumacher, G.; Klingelhöffer, H.; Wiedenmann, A.; Šaroun, J.; Keiderling, U. In situ observation of morphological changes of γ’ precipitates in a pre-deformed single-crystal Ni-base superalloy. J. Appl. Cryst. 2011, 44, 935–944. [Google Scholar] [CrossRef]
- Collins, D.M.; Stone, H.J. A modelling approach to yield strength optimisation in a nickel-base superalloy. Int. J. Plast. 2014, 54, 96–112. [Google Scholar] [CrossRef]
- Petrenec, M.; Obrtlík, K.; Polák, J.; Kruml, T. Fatigue behaviour of cast nickel based superalloy Inconel 792–5A at 700 °C. Mater. Technol. 2006, 40, 175–178. [Google Scholar]
- Dahl, K.V.; Hald, J. Identification of Precipitates in an IN792 Gas Turbine Blade after Service Exposure. Pract. Metallogr. 2013, 50, 432–450. [Google Scholar] [CrossRef]
- Petrenec, M.; Obrtlík, K.; Polák, J.; Man, J. Dislocation structures in nickel based superalloy Inconel 792–5A fatigued at room temperature and 700 °C. Mater. Sci. Forum 2008, 567–568, 429–432. [Google Scholar] [CrossRef]
- Podhorská, B.; Kudrman, J.; Hrbáček, K. Tepelné zpracování, mechanické vlastnosti a strukturní stabilita perspektivních litých niklových superslitin (in Czech). In METAL 2004, Proceedings of the 13th International Metallurgical & Material Conference, Hradec nad Moravicí, Czech Republic, May 2004; Tanger: Ostrava, Czech Republic, 2004; pp. 101–111. [Google Scholar]
- Strunz, P.; Petrenec, M.; Gasser, U. Precipitate microstructure evolution in low-cycle fatigued Inconel superalloys. In Proceedings of the 15th International Small-Angle Scattering Conference; Sydney, Australia, 18–23 November 2012, McGillivary, D., Trewhella, J., Gilbert, E.P., Hanley, T.L., Eds.; Australian Nuclear Science and Technology Organization: Lucas Heights, New South Wales, Australia; 1921268158P039, Metals and Metallic Materials Symposium. Available online: http://trove.nla.gov.au/version/197519597 (accessed on 1 December 2015).
- Strunz, P.; Mortensen, K.; Janssen, S. SANS-II at SINQ: Installation of the former Risø-SANS facility. Phys. B 2004, 350, e783–e785. [Google Scholar] [CrossRef]
- Strunz, P.; Šaroun, J.; Mikula, P.; Lukáš, P.; Eichhorn, F. Double-Bent-Crystal Small-Angle Neutron Scattering setting and its applications. J. Appl. Cryst. 1997, 30, 844–848. [Google Scholar] [CrossRef]
- Strunz, P.; Šaroun, J.; Keiderling, U.; Wiedenmann, A.; Przenioslo, R. General formula for determination of cross-section from measured SANS intensities. J. Appl. Cryst. 2000, 33, 829–833. [Google Scholar] [CrossRef]
- Strunz, P.; Gilles, R.; Mukherji, D.; Wiedenmann, A. Evaluation of anisotropic small-angle neutron scattering data; a faster approach. J. Appl. Cryst. 2003, 36, 854–859. [Google Scholar] [CrossRef]
- Kohlbrecher, J. SASfit: A Program for Fitting Simple Structural Models to Small Angle Scattering Data. 2014. Available online: https://kur.web.psi.ch/sans1/SANSSoft/sasfit.html, https://kur.web.psi.ch/sans1/SANSSoft/sasfit.pdf (accessed on 1 December 2015).










| Cr | Mo | C | Co | Fe | Zr | Nb | Al | B | Ti | Ta | W | Ni | SLD (109 cm−2) | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| alloy | 12.28 | 1.81 | 0.078 | 8.87 | 0.16 | 0.031 | 0.1 | 3.36 | 0.015 | 3.98 | 4.12 | 4.1 | rest | 64.1 |
| γ´ | 3.48 | 4.92 | 4.61 | 7.17 | 7.17 | 2.56 | rest | 66.8 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Strunz, P.; Petrenec, M.; Polák, J.; Gasser, U.; Farkas, G. Formation and Dissolution of γ’ Precipitates in IN792 Superalloy at Elevated Temperatures. Metals 2016, 6, 37. https://doi.org/10.3390/met6020037
Strunz P, Petrenec M, Polák J, Gasser U, Farkas G. Formation and Dissolution of γ’ Precipitates in IN792 Superalloy at Elevated Temperatures. Metals. 2016; 6(2):37. https://doi.org/10.3390/met6020037
Chicago/Turabian StyleStrunz, Pavel, Martin Petrenec, Jaroslav Polák, Urs Gasser, and Gergely Farkas. 2016. "Formation and Dissolution of γ’ Precipitates in IN792 Superalloy at Elevated Temperatures" Metals 6, no. 2: 37. https://doi.org/10.3390/met6020037
APA StyleStrunz, P., Petrenec, M., Polák, J., Gasser, U., & Farkas, G. (2016). Formation and Dissolution of γ’ Precipitates in IN792 Superalloy at Elevated Temperatures. Metals, 6(2), 37. https://doi.org/10.3390/met6020037