Cavity Behavior of Fine-Grained 5A70 Aluminum Alloy during Superplastic Formation
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Superplastic Tensile Tests
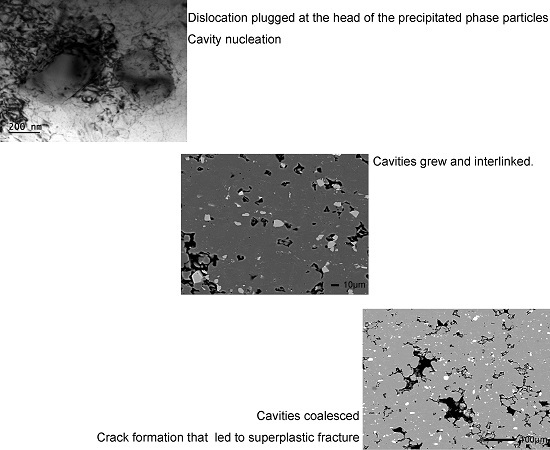
3.2. Cavity Nucleation
3.3. Cavity Growth
3.3.1. Cavity Growth Controlled by Diffusion and Plastic
3.3.2. Effect of Superplastic Diffusion on Cavity Growth
3.3.3. Effect of the Fine-Grained Structure on Cavity Growth
3.4. Crack Formation
4. Conclusions
- (1)
- Strain hardening occurs in fine-grained 5A70 alloys in the superplastic tensile state at temperatures ranging from 400 to 550 °C, when the strain rate is 1 × 10−3 s−1.
- (2)
- The dislocation density of the fine-grained 5A70 alloy was ~1014 m2, which piled up at the head of the second phase particles and the grain boundaries, resulting in the increase of true stress and the nucleation of the cavities.
- (3)
- Cavity growth mainly occurred at the stage of diffusion growth and superplastic diffusion growth due to the diffusion of the voids into adjacent cavities with a cavity radius smaller than the grain size.
- (4)
- Plastic-controlled growth dominated the cavity interlinkage and coalescence process, which eventually led to superplastic fractures.
- (5)
- At 500 and 550 °C, the abnormal grain growth of FG 5A70 alloy during dynamic recrystallization was higher than 400 and 450 °C due to the decrease in Mg-rich phase particles and the Helmholtz free energies.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bolt, P.J.; Lamboo, N.A.P.M.; Rozier, P.J.C.M. Feasibility of warm drawing of aluminium products. J. Mater. Process. Technol. 2001, 115, 118–121. [Google Scholar] [CrossRef]
- Naka, T.; Torikai, G.; Hino, R.; Yoshida, F. The effects of temperature and forming speed on the forming limit diagram for type 5083 aluminum–magnesium alloy sheet. J. Mater. Process. Technol. 2001, 113, 648–653. [Google Scholar] [CrossRef]
- Tokuda, M.; Inaba, T.; Ohigashi, H.; Kurakake, A. Discussions on constitutive equations of superplastic 5083 aluminum alloy. Int. J. Mech. Sci. 2001, 43, 2035–2046. [Google Scholar] [CrossRef]
- Beijing Institute of Aeronautical Materials Group. Materials Technology of Aeronautics; Aviation Industry Press: Beijing, China, 2014; pp. 131–143. ISBN 978-7-5165-0298-3. [Google Scholar]
- Smolej, A.; Klobčar, D.; Skaza, B.; Nagode, A.; Slaček, E.; Dragojević, V.; Smolej, S. Superplasticity of the rolled and friction stir processed Al–4.5 Mg–0.35Sc–0.15Zr alloy. Mater. Sci. Eng. A 2014, 590, 239–245. [Google Scholar] [CrossRef]
- Charit, I.; Mishra, R.S. Evaluation of microstructure and superplasticity in friction stir processed 5083 Al alloy. J. Mater. Res. 2004, 19, 3329–3342. [Google Scholar] [CrossRef]
- Lee, Y.B.; Shin, D.H.; Park, K.T.; Nam, W.J. Effect of annealing temperature on microstructures and mechanical properties of a 5083 Al alloy deformed at cryogenic temperature. Scr. Mater. 2004, 51, 355–359. [Google Scholar] [CrossRef]
- Verma, R.; Ghosh, A.K.; Kim, S.; Kim, C. Grain refinement and superplasticity in 5083 Al. Mater. Sci. Eng. A 1995, 191, 143–150. [Google Scholar] [CrossRef]
- Soer, W.A.; Chezan, A.R.; Hosson, J.T.M.D. Deformation and reconstruction mechanisms in coarse-grained superplastic Al–Mg alloys. Acta Mater. 2006, 54, 3827–3833. [Google Scholar] [CrossRef] [Green Version]
- Hasegawa, T.; Yasuno, T.; Nagai, T.; Takahashi, T. Origin of superplastic elongation in aluminum alloys produced by mechanical milling. Acta Mater. 1986, 46, 6001–6007. [Google Scholar] [CrossRef]
- Orozco-Caballero, A.; Ruano, O.A.; Rauch, E.F.; Carreño, F. Severe friction stir processing of an Al-Zn-Mg-Cu alloy: Misorientation and its influence on superplasticity. Mater. Des. 2018, 137, 128–139. [Google Scholar] [CrossRef]
- Patel, V.V.; Badheka, V.; Kumar, A. Effect of polygonal pin profiles on friction stir processed superplasticity of AA7075 alloy. J. Mater. Process. Technol. 2017, 240, 68–76. [Google Scholar] [CrossRef]
- Wang, Y.; Mishra, R.S. Finite element simulation of selective superplastic forming of friction stir processed 7075 Al alloy. Mater. Sci. Eng. A 2007, 463, 245–248. [Google Scholar] [CrossRef]
- Garcia-Infanta, J.M.; Zhilyaev, A.P.; Sharafutdinov, A.; Ruano, O.A.; Carreño, F. An evidence of high strain rate superplasticity at intermediate homologous temperatures in an Al–Zn–Mg–Cu alloy processed by high-pressure torsion. J. Alloy. Compd. 2009, 473, 163–166. [Google Scholar] [CrossRef]
- Li, S.; Huang, Z.G.; Jin, S.Y.; Wang, B.Y.; Liu, X.Q.; Lei, K. Heat Treatment Process of Cold Rolled 5A70 Aluminum alloy Superplastic Sheet. Chin. J. Rare. Met. 2018, 42, 283–288. [Google Scholar] [CrossRef]
- Chokshi, A.H.; Langdon, T.G. The influence of rolling direction on the mechanical behavior and formation of cavity stringers in the superplastic Zn-22% Al alloy. Acta Metall. 1989, 37, 715–723. [Google Scholar] [CrossRef]
- Nieh, T.G.; Hsiung, L.M.; Wadsworth, J.; Kaibyshev, R. High strain rate superplasticity in a continuously recrystallized Al–6%Mg–0.3%Sc alloy. Acta Mater. 1998, 46, 2789–2800. [Google Scholar] [CrossRef]
- Pilling, J.; Ridley, N. Effect of hydrostatic pressure on cavitation in superplastic aluminium alloys. Acta Metall. 1986, 34, 669–679. [Google Scholar] [CrossRef]
- Chen, C.L.; Tan, M.J. Cavity growth and filament formation of superplastically deformed Al 7475 Alloy. Mater. Sci. Eng. A 2001, 298, 235–244. [Google Scholar] [CrossRef]
- Conrad, H.; Cao, W.D.; Lu, X.P.; Sprecher, A.F. Effect of electric field on cavitation in superplastic aluminum alloy 7475. Mater. Sci. Eng. A 2001, 138, 247–258. [Google Scholar] [CrossRef]
- Engler, O.; Kuhnke, K.; Hasenclever, J. Development of intermetallic particles during solidification and homogenization of two AA 5xxx series Al-Mg alloys with different Mg contents. J. Alloy. Compd. 2017, 728, 669–681. [Google Scholar] [CrossRef]
- Duan, Y.L.; Xu, G.F.; Xiao, D.; Zhou, L.Q.; Deng, Y.; Yin, Z.M. Excellent superplasticity and deformation mechanism of Al–Mg–Sc–Zr alloy processed via simple free forging. Mater. Sci. Eng. A 2015, 624, 124–131. [Google Scholar] [CrossRef]
- Lee, S.; Utsunomiya, A.; Akamatsu, H.; Neishi, K.; Furukawa, M.; Horita, Z.; Langdon, T.G. Influence of scandium and zirconium on grain stability and superplastic ductilities in ultrafine-grained Al–Mg alloys. Acta Mater. 2002, 50, 553–564. [Google Scholar] [CrossRef]
- Horita, Z.; Furukawa, M.; Nemoto, M.; Barnes, A.J.; Langdon, T.G. Superplastic forming at high strain rates after severe plastic deformation. Acta Mater. 2000, 48, 3633–3640. [Google Scholar] [CrossRef]
- Gashti, S.O.; Fattah-alhosseini, A.; Mazaheri, Y.; Keshavarz, M.K. Effects of grain size and dislocation density on strain hardening behavior of ultrafine grained AA1050 processed by accumulative roll bonding. J. Alloy. Compd. 2016, 685, 854–861. [Google Scholar] [CrossRef]
- Mikhaylovskaya, A.V.; Yakovtseva, O.A.; Golovin, I.S.; Pozdniakov, A.V.; Portnoy, V.K. Superplastic deformation mechanisms in fine-grained Al–Mg based alloys. Mater. Sci. Eng. A 2015, 627, 31–41. [Google Scholar] [CrossRef]
- Cao, F.R.; Li, Z.L.; Zhang, N.X.; Ding, H.; Yu, F.X.; Zuo, L. Superplasticity, flow and fracture mechanism in an Al–12.7Si–0.7Mg alloy. Mater. Sci. Eng. A 2013, 571, 167–183. [Google Scholar] [CrossRef]
- Chokshi, A.H. Cavity nucleation and growth in superplasticity. Mater. Sci. Eng. A 2005, 410–411, 95–99. [Google Scholar] [CrossRef]
- Cao, F.R. Metal Superplasticity; Metallurgical Industry Press: Beijing, China, 2014; pp. 211–212. ISBN 978-7-5024-6690-9. [Google Scholar]
- Langdon, T.G. A unified approach to grain boundary sliding in creep and superplasticity. Acta Mater. 1994, 42, 2437–2443. [Google Scholar] [CrossRef]
- Mohamed, F.A. Interpretation of superplastic flow in terms of a threshold stress. J. Mater. Sci. 1983, 18, 582–592. [Google Scholar] [CrossRef]
- Raj, R.; Ashby, M.F. Intergranular fracture at elevated temperature Rupture intergranulaire aux temperatures elevees Intergranularer Bruch bei Höheren Temperaturen. Acta Metall. 1975, 23, 653–666. [Google Scholar] [CrossRef]
- Bae, D.H.; Ghosh, A.K. Cavity formation and early growth in a superplastic Al–Mg alloy. Acta Mater. 2002, 50, 511–523. [Google Scholar] [CrossRef]
- Kawasaki, M.; Xu, C.; Langdon, T.G. An investigation of cavity growth in a superplastic aluminum alloy processed by ECAP. Acta Mater. 2005, 53, 5353–5364. [Google Scholar] [CrossRef]
- Chokshi, A.H.; Langdon, T.G. A model for diffusional cavity growth in superplasticity. Acta Metall. 1987, 35, 1089–1101. [Google Scholar] [CrossRef]
- Liu, Z.Y. A new model for cavity nucleation. Trans. Nonferrous Met. Soc. China. 1997, 7, 145–148. [Google Scholar]
- Ghosh, A.K.; Bae, D.H.; Materials, S.L. Initiation and Early Stages of Cavity Growth during Superplastic and Hot Deformation. Mater. Science Forum. 1999, 304–306, 609–616. [Google Scholar] [CrossRef]
- Lee, C.J.; Huang, J.C. Cavitation characteristics in AZ31 Mg alloys during LTSP or HSRSP. Acta Mater. 2004, 52, 3111–3122. [Google Scholar] [CrossRef]
- Takigawa, Y.; Aguirre, J.V.; Taleff, E.M.; Higashi, K. Cavitation during grain-boundary-sliding deformation in an AZ61 magnesium alloy. Mater. Sci. Eng. A 2008, 497, 139–146. [Google Scholar] [CrossRef]
- Mao, W.; Yu, Y. Effect of elastic reaction stress on plastic behaviors of grains in polycrystalline aggregate during tensile deformation. Mater. Sci. Eng. A 2004, 367, 277–281. [Google Scholar] [CrossRef]
- Masuda, H.; Kanazawa, T.; Tobe, H.; Sato, E. Dynamic anisotropic grain growth during superplasticity in Al–Mg–Mn alloy. Scr. Mater. 2018, 149, 84–87. [Google Scholar] [CrossRef]









| Mg | Fe | Cu | Si | Zn | Ti | Mn | Al |
|---|---|---|---|---|---|---|---|
| 5.72 | 0.20 | 0.058 | 0.080 | 0.020 | 0.043 | 0.60 | Bal. |
| Temperature T/°C | Applied Stress σ/MPa | Strain-Rate Sensitivity m | Atomic Volume V/m3 | Strain Rate ε/s−1 |
|---|---|---|---|---|
| 500 | 3.70 | 0.47 | 2.34 × 10−2 | 1 × 10−3 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, S.; Jin, S.; Huang, Z. Cavity Behavior of Fine-Grained 5A70 Aluminum Alloy during Superplastic Formation. Metals 2018, 8, 1065. https://doi.org/10.3390/met8121065
Li S, Jin S, Huang Z. Cavity Behavior of Fine-Grained 5A70 Aluminum Alloy during Superplastic Formation. Metals. 2018; 8(12):1065. https://doi.org/10.3390/met8121065
Chicago/Turabian StyleLi, Sheng, Shunyao Jin, and Zhongguo Huang. 2018. "Cavity Behavior of Fine-Grained 5A70 Aluminum Alloy during Superplastic Formation" Metals 8, no. 12: 1065. https://doi.org/10.3390/met8121065
APA StyleLi, S., Jin, S., & Huang, Z. (2018). Cavity Behavior of Fine-Grained 5A70 Aluminum Alloy during Superplastic Formation. Metals, 8(12), 1065. https://doi.org/10.3390/met8121065