Mechanical and Thermal Stress Behavior of a Conservative Proposed Veneer Preparation Design for Restoring Misaligned Anterior Teeth: A 3D Finite Element Analysis
Abstract
:1. Introduction
2. Materials and Methods
2.1. 3D-FEA Design of Model Geometry
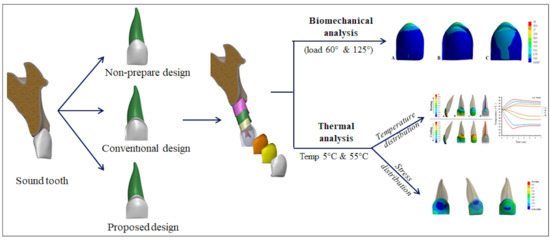
- Model A: Non-preparation design
- Model B: Conventional preparation design
- Model C: Proposed preparation design
2.2. Biomechanical Stress Analysis
2.3. Transient Thermal Finite Element Analysis
3. Results
3.1. Mechanical Loads
3.2. Transient Thermal Loads
3.2.1. Temperature Distribution
3.2.2. Stress Distribution
4. Discussion
5. Conclusions
- The preparation design has a significant effect of the mechanical behavior of laminate veneer restorations, which influences the integrity and survival rate of teeth restored with laminate veneer restorations, and the most effective preparation design recommended in this study for ceramic veneers is the proposed design.
- Different angulations induce different patterns of stress concentration. The fracture potential of veneer restorations was higher and more non-uniform at an angle of 125° than at 60°.
- In all types of preparation designs, the temperature changes were within the physiologic limits without enhancing any risk to the supporting hard tissues and dental pulp.
- Cold thermal loading was much effective than hot thermal loading. Thus, cold simulations yield thermal stresses of higher magnitude, which increases the risk of failure and short-term success of laminate veneers.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Ethical Approval
Data Availability
References
- Shetty, A.; Kaiwar, A.; Shubhashini, N.; Ashwini, P.; Naveen, D.; Adarsha, M.; Shetty, M.; Meena, N. Survival rates of porcelain laminate restoration based on different incisal preparation designs: An analysis. J. Conserv. Dent. 2011, 14, 10–15. [Google Scholar] [CrossRef] [Green Version]
- Beier, U.S.; Kapferer, I.; Burtscher, D.; Dumfahrt, H. Clinical performance of porcelain laminate veneers for up to 20 years. Int. J. Prosthodont. 2012, 25, 79–85. [Google Scholar]
- Usumez, A.; Aykent, F. Bond strengths of porcelain laminate veneers to tooth surfaces prepared with acid and Er, Cr:YSGG laser etching. J. Prosthet. Dent. 2003, 90, 24–30. [Google Scholar] [CrossRef]
- Celik Koycu, B.; Imirzalioglu, P.; Ozden, U.A. Three-dimensional finite element analysis of stress distribution in inlay-restored mandibular first molar under simultaneous thermomechanical loads. Dent. Mat. J. 2016, 35, 180–186. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Farias-Neto, A.; Gomes, E.M.; Sanchez-Ayala, A.; Sanchez-Ayala, A.; Vilanova, L.S. Esthetic Rehabilitation of the Smile with No-Prep Porcelain Laminates and Partial Veneers. Case Rep. Dent. 2015, 2015, 452765. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lin, T.M.; Liu, P.R.; Ramp, L.C.; Essig, M.E.; Givan, D.A.; Pan, Y.H. Fracture resistance and marginal discrepancy of porcelain laminate veneers influenced by preparation design and restorative material in vitro. J. Dent. 2012, 40, 202–209. [Google Scholar] [CrossRef]
- Ustun, O.; Ozturk, A.N. The evaluation of stress patterns in porcelain laminate veneers with different restoration designs and loading angles induced by functional loads: A three-dimensional finite element analysis study. Niger. J. Clin. Pract. 2018, 21, 337–342. [Google Scholar]
- Morita, R.K.; Hayashida, M.F.; Pupo, Y.M. Minimally Invasive Laminate Veneers: Clinical Aspects in Treatment Planning and Cementation Procedures. Case Rep. Dent. 2016, 2016, 1839793. [Google Scholar] [CrossRef]
- Zarone, F.; Epifania, E.; Leone, G.; Sorrentino, R.; Ferrari, M. Dynamometric assessment of the mechanical resistance of porcelain veneers related to tooth preparation: A comparison between two techniques. J. Prosthet. Dent. 2006, 95, 354–363. [Google Scholar] [CrossRef]
- Zarone, F.; Apicella, D.; Sorrentino, R.; Ferro, V.; Aversa, R.; Apicella, A. Influence of tooth preparation design on the stress distribution in maxillary central incisors restored by means of alumina porcelain veneers: A 3D-finite element analysis. Dent. Mat. Off. Publ. Acad. Dent. Mat. 2006, 21, 1178–1188. [Google Scholar] [CrossRef]
- Ge, C.; Green, C.C.; Sederstrom, D.; McLaren, E.A.; White, S.N. Effect of porcelain and enamel thickness on porcelain veneer failure loads in vitro. J. Prosthet. Dent. 2014, 111, 380–387. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, K.K.; Chiayabutr, Y.; Phillips, K.M.; Kois, J.C. Influence of preparation design and existing condition of tooth structure on load to failure of ceramic laminate veneers. J. Prosthet. Dent. 2011, 105, 374–382. [Google Scholar] [CrossRef]
- Li, Z.; Yang, Z.; Zuo, L.; Meng, Y. A three-dimensional finite element study on anterior laminate veneers with different incisal preparations. J. Prosthet. Dent. 2014, 112, 325–333. [Google Scholar] [CrossRef] [PubMed]
- Castelnuovo, J.; Tjan, A.H.; Phillips, K.; Nicholls, J.I.; Kois, J.C. Fracture load and mode of failure of ceramic veneers with different preparations. J. Prosthet. Dent. 2000, 83, 171–180. [Google Scholar] [CrossRef]
- Jankar, A.S.; Kale, Y.; Kangane, S.; Ambekar, A.; Sinha, M.; Chaware, S. Comparative evaluation of fracture resistance of Ceramic Veneer with three different incisal design preparations—An In-vitro Study. J. Int. Oral Health 2014, 6, 48–54. [Google Scholar]
- Chander, G.; Padmanabhan, T. Finite Element Stress Analysis of Diastema Closure with Ceramic Laminate Veneers. J. Prosthodont. Off. J. Am. Coll. Prosthodont. 2009, 18, 577–581. [Google Scholar] [CrossRef]
- Guler, M.S.; Guler, C.; Cakici, F.; Cakici, E.B.; Sen, S. Finite element analysis of thermal stress distribution in different restorative materials used in class V cavities. Niger. J. Clin. Pract. 2016, 19, 30–34. [Google Scholar]
- Cotert, H.; Dundar, M.; Oztürk, B. The Effect of Various Preparation Designs on the Survival of Porcelain Laminate Veneers. J. Adhes. Dent. 2009, 11, 405–411. [Google Scholar]
- Cornacchia, T.; Las Casas, E.; Cimini, C.; Peixoto, R. 3D finite element analysis on esthetic indirect dental restorations under thermal and mechanical loading. Med. Biol. Eng. Comp. 2010, 48, 1107–1113. [Google Scholar] [CrossRef]
- Hussein, F.; Salloomi, K.; Abdulrahman, B.; Al-Zahawi, A.; Sabri, L. Effect of thread depth and implant shape on stress distribution in anterior and posterior regions of mandible bone: A finite element analysis. Dent. Res. J. 2019, 16, 200–207. [Google Scholar]
- Gonçalves, W.; Adelino, V.; Barão, R.; Gomes, É.; Delben, J.; Ribeiro, R. 3 FEA in Dentistry: A Useful Tool to Investigate the Biomechanical Behavior of Implant Supported Prosthesis; Intech Open: London, UK, 2013. [Google Scholar]
- Sang, Y.H.; Hu, H.C.; Lu, S.H.; Wu, Y.W.; Li, W.R.; Tang, Z.H. Accuracy Assessment of Three-dimensional Surface Reconstructions of In vivo Teeth from Cone-beam Computed Tomography. Chin. Med. J. 2016, 129, 1464–1470. [Google Scholar] [CrossRef] [PubMed]
- Poiate, I.A.; de Vasconcellos, A.B.; de Santana, R.B.; Poiate, E. Three-dimensional stress distribution in the human periodontal ligament in masticatory, parafunctional, and trauma loads: Finite element analysis. J. Periodontol. 2009, 80, 1859–1867. [Google Scholar] [CrossRef] [PubMed]
- Geng, J.P.; Tan, K.B.; Liu, G.R. Application of finite element analysis in implant dentistry: A review of the literature. J. Prosthet. Dent. 2001, 85, 585–598. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nelson, S.J. Wheeler’s Dental Anatomy, Physiology, and Occlusion; Elsevier: Amsterdam, The Netherlands; Saunders: St. Louis, MO, USA, 2014; p. 105. [Google Scholar]
- Tuna, M.; Sunbuloglu, E.; Bozdag, E. Finite element simulation of the behavior of the periodontal ligament: A validated nonlinear contact model. J. Biomech. 2014, 47, 2883–2890. [Google Scholar] [CrossRef]
- Yamamoto, T.; Hasegawa, T.; Yamamoto, T.; Hongo, H.; Amizuka, N. Histology of human cementum: Its structure, function, and development. Jpn. Dent. Sci. Rev. 2016, 52, 63–74. [Google Scholar] [CrossRef] [Green Version]
- Kim, H.J.; Yu, S.K.; Lee, M.H.; Lee, H.J.; Kim, H.J.; Chung, C.H. Cortical and cancellous bone thickness on the anterior region of alveolar bone in Korean: A study of dentate human cadavers. J. Adv. Prosthodont. 2012, 4, 146–152. [Google Scholar] [CrossRef] [Green Version]
- Chai, S.Y.; Bennani, V.; Aarts, J.M.; Lyons, K. Incisal preparation design for ceramic veneers: A critical review. J. Am. Dent. Assoc. 2018, 149, 25–37. [Google Scholar] [CrossRef]
- Celebi, A.T.; Icer, E.; Eren, M.M.; Baykasoglu, C.; Mugan, A.; Yildiz, E. Thermal-stress analysis of ceramic laminate veneer restorations with different incisal preparations using micro-computed tomography-based 3D finite element models. J. Mech. Behav. Biomed. Mat. 2017, 75, 302–313. [Google Scholar] [CrossRef]
- Arat Bilhan, S.; Baykasoglu, C.; Bilhan, H.; Kutay, O.; Mugan, A. Effect of attachment types and number of implants supporting mandibular overdentures on stress distribution: A computed tomography-based 3D finite element analysis. J. Biomech. 2015, 48, 130–137. [Google Scholar] [CrossRef]
- Babayi, M.; Ashtiani, M.N. Effects of Cyclic Thermal Loads on Bone-Implant Interface in Dental Prostheses. Zahedan J. Res. Med. Sci. 2017, 19, e12081. [Google Scholar] [CrossRef] [Green Version]
- Pałka, K.; Bieniaś, J.; Dębski, H.; Niewczas, A. Finite element analysis of thermo-mechanical loaded teeth. Comput. Mat. Sci. 2012, 64, 289–294. [Google Scholar] [CrossRef]
- Dejak, B.; Mlotkowski, A.; Langot, C. Three-dimensional finite element analysis of molars with thin-walled prosthetic crowns made of various materials. Dent. Mat: Off. Publ. Acad. Dent. Mat. 2012, 28, 433–441. [Google Scholar] [CrossRef] [PubMed]
- Benazzi, S.; Nguyen, H.N.; Kullmer, O.; Kupczik, K. Dynamic Modelling of Tooth Deformation Using Occlusal Kinematics and Finite Element Analysis. PLoS ONE 2016, 11, e0152663. [Google Scholar] [CrossRef] [PubMed]
- Arora, A.; Upadhyaya, V.; Arora, S.J.; Jain, P.; Yadav, A. Evaluation of fracture resistance of ceramic veneers with different preparation designs and loading conditions: An in vitro study. J. Ind. Prosthodont. Soc. 2017, 17, 325–331. [Google Scholar]
- Anusavice, K. Phillips’ Science of Dental Materials; Saunders: Philadelphia, PA, USA, 2012; p. 272. [Google Scholar]
- Zach, L.; Cohen, G. Thermogenesis in operative techniques: Comparison of four methods. J. Prosthet. Dent. 1962, 12, 977–984. [Google Scholar] [CrossRef]
- Gungor, M.A.; Kucuk, M.; Dundar, M.; Karaoglu, C.; Artunc, C. Effect of temperature and stress distribution on all-ceramic restorations by using a three-dimensional finite element analysis. J. Oral Rehabil. 2004, 31, 172–178. [Google Scholar] [CrossRef]
- Lin, M.; Xu, F.; Lu, T.J.; Bai, B.F. A review of heat transfer in human tooth—Experimental characterization and mathematical modeling. Dent. Mat: Off. Publ. Acad. Dent. Mat. 2010, 26, 501–513. [Google Scholar] [CrossRef]
- Farah, R.F.I. Effect of cooling water temperature on the temperature changes in pulp chamber and at handpiece head during high-speed tooth preparation. Restor. Dent. Endod. 2018, 44, 1116523. [Google Scholar] [CrossRef]













| Material | Young’s Modulus (GPa) | Poisson’s Ratio | Density (g/cm3) | Specific Heat (J/(g °C)) | Thermal Expansion (1/ °C) | Thermal Conductivity (J/(s mm °C)) | References |
|---|---|---|---|---|---|---|---|
| Enamel | 84.1 | 0.33 | 3 | 0.754 | 1.70 × 10−5 | 0.92 × 10−3 | [33,34] |
| Dentin | 18.6 | 0.31 | 2.20 | 1.172 | 1.06 × 10−5 | 0.63 × 10−3 | [33,34] |
| Cementum | 15.5 | 0.31 | 2.06 | 0.824 | 1.1 × 10−5 | 0.62 × 10−3 | [35] |
| Periodontal Ligament | 0.069 | 0.45 | 1.1 | 2.290 | 1.06 × 10−5 | 0.59 × 10−3 | [23,30] |
| Cancellous Bone | 1.37 | 0.30 | 0.62 | 1.16 | 1.0 × 10−5 | 0.39 × 10−3 | [4] |
| Cortical Bone | 13.7 | 0.30 | 2.06 | 1.26 | 1.0 × 10−5 | 0.38 × 10−3 | [4] |
| Pulp | 0.02 | 0.45 | 1 | 4.2 | 1.81 × 10−5 | 0.63 × 10−3 | [17,23] |
| Adhesive Layer | 4.5 | 0.3 | 2.02 | 0.824 | 1.06 × 10−5 | 0.4 × 10−3 | [33] |
| Luting cement | 8.3 | 0.24 | 1.1 | 0.824 | 3.0 × 10−5 | 1.091 × 10−3 | [7,30] |
| Celtra Duo (Dentsply) | 70 | 0.22 | 2.6 | 0.973 | 1.18 × 10−5 | 1.463 × 10−3 | Dentsply, manufacturer |
| Preparation Designs | Load Angle | Ceramic Veneer | Cement Layer | Tooth Structures | ||||
|---|---|---|---|---|---|---|---|---|
| Enamel | Dentin | PDL | Cementum | Bone | ||||
| Non- preparation (Mod A) | 60° | 75.96 | 42.73 | 26.87 | 20.87 | 15.34 | 12.31 | 54.40 |
| 125° | 107.88 | 51.34 | 43.62 | 29.27 | 17.22 | 15.41 | 62.28 | |
| Conventional | 60° | 122.99 | 31.99 | 33.16 | 21.90 | 17.29 | 13.15 | 55.99 |
| (Mod B) | 125° | 149.31 | 44.03 | 40.69 | 30.61 | 17.39 | 16.47 | 62.09 |
| Proposed(Mod C) | 60° | 64.87 | 32.01 | 23.45 | 19.54 | 15.10 | 12.359 | 55.57 |
| 125° | 81.12 | 44.98 | 35.07 | 29.65 | 18.15 | 16.53 | 61.73 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dawood, S.N.; Al-Zahawi, A.R.; Sabri, L.A. Mechanical and Thermal Stress Behavior of a Conservative Proposed Veneer Preparation Design for Restoring Misaligned Anterior Teeth: A 3D Finite Element Analysis. Appl. Sci. 2020, 10, 5814. https://doi.org/10.3390/app10175814
Dawood SN, Al-Zahawi AR, Sabri LA. Mechanical and Thermal Stress Behavior of a Conservative Proposed Veneer Preparation Design for Restoring Misaligned Anterior Teeth: A 3D Finite Element Analysis. Applied Sciences. 2020; 10(17):5814. https://doi.org/10.3390/app10175814
Chicago/Turabian StyleDawood, Shilan Nawzad, Abdulsalam Rasheed Al-Zahawi, and Laith Abed Sabri. 2020. "Mechanical and Thermal Stress Behavior of a Conservative Proposed Veneer Preparation Design for Restoring Misaligned Anterior Teeth: A 3D Finite Element Analysis" Applied Sciences 10, no. 17: 5814. https://doi.org/10.3390/app10175814
APA StyleDawood, S. N., Al-Zahawi, A. R., & Sabri, L. A. (2020). Mechanical and Thermal Stress Behavior of a Conservative Proposed Veneer Preparation Design for Restoring Misaligned Anterior Teeth: A 3D Finite Element Analysis. Applied Sciences, 10(17), 5814. https://doi.org/10.3390/app10175814