Emission Modes in Electrospray Thrusters Operating with High Conductivity Ionic Liquids
Abstract
:1. Introduction and Background
1.1. Colloid Electrospray Thruster Lifetime
1.2. Electrospray Emission Modes
2. EMI-Im Electrospray Emission Mode Classification Experiments
2.1. Experiment Setup
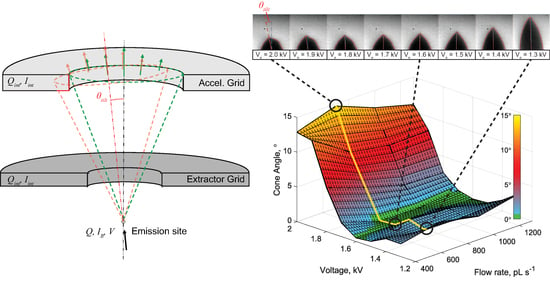
2.2. Steady-State Emission: Results
2.3. Steady-State Emission: Discussion
2.4. Transient Emission: Results
2.5. Transient Emission: Discussion
3. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| EHD | Electrohydrodynamic |
| CMTS | Colloid Microthruster System |
| UCLA | University of California, Los Angeles |
| HOAGIE | Highly Optimizable Apparatus for Groundbreaking Investigations in Electrosprays |
| EMI-Im | 1-ethyl-3-methylimidazolium bis(triflouromethylsulfonyl)amide |
| DRS | Disturbance Reduction System |
| ST-7 | Space Technology 7 |
| LDM | Long-Distance Microscope |
| POF | Pressure-over-fluid |
| QCM | Quartz Crystal Microbalance |
References
- Legge, R.S.; Lozano, P.C. Electrospray Propulsion Based on Emitters Microfabricated in Porous Metals. J. Propuls. Power 2011, 27, 485–495. [Google Scholar] [CrossRef]
- Mennesson, B.; Kiessling, A.; Warfield, K. HabEx: Habitable Exoplanet Observatory Interim Report; Technical Report; Astronomy, Physics and Space Technology Directorate, Jet Propulsion Laboratory: Pasadena, CA, USA, 2018. [Google Scholar]
- Demmons, N.R.; Lamarre, N.; Ziemer, J.K.; Parker, M.; Spence, D. Electrospray Thruster Propellant Feedsystem for a Gravity Wave Observatory Mission; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2016. [Google Scholar] [CrossRef]
- Hruby, V.; Spence, D.; Demmons, N.; Roy, T.; Ehrbar, E.; Zwahlen, J.; Martin, R.; Ziemer, J.; Connolly, W.; Rhodes, S. ST7-DRS colloid thruster system development and performance summary. In Proceedings of the 44th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Hartford, CT, USA, 21–23 July 2008; pp. 1–32. [Google Scholar] [CrossRef]
- Ziemer, J. Performance of Electrospray Thrusters. In Proceedings of the International Electric Propulsion Conference, Ann Arbor, MI, USA, 20–24 September 2009. [Google Scholar]
- Ziemer, J.; Marrese-Reading, C.; Dunn, C.; Romero-Wolf, A.; Cutler, C.; Javidnia, S.; Li, T.; Li, I.; Franklin, G.; Barela, P.; et al. Colloid Microthruster Flight Performance Results from Space Technology 7 Disturbance Reduction System. In Proceedings of the International Electric Propulsion Conference, Atlanta, GA, USA, 8–12 October 2017. [Google Scholar]
- Amaro-Seoane, P.; Audley, H.; Babak, S.; Baker, J.; Barausse, E.; Bender, P.; Berti, E.; Binetruy, P.; Born, M.; Bortoluzzi, D. Laser interferometer space antenna. arXiv 2017, arXiv:1702.00786. [Google Scholar]
- Chiu, Y.H.; Dressler, R.A. Ionic Liquids for Space Propulsion; ACS Symposium Series; American Chemical Society: Washington, DC, USA, 2007; Volume 975, pp. 138–160. [Google Scholar] [CrossRef]
- Thuppul, A.; Wright, P.L.; Collins, A.L.; Ziemer, J.K.; Wirz, R.E. Lifetime Considerations for Electrospray Thrusters. Aerospace 2020, 7, 108. [Google Scholar] [CrossRef]
- Ziemer, J.K.; Randolph, T.M.; Gamero-Castañ, M.; Hruby, V.; Connolly, W.; Demmons, N.; Ehrbar, E.; Martin, R.; Roy, T.; Spence, D.; et al. Flight Hardware Development of Colloid Microthruster Technology for the Space Technology 7 and LISA Missions. In Proceedings of the 30th International Electric Propulsion Conference, 2007, IEPC-2007-288, Florence, Italy, 17–20 September 2007. [Google Scholar]
- Grifoll, J.; Rosell-Llompart, J. Continuous droplets’ charge method for the Lagrangian simulation of electrostatic sprays. J. Electrostat. 2014, 72, 357–364. [Google Scholar] [CrossRef] [Green Version]
- Davis, M.J.; Collins, A.L.; Wirz, R.E. Electrospray Plume Evolution Via Discrete Simulations. In Proceedings of the 36th International Electric Propulsion Conference, 2019, EPC-2019-590, Vienna, Austria, 15–20 September 2019. [Google Scholar]
- Verdoold, S.; Agostinho, L.L.F.; Yurteri, C.U.; Marijnissen, J.C.M. A generic electrospray classification. J. Aerosol. Sci. 2014, 67, 87–103. [Google Scholar] [CrossRef]
- Juraschek, R.; Rollgen, F. Pulsation phenomena during electrospray ionization. Int. J. Mass Spectrom. 1998, 177, 1–15. [Google Scholar] [CrossRef]
- Chen, C.H.; Saville, D.A.; Aksay, I.A. Scaling laws for pulsed electrohydrodynamic drop formation. Appl. Phys. Lett. 2006, 89, 124103. [Google Scholar] [CrossRef] [Green Version]
- Bober, D.B.; Chen, C.H. Pulsating electrohydrodynamic cone-jets: From choked jet to oscillating cone. J. Fluid Mech. 2011, 689, 552–563. [Google Scholar] [CrossRef] [Green Version]
- Cloupeau, M.; Prunet-Foch, B. Electrostatic spraying of liquids in cone-jet mode. J. Electrostat. 1989, 22, 135–159. [Google Scholar] [CrossRef]
- Hohman, M.M.; Shin, M.; Rutledge, G.; Brenner, M.P. Electrospinning and electrically forced jets. I. Stability theory. Phys. Fluids 2001, 13, 2201–2220. [Google Scholar] [CrossRef] [Green Version]
- Hohman, M.M.; Shin, M.; Rutledge, G.; Brenner, M.P. Electrospinning and electrically forced jets. II. Applications. Phys. Fluids 2001, 13, 2221–2236. [Google Scholar] [CrossRef] [Green Version]
- Marginean, I.; Nemes, P.; Parvin, L.; Vertes, A. How much charge is there on a pulsating Taylor cone? Appl. Phys. Lett. 2006, 89, 064104. [Google Scholar] [CrossRef] [Green Version]
- Jaworek, A.; Krupa, A. Jet and drops formation in electrohydrodynamic spraying of liquids. A systematic approach. Exp. Fluids 1999, 27, 43–52. [Google Scholar] [CrossRef]
- Zeleny, J. On the conditions of instability of electrified drops with applications to the electrical discharge from liquid points. Proc. Camb. Philo. Soc. 1915, 18, 71–83. [Google Scholar]
- Ziemer, J.K.; Randolph, T.; Hruby, V.; Spence, D.; Demmons, N.; Roy, T.; Connolly, W.; Ehrbar, E.; Zwahlen, J.; Martin, R. Colloid microthrust propulsion for the space technology 7 (ST7) and LISA missions. In Proceedings of the AIP Conference, Jammu, India, 17–18 April 2006; American Institute of Physics: College Park, MD, USA, 2006; Volume 873, pp. 548–555. [Google Scholar] [CrossRef]
- Krejci, D.; Mier-Hicks, F.; Thomas, R.; Haag, T.; Lozano, P. Emission characteristics of passively fed electrospray microthrusters with propellant reservoirs. J. Spacecr. Rocket 2017, 54, 447–458. [Google Scholar] [CrossRef]
- Courtney, D.G.; Dandavino, S.; Shea, H. Comparing direct and indirect thrust measurements from passively fed ionic electrospray thrusters. J. Propuls. Power 2016, 32, 392–407. [Google Scholar] [CrossRef] [Green Version]
- Taylor, G.I. Disintegration of water drops in an electric field. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1964, 280, 383–397. [Google Scholar] [CrossRef]
- Rayleigh, L. On the equilibrium of liquid conducting masses charged with electricity. Philos. Mag. 1882, 14, 184–186. [Google Scholar] [CrossRef] [Green Version]
- Demmons, N.R.; Courtney, D.; Alvarez, N.; Wood, Z. Component-Level Development and Testing of a Colloid Micro-Thruster (CMT) System for the LISA Mission. In Proceedings of the AIAA Propulsion and Energy Forum, Indianapolis, IN, USA, 19–22 August 2019. [Google Scholar] [CrossRef]
- Demmons, N.R.; Wood, Z.; Alvarez, N. Characterization of a High Thrust, Pressure-Fed Electrospray Thruster for Precision Attitude Control Applications. In Proceedings of the AIAA Propulsion and Energy Forum, Indianapolis, IN, USA, 19–22 August 2019. [Google Scholar] [CrossRef]
- Rosell-Llompart, J.; Grifoll, J.; Loscertales, I.G. Electrosprays in the cone-jet mode: From Taylor cone formation to spray development. J. Aerosol. Sci. 2018, 125, 2–31. [Google Scholar] [CrossRef]
- Gan, Y.; Luo, Z.; Cheng, Y.; Xu, J. The electro-spraying characteristics of ethanol for application in a small-scale combustor under combined electric field. Appl. Therm. Eng. 2015, 87, 595–604. [Google Scholar] [CrossRef]
- Demmons, N.; Hruby, V.; Spence, D.; Roy, T.; Ehrbar, E.; Zwahlen, J.; Martin, R.; Ziemer, J.; Randolph, T. ST7-DRS Mission Colloid Thruster Development. In Proceedings of the American Institute of Aeronautics and Astronautics, 2008, Joint Propulsion Conferences, Honolulu, HI, USA, 18–21 August 2008. [Google Scholar] [CrossRef]
- Wirz, R.E.; Collins, A.L.; Thuppul, A.L.; Wright, P.; Uchizono, N.M.; Huh, H.; Davis, M.J.; Ziemer, J.K.; Demmons, N.R. Electrospray Thruster Performance and Lifetime Investigation for the LISA Mission. In Proceedings of the AIAA Propulsion and Energy Forum, Indianapolis, IN, USA, 19–22 August 2019. [Google Scholar] [CrossRef]
- Wright, P.L.; Thuppul, A.; Wirz, R.E. Life-Limiting Emission Modes for Electrospray Thrusters. In Proceedings of the AIAA Propulsion and Energy Forum, Cincinnati, OH, USA, 9–11 July 2018. [Google Scholar] [CrossRef]
- Fröba, A.P.; Kremer, H.; Leipertz, A. Density, Refractive Index, Interfacial Tension, and Viscosity of Ionic Liquids [EMIM][EtSO4], [EMIM][NTf2], [EMIM][N(CN)2], and [OMA][NTf2] in Dependence on Temperature at Atmospheric Pressure. J. Phys. Chem. B 2008, 112, 12420–12430. [Google Scholar] [CrossRef] [PubMed]
- Schreiner, C.; Zugmann, S.; Hartl, R.; Gores, H.J. Fractional Walden Rule for Ionic Liquids: Examples from Recent Measurements and a Critique of the So-Called Ideal KCl Line for the Walden Plot. J. Chem. Eng. Data 2010, 55, 1784–1788. [Google Scholar] [CrossRef]
- Geppert-Rybczyńska, M.; Lehmann, J.K.; Heintz, A. Surface Tensions and the Gibbs Excess Surface Concentration of Binary Mixtures of the Ionic Liquid 1-Ethyl-3-methylimidazolium Bis[(trifluoromethyl)sulfonyl]imide with Tetrahydrofuran and Acetonitrile. J. Chem. Eng. Data 2011, 56, 1443–1448. [Google Scholar] [CrossRef]
- Daguenet, C.; Dyson, P.J.; Krossing, I.; Oleinikova, A.; Slattery, J.; Wakai, C.; Weingärtner, H. Dielectric Response of Imidazolium-Based Room-Temperature Ionic Liquids. J. Phys. Chem. B 2006, 110, 12682–12688. [Google Scholar] [CrossRef]
- Ziemer, J.; Marrese-Reading, C.; Cutler, C.; Dunn, C.; Romero-Wolf, A.; Javidnia, S.; Li, T.; Li, I.; Barela, P.; Demmons, N.; et al. In-Flight Verification and Validation of Colloid Microthruster Performance; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2018. [Google Scholar]
- Ziemer, J.K.; Marrese-Reading, C.; Arestie, S.; Demmons, N.R.; Wirz, R.E.; Collins, A.; Gamero, M. Progress on Developing LISA Microthruster Technology. In Proceedings of the AIAA Propulsion and Energy 2020 Forum, Virtual Event, 24–28 August 2020. [Google Scholar] [CrossRef]
- Carmain, A.; Dunn, C.; Folkner, W.; Hruby, V.; Spence, D.; Demmons, N.; Roy, T.; McCormick, R.; Gasdaska, C.; Young, J.; et al. Space Technology 7 disturbance reduction system—Precision control flight validation. In Proceedings of the 2006 IEEE Aerospace Conference, Big Sky, MT, USA, 4–11 March 2006; p. 7. [Google Scholar]
- Thuppul, A.; Wright, P.L.; Uchizono, N.M.; Collins, A.L.; Wirz, R.E. Spatially-Resolved Mass Flux and Current Measurements for Electrospray Plumes. In Proceedings of the 36th International Electric Propulsion Conference, 2019, IEPC-2019-571, Vienna, Austria, 15–20 September 2019. [Google Scholar]
- Uchizono, N.M.; Collins, A.L.; Thuppul, A.; Wright, P.L.; Eckhardt, D.Q.; Ziemer, J.Z.; Wirz, R.E. Electrospray Steady-State and Transient Emission Behavior. In Proceedings of the 36th International Electric Propulsion Conference, 2019, IEPC-2019-368, Vienna, Austria, 15–20 September 2019. [Google Scholar]
- Hayati, A.; Bailey, A.I.; Tadros, T.F. Mechanism of stable jet formation in electrohydrodynamic atomization. Nature 1986, 319, 41–43. [Google Scholar] [CrossRef]
- Shtern, V.; Barrero, A. Striking features of fluid flows in Taylor cones related to electrosprays. J. Aerosol. Sci. 1994, 25, 1049–1063. [Google Scholar] [CrossRef]
- Shtern, V.; Barrero, A. Instability nature of the swirl appearance in liquid cones. Phys. Rev. E 1995, 52, 627–635. [Google Scholar] [CrossRef]
- Shtern, V.; Barrero, A. Bifurcation of swirl in liquid cones. J. Fluid Mech. 1995, 300, 169–205. [Google Scholar] [CrossRef]
- Barrero, A.; Gañán-Calvo, A.M.; Davila, J.; Palacios, A.; Gomez-Gonzales, E. The Role of the Electrical Conductivity and Viscosity on the Motions inside Taylor Cone. J. Electrostat. 1999, 47, 13–26. [Google Scholar] [CrossRef]
- de la Mora, J.F.; Loscertales, I.G. The current emitted by highly conducting Taylor cones. J. Fluid Mech. 1994, 260, 155–184. [Google Scholar] [CrossRef]
- Chen, D.R.; Pui, D.Y.H. Experimental Investigation of Scaling Laws for Electrospraying: Dielectric Constant Effect. Aerosol. Sci. Technol. 1997, 27, 367–380. [Google Scholar] [CrossRef]
- Gamero-Castaño, M.; de la Mora, J.F. Direct measurement of ion evaporation kinetics from electrified liquid surfaces. J. Chem. Phys. 2000, 113, 815–832. [Google Scholar] [CrossRef] [Green Version]
- Nayak, R.; Padhye, R.; Kyratzis, I.L.; Truong, Y.B.; Arnold, L. Effect of viscosity and electrical conductivity on the morphology and fiber diameter in melt electrospinning of polypropylene. Text. Res. J. 2013, 83, 606–617. [Google Scholar] [CrossRef]
- de la Mora, J. On the Outcome of the Coulombic Fission of a Charged Isolated Drop. J. Colloid Interface Sci. 1996, 178, 209–218. [Google Scholar] [CrossRef]
- Deng, W.; Gomez, A. Full transient response of Taylor cones to a step change in electric field. Microfluid. Nanofluid. 2012, 12, 383–393. [Google Scholar] [CrossRef]











| Property | Symbol | Value at 298 K | Reference |
|---|---|---|---|
| Density | 1518.48 kg m−3 | [35] | |
| Viscosity | 0.03246 Pa s | [36] | |
| Surface Tension | 0.0359 N m−1 | [37] | |
| Electrical Conductivity | 0.921 S m−1 | [36] | |
| Relative Permittivity | 12.25 | [38] |
| Extraction Voltage | Cone Tilt Angle | Reconstructed Angle | ||
|---|---|---|---|---|
| Image | Plume Profile | Polar Angle | Azimuthal Angle | |
| 1.8 kV | 10° | 2° | 10° | 11° |
| 1.9 kV | 13° | 8° | 15° | 31° |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Uchizono, N.M.; Collins, A.L.; Thuppul, A.; Wright, P.L.; Eckhardt, D.Q.; Ziemer, J.; Wirz, R.E. Emission Modes in Electrospray Thrusters Operating with High Conductivity Ionic Liquids. Aerospace 2020, 7, 141. https://doi.org/10.3390/aerospace7100141
Uchizono NM, Collins AL, Thuppul A, Wright PL, Eckhardt DQ, Ziemer J, Wirz RE. Emission Modes in Electrospray Thrusters Operating with High Conductivity Ionic Liquids. Aerospace. 2020; 7(10):141. https://doi.org/10.3390/aerospace7100141
Chicago/Turabian StyleUchizono, Nolan M., Adam L. Collins, Anirudh Thuppul, Peter L. Wright, Daniel Q. Eckhardt, John Ziemer, and Richard E. Wirz. 2020. "Emission Modes in Electrospray Thrusters Operating with High Conductivity Ionic Liquids" Aerospace 7, no. 10: 141. https://doi.org/10.3390/aerospace7100141
APA StyleUchizono, N. M., Collins, A. L., Thuppul, A., Wright, P. L., Eckhardt, D. Q., Ziemer, J., & Wirz, R. E. (2020). Emission Modes in Electrospray Thrusters Operating with High Conductivity Ionic Liquids. Aerospace, 7(10), 141. https://doi.org/10.3390/aerospace7100141