Quantum PBR Theorem as a Monty Hall Game
Abstract
:1. Introduction
2. PBR Theorem
3. Classic Monty Hall
4. Ignorant Monty Hall
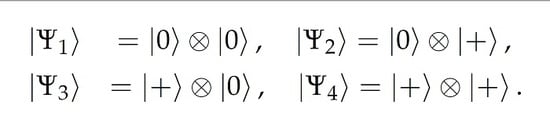
5. ψ-Ontic Monty Hall Game
6. ψ-Epistemic Monty Hall Game
7. Quantum Teleportation
7.1. Monty Hall Teleportation
7.2. Unreliable Teleportation
8. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bell, J.S. On the Einstein–Podolsky–Rosen paradox. Phys. Phys. Fiz. 1964, 1, 195–200. [Google Scholar] [CrossRef] [Green Version]
- Brunner, N.; Cavalcanti, D.; Pironio, S.; Scarani, V.; Wehner, S. Bell nonlocality. Rev. Mod. Phys. 2014, 86, 419. [Google Scholar] [CrossRef] [Green Version]
- Brunner, N.; Linden, N. Connection between Bell nonlocality and Bayesian game theory. Nat. Commun. 2013, 4, 2057. [Google Scholar] [CrossRef]
- Pappa, A.; Kumar, N.; Lawson, T.; Santha, M.; Zhang, S.; Diamanti, E.; Kerenidis, I. Nonlocality and conflicting interest games. Phys. Rev. Lett. 2015, 114, 020401. [Google Scholar] [CrossRef] [Green Version]
- Almeida, M.L.; Bancal, J.; Brunner, N.; Acín, A.; Gisin, N.; Pironio, S. Guess your neighbor’s input: A multipartite nonlocal game with no quantum advantage. Phys. Rev. Lett. 2010, 104, 230404. [Google Scholar] [CrossRef] [Green Version]
- Coles, P.J.; Berta, M.; Tomamichel, M.; Wehner, S. Entropic uncertainty relations and their applications. Rev. Mod. Phys. 2017, 89, 015002. [Google Scholar] [CrossRef] [Green Version]
- Coles, P.J.; Katariya, V.; Lloyd, S.; Marvian, I.; Wilde, M.M. Entropic energy-time uncertainty relation. Phys. Rev. Lett. 2019, 122, 100401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Eisert, J.; Wilkens, M.; Lewenstein, M. Quantum games and quantum strategies. Phys. Rev. Lett. 1999, 83, 3077. [Google Scholar] [CrossRef] [Green Version]
- Benjamin, S.C.; Simon, C.; Hayden, P.M. Multiplayer quantum games. Phys. Rev. A 2001, 64, 030301. [Google Scholar] [CrossRef] [Green Version]
- Khan, F.S.; Solmeyer, N.; Balu, R.; Humble, T.S. Quantum games: A review of the history, current state, and interpretation. Quantum Inf. Process. 2018, 17, 309. [Google Scholar] [CrossRef] [Green Version]
- Rodriguez, A.; Mendes, B. Probability, Decisions and Games: A Gentle Introduction Using R; John Wiley & Sons: Hoboken, NJ, USA, 2018. [Google Scholar]
- Rosenthal, J.S. Monty Hall, Monty fall, Monty crawl. Math. Horiz. 2008, 16, 5–7. [Google Scholar] [CrossRef]
- Gill, R. Monty Hall problem. Int. Encycl. Stat. Sci. 2010, 858–863. Available online: https://www.math.leidenuniv.nl/~gill/mhp-statprob.pdf (accessed on 31 December 2019).
- Lucas, S.; Rosenhouse, J.; Schepler, A. The Monty Hall problem, reconsidered. Math. Mag. 2009, 82, 332–342. [Google Scholar] [CrossRef]
- Li, C.; Zhang, Y.; Huang, Y.; Guo, G. Quantum strategies of quantum measurements. Phys. Lett. A 2001, 280, 257–260. [Google Scholar] [CrossRef] [Green Version]
- Flitney, A.P.; Abbott, D. Quantum version of the Monty Hall problem. Phys. Rev. A 2002, 65, 062318. [Google Scholar] [CrossRef] [Green Version]
- D’Ariano, G.M.; Gill, R.D.; Keyl, M.; Kümmerer, B.; Maassen, H.; Werner, R.F. The quantum Monty Hall problem. Quant. Inf. Comput. 2002, 2, 355–466. [Google Scholar]
- Khan, S.; Ramzan, M.; Khan, M.K. Quantum Monty Hall problem under decoherence. Commun. Theor. Phys. 2010, 54, 47. [Google Scholar] [CrossRef] [Green Version]
- Kurzyk, D.; Glos, A. Quantum inferring acausal structures and the Monty Hall problem. Quantum Inf. Process. 2016, 15, 4927–4937. [Google Scholar] [CrossRef] [Green Version]
- Zander, C.; Casas, M.; Plastino, A.; Plastino, A.R. Positive operator valued measures and the quantum Monty Hall problem. An. Acad. Bras. Ciênc. 2006, 78, 417–422. [Google Scholar] [CrossRef] [Green Version]
- Paul, S.; Behera, B.K.; Panigrahi, P.K. Playing quantum Monty Hall game in a quantum computer. arXiv 2019, arXiv:1901.01136. [Google Scholar]
- Pusey, M.F.; Barrett, J.; Rudolph, T. On the reality of the quantum state. Nat. Phys. 2012, 8, 475–478. [Google Scholar] [CrossRef] [Green Version]
- Harrigan, N.; Spekkens, R.W. Einstein, incompleteness, and the epistemic view of quantum states. Found. Phys. 2010, 40, 125–157. [Google Scholar] [CrossRef] [Green Version]
- Lewis, P.G.; Jennings, D.; Barrett, J.; Rudolph, T. Distinct quantum states can be compatible with a single state of reality. Phys. Rev. Lett. 2012, 109, 150404. [Google Scholar] [CrossRef] [Green Version]
- Schlosshauer, M.; Fine, A. Implications of the Pusey–Barrett–Rudolph quantum no-go theorem. Phys. Rev. Lett. 2012, 108, 260404. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Aaronson, S.; Bouland, A.; Chua, L.; Lowther, G. ψ-epistemic theories: The role of symmetry. Phys. Rev. A 2013, 88, 032111. [Google Scholar] [CrossRef]
- Patra, M.K.; Pironio, S.; Massar, S. No-go theorems for ψ-epistemic models based on a continuity assumption. Phys. Rev. Lett. 2013, 111, 090402. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Schlosshauer, M.; Fine, A. No-go theorem for the composition of quantum systems. Phys. Rev. Lett. 2014, 112, 070407. [Google Scholar] [CrossRef] [Green Version]
- Mansfield, S. Reality of the quantum state: Towards a stronger ψ-ontology theorem. Phys. Rev. A 2016, 94, 042124. [Google Scholar] [CrossRef] [Green Version]
- Leifer, M.S. Is the quantum state real? An extended review of ψ-ontology theorems. Quanta 2014, 3, 67–155. [Google Scholar] [CrossRef]
- Jennings, D.; Leifer, M. No return to classical reality. Contemp. Phys. 2016, 57, 60–82. [Google Scholar] [CrossRef] [Green Version]
- Leifer, M.S.; Pusey, M.F. Is a time symmetric interpretation of quantum theory possible without retrocausality? Proc. R. Soc. A Math. Phys. Eng. Sci. 2017, 473, 20160607. [Google Scholar] [CrossRef]
- Leifer, M.S. ψ-epistemic models are exponentially bad at explaining the distinguishability of quantum states. Phys. Rev. Lett. 2014, 112, 160404. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Barrett, J.; Cavalcanti, E.G.; Lal, R.; Maroney, O.J.E. No ψ-epistemic model can fully explain the indistinguishability of quantum states. Phys. Rev. Lett. 2014, 112, 250403. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Branciard, C. How ψ-epistemic models fail at explaining the indistinguishability of quantum states. Phys. Rev. Lett. 2014, 113, 020409. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Montina, A. Epistemic view of quantum states and communication complexity of quantum channels. Phys. Rev. Lett. 2012, 109, 110501. [Google Scholar] [CrossRef] [Green Version]
- Montina, A. Communication complexity and the reality of the wave function. Mod. Phys. Lett. A 2015, 30, 1530001. [Google Scholar] [CrossRef] [Green Version]
- Perry, C.; Jain, R.; Oppenheim, J. Communication tasks with infinite quantum-classical separation. Phys. Rev. Lett. 2015, 115, 030504. [Google Scholar] [CrossRef] [Green Version]
- Bandyopadhyay, S.; Jain, R.; Oppenheim, J.; Perry, C. Conclusive exclusion of quantum states. Phys. Rev. A 2014, 89, 022336. [Google Scholar] [CrossRef] [Green Version]
- Arunachalam, S.; Molina, A.; Russo, V. Quantum hedging in two-round prover-verifier interactions. arXiv 2017, arXiv:1310.7954. [Google Scholar]
- Myrvold, W.C. ψ-ontology result without the Cartesian product assumption. Phys. Rev. A 2018, 97, 052109. [Google Scholar] [CrossRef] [Green Version]
- Bennett, C.H.; Brassard, G.; Crépeau, C.; Jozsa, R.; Peres, A.; Wootters, W.K. Teleporting an unknown quantum state via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 1993, 70, 1895. [Google Scholar] [CrossRef] [Green Version]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Caves, C.M.; Fuchs, C.A.; Schack, R. Conditions for compatibility of quantum-state assignments. Phys. Rev. A 2002, 66, 062111. [Google Scholar] [CrossRef] [Green Version]
- Heinosaari, T.; Kerppo, O. Antidistinguishability of pure quantum states. J. Phys. A Math. Theor. 2018, 51, 365303. [Google Scholar] [CrossRef] [Green Version]
- Nigg, D.; Monz, T.; Schindler, P.; Martinez, E.A.; Hennrich, M.; Blatt, R.; Pusey, M.F.; Rudolph, T.; Barrett, J. Can different quantum state vectors correspond to the same physical state? An experimental test. New J. Phys. 2015, 18, 013007. [Google Scholar] [CrossRef]
- Miller, D.J. Alternative experimental protocol to demonstrate the Pusey–Barrett–Rudolph theorem. Phys. Rev. A 2013, 87, 014103. [Google Scholar] [CrossRef] [Green Version]
- Ringbauer, M.; Duffus, B.; Branciard, C.; Cavalcanti, E.G.; White, A.G.; Fedrizzi, A. Measurements on the reality of the wavefunction. Nat. Phys. 2015, 11, 249–254. [Google Scholar] [CrossRef] [Green Version]
- Liao, K.; Zhang, X.; Guo, G.; Ai, B.; Yan, H.; Zhu, S. Experimental test of the no-go theorem for continuous ψ-epistemic models. Sci. Rep. 2016, 6, 26519. [Google Scholar] [CrossRef] [Green Version]
- Li, W.; Li, C.; Guo, G. Probabilistic teleportation and entanglement matching. Phys. Rev. A 2000, 61, 034301. [Google Scholar] [CrossRef] [Green Version]
- Lu, H.; Guo, G. Teleportation of a two-particle entangled state via entanglement swapping. Phys. Lett. A 2000, 276, 209–212. [Google Scholar] [CrossRef]
- Agrawal, P.; Pati, A.K. Probabilistic quantum teleportation. Phys. Lett. A 2002, 305, 12–17. [Google Scholar] [CrossRef] [Green Version]
- Fortes, R.; Rigolin, G. Fighting noise with noise in realistic quantum teleportation. Phys. Rev. A 2015, 92, 012338. [Google Scholar] [CrossRef] [Green Version]
- Fortes, R.; Rigolin, G. Probabilistic quantum teleportation in the presence of noise. Phys. Rev. A 2016, 93, 062330. [Google Scholar] [CrossRef] [Green Version]
- Knoll, L.T.; Schmiegelow, C.T.; Larotonda, M.A. Noisy quantum teleportation: An experimental study on the influence of local environments. Phys. Rev. A 2014, 90, 042332. [Google Scholar] [CrossRef] [Green Version]
- Carlo, G.G.; Benenti, G.; Casati, G. Teleportation in a noisy environment: A quantum trajectories approach. Phys. Rev. Lett. 2003, 91, 257903. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kumar, D.; Pandey, P.N. Effect of noise on quantum teleportation. Phys. Rev. A 2013, 68, 012317. [Google Scholar] [CrossRef]
- Simon, C. Towards a global quantum network. Nat. Photonics 2017, 11, 678–680. [Google Scholar] [CrossRef] [Green Version]
- Ren, J.; Xu, P.; Yong, H.; Zhang, L.; Liao, S.; Yin, J.; Liu, W.; Cai, W.; Yang, M.; Li, L.; et al. Ground-to-satellite quantum teleportation. Nature 2017, 549, 70–73. [Google Scholar] [CrossRef] [Green Version]
- Azuma, K.; Tamaki, K.; Lo, H. All-photonic quantum repeaters. Nat. Commun. 2015, 6, 6787. [Google Scholar] [CrossRef] [Green Version]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rajan, D.; Visser, M. Quantum PBR Theorem as a Monty Hall Game. Quantum Rep. 2020, 2, 39-48. https://doi.org/10.3390/quantum2010003
Rajan D, Visser M. Quantum PBR Theorem as a Monty Hall Game. Quantum Reports. 2020; 2(1):39-48. https://doi.org/10.3390/quantum2010003
Chicago/Turabian StyleRajan, Del, and Matt Visser. 2020. "Quantum PBR Theorem as a Monty Hall Game" Quantum Reports 2, no. 1: 39-48. https://doi.org/10.3390/quantum2010003
APA StyleRajan, D., & Visser, M. (2020). Quantum PBR Theorem as a Monty Hall Game. Quantum Reports, 2(1), 39-48. https://doi.org/10.3390/quantum2010003