CFD-DEM Simulation for the Distribution and Motion Feature of Solid Particles in Single-Channel Pump
Abstract
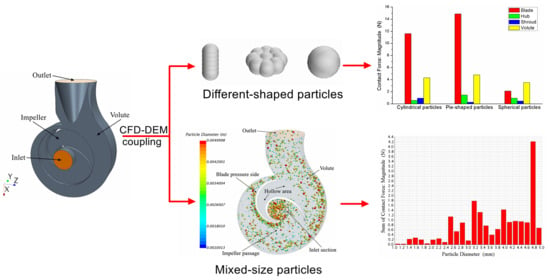
:1. Introduction
2. Methodology
2.1. Computational Domain and Meshing
2.2. Governing Equations
2.3. CFD-DEM Coupling
2.4. Particle Model
2.5. Fluid Phase Setup
3. Results and Discussion
3.1. Validation
3.2. Mixed-Sized Particle
3.2.1. Particle Trajectory and Distribution
3.2.2. Particle Velocity Distribution
3.2.3. Particle Contact Force Distribution
3.3. Different-Shaped Particles
3.3.1. Particle Trajectory and Distribution
3.3.2. Velocity Field of Liquid Phase
3.3.3. Collision Between Particle and Wall
4. Conclusions
- Particles tended to maintain a steady trajectory towards the volute that corresponds with the shape of the impeller blade. The smaller particles had a much more uniform distribution in the passages of the impeller and volute than the larger ones.
- The smaller-sized particles possessed a greater velocity distribution range and velocity peak but a smaller contact force compared with the larger particles. Besides this, there were apparent slip velocities between the liquid and solid-phase flows inside the pump.
- The trajectories of the cylindrical and pie-shaped particles in the impeller were close to the pressure side. However, the spherical particles were dispersed more uniformly in the impeller and volute than the other two cases.
- The pie-shaped particles had the most severe collisions, and the spherical particles had the least in total. The hub and shroud wall suffered a minor contact force, but the blade and volute wall both sustained a considerable contact force.
- In order to reduce wear, the blades and volute can be designed to have more wear resistance than other parts. Moreover, before entering the pump, the particles or foreign bodies can be made as round as possible to reduce wear.
- According to the limited particle model and flow parameters in this research, the other shapes and rigidity of particles with different fluid viscosities and other fluid properties could be factored into the CFD-DEM coupling method for future research.
Author Contributions
Funding
Conflicts of Interest
References
- AOKI, M. Instantaneous interblade pressure distributions and fluctuating radial thrust in a single-blade centrifugal pump. Bull. JSME 1984, 27, 2413–2420. [Google Scholar] [CrossRef]
- Benra, F.; Dohmen, H. Numerical and experimental evaluation of the time-variant flow field in a single-blade centrifugal pump. In Proceedings of the 5th International Conference on Heat Transfer, Fluid Mechanics and Thermodynamics, Sun City, South Africa, 1–4 July 2007. [Google Scholar]
- Benra, F.-K.; Dohmen, H.J. Investigation on the time-variant flow in a single-blade centrifugal pump. In Proceedings of the 5th WSEAS International Conference on Fluid Mechanics (FLUIDS’08), Acapulco, Mexico, 25–27 January 2008. [Google Scholar]
- Nishi, Y.; Matsuo, N.; Fukutomi, J. A study on internal flow in a new type of sewage pump. J. Fluid Sci. Technol. 2009, 4, 648–660. [Google Scholar] [CrossRef] [Green Version]
- Auvinen, M.; Ala-Juusela, J.; Pedersen, N.; Siikonen, T. Time-accurate turbomachinery simulations with Open-Source® CFD: Flow analysis of a single-channel pump with OpenFOAM®. In Proceedings of the ECCOMAS CFD, Lisbon, Portugal, 14–17 June 2010. [Google Scholar]
- Tsuji, Y.; Kawaguchi, T.; Tanaka, T. Discrete particle simulation of two-dimensional fluidized bed. Powder Technol. 1993, 77, 79–87. [Google Scholar] [CrossRef]
- Kafui, K.; Thornton, C.; Adams, M. Discrete particle-continuum fluid modelling of gas–solid fluidised beds. Chem. Eng. Sci. 2002, 57, 2395–2410. [Google Scholar] [CrossRef]
- Cleary, P.W.; Sawley, M.L. DEM modelling of industrial granular flows: 3D case studies and the effect of particle shape on hopper discharge. Appl. Math. Model. 2002, 26, 89–111. [Google Scholar] [CrossRef]
- Santamarina, J.; Cho, G.-C. Soil behaviour: The role of particle shape. In Advances in Geotechnical Engineering: The Skempton Conference: Proceedings of a Three Day Conference on Advances in Geotechnical Engineering, Organised by the Institution of Civil Engineers and Held at the Royal Geographical Society, London, UK, on 29–31 March 2004; Thomas Telford: London, UK, 2015; pp. 604–617. [Google Scholar]
- Pena, A.; Garcia-Rojo, R.; Herrmann, H.J. Influence of particle shape on sheared dense granular media. Granul. Matter 2007, 9, 279–291. [Google Scholar] [CrossRef] [Green Version]
- Huang, S.; Yang, F.; Su, X. Unsteady Numerical Simulation for Solid-Liquid Two-Phase Flow in Centrifugal Pump by CFD-DEM Coupling. Sci. Technol. Rev. 2014, 27, 15. [Google Scholar]
- Liu, D.; Tang, C.; Ding, S.; Fu, B. CFD-DEM Simulation for Distribution and Motion Feature of Crystal Particles in Centrifugal Pump. Int. J. Fluid Mach. Syst. 2017, 10, 378–384. [Google Scholar] [CrossRef]
- Yuanwen, L.; Shaojun, L.; Xiaozhou, H. Research on reflux in deep-sea mining pump based on DEM-CFD. Mar. Georesour. Geotechnol. 2020, 38, 744–752. [Google Scholar] [CrossRef]
- Lee, J.-G.; Kim, Y.-J. Effect of The Impeller Discharge Angle on the Performance of a Spurt Vacuum Pump. Appl. Sci. Converg. Technol. 2017, 26, 1–5. [Google Scholar] [CrossRef]
- Chu, K.; Yu, A. Numerical simulation of complex particle–fluid flows. Powder Technol. 2008, 179, 104–114. [Google Scholar] [CrossRef]
- Huang, S.; Su, X.; Qiu, G. Transient numerical simulation for solid-liquid flow in a centrifugal pump by DEM-CFD coupling. Eng. Appl. Comput. Fluid Mech. 2015, 9, 411–418. [Google Scholar] [CrossRef] [Green Version]
- Mindlin, R.D. Compliance of elastic bodies in contact. J. Appl. Mech. ASME 1949, 16, 259–268. [Google Scholar]
- Hertz, H. Ueber die Berührung fester elastischer Körper. J. Für Die Reine Und Angew. Math. 1882, 1882, 156–171. [Google Scholar]












| Grid Number | Head [m] | Deviation [%] |
|---|---|---|
| 250,493 | 18.09 | |
| 331,976 | 18.26 | 0.93 |
| 427,510 | 18.37 | 0.60 |
| 535,665 | 18.41 | 0.22 |
| 689,142 | 18.43 | 0.11 |
| Collision Coefficient | Particle-Particle | Particle-Wall |
|---|---|---|
| Restitution | 0.5 | 0.5 |
| Static friction | 0.61 | 0.8 |
| Rolling friction | 0.01 | 0.01 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, C.; Kim, Y.-J. CFD-DEM Simulation for the Distribution and Motion Feature of Solid Particles in Single-Channel Pump. Energies 2020, 13, 4988. https://doi.org/10.3390/en13194988
Tang C, Kim Y-J. CFD-DEM Simulation for the Distribution and Motion Feature of Solid Particles in Single-Channel Pump. Energies. 2020; 13(19):4988. https://doi.org/10.3390/en13194988
Chicago/Turabian StyleTang, Cheng, and Youn-Jea Kim. 2020. "CFD-DEM Simulation for the Distribution and Motion Feature of Solid Particles in Single-Channel Pump" Energies 13, no. 19: 4988. https://doi.org/10.3390/en13194988
APA StyleTang, C., & Kim, Y. -J. (2020). CFD-DEM Simulation for the Distribution and Motion Feature of Solid Particles in Single-Channel Pump. Energies, 13(19), 4988. https://doi.org/10.3390/en13194988