Thermoelectric Properties of Highly-Crystallized Ge-Te-Se Glasses Doped with Cu/Bi
Abstract
:1. Introduction
2. Materials and Methods
2.1. Reagents
2.2. Synthesis
2.3. Powder X-ray Diffraction
2.4. Hall Measurement
2.5. Electrical and Thermal Transport
2.6. Microscopic Analysis
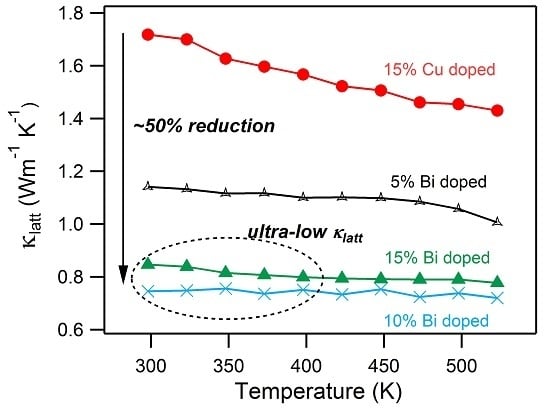
3. Results and Discussion
4. Conclusions
Supplementary Materials
Acknowledgments
Author Contributions
Conflicts of Interest
References
- LaLonde, A.D.; Pei, Y.; Wang, H.; Snyder, G.J. Lead telluride alloy thermoelectrics. Mater. Today 2011, 14, 526–532. [Google Scholar] [CrossRef]
- Dresselhaus, M.S.; Chen, G.; Tang, M.Y.; Yang, R.G.; Lee, H.; Wang, D.Z.; Ren, Z.F.; Fleurial, J.-P.; Gogna, P. New Directions for Low-Dimensional Thermoelectric Materials. Adv. Mater. 2007, 19, 1043–1053. [Google Scholar] [CrossRef]
- Li, J.-F.; Liu, W.-S.; Zhao, L.-D.; Zhou, M. High-performance nanostructured thermoelectric materials. NPG Asia Mater. 2010, 2, 152–158. [Google Scholar] [CrossRef]
- Terry, T.T. Thermoelectric Materials, Phenomena, and Applications: A Bird’s Eye View. MRS Bull. 2006, 31, 188–198. [Google Scholar]
- Snyder, G.J.; Toberer, E.S. Complex thermoelectric materials. Nat. Mater. 2008, 7, 105–114. [Google Scholar] [PubMed]
- Hicks, L.D.; Dresselhaus, M.S. Effect of quantum-well structures on the thermoelectric figure of merit. Phys. Rev. B 1993, 47, 12727–12731. [Google Scholar] [CrossRef]
- Sootsman, J.R.; Kong, H.; Uher, C.; D’Angelo, J.J.; Wu, C.-I.; Hogan, T.P.; Caillat, T.; Kanatzidis, M.G. Large Enhancements in the Thermoelectric Power Factor of Bulk PbTe at High Temperature by Synergistic Nanostructuring. Angew. Chem. Int. Ed. 2008, 47, 8618–8622. [Google Scholar] [CrossRef] [PubMed]
- Biswas, K.; He, J.; Blum, I.D.; Wu, C.-I.; Hogan, T.P.; Seidman, D.N.; Dravid, V.P.; Kanatzidis, M.G. High-performance bulk thermoelectrics with all-scale hierarchical architectures. Nature 2012, 489, 414–418. [Google Scholar] [CrossRef] [PubMed]
- Poudeu, P.F.P.; D’Angelo, J.; Downey, A.D.; Short, J.L.; Hogan, T.P.; Kanatzidis, M.G. High Thermoelectric Figure of Merit and Nanostructuring in Bulk p-type Na1−xPbmSbyTem+2. Angew. Chem. Int. Ed. 2006, 45, 3835–3839. [Google Scholar] [CrossRef] [PubMed]
- Biswas, K.; He, J.; Zhang, Q.; Wang, G.; Uher, C.; Dravid, V.P.; Kanatzidis, M.G. Strained endotaxial nanostructures with high thermoelectric figure of merit. Nat. Chem. 2011, 3, 160–166. [Google Scholar] [CrossRef] [PubMed]
- Cook, B.A.; Kramer, M.J.; Harringa, J.L.; Han, M.-K.; Chung, D.-Y.; Kanatzidis, M.G. Analysis of Nanostructuring in High Figure-of-Merit Ag1–xPbmSbTe2+m Thermoelectric Materials. Adv. Funct. Mater. 2009, 19, 1254–1259. [Google Scholar] [CrossRef]
- Poudeu, P.F.P.; Guéguen, A.; Wu, C.-I.; Hogan, T.; Kanatzidis, M.G. High Figure of Merit in Nanostructured n-Type KPbmSbTem+2 Thermoelectric Materials. Chem. Mater. 2010, 22, 1046–1053. [Google Scholar] [CrossRef]
- Zide, J.M.O.; Vashaee, D.; Bian, Z.X.; Zeng, G.; Bowers, J.E.; Shakouri, A.; Gossard, A.C. Demonstration of electron filtering to increase the Seebeck coefficient in InGaAs/InGaAlAs superlattices. Phys. Rev. B 2006, 74, 205335. [Google Scholar] [CrossRef]
- Banik, A.; Shenoy, U.S.; Anand, S.; Waghmare, U.V.; Biswas, K. Mg Alloying in SnTe Facilitates Valence Band Convergence and Optimizes Thermoelectric Properties. Chem. Mater. 2015, 27, 581–587. [Google Scholar] [CrossRef]
- Pei, Y.; Shi, X.; LaLonde, A.; Wang, H.; Chen, L.; Snyder, G.J. Convergence of electronic bands for high performance bulk thermoelectrics. Nature 2011, 473, 66–69. [Google Scholar] [PubMed]
- Banik, A.; Shenoy, U.S.; Saha, S.; Waghmare, U.V.; Biswas, K. High Power Factor and Enhanced Thermoelectric Performance of SnTe-AgInTe2: Synergistic Effect of Resonance Level and Valence Band Convergence. J. Am. Chem. Soc. 2016, 138, 13068–13075. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Q.; Liao, B.; Lan, Y.; Lukas, K.; Liu, W.; Esfarjani, K.; Opeil, C.; Broido, D.; Chen, G.; Ren, Z. High thermoelectric performance by resonant dopant indium in nanostructured SnTe. Proc. Natl. Acad. Sci. USA 2013, 110, 13261–13266. [Google Scholar] [CrossRef] [PubMed]
- Carruthers, P. Theory of Thermal Conductivity of Solids at Low Temperatures. Rev. Mod. Phys. 1961, 33, 92–138. [Google Scholar] [CrossRef]
- Zhao, W.; Wei, P.; Zhang, Q.; Dong, C.; Liu, L.; Tang, X. Enhanced Thermoelectric Performance in Barium and Indium Double-Filled Skutterudite Bulk Materials via Orbital Hybridization Induced by Indium Filler. J. Am. Chem. Soc. 2009, 131, 3713–3720. [Google Scholar] [CrossRef] [PubMed]
- Brown, S.R.; Kauzlarich, S.M.; Gascoin, F.; Snyder, G.J. Yb14MnSb11: New High Efficiency Thermoelectric Material for Power Generation. Chem. Mater. 2006, 18, 1873–1877. [Google Scholar] [CrossRef]
- Toberer, E.S.; May, A.F.; Snyder, G.J. Zintl Chemistry for Designing High Efficiency Thermoelectric Materials. Chem. Mater. 2010, 22, 624–634. [Google Scholar] [CrossRef]
- Kauzlarich, S.M.; Brown, S.R.; Snyder, G.J. Zintl phases for thermoelectric devices. Dalton Trans. 2007, 21, 2099–2107. [Google Scholar] [CrossRef] [PubMed]
- Venkatasubramanian, R.; Siivola, E.; Colpitts, T.; O’Quinn, B. Thin-film thermoelectric devices with high room-temperature figures of merit. Nature 2001, 413, 597–602. [Google Scholar] [CrossRef] [PubMed]
- Zhang, S.-N.; He, J.; Zhu, T.-J.; Zhao, X.-B.; Tritt, T.M. Thermal conductivity and specific heat of bulk amorphous chalcogenides Ge20Te80−xSex (x = 0, 1, 2, 8). J. Non-Cryst. Solids 2009, 355, 79–83. [Google Scholar] [CrossRef]
- Lucas, P.; Conseil, C.; Yang, Z.; Hao, Q.; Cui, S.; Boussard-Pledel, C.; Bureau, B.; Gascoin, F.; Caillaud, C.; Gulbiten, O.; et al. Thermoelectric bulk glasses based on the Cu–As–Te–Se system. J. Mater. Chem. A 2013, 1, 8917–8925. [Google Scholar] [CrossRef]
- Cui, S.; Boussard-plédel, C.; Calvez, L.; Rojas, F.; Chen, K.; Ning, H.; Reece, M.J.; Guizouarn, T.; Bureau, B. Comprehensive study of tellurium based glass ceramics for thermoelectric application. Adv. Appl. Ceram. 2015, 114, 42–47. [Google Scholar] [CrossRef]
- Zhu, T.J.; Yan, F.; Zhao, X.B.; Zhang, S.N.; Chen, Y.; Yang, S.H. Preparation and thermoelectric properties of bulk in situ nanocomposites with amorphous/nanocrystal hybrid structure. J. Phys. Appl. Phys. 2007, 40, 6094. [Google Scholar] [CrossRef]
- Gonçalves, A.P.; Lopes, E.B.; Delaizir, G.; Vaney, J.B.; Lenoir, B.; Piarristeguy, A.; Pradel, A.; Monnier, J.; Ochin, P.; Godart, C. Semiconducting glasses: A new class of thermoelectric materials? J. Solid State Chem. 2012, 193, 26–30. [Google Scholar] [CrossRef]
- Gonçalves, A.P.; Lopes, E.B.; Rouleau, O.; Godart, C. Conducting glasses as new potential thermoelectric materials: The Cu–Ge–Te case. J. Mater. Chem. 2010, 20, 1516–1521. [Google Scholar] [CrossRef]
- Vaney, J.B.; Delaizir, G.; Alleno, E.; Rouleau, O.; Piarristeguy, A.; Monnier, J.; Godart, C.; Ribes, M.; Escalier, R.; Pradel, A.; et al. A comprehensive study of the crystallization of Cu–As–Te glasses: Microstructure and thermoelectric properties. J. Mater. Chem. A 2013, 1, 8190–8200. [Google Scholar] [CrossRef]
- Vaney, J.B.; Piarristeguy, A.; Pradel, A.; Alleno, E.; Lenoir, B.; Candolfi, C.; Dauscher, A.; Gonçalves, A.P.; Lopes, E.B.; Delaizir, G.; et al. Thermal stability and thermoelectric properties of CuxAs40−xTe60−ySey semiconducting glasses. J. Solid State Chem. 2013, 203, 212–217. [Google Scholar] [CrossRef]
- Gonçalves, A.P.; Delaizir, G.; Lopes, E.B.; Ferreira, L.M.; Rouleau, O.; Godart, C. Chalcogenide Glasses as Prospective Thermoelectric Materials. J. Electron. Mater. 2011, 40, 1015–1017. [Google Scholar] [CrossRef]
- Perumal, S.; Roychowdhury, S.; Biswas, K. High performance thermoelectric materials and devices based on GeTe. J. Mater. Chem. C 2016, 4, 7520–7536. [Google Scholar] [CrossRef]
- Conseil, C.; Shiryaev, V.S.; Cui, S.; Boussard-Pledel, C.; Troles, J.; Velmuzhov, A.P.; Potapov, A.M.; Suchkov, A.I.; Churbanov, M.F.; Bureau, B. Preparation of High Purity Te-Rich Ge-Te-Se Fibers for 5–15 µm Infrared Range. J. Light. Technol. 2013, 31, 1703–1707. [Google Scholar] [CrossRef]
- Wilhelm, A.A.; Boussard-Plédel, C.; Coulombier, Q.; Lucas, J.; Bureau, B.; Lucas, P. Development of Far-Infrared-Transmitting Te Based Glasses Suitable for Carbon Dioxide Detection and Space Optics. Adv. Mater. 2007, 19, 3796–3800. [Google Scholar] [CrossRef]
- Rodríguez-Carvajal, J. Recent advances in magnetic structure determination by neutron powder diffraction. Phys. B Condens. Matter 1993, 192, 55–69. [Google Scholar] [CrossRef]
- Palatnik, L.; Komnik, Y.; Koshkin, V.; Belava, E. A group of ternary semiconducting compounds. Dokl. Akad. Nauk 1961, 137, 68–71. [Google Scholar]
- Banik, A.; Vishal, B.; Perumal, S.; Datta, R.; Biswas, K. The origin of low thermal conductivity in Sn1−xSbxTe: Phonon scattering via layered intergrowth nanostructures. Energy Environ. Sci. 2016, 9, 2011–2019. [Google Scholar] [CrossRef]
- Perumal, S.; Roychowdhury, S.; Biswas, K. Reduction of thermal conductivity through nanostructuring enhances the thermoelectric figure of merit in Ge1−xBixTe. Inorg. Chem. Front. 2016, 3, 125–132. [Google Scholar] [CrossRef]
- Wang, H.; LaLonde, A.D.; Pei, Y.; Snyder, G.J. The Criteria for Beneficial Disorder in Thermoelectric Solid Solutions. Adv. Funct. Mater. 2013, 23, 1586–1596. [Google Scholar] [CrossRef]
- Gelbstein, Y.; Davidow, J. Highly efficient functional GexPb1−xTe based thermoelectric alloys. Phys. Chem. Chem. Phys. 2014, 16, 20120–20126. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Q.; Cao, F.; Liu, W.; Lukas, K.; Yu, B.; Chen, S.; Opeil, C.; Broido, D.; Chen, G.; Ren, Z. Heavy Doping and Band Engineering by Potassium to Improve the Thermoelectric Figure of Merit in p-Type PbTe, PbSe, and PbTe1–ySey. J. Am. Chem. Soc. 2012, 134, 10031–10038. [Google Scholar] [CrossRef] [PubMed]
- Jiehe Sui, J.S. Effect of Cu concentration on thermoelectric properties of nanostructured p-type MgAg0.97−xCuxSb0.99. Acta Mater. 2015. [Google Scholar] [CrossRef]
- Yu, B.; Zhang, Q.; Wang, H.; Wang, X.; Wang, H.; Wang, D.; Wang, H.; Snyder, G.J.; Chen, G.; Ren, Z.F. Thermoelectric property studies on thallium-doped lead telluride prepared by ball milling and hot pressing. J. Appl. Phys. 2010, 108, 016104. [Google Scholar] [CrossRef]
- Ma, Y.; Hao, Q.; Poudel, B.; Lan, Y.; Yu, B.; Wang, D.; Chen, G.; Ren, Z. Enhanced Thermoelectric Figure-of-Merit in p-Type Nanostructured Bismuth Antimony Tellurium Alloys Made from Elemental Chunks. Nano Lett. 2008, 8, 2580–2584. [Google Scholar] [CrossRef] [PubMed]
- Kuznetsova, L.A.; Kuznetsov, V.L.; Rowe, D.M. Thermoelectric properties and crystal structure of ternary compounds in the Ge(Sn,Pb)Te–Bi2Te3 systems. J. Phys. Chem. Solids 2000, 61, 1269–1274. [Google Scholar] [CrossRef]
- Shelimova, L.E.; Karpinsky, O.G.; Kretova, M.A.; Avilov, E.S.; Fleurial, J.P. Crystal structure and thermoelectric properties of the mixed layered compounds of the (GeTe)m(Bi2Te3)m homologous series. In Proceedings of the XVI International Conference on Thermoelectrics 1997, Dresden, Germany, 26–29 August 1997; pp. 481–484. [Google Scholar]
- Lee, J.K.; Oh, M.W.; Kim, B.S.; Min, B.K.; Lee, H.W.; Park, S.D. Influence of Mn on crystal structure and thermoelectric properties of GeTe compounds. Electron. Mater. Lett. 2014, 10, 813–817. [Google Scholar] [CrossRef]
- Perumal, S.; Roychowdhury, S.; Negi, D.S.; Datta, R.; Biswas, K. High Thermoelectric Performance and Enhanced Mechanical Stability of p-type Ge1–xSbxTe. Chem. Mater. 2015, 27, 7171–7178. [Google Scholar]
- Fahrnbauer, F.; Souchay, D.; Wagner, G.; Oeckler, O. High Thermoelectric Figure of Merit Values of Germanium Antimony Tellurides with Kinetically Stable Cobalt Germanide Precipitates. J. Am. Chem. Soc. 2015, 137, 12633–12638. [Google Scholar] [CrossRef] [PubMed]
- Kim, H.-S.; Gibbs, Z.M.; Tang, Y.; Wang, H.; Snyder, G.J. Characterization of Lorenz number with Seebeck coefficient measurement. APL Mater. 2015, 3, 041506. [Google Scholar] [CrossRef]
- Zhang, L.; Wang, J.; Cheng, Z.; Sun, Q.; Li, Z.; Dou, S. Lead-free SnTe-based thermoelectrics: Enhancement of thermoelectric performance by doping with Gd/Ag. J. Mater. Chem. A 2016, 4, 7936–7942. [Google Scholar] [CrossRef]
- Inayat, S.B.; Rader, K.R.; Hussain, M.M. Nano-materials Enabled Thermoelectricity from Window Glasses. Sci. Rep. 2012, 2, 841. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Li, D.; Qin, X.; Yang, X.; Liu, Y.; Zhang, J.; Dou, Y.; Song, C.; Xin, H. Enhanced thermoelectric performance through carrier scattering at heterojunction potentials in BiSbTe based composites with Cu3SbSe4 nanoinclusions. J. Mater. Chem. C 2015, 3, 7045–7052. [Google Scholar] [CrossRef]
- LaLonde, A.D.; Pei, Y.; Snyder, G.J. Reevaluation of PbTe1−xIx as high performance n-type thermoelectric material. Energy Environ. Sci. 2011, 4, 2090–2096. [Google Scholar] [CrossRef]
- Hsu, K.F.; Loo, S.; Guo, F.; Chen, W.; Dyck, J.S.; Uher, C.; Hogan, T.; Polychroniadis, E.K.; Kanatzidis, M.G. Cubic AgPbmSbTe2+m: Bulk Thermoelectric Materials with High Figure of Merit. Science 2004, 303, 818–821. [Google Scholar] [CrossRef] [PubMed]
- Bali, A.; Wang, H.; Snyder, G.J.; Mallik, R.C. Thermoelectric properties of indium doped PbTe1−ySey alloys. J. Appl. Phys. 2014, 116, 033707. [Google Scholar] [CrossRef]








| M | X | Sample | Representation |
|---|---|---|---|
| Cu | 0 | Ge20Te77Se3 | GTS |
| 5 | (Ge20Te77Se3)95Cu5 | GTS-Cu05 | |
| 10 | (Ge20Te77Se3)90Cu10 | GTS-Cu10 | |
| 15 | (Ge20Te77Se3)85Cu15 | GTS-Cu15 | |
| Bi | 5 | (Ge20Te77Se3)95Bi5 | GTS-Bi05 |
| 10 | (Ge20Te77Se3)90Bi10 | GTS-Bi10 | |
| 15 | (Ge20Te77Se3)85Bi15 | GTS-Bi15 |
| Sample | Carrier Concentration n (cm−3) | Mobility, µ (cm2 V−1 s−1) |
|---|---|---|
| GTS-Cu15 | 2.81 × 1020 | 24.25 |
| GTS-Bi05 | 1.09 × 1020 | 36.5 |
| GTS-Bi10 | 2.38 × 1020 | 16.8 |
| GTS-Bi15 | 2.39 × 1020 | 25.57 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Srinivasan, B.; Boussard-Pledel, C.; Dorcet, V.; Samanta, M.; Biswas, K.; Lefèvre, R.; Gascoin, F.; Cheviré, F.; Tricot, S.; Reece, M.; et al. Thermoelectric Properties of Highly-Crystallized Ge-Te-Se Glasses Doped with Cu/Bi. Materials 2017, 10, 328. https://doi.org/10.3390/ma10040328
Srinivasan B, Boussard-Pledel C, Dorcet V, Samanta M, Biswas K, Lefèvre R, Gascoin F, Cheviré F, Tricot S, Reece M, et al. Thermoelectric Properties of Highly-Crystallized Ge-Te-Se Glasses Doped with Cu/Bi. Materials. 2017; 10(4):328. https://doi.org/10.3390/ma10040328
Chicago/Turabian StyleSrinivasan, Bhuvanesh, Catherine Boussard-Pledel, Vincent Dorcet, Manisha Samanta, Kanishka Biswas, Robin Lefèvre, Franck Gascoin, François Cheviré, Sylvain Tricot, Michael Reece, and et al. 2017. "Thermoelectric Properties of Highly-Crystallized Ge-Te-Se Glasses Doped with Cu/Bi" Materials 10, no. 4: 328. https://doi.org/10.3390/ma10040328
APA StyleSrinivasan, B., Boussard-Pledel, C., Dorcet, V., Samanta, M., Biswas, K., Lefèvre, R., Gascoin, F., Cheviré, F., Tricot, S., Reece, M., & Bureau, B. (2017). Thermoelectric Properties of Highly-Crystallized Ge-Te-Se Glasses Doped with Cu/Bi. Materials, 10(4), 328. https://doi.org/10.3390/ma10040328