Incorporation of Rubber Powder as Filler in a New Dry-Hybrid Technology: Rheological and 3D DEM Mastic Performances Evaluation
Abstract
:1. Introduction
- Rheological approach, which comprises a macro-scale laboratory analysis and a micro-scale simulation. In the first, Frequency Sweep tests (FS) have been implemented. In the second, a micromechanical modeling, able to predict the rheological behavior of mastics starting from the understanding of their internal interaction, has been used. Since Discrete particle Element Method (DEM) treats particles as distinct bodies that interact together at contact points, it can be a very useful tool [21,22,23,24,25]. The software Particle Flow Code (PFC) was used in this study [26]. The DEM simulation, in particular, has been used in order to evaluate the rubber effects and the interaction among limestone filler, rubber and bituminous matrix inside the mastics.
- Performance approach, which comprises Multiple Stress Creep Recovery (MSCR) tests, in order to evaluate the permanent deformation resistance of the mastics also in nonlinear analysis.
2. Materials
2.1. Mastics Design
2.2. Mastics Volumetric Analysis
2.3. Mastics Production
- limestone filler was inserted into a 160 °C oven for 24 h, to ensure moisture free particle surfaces;
- the bitumen was stored in a 5 L tin, preheated 7 h into a 160 °C oven, to make bitumen homogeneous and ready to mix;
- the accurate amount of bitumen was poured into a 1 L tin, and the tin with the bitumen was placed on a hot plate and kept at 160 °C;
- the bitumen was mechanically stirred for 30 s;
- the accurate mass of the limestone filler and crumb rubber was slowly added, and the mixing process followed so that fillers were homogeneously dispersed in the bitumen; and
- the obtained mastic was poured in a silicon mold (2 mm high and 8 mm diameter) and stored at 5 °C before starting the test.
3. Mastics Rheological Behavior
3.1. Macro-Scale Laboratory Analysis
3.2. DEM Micro-Scale Simulation
3.2.1. Introduction
3.2.2. Definition of the Model Geometry
3.2.3. Description of the Contact Materials Parameters
- specific heat at constant volume;
- coefficient of linear thermal expansion; and
- thermal resistance per unit length.
- in the first one, linear contact model was applied, in which the normal and shear stiffness of a discrete element chang
3.2.4. Boundary and Loading Conditions of the Frequency Sweep Test
3.2.5. Obtained Results
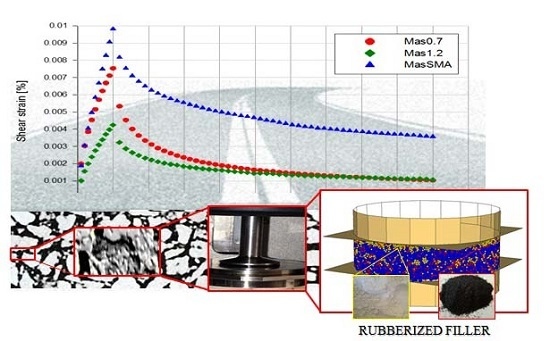
Macro-Scale Results
Micro-Scale Results
- origin in the center of the upper horizontal plane of the model, which simulates the oscillating plate;
- z direction coincident to the vertical axis of symmetry of the sample, pointing to the lower plate.
4. Mastic Resistance Performance
5. Conclusions
- The presence of fine crumb rubber and limestone filler improves the mastic stiffness at high temperatures, increasing the mastic rutting resistance. The frequency sweep tests and the Multiple Stress Creep Recovery have shown that at high temperatures the mastic containing crumb rubber and limestone filler has higher complex modulus than the one with only limestone filler.
- The fine crumb rubber reduces the mastic thermo-sensitivity, because at low temperatures the mastic containing both rubber and limestone filler not tends to glassy modulus, increasing the mastic resistance to thermal cracking.
- The Discrete particle Element Method is a valuable and hopeful tool to study the rheological behavior of asphalt mastics.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Sangiorgi, C.; Tataranni, P.; Simone, A.; Vignali, V.; Lantieri, C.; Dondi, G. Waste bleaching clays as fillers in hot bituminous mixtures. Construct. Build. Mater. 2014, 73, 320–325. [Google Scholar] [CrossRef]
- Mazzotta, F.; Sangiorgi, C.; Vignali, V.; Lantieri, C.; Dondi, G. Rheological characterization of bituminous mastics added with waste bleaching clays. In Proceedings of the 8th RILEM Symposium on Testing and Characterization of Sustainable and Innovative Bituminous Materials, Ancona, Italy, 7–9 October 2015.
- Dondi, G.; Sangiorgi, C.; Lantieri, C.; Simone, A.; Vignali, V.; Lamperti, R. Performance evaluation of Construction and Demolition and other waste materials. In Proceedings of the 3rd International Conference on Transportation Infrastructures, ICTI 2014, Pisa, Italy, 22–25 April 2014.
- Lamperti, R.; Grenfell, J.; Sangiorgi, C.; Lantieri, C.; Airey, G.D. Influence of waxes on adhesion properties of bituminous binders. Construct. Build. Mater. 2015, 76, 404–412. [Google Scholar] [CrossRef]
- Sangiorgi, C.; Tataranni, P.; Simone, A.; Vignali, V.; Lantieri, C.; Dondi, G. Assessment of waste bleaching clay as alternative filler for the production of Porous Asphalts. Construct. Build. Mater. 2016, 109, 1–7. [Google Scholar] [CrossRef]
- Lo Presti, D. Recycled Tyre Rubber Modified Bitumens for road asphalt mixtures: A literature review. Construct. Build. Mater. 2013, 49, 863–888. [Google Scholar] [CrossRef]
- Fontes, L.P.T.L.; Trichês, G.; Pais, J.C.; Pereira, P.A.A. Evaluating permanent deformation in asphalt rubber mixtures. Construct. Build. Mater. 2009, 24, 1193–1200. [Google Scholar] [CrossRef]
- Thodesen, C.; Xiao, F.; Amirkhanian, S.N. Modeling viscosity behavior of crumb rubber modified binders. Construct. Build. Mater. 2009, 23, 3053–3062. [Google Scholar] [CrossRef]
- Dondi, G.; Mazzotta, F.; Simone, A.; Vignali, V.; Sangiorgi, C.; Lantieri, C. Evaluation of different short term aging procedures with neat, warm and modified binder. Construct. Build. Mater. 2016, 106, 282–289. [Google Scholar] [CrossRef]
- Yildirim, Y.; Ideker, J.; Hazlett, D. Evaluation of viscosity values for mixing and compaction temperatures. J. Mater. Civ. Eng. 2006, 18, 545–553. [Google Scholar] [CrossRef]
- Wei, J.; Huang, X.; Zhang, Y. Influence of commercial wax on performance of Asphalt. J. Mater. Civ. Eng. 2010, 22, 760–766. [Google Scholar] [CrossRef]
- Oliveira, J.R.M.; Silva, H.M.R.D.; Abreu, L.P.F.; Fernandes, S.R.M. Use of a warm mix asphalt additive to reduce the production temperatures and to improve the performance of asphalt rubber mixtures. J. Clean. Prod. 2012, 41, 15–22. [Google Scholar] [CrossRef]
- Lougheed, T.J.; Papagiannakis, A.T. Viscosity characteristics of rubber-modified asphalts. J. Mater. Civ. Eng. 1996, 8, 153–156. [Google Scholar] [CrossRef]
- Xie, Z.; Shen, J. Performance of porous European mix (PEM) pavements added with crumb rubbers in dry process. Int. J. Pavement Eng. 2016, 17, 637–646. [Google Scholar] [CrossRef]
- U.S. Department of Health and Human Services—Public Health Service—Centers for Disease Control and Prevention. Hazard Review—Health Effects of Occupational Exposure to Asphalt; Publication No. 2001-110; NIOSH—Publications Dissemination: Cincinnati, ON, USA, 2000.
- Fornai, D.; Persici, V.; Lupi, C. Occupational Health Risk Assessment for the Workers Exposed to Rubberized Asphalt Fumes. Available online: http://www.ecopneus.it/_public-file/Occupational%20health%20risk%20assessment%20for%20the%20workers%20exposed%20to%20rubberized%20asphalt%20fumes.pdf (accessed on 14 October 2016).
- Woodward, D.; Millar, P.; Lantieri, C.; Sangiorgi, C.; Vignali, V. The wear of stone mastic asphalt due to slow speed high stress simulated laboratory trafficking. Construct. Build. Mater. 2016, 110, 270–277. [Google Scholar] [CrossRef]
- Praticò, F.G.; Vaiana, R. A study on volumetric versus surface properties of wearing courses. Construct. Build. Mater. 2013, 38, 766–775. [Google Scholar] [CrossRef]
- Vaiana, R.; Praticò, F.G.; Iuele, T.; Gallelli, V.; Minani, V. Effect of asphalt mix properties on surface texture: An experimental study. Appl. Mech. Mater. 2013, 368, 1056–1106. [Google Scholar] [CrossRef]
- Boscaino, G.; Pratico’, F.G.; Vaiana, R. Tyre/road noise on different road pavements: Synergetic influence of acoustical absorbing coefficient and surface texture. In Proceedings of the 10th EAEC European Congress, Belgrade, Serbia, 30 May–1 June 2005.
- Abbas, A.; Masad, E.; Papagiannakis, T.; Shenoy, A. Modelling asphalt mastic stiffness using discrete element analysis and micromechanics-based models. Int. J. Pavement Eng. 2005, 6, 137–146. [Google Scholar] [CrossRef]
- Zelelew, H.; Mahmoud, E.; Papagiannakis, T. Modeling asphalt concrete cyclic creep behavior. In Proceedings of the 3rd International Conference on Transportation Infrastructures, ICTI 2014, Pisa, Italy, 22–25 April 2014.
- Buttlar, W.G.; Bozkurt, D.; Al-Khateeb, G.; Waldhoff, A.S. Understanding asphalt mastic behaviour through micromechanics. Transp. Res. Rec. 1999, 1681, 157–169. [Google Scholar] [CrossRef]
- Dondi, G.; Simone, A.; Vignali, V.; Manganelli, G. Discrete particle element analysis of aggregate interaction in granular mixes for asphalt: Combined DEM and experimental study. In Proceedings of the 7th RILEM International Conference on Cracking in Pavements, Delft, The Netherlands, 20–22 June 2012.
- Majidi, B.; Taghavi, S.M.; Fafard, M.; Ziegler, D.P.; Alamdari, H. Discrete element method modeling of the rheological properties of coke/pitch mixtures. Materials 2016, 9, 5. [Google Scholar] [CrossRef]
- Particle Flow Code 3D; Itasca Consulting Group: Minneapolis, MN, USA, 2010.
- Capitolato Speciale D’appalto—Norme Tecniche Pavimentazioni Stradali/Autostradali; ANAS spa; ANAS spa: Rome, Italy, 2012.
- Bitumen and Bituminous Binders. Determination of Needle Penetration; EN 1426; British Standards Institution: London, UK, 2015.
- Bitumen and Bituminous Binders. Determination of the Softening Point. Ring and Ball Method; EN1427; British Standards Institution: London, UK, 2015.
- Bitumen and Bituminous Binders. Determination of the Tensile Properties of Modified Bitumen by the Force Ductility Method; EN13589; British Standards Institution: London, UK, 2008.
- Bitumen and Bituminous Binders. Determination of Dynamic Viscosity by Vacuum Capillary; EN12596; British Standards Institution: London, UK, 2007.
- Bitumen and Bituminous Binders. Determination of the Elastic Recovery of Modified Bitumen; EN13398; British Standards Institution: London, UK, 2007.
- Tests for Geometrical Properties of Aggregates. Assessment of Fines. Grading of Filler Aggregates (Air Jet Sieving); EN 933-10; British Standards Institution: London, UK, 2009.
- Tests for Mechanical and Physical Properties of Aggregates. Determination of the Particle Density of Filler. Pyknometer Method; EN 1097-7; British Standards Institution: London, UK, 2008.
- Tests for Mechanical and Physical Properties of Aggregates. Determination of the Voids of Dry Compacted Filler; EN 1097-4; British Standards Institution: London, UK, 2008.
- Prove Sugli Aggregati Complementari (Filler) Utilizzati Nelle Miscele Bituminose—Parte 1: Prova con Anello e Biglia; EN 13179-1; British Standards Institution: London, UK, 2013.
- Bitumen and Bituminous Binders—Determination of Complex Shear Modulus and Phase Angle—Dynamic Shear Rheometer (Dsr); EN 14770; British Standards Institution: London, UK, 2012.
- Dondi, G.; Mazzotta, F.; Sangiorgi, C.; Pettinari, M.; Simone, A.; Vignali, V.; Tataranni, P. Influence of cement and limestone filler on the rheological properties of mastic in cold bituminous recycled mixtures. In Proceedings of the 3rd International Conference on Transportation Infrastructures, ICTI 2014, Pisa, Italy, 22–25 April 2014.
- Tan, Y.; Guo, M. Study on the phase behavior of asphalt mastic. Construct. Build. Mater. 2013, 47, 311–317. [Google Scholar] [CrossRef]
- Trinkle, S.; Friedrich, C. Van Gurp-Palmen-plot: A way to characterize polydispersity of linear polymers. Rheol. Acta 2001, 40, 322–328. [Google Scholar] [CrossRef]
- Zheng, Q.; Du, M.; Yang, B.B.; Wu, G. Relationship between dynamic rheological behavior and phase separation of poly (methyl methacrylate)/poly (styrene-co-acrylonitrile). Polymer 2001, 42, 5743–5747. [Google Scholar] [CrossRef]
- Vignali, V.; Mazzotta, F.; Sangiorgi, C.; Simone, A.; Lantieri, C.; Dondi, G. Rheological and 3D DEM characterization of potential rutting of cold bituminous mastics. Construct. Build. Mater. 2014, 73, 339–349. [Google Scholar] [CrossRef]
- Wu, J.; Collop, A.C.; McDowell, G.R. Discrete element modeling of constant strain rate compression tests on idealized asphalt mixture. J. Mater. Civ. Eng. 2011, 23, 2–11. [Google Scholar] [CrossRef]
- Collop, A.C.; McDowell, G.R.; Lee, Y. Use of the distinct element method to model the deformation behavior of an idealized asphalt mixture. Int. J. Pavement Eng. 2004, 5, 1–7. [Google Scholar] [CrossRef]
- Kose, S.; Guler, M.; Bahia, H.H.; Masad, E. Distribution of strains within asphalt binders in HMA using imaging and finite element techniques. In Proceedings of the 79th Annual Meeting on Transportation Research Board, Washington, DC, USA, 9–13 January 2000.
- Hammoum, F.; De la Roche, C.; Piau, J.M.; Stefani, C. Experimental investigation of fracture and healing of bitumen at pseudo-contact of two aggregates. In Proceedings of the 9th International Conference on Asphalt Pavements, Copenhagen, Denmark, 17–22 August 2002.
- Collop, A.C.; McDowell, G.R.; Lee, Y. On the use of discrete element modelling to simulate the viscoelastic deformation behaviour of an idealized asphalt mixture. Geomech. Geoeng. 2007, 2, 77–86. [Google Scholar] [CrossRef]
- Abbas, A.; Masad, E.; Papagiannakis, T.; Shenoy, A. Micromechanical modelling of the viscoelastic behavior of asphalt mixtures using the discrete-element method. Int. J. Geomech. 2007, 7, 131–139. [Google Scholar] [CrossRef]
- Liu, Y.; You, Z. Simulation of cyclic loading tests for asphalt mixtures using user defined models within discrete element method. In Proceedings of the Geocongress, ASCE, Reston, VA, USA, 9–12 March 2008.
- Liu, Y.; Dai, Q.; You, Z. Viscoelastic model for discrete element simulation of asphalt mixtures. J. Eng. Mech. 2009, 135, 324–333. [Google Scholar] [CrossRef]
- Chen, J.; Pan, T.; Huang, X. Discrete element modeling of asphalt concrete cracking using a user-defined three-dimensional micromechanical approach. J. Wuhan Univ. Technol. Mater. 2011, 26, 1215–1221. [Google Scholar] [CrossRef]
- Adhikari, S.; You, Z. 3D discrete element models of the hollow cylindrical asphalt concrete specimens subject to the internal pressure. Int. J. Pavement Eng. 2010, 11, 429–439. [Google Scholar] [CrossRef]
- Zelelew, H.M. Simulation of the Permanent Deformation of Asphalt Concrete Mixtures Using Discrete Element Method (DEM). Ph.D. Thesis, Department of Civil and Environmental Engineering, Washington State University, Pullman, WA, USA, 2008. [Google Scholar]
- Abbas, A. Simulation of the Micromechanical Behavior of Asphalt Mixtures Using the Discrete Element Method. Ph.D. Thesis, Washington State University, Pullman, WA, USA, 2008. [Google Scholar]
- Baumgaertel, M.; Winter, H.H. Determination of discrete relaxation and retardation time spectra from dynamic mechanical data. Rheol. Acta 1989, 28, 511–559. [Google Scholar] [CrossRef]
- Standard Test Method for Multiple Stress Creep and Recovery (MSCR) of Asphalt Binder Using a Dynamic Shear Rheometer; ASTM D7405; ASTM International: West Conshohocken, PA, USA, 2015.











| SMA | Bitumen | Limestone Filler | Crumb Rubber |
|---|---|---|---|
| SMA | 6.60 | 9.00 | 0.00 |
| SMA1.2 | 8.50 | 5.00 | 1.20 |
| SMA0.7 | 7.50 | 5.00 | 0.75 |
| Property | Unit | Characteristic Value | Standard |
|---|---|---|---|
| Penetration @25 °C | dmm | 25 ÷ 55 | EN 1426 [28] |
| Softening Point | °C | 70 | EN 1427 [29] |
| Force Ductility test @10 °C | J/cm3 | 3 | EN 13589 [30] |
| Dynamic Viscosity @160 °C | Pa·s | 0.4 ÷ 0.7 | EN 12596 [31] |
| Elastic Recovery @25 °C | % | 80 | EN 13398 [32] |
| Property | Unit | Limestone Filler | Crumb Rubber | Standard |
|---|---|---|---|---|
| Particle size range | mm | 0 ÷ 0.063 | 0 ÷ 0.4 | EN 933-10 [33] |
| Particle density | Mg/m3 | 2.73 | 1.01 | EN 1097-7 [34] |
| Rigden voids | % | 33.82 | – | EN 1097-4 [35] |
| Δ Ring and Ball | °C | 8 | 12 | EN 13179-1 [36] |
| Mastic | Cf (%) | Cr (%) | Rf | Rr |
|---|---|---|---|---|
| MasSMA | 136 | 0 | 1.36 | 0.00 |
| Mas1.2 | 59 | 14 | 0.59 | 0.14 |
| Mas0.7 | 67 | 10 | 0.67 | 0.10 |
| Mastic | Vf (%) | Vr (%) | Vb (%) |
|---|---|---|---|
| MasSMA | 33 | 0 | 67 |
| Mas1.2 | 16 | 11 | 73 |
| Mas0.7 | 19 | 7 | 74 |
| Mastic | γ LVE (%) | Temperature (°C) |
|---|---|---|
| MasSMA | 0.5 | 10 |
| Mas1.2 | 0.8 | 10 |
| Mas0.7 | 0.8 | 10 |
| Property | Bitumen | Limestone Filler | Crumb Rubber |
|---|---|---|---|
| Specific heat at constant volume (J/kg·°C) | 1630 | 908 | 1737 |
| Coefficient of linear thermal expansion (1/°C) | 1.7 × 10−5 | 8 × 10−6 | 7 × 10−5 |
| Thermal resistance per unit length (°C/W·m) | 5.88 | 0.80 | 5.20 |
| Contact Model Parameter | “Solver” Value | ||
|---|---|---|---|
| MasSMA | Mas0.7 | Mas1.2 | |
| Stiffness for Kelvin section (MPa) | 0.13 | 0.10 | 0.19 |
| Stiffness for Maxwell section (MPa) | 22.67 | 5.51 | 13.90 |
| Viscosity for Kelvin section (MPa·s) | 6.97 | 2.10 | 7.72 |
| Viscosity for Maxwell section (MPa·s) | 44.50 | 37.46 | 144.59 |
| Property | G* | δ |
|---|---|---|
| MasSMA | 6.67 | 4.45 |
| Mas1.2 | 4.94 | 3.45 |
| Mas0.7 | 5.93 | 4.04 |
| Property | G* | δ | ||||
|---|---|---|---|---|---|---|
| LF | MF | HF | LF | MF | HF | |
| Mas1.2 | 6.15 | 5.66 | 3.20 | 4.00 | 3.41 | 3.10 |
| Mas0.7 | 7.14 | 6.94 | 3.51 | 4.78 | 4.07 | 3.45 |
| MasSMA | 9.30 | 7.39 | 3.84 | 5.10 | 4.44 | 4.04 |
| Temperature (°C) | 10 | 30 | 60 |
|---|---|---|---|
| MasSMA | 2.809 | 6.173 | 11.357 |
| Mas0.7 | 2.015 | 6.012 | 14.567 |
| Mas1.2 | 2.477 | 8.138 | 17.323 |
| Temperature (°C) | Mastic | xdisp inf | xdisp sup | ydisp inf | ydisp sup | zdisp inf | zdisp sup |
|---|---|---|---|---|---|---|---|
| 10 | MasSMA | 0.000039 | 0.95 | 0.000038 | 1.10 | 0.000001 | 0.11 |
| Mas0.7 | 0.000041 | 1.29 | 0.000042 | 1.22 | 0.000001 | 0.14 | |
| Mas1.2 | 0.000040 | 1.31 | 0.000040 | 1.29 | 0.000001 | 0.14 | |
| 30 | MasSMA | 0.000049 | 1.50 | 0.000045 | 1.40 | 0.000003 | 0.30 |
| Mas0.7 | 0.000050 | 1.60 | 0.000048 | 1.70 | 0.000004 | 0.41 | |
| Mas1.2 | 0.000043 | 1.40 | 0.000044 | 1.35 | 0.000002 | 0.20 | |
| 60 | MasSMA | 0.000060 | 2.10 | 0.000056 | 2.20 | 0.000007 | 0.80 |
| Mas0.7 | 0.000055 | 1.80 | 0.000052 | 2.01 | 0.000006 | 0.67 | |
| Mas1.2 | 0.000047 | 1.70 | 0.000045 | 1.80 | 0.000005 | 0.50 |
| Temperature (°C) | Mastic | Disp A-A 0.01 Hz | Disp A-A 10 Hz | ∆ |
|---|---|---|---|---|
| 10 | MasSMA | 0.295 | 0.580 | 0.285 |
| Mas0.7 | 0.560 | 0.796 | 0.131 | |
| Mas1.2 | 0.665 | 0.595 | 0.035 | |
| 30 | MasSMA | 0.950 | 1.060 | 0.225 |
| Mas0.7 | 0.835 | 1.195 | 0.115 | |
| Mas1.2 | 1.080 | 0.980 | 0.030 | |
| 60 | MasSMA | 1.160 | 1.475 | 0.470 |
| Mas0.7 | 1.145 | 1.265 | 0.120 | |
| Mas1.2 | 1.005 | 1.180 | 0.020 |
| Mastic | 0.1 kPa | 3.2 kPa |
|---|---|---|
| MasSMA | 40 | 20 |
| Mas1.2 | 87 | 32 |
| Mas0.7 | 61 | 25 |
| Mastic | 0.1 kPa | 3.2 kPa |
|---|---|---|
| MasSMA | 0.03 | 0.08 |
| Mas1.2 | 0.01 | 0.02 |
| Mas0.7 | 0.01 | 0.03 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vignali, V.; Mazzotta, F.; Sangiorgi, C.; Simone, A.; Lantieri, C.; Dondi, G. Incorporation of Rubber Powder as Filler in a New Dry-Hybrid Technology: Rheological and 3D DEM Mastic Performances Evaluation. Materials 2016, 9, 842. https://doi.org/10.3390/ma9100842
Vignali V, Mazzotta F, Sangiorgi C, Simone A, Lantieri C, Dondi G. Incorporation of Rubber Powder as Filler in a New Dry-Hybrid Technology: Rheological and 3D DEM Mastic Performances Evaluation. Materials. 2016; 9(10):842. https://doi.org/10.3390/ma9100842
Chicago/Turabian StyleVignali, Valeria, Francesco Mazzotta, Cesare Sangiorgi, Andrea Simone, Claudio Lantieri, and Giulio Dondi. 2016. "Incorporation of Rubber Powder as Filler in a New Dry-Hybrid Technology: Rheological and 3D DEM Mastic Performances Evaluation" Materials 9, no. 10: 842. https://doi.org/10.3390/ma9100842
APA StyleVignali, V., Mazzotta, F., Sangiorgi, C., Simone, A., Lantieri, C., & Dondi, G. (2016). Incorporation of Rubber Powder as Filler in a New Dry-Hybrid Technology: Rheological and 3D DEM Mastic Performances Evaluation. Materials, 9(10), 842. https://doi.org/10.3390/ma9100842