Modeling Orbital Error in InSAR Interferogram Using Frequency and Spatial Domain Based Methods
Abstract
:1. Introduction
2. Methods
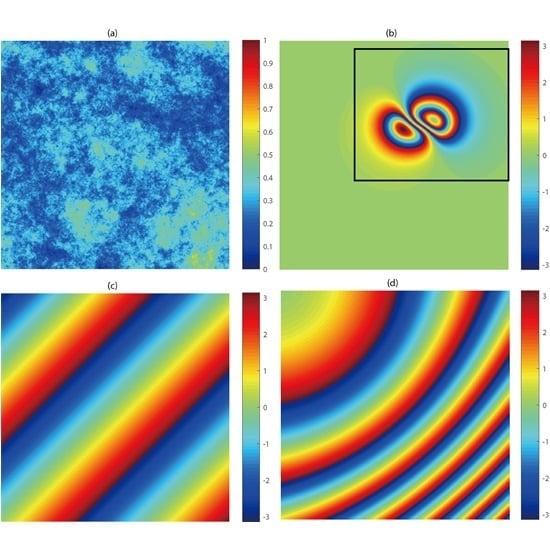
2.1. Modeling Orbital Error in Frequency Domain
2.2. Modeling Orbital Error in Spatial Domain
2.2.1. Preprocess: Multi-Looking and Manually Masking
2.2.2. Polynomial Model
2.2.3. Iteratively Reweighted Least Squares Fitting
2.2.4. Model Selection
- Split data into subsamples with equivalent size .
- For , set validation data to be the subsample, and training data to be the other subsamples.
- Fit each model to and evaluate its performance on through weighted root-mean-square error (WRMSE).
- Pick and that leads to minimum WRMSE by averaging results.
3. Results
3.1. Synthetic Data
3.2. Real Data
3.2.1. Datong Area
3.2.2. Tohoku-Oki Area
4. Discussion
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Berardino, P.; Fornaro, G.; Lanari, R.; Sansosti, E. A new algorithm for surface deformation monitoring based on small baseline differential sar interferograms. IEEE Trans. Geosci. Remote Sens. 2002, 40, 2375–2383. [Google Scholar] [CrossRef]
- Ferretti, A.; Prati, C.; Rocca, F. Permanent scatterers in sar interferometry. IEEE Trans. Geosci. Remote Sens. 2001, 39, 8–20. [Google Scholar] [CrossRef]
- Massonnet, D.; Feigl, K.L. Radar interferometry and its application to changes in the earth’s surface. Rev. Geophys. 1998, 36, 441–500. [Google Scholar] [CrossRef]
- Diao, F.; Xiong, X.; Wang, R.; Zheng, Y.; Walter, T.R.; Weng, H.; Li, J. Overlapping post-seismic deformation processes: Afterslip and viscoelastic relaxation following the 2011 Mw9.0 Tohoku (Japan) earthquake. Geophys. J. Int. 2013, 196, 218–229. [Google Scholar] [CrossRef]
- Copley, A.; Hollingsworth, J.; Bergman, E. Constraints on fault and lithosphere rheology from the coseismic slip and postseismic afterslip of the 2006 Mw7.0 Mozambique earthquake. J. Geophys. Res. Solid Earth 2012, 117. [Google Scholar] [CrossRef]
- Liu, Y.; Xu, C.; Li, Z.; Wen, Y.; Chen, J.; Li, Z. Time-dependent afterslip of the 2009 Mw6.3 Dachaidan earthquake (China) and viscosity beneath the qaidam basin inferred from postseismic deformation observations. Remote Sens. 2016, 8, 649. [Google Scholar] [CrossRef]
- Fattahi, H.; Amelung, F. Insar uncertainty due to orbital errors. Geophys. J. Int. 2014, 199, 549–560. [Google Scholar] [CrossRef]
- Thiel, C.; Schmullius, C. Impact of tree species on magnitude of palsar interferometric coherence over siberian forest at frozen and unfrozen conditions. Remote Sens. 2014, 6, 1124–1136. [Google Scholar] [CrossRef]
- Zebker, H.A.; Villasenor, J. Decorrelation in interferometric radar echoes. IEEE Trans. Geosci. Remote Sens. 1992, 30, 950–959. [Google Scholar] [CrossRef]
- Ding, X.-L.; Li, Z.-W.; Zhu, J.-J.; Feng, G.-C.; Long, J.-P. Atmospheric effects on insar measurements and their mitigation. Sensors 2008, 8, 5426–5448. [Google Scholar] [CrossRef] [PubMed]
- Jiang, M.; Li, Z.; Ding, X.; Zhu, J.-J.; Feng, G. Modeling minimum and maximum detectable deformation gradients of interferometric sar measurements. Int. J. Appl. Earth Obs. Geoinf. 2011, 13, 766–777. [Google Scholar] [CrossRef]
- Xu, B.; Li, Z.-W.; Wang, Q.-J.; Jiang, M.; Zhu, J.-J.; Ding, X.-L. A refined strategy for removing composite errors of sar interferogram. IEEE Geosci. Remote Sens. Lett. 2014, 11, 143–147. [Google Scholar] [CrossRef]
- Rosen, P.A.; Hensley, S.; Peltzer, G.; Simons, M. Updated repeat orbit interferometry package released. Eos Trans. Am. Geophys. Union 2004, 85, 47. [Google Scholar] [CrossRef]
- Knedlik, S.; Loffeld, O.; Hein, A.; Arndt, C. A novel approach to accurate baseline estimation. In Proceedings of the IEEE 1999 International Geoscience and Remote Sensing Symposium (IGARSS’99), Hamburg, Germany, 28 June–2 July 1999; pp. 254–256. [Google Scholar]
- Pepe, A.; Berardino, P.; Bonano, M.; Euillades, L.D.; Lanari, R.; Sansosti, E. Sbas-based satellite orbit correction for the generation of dinsar time-series: Application to radarsat-1 data. IEEE Trans. Geosci. Remote Sens. 2011, 49, 5150–5165. [Google Scholar] [CrossRef]
- Kohlhase, A.; Feigl, K.; Massonnet, D. Applying differential inSAR to orbital dynamics: A new approach for estimating ERS trajectories. J. Geod. 2003, 77, 493–502. [Google Scholar] [CrossRef]
- Biggs, J.; Wright, T.; Lu, Z.; Parsons, B. Multi-interferogram method for measuring interseismic deformation: Denali fault, Alaska. Geophys. J. Int. 2007, 170, 1165–1179. [Google Scholar] [CrossRef]
- Bähr, H.; Hanssen, R.F. Reliable estimation of orbit errors in spaceborne SAR interferometry. J. Geod. 2012, 86, 1147–1164. [Google Scholar] [CrossRef]
- Wang, H.; Wright, T.; Biggs, J. Interseismic slip rate of the northwestern Xianshuihe fault from inSAR data. Geophys. Res. Lett. 2009, 36. [Google Scholar] [CrossRef]
- Feng, G.; Ding, X.; Li, Z.; Jiang, M.; Zhang, L.; Omura, M. Calibration of an insar-derived coseimic deformation map associated with the 2011 Mw-9.0 Tohoku-oki earthquake. IEEE Geosci. Remote Sens. Lett. 2012, 9, 302–306. [Google Scholar] [CrossRef]
- Béjar-Pizarro, M.; Socquet, A.; Armijo, R.; Carrizo, D.; Genrich, J.; Simons, M. Andean structural control on interseismic coupling in the north Chile subduction zone. Nat. Geosci. 2013, 6, 462–467. [Google Scholar] [CrossRef]
- Gourmelen, N.; Amelung, F.; Lanari, R. Interferometric synthetic aperture radar–GPS integration: Interseismic strain accumulation across the Hunter Mountain fault in the eastern California shear zone. J. Geophys. Res. Solid Earth 2010, 115. [Google Scholar] [CrossRef] [Green Version]
- Shirzaei, M.; Walter, T.R. Estimating the effect of satellite orbital error using wavelet-based robust regression applied to inSAR deformation data. IEEE Trans. Geosci. Remote Sens. 2011, 49, 4600–4605. [Google Scholar] [CrossRef]
- Bähr, H. Orbital Effects in Spaceborne Synthetic Aperture Radar Interferometry; KIT Scientific Publishing: Karlsruhe, Germany, 2013. [Google Scholar]
- Zebker, H.A.; Chen, K. Accurate estimation of correlation in inSAR observations. IEEE Geosci. Remote Sens. Lett. 2005, 2, 124–127. [Google Scholar] [CrossRef]
- Spagnolini, U. 2-D phase unwrapping and instantaneous frequency estimation. IEEE Trans. Geosci. Remote Sens. 1995, 33, 579–589. [Google Scholar] [CrossRef]
- Jiang, M.; Ding, X.; Li, Z. Hybrid approach for unbiased coherence estimation for multitemporal inSAR. IEEE Trans. Geosci. Remote Sens. 2014, 52, 2459–2473. [Google Scholar] [CrossRef]
- Holland, P.W.; Welsch, R.E. Robust regression using iteratively reweighted least-squares. Commun. Stat. Theory Methods 1977, 6, 813–827. [Google Scholar] [CrossRef]
- Bishop, C.M. Pattern Recognition and Machine Learning; Springer: Berlin, Germany, 2006. [Google Scholar]
- Okada, Y. Surface deformation due to shear and tensile faults in a half-space. Bull. Seismol. Soc. Am. 1985, 75, 1135–1154. [Google Scholar]
- Jiang, M.; Ding, X.; Li, Z.; Tian, X.; Zhu, W.; Wang, C.; Xu, B. The improvement for baran phase filter derived from unbiased inSAR coherence. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2014, 7, 3002–3010. [Google Scholar] [CrossRef]
- Hanssen, R.F. Radar Interferometry: Data Interpretation and Error Analysis; Springer: New York, NY, USA, 2001; Volume 2. [Google Scholar]
- Jiang, M.; Yong, B.; Tian, X.; Malhotra, R.; Hu, R.; Li, Z.; Yu, Z.; Zhang, X. The potential of more accurate insar covariance matrix estimation for land cover mapping. ISPRS J. Photogramm. Remote Sens. 2017, 126, 120–128. [Google Scholar] [CrossRef]
- Chen, C.W.; Zebker, H.A. Phase unwrapping for large SAR interferograms: Statistical segmentation and generalized network models. IEEE Trans. Geosci. Remote Sens. 2002, 40, 1709–1719. [Google Scholar] [CrossRef]
- Jónsson, S.; Zebker, H.; Segall, P.; Amelung, F. Fault slip distribution of the 1999 Mw7.1 Hector mine, California, earthquake, estimated from satellite radar and GPS measurements. Bull. Seismol. Soc. Am. 2002, 92, 1377–1389. [Google Scholar] [CrossRef]
- Feigl, K.L.; Thurber, C.H. A method for modelling radar interferograms without phase unwrapping: Application to the M 5 Fawnskin, California earthquake of 1992 December 4. Geophys. J. Int. 2009, 176, 491–504. [Google Scholar] [CrossRef]







| Location | Sensor | Track | Master (yyyy-mm-dd) | Slave (yyyy-mm-dd) | (m) | Pass | Char. Def. |
|---|---|---|---|---|---|---|---|
| Datong | GF-3 | - | 2017-04-01 | 2017-06-27 | 536 | D | local |
| Datong | Sentinel-1 | 40 | 2015-10-15 | 2015-10-27 | 87 | A | local |
| Tohoku-Oki | ASAR | 347 | 2011-02-19 | 2011-03-21 | 163 | D | global |
| Tohoku-Oki | ASAR | 74 | 2011-03-02 | 2011-04-01 | −121 | D | global |
| Sensor | Track | Unit Vector of LOS [East North Up] | Number of GPS Station | RMSE before Correction (cm) | RMSE after Correction (cm) | RMSE Reduction (%) |
|---|---|---|---|---|---|---|
| ASAR | 347 | [0.64 0.11 0.75] | 97 | 35.55 | 9.52 | 73.22 |
| ASAR | 74 | [0.65 0.11 0.75] | 23 | 12.24 | 8.39 | 31.45 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, X.; Malhotra, R.; Xu, B.; Qi, H.; Ma, Y. Modeling Orbital Error in InSAR Interferogram Using Frequency and Spatial Domain Based Methods. Remote Sens. 2018, 10, 508. https://doi.org/10.3390/rs10040508
Tian X, Malhotra R, Xu B, Qi H, Ma Y. Modeling Orbital Error in InSAR Interferogram Using Frequency and Spatial Domain Based Methods. Remote Sensing. 2018; 10(4):508. https://doi.org/10.3390/rs10040508
Chicago/Turabian StyleTian, Xin, Rakesh Malhotra, Bing Xu, Haoping Qi, and Yuxiao Ma. 2018. "Modeling Orbital Error in InSAR Interferogram Using Frequency and Spatial Domain Based Methods" Remote Sensing 10, no. 4: 508. https://doi.org/10.3390/rs10040508
APA StyleTian, X., Malhotra, R., Xu, B., Qi, H., & Ma, Y. (2018). Modeling Orbital Error in InSAR Interferogram Using Frequency and Spatial Domain Based Methods. Remote Sensing, 10(4), 508. https://doi.org/10.3390/rs10040508