Global Assessment of the GNSS Single Point Positioning Biases Produced by the Residual Tropospheric Delay
Abstract
:1. Introduction
2. Influences of Residual Tropospheric Delays on Single Point Positioning
3. Materials and Methods
3.1. ZTD Model
3.1.1. Combined Model
3.1.2. Empirical Model
3.2. Products
3.2.1. VMF-ZTD
3.2.2. IGS-ZTD
3.3. Data Processing Strategy
4. Result
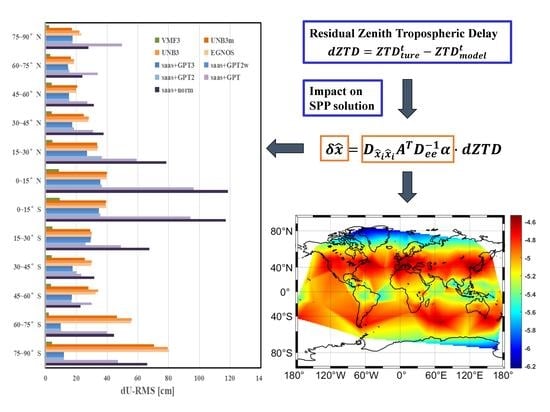
4.1. The Residual Zenith Tropospheric Delay (dZTD)
4.2. The Impacts of the Residual Zenith Tropospheric Delay on the SPP Solution
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zheng, F.; Gu, S.; Gong, X.; Lou, Y.; Fan, L.; Shi, C. Real-Time Single-Frequency Pseudorange Positioning in China Based on Regional Satellite Clock and Ionospheric Models. GPS Solut. 2020, 24, 1–13. [Google Scholar] [CrossRef]
- Tregoning, P.; Herring, T.A. Impact of a Priori Zenith Hydrostatic Delay Errors on GPS Estimates of Station Heights and Zenith Total Delays. Geophys. Res. Lett. 2006, 33. [Google Scholar] [CrossRef] [Green Version]
- Janes, H.W.; Langley, R.B.; Newby, S.P. Analysis of Tropospheric Delay Prediction Models: Comparisons with Ray-Tracing and Implications for GPS Relative Positioning. Bull. Géodésique 1991, 65, 151–161. [Google Scholar] [CrossRef]
- Davis, J.L.; Herring, T.A.; Shapiro, I.I.; Rogers, A.E.E.; Elgered, G. Geodesy by Radio Interferometry: Effects of Atmospheric Modeling Errors on Estimates of Baseline Length. Radio Sci. 1985, 20, 1593–1607. [Google Scholar] [CrossRef]
- Jin, S.G.; Luo, O.; Ren, C. Effects of Physical Correlations on Long-Distance GPS Positioning and Zenith Tropospheric Delay Estimates. Adv. Space Res. 2010, 46, 190–195. [Google Scholar] [CrossRef]
- Kačmařík, M.; Douša, J.; Dick, G.; Zus, F.; Brenot, H.; Möller, G.; Pottiaux, E.; Kapłon, J.; Hordyniec, P.; Václavovic, P.; et al. Inter-Technique Validation of Tropospheric Slant Total Delays. Atmos. Meas. Tech. 2017, 10, 2183–2208. [Google Scholar] [CrossRef] [Green Version]
- Hopfield, H.S. Two-Quartic Tropospheric Refractivity Profile for Correcting Satellite Data. J. Geophys. Res. 1969, 74, 4487–4499. [Google Scholar] [CrossRef]
- Saastamoinen, J. Introduction to Practical Computation of Astronomical Refraction. Bull. Geod. 1972, 106, 383–397. [Google Scholar] [CrossRef]
- Leandro, R.F.; Langley, R.B.; Santos, M.C. UNB3m_pack: A Neutral Atmosphere Delay Package for Radiometric Space Techniques. GPS Solut. 2008, 12, 65–70. [Google Scholar] [CrossRef]
- Penna, N.; Dodson, A.; Chen, W. Assessment of EGNOS Tropospheric Correction Model. J. Navig. 2001, 54, 37–55. [Google Scholar] [CrossRef] [Green Version]
- Landskron, D.; Böhm, J. VMF3/GPT3: Refined Discrete and Empirical Troposphere Mapping Functions. J. Geod. 2018, 92, 349–360. [Google Scholar] [CrossRef] [PubMed]
- Hofmeister, A.; Böhm, J. Application of Ray-Traced Tropospheric Slant Delays to Geodetic VLBI Analysis. J. Geod. 2017, 91, 945–964. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yao, Y.; Hu, Y.; Yu, C.; Zhang, B.; Guo, J. An Improved Global Zenith Tropospheric Delay Model GZTD2 Considering Diurnal Variations. Nonlin. Process. Geophys. 2016, 23, 127–136. [Google Scholar] [CrossRef] [Green Version]
- Li, W.; Yuan, Y.; Ou, J.; Chai, Y.; Li, Z.; Liou, Y.-A.; Wang, N. New Versions of the BDS/GNSS Zenith Tropospheric Delay Model IGGtrop. J. Geod. 2015, 89, 73–80. [Google Scholar] [CrossRef]
- Schüler, T. The TropGrid2 Standard Tropospheric Correction Model. GPS Solut. 2014, 18, 123–131. [Google Scholar] [CrossRef]
- Yao, Y.; Xu, C.; Shi, J.; Cao, N.; Zhang, B.; Yang, J. ITG: A New Global GNSS Tropospheric Correction Model. Sci. Rep. 2015, 5, 10273. [Google Scholar] [CrossRef] [Green Version]
- Dousa, J.; Elias, M. An Improved Model for Calculating Tropospheric Wet Delay. Geophys. Res. Lett. 2014, 41, 4389–4397. [Google Scholar] [CrossRef]
- Li, W.; Yuan, Y.; Ou, J.; He, Y. IGGtrop_SH and IGGtrop_rH: Two Improved Empirical Tropospheric Delay Models Based on Vertical Reduction Functions. IEEE Trans. Geosci. Remote Sens. 2018, 56, 5276–5288. [Google Scholar] [CrossRef]
- Xu, C.; Yao, Y.; Shi, J.; Zhang, Q.; Peng, W. Development of Global Tropospheric Empirical Correction Model with High Temporal Resolution. Remote Sens. 2020, 12, 721. [Google Scholar] [CrossRef] [Green Version]
- Hofmeister, A. Determination of Path Delays in the Atmosphere for Geodetic VLBI by Means of Ray-Tracing. Ph.D. Thesis, Technischen Universitat Wien Fakultat fur Mathematik und Geoinformation, Vienna, Austria, 2016. [Google Scholar]
- Meunram, P.; Satirapod, C. Spatial Variation of Precipitable Water Vapor Derived from GNSS CORS in Thailand. Geod. Geodyn. 2019, 10, 140–145. [Google Scholar] [CrossRef]
- Tuka, A.; El-Mowafy, A. Performance Evaluation of Different Troposphere Delay Models and Mapping Functions. Measurement 2013, 46, 928–937. [Google Scholar] [CrossRef] [Green Version]
- Liu, J.; Chen, X.; Sun, J.; Liu, Q. An Analysis of GPT2/GPT2w+Saastamoinen Models for Estimating Zenith Tropospheric Delay over Asian Area. Adv. Space Res. 2017, 59, 824–832. [Google Scholar] [CrossRef]
- Zhang, H.; Yuan, Y.; Li, W.; Li, Y.; Chai, Y. Assessment of Three Tropospheric Delay Models (IGGtrop, EGNOS and UNB3m) Based on Precise Point Positioning in the Chinese Region. Sensors 2016, 16, 122. [Google Scholar] [CrossRef] [Green Version]
- Yao, Y.; Xu, X.; Xu, C.; Peng, W.; Wan, Y. GGOS Tropospheric Delay Forecast Product Performance Evaluation and Its Application in Real-Time PPP. J. Atmos. Sol. Terr. Phys. 2018, 175, 1–17. [Google Scholar] [CrossRef]
- Yuan, Y.; Holden, L.; Kealy, A.; Choy, S.; Hordyniec, P. Assessment of Forecast Vienna Mapping Function 1 for Real-Time Tropospheric Delay Modeling in GNSS. J. Geod. 2019, 93, 1501–1514. [Google Scholar] [CrossRef]
- Li, F.; Zhang, Q.; Zhang, S.; Lei, J.; Li, W. Evaluation of Spatio-Temporal Characteristics of Different Zenith Tropospheric Delay Models in Antarctica. Radio Sci. 2020, 55, e2019RS006909. [Google Scholar] [CrossRef]
- Nie, Z.; Liu, F.; Gao, Y. Real-Time Precise Point Positioning with a Low-Cost Dual-Frequency GNSS Device. GPS Solut. 2020, 24, 1–11. [Google Scholar] [CrossRef]
- Xu, G.; Xu, Y. GPS Theory, Algorithms and Applications; Springer: Berlin/Heidelberg, Germany, 2016; ISBN 978-3-662-50365-2. [Google Scholar]
- Boehm, J.; Heinkelmann, R.; Schuh, H. Short Note: A Global Model of Pressure and Temperature for Geodetic Applications. J. Geod. 2007, 81, 679–683. [Google Scholar] [CrossRef]
- Lagler, K.; Schindelegger, M.; Böhm, J.; Krásná, H.; Nilsson, T. GPT2: Empirical Slant Delay Model for Radio Space Geodetic Techniques: GPT2: EMPIRICAL SLANT DELAY MODEL. Geophys. Res. Lett. 2013, 40, 1069–1073. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Böhm, J.; Möller, G.; Schindelegger, M.; Pain, G.; Weber, R. Development of an Improved Empirical Model for Slant Delays in the Troposphere (GPT2w). GPS Solut. 2015, 19, 433–441. [Google Scholar] [CrossRef] [Green Version]
- Collins, P.; Langley, R.; LaMance, J. Limiting Factors in Tropospheric Propagation Delay Error Modelling for GPS Airborne Navigation. In Proceedings of the institute of navigation 52nd annual meeting, Cambridge, MA, USA, 19–21 June 1996; p. 10. [Google Scholar]
- Boehm, J. Vienna Mapping Functions in VLBI Analyses. Geophys. Res. Lett. 2004, 31, L01603. [Google Scholar] [CrossRef]
- Kouba, J. Implementation and Testing of the Gridded Vienna Mapping Function 1 (VMF1). J. Geod. 2008, 82, 193–205. [Google Scholar] [CrossRef]
- Dow, J.M.; Neilan, R.E.; Rizos, C. The International GNSS Service in a Changing Landscape of Global Navigation Satellite Systems. J. Geod. 2009, 83, 191–198. [Google Scholar] [CrossRef]
- Byun, S.H.; Bar-Sever, Y.E. A New Type of Troposphere Zenith Path Delay Product of the International GNSS Service. J. Geod. 2009, 83, 1–7. [Google Scholar] [CrossRef] [Green Version]
- Dach, R.; Lutz, S.; Walser, P.; Fridez, P. (Eds.) Bernese GNSS Software Version 5.2; User Manual, Astronomical Institute; University of Bern, Bern Open Publishing: Bern, Switzerland, 2015; ISBN 978-3-906813-05-9. [Google Scholar] [CrossRef]
- Hackman, C.; Guerova, G.; Byram, S.; Dousa, J.; Hugentobler, U. International GNSS Service (IGS) troposphere products and working group activities. FIG Working Week. In Proceedings of the Wisdom of the Ages to the Challenges of the Modern World, Sofia, Bulgaria, 17–21 May 2015; pp. 1–14. [Google Scholar]
- Villiger, A.; Dach, R. (Eds.) International GNSS Service Technical Report 2018 (IGS Annual Report). IGS Cent. Bur. Univ. Bern 2019. [Google Scholar] [CrossRef]
- Boehm, J.; Niell, A.; Tregoning, P.; Schuh, H. Global Mapping Function (GMF): A New Empirical Mapping Function Based on Numerical Weather Model Data. Geophys. Res. Lett. 2006, 33, L07304. [Google Scholar] [CrossRef] [Green Version]
- Liangke, H.; Lilong, L.; Chaolong, Y. A Zenith Tropospheric Delay Correction Model Based on the Regional CORS Network. Geod. Geodyn. 2012, 3, 53–62. [Google Scholar] [CrossRef] [Green Version]











| Meteorological Models | Data Sources | Representation | Temporal Variability | Output Parameters |
|---|---|---|---|---|
| norm | a set of standard meteorological parameters on sea level | / | / | P, T, e |
| GPT | meteorological reanalysis data ERA-40 for 3 years from 1999 to 2002 | Spherical harmonics up to degree and order 9 at mean sea level | mean and annual terms | P, T, (ah, aw) |
| GPT2 | 10-year meteorological reanalysis data ERA-Interim | 5° × 5° global grid | mean, annual and semi-annual terms | P, T, dT, e, (ah, aw) |
| GPT2w | monthly mean pressure-level data of ERA-Interim fields by the ECMWF | 5° × 5° and 1° × 1° global grid | mean, annual and semi-annual terms | P, T, e, Tm, λ, (ah, aw) |
| GPT3 | 10 years of ECMWF ERA-Interim re-analysis data | 5° × 5° and 1° × 1° global grid | mean, annual and semi-annual terms | P, T, e, Tm, λ, (ah, aw), (, , , ) |
| EGNOS | UNB3 | UNB3m | |
|---|---|---|---|
| five meteorological parameters | P, T, e, , | P, T, e, , | P, T, RH, , |
| Input parameters | height (H), latitude () and day of year (DOY) | ||
| Output parameters | ZTD | ||
| Remarks | Simplification of UNB3 | / | Improved wet delay calculation accuracy based on UNB3 |
| VMF-ZTD | IGS-ZTD | |
|---|---|---|
| Data Sources | ECMWF ERA-Interim re-analysis data | GNSS observation data |
| Time resolution | 6 h | 5 min |
| Calculation method | Ray-tracing | PPP (software: Bernese GNSS Software 5.2; mapping function: GMF) |
| Spring | Summer | Autumn | Winter | ||||
|---|---|---|---|---|---|---|---|
| DOY | num | DOY | num | DOY | num | DOY | num |
| 80 | 386 | 172 | 404 | 266 | 374 | 356 | 376 |
| 81 | 374 | 173 | 400 | 267 | 372 | 357 | 377 |
| 82 | 390 | 174 | 401 | 268 | 372 | 358 | 380 |
| 83 | 392 | 175 | 403 | 269 | 373 | 359 | 379 |
| 84 | 382 | 176 | 394 | 270 | 377 | 360 | 377 |
| 85 | 381 | 177 | 394 | 271 | 381 | 361 | 382 |
| 86 | 386 | 178 | 399 | 272 | 384 | 362 | 380 |
| Models | Mean | RMS | ||||
|---|---|---|---|---|---|---|
| N (cm) | E (cm) | U (cm) | N (cm) | E (cm) | U (cm) | |
| saas+norm | 0.16 | 0.04 | −35.60 | 4.08 | 3.31 | 44.55 |
| saas+GPT | −0.08 | 0.01 | −8.51 | 3.55 | 2.80 | 38.22 |
| saas+GPT2 | 0.03 | 0.01 | −1.20 | 1.97 | 1.49 | 20.37 |
| saas+GPT2w | 0.03 | 0.01 | −3.13 | 1.86 | 1.40 | 19.14 |
| saas+GPT3 | 0.03 | 0.01 | −3.13 | 1.86 | 1.40 | 19.14 |
| EGNOS | −0.02 | 0.00 | 7.83 | 2.54 | 1.88 | 26.11 |
| UNB3 | −0.03 | 0.00 | 8.03 | 2.56 | 1.89 | 26.28 |
| UNB3m | 0.04 | 0.01 | 1.81 | 2.42 | 1.78 | 24.75 |
| VMF3 | −0.01 | 0.00 | 0.79 | 0.59 | 0.43 | 5.93 |
| Altitude | <100 m | 100~300 m | 300~500 m | 500~1000 m | 1000~2000 m | >2000 m |
|---|---|---|---|---|---|---|
| Num | 185 | 80 | 27 | 58 | 43 | 15 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, L.; Wang, J.; Li, H.; Balz, T. Global Assessment of the GNSS Single Point Positioning Biases Produced by the Residual Tropospheric Delay. Remote Sens. 2021, 13, 1202. https://doi.org/10.3390/rs13061202
Yang L, Wang J, Li H, Balz T. Global Assessment of the GNSS Single Point Positioning Biases Produced by the Residual Tropospheric Delay. Remote Sensing. 2021; 13(6):1202. https://doi.org/10.3390/rs13061202
Chicago/Turabian StyleYang, Ling, Jinfang Wang, Haojun Li, and Timo Balz. 2021. "Global Assessment of the GNSS Single Point Positioning Biases Produced by the Residual Tropospheric Delay" Remote Sensing 13, no. 6: 1202. https://doi.org/10.3390/rs13061202
APA StyleYang, L., Wang, J., Li, H., & Balz, T. (2021). Global Assessment of the GNSS Single Point Positioning Biases Produced by the Residual Tropospheric Delay. Remote Sensing, 13(6), 1202. https://doi.org/10.3390/rs13061202