Electrical Double Percolation of Polybutadiene/Polyethylene Glycol Blends Loaded with Conducting Polymer Nanofibers
Abstract
:1. Introduction
2. Materials and Methods
2.1. Preparation of PB/PEG Blends Loaded with P3HT Nanofibers
2.2. Characterization of PB/PEG Blends Loaded with P3HT Nanofibers
3. Results and Discussion
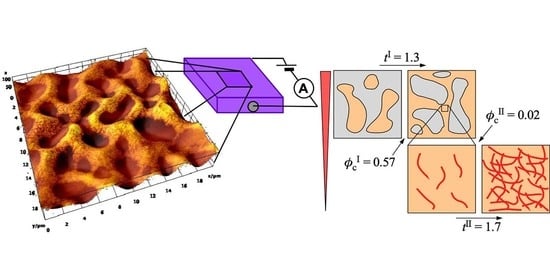
3.1. Selective Localization of P3HT Nanofibers in PB/PEG Blends
3.2. Electrical Conductivity of PB/PEG Blends Loaded with P3HT Nanofiber
3.3. Field-Effect Mobility of PB/PEG Blends Loaded with P3HT Nanofiber
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Sumita, M.; Sakata, K.; Asai, S.; Miyasaka, K.; Nakagawa, H. Dispersion of fillers and the electrical-conductivity of polymer blends filled with carbon-black. Polym. Bull. 1991, 25, 265–271. [Google Scholar] [CrossRef]
- Sumita, M.; Sakata, K.; Hayakawa, Y.; Asai, S.; Miyasaka, K.; Tanemura, M. Double percolation effect on the electrical-conductivity of conductive particles filled polymer blends. Colloid Polym. Sci. 1992, 270, 134–139. [Google Scholar] [CrossRef]
- Gubbels, F.; Blacher, S.; Vanlathem, E.; Jerome, R.; Deltour, R.; Brouers, F.; Teyssie, P. Design of electrical conductive composites—Key role of the morphology on the electrical-properties of carbon-black filled polymer blends. Macromolecules 1995, 28, 1559–1566. [Google Scholar] [CrossRef]
- Gubbels, F.; Jerome, R.; Vanlathem, E.; Deltour, R.; Blacher, S.; Brouers, F. Kinetic and thermodynamic control of the selective localization of carbon black at the interface of immiscible polymer blends. Chem. Mater. 1998, 10, 1227–1235. [Google Scholar] [CrossRef]
- Thongruang, W.; Balik, C.M.; Spontak, R.J. Volume-exclusion effects in polyethylene blends filled with carbon black, graphite, or carbon fiber. J. Polym. Sci. Polym. Phys. 2002, 40, 1013–1025. [Google Scholar] [CrossRef]
- Dai, K.; Xu, X.B.; Li, Z.M. Electrically conductive carbon black (CB) filled in situ microfibrillar poly(ethylene terephthalate) (PET)/polyethylene (PE) composite with a selective CB distribution. Polymer 2007, 48, 849–859. [Google Scholar] [CrossRef]
- Yui, H.; Wu, G.Z.; Sano, H.; Sumita, M.; Kino, K. Morphology and electrical conductivity of injection-molded polypropylene/carbon black composites with addition of high-density polyethylene. Polymer 2006, 47, 3599–3608. [Google Scholar] [CrossRef]
- Tan, Y.Q.; Song, Y.H.; Cao, Q.; Zheng, Q.A. Characterization of carbon black-filled immiscible polypropylene/polystyrene blends. Polym. Int. 2011, 60, 823–832. [Google Scholar] [CrossRef]
- Shi, Y.Y.; Yang, J.H.; Huang, T.; Zhang, N.; Chen, C.; Wang, Y. Selective localization of carbon nanotubes at the interface of poly(L-lactide)/ethylene-co-vinyl acetate resulting in lowered electrical resistivity. Compos. Part B Eng. 2013, 55, 463–469. [Google Scholar] [CrossRef]
- Patra, R.; Suin, S.; Mandal, D.; Khatua, B.B. Sequential mixing as effective method in the reduction of percolation threshold of multiwall carbon nanotube in poly(methyl methacrylate)/high-density poly(ethylene)/MWCNT nanocomposites. J. Appl. Polym. Sci. 2014, 131, 40235. [Google Scholar] [CrossRef]
- Roman, C.; Garcia-Morales, M.; Gupta, J.; McNally, T. On the phase affinity of multi-walled carbon nanotubes in PMMA: LDPE immiscible polymer blends. Polymer 2017, 118, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Yang, Y.; Li, L.Y.; Yin, B.; Yang, M.B. An effective strategy to achieve ultralow electrical percolation threshold with CNTs anchoring at the interface of PVDF/PS Bi-continuous structures to form an interfacial conductive layer. Macromol. Mater. Eng. 2020, 305, 1900835. [Google Scholar] [CrossRef]
- Roman, C.; Garcia-Morales, M.; Olariu, M.A.; McNally, T. Effect of selective distribution of MWCNTs on the solid-state rheological and dielectric properties of blends of PMMA and LDPE. J. Mater. Sci. 2020, 55, 8526–8540. [Google Scholar] [CrossRef]
- Sethy, S.; Samantara, L.; Satapathy, B.K. Phase-selective micro-structural effects on rheological-networks, segmental relaxation, and electrical conductivity behavior of melt-mixed polyamide-12/polypropylene-multi walled carbon nanotubes ternary nanocomposites. Polym. Eng. Sci. 2020, 1–15. [Google Scholar] [CrossRef]
- Schenning, A.P.; Meijer, E.W. Supramolecular electronics; nanowires from self-assembled pi-conjugated systems. Chem. Commun. 2005, 3245–3258. [Google Scholar] [CrossRef]
- Aleshin, A.N. Quasi-one-dimensional transport in conducting polymer nanowires. Phys. Solid State 2007, 49, 2015–2033. [Google Scholar] [CrossRef]
- Briseno, A.; Mannsfeld, S.; Jenekhe, S.; Bao, Z.; Xia, Y. Introducing organic nanowire transistors. Mater. Today 2008, 11, 38–47. [Google Scholar] [CrossRef]
- Ihn, K.J.; Moulton, J.; Smith, P. Whiskers of poly(3-alkylthiophene)s. J. Polym. Sci. Polym. Phys. 1993, 31, 735–742. [Google Scholar] [CrossRef]
- Merlo, J.A.; Frisbie, C.D. Field effect conductance of conducting polymer nanofibers. J. Polym. Sci. Polym. Phys. 2003, 41, 2674–2680. [Google Scholar] [CrossRef]
- Merlo, J.A.; Frisbie, C.D. Field effect transport and trapping in regioregular polythiophene nanofibers. J. Phys. Chem. B 2004, 108, 19169–19179. [Google Scholar] [CrossRef]
- Samitsu, S.; Shimomura, T.; Heike, S.; Hashizume, T.; Ito, K. Effective production of poly(3-alkylthiophene) nanofibers by means of whisker method using anisole solvent: Structural, optical, and electrical properties. Macromolecules 2008, 41, 8000–8010. [Google Scholar] [CrossRef]
- Oosterbaan, W.D.; Bolsée, J.-C.; Gadisa, A.; Vrindts, V.; Bertho, S.; D’Haen, J.; Cleij, T.J.; Lutsen, L.; McNeill, C.R.; Thomsen, L.; et al. Alkyl-chain-length-independent hole mobility via morphological control with poly(3-alkylthiophene) nanofibers. Adv. Funct. Mater. 2010, 20, 792–802. [Google Scholar] [CrossRef]
- Samitsu, S.; Shimomura, T.; Heike, S.; Hashizume, T.; Ito, K. Field-effect carrier transport in poly(3-alkylthiophene) nanofiber networks and isolated nanofibers. Macromolecules 2010, 43, 7891–7894. [Google Scholar] [CrossRef]
- Shimomura, T.; Takahashi, T.; Ichimura, Y.; Nakagawa, S.; Noguchi, K.; Heike, S.; Hashizume, T. Relationship between structural coherence and intrinsic carrier transport in an isolated poly(3-hexylthiophene) nanofiber. Phys. Rev. B 2011, 83. [Google Scholar] [CrossRef]
- Qiu, L.; Lim, J.A.; Wang, X.; Lee, W.H.; Hwang, M.; Cho, K. Versatile use of vertical-phase-separation-induced bilayer structures in organic thin-film transistors. Adv. Mater. 2008, 20, 1141–1145. [Google Scholar] [CrossRef]
- Qiu, L.; Lee, W.H.; Wang, X.; Kim, J.S.; Lim, J.A.; Kwak, D.; Lee, S.; Cho, K. Organic thin-film transistors based on polythiophene nanowires embedded in insulating polymer. Adv. Mater. 2009, 21, 1349–1353. [Google Scholar] [CrossRef]
- Lim, J.A.; Kim, J.-H.; Qiu, L.; Lee, W.H.; Lee, H.S.; Kwak, D.; Cho, K. Inkjet-printed single-droplet organic transistors based on semiconductor nanowires embedded in insulating polymers. Adv. Funct. Mater. 2010, 20, 3292–3297. [Google Scholar] [CrossRef]
- Kim, F.S.; Jenekhe, S.A. Charge transport in poly(3-butylthiophene) nanowires and their nanocomposites with an insulating polymer. Macromolecules 2012, 45, 7514–7519. [Google Scholar] [CrossRef]
- Yoshida, K.-I.; Kawasaki, M.; Toda, Y.; Yamashita, S.; Shimomura, T. Microscopic conduction pathways of poly(3-hexylthiophene) nanofibers embedded in polymer film. Polym. J. 2012, 44, 371–374. [Google Scholar] [CrossRef]
- Benetti, E.M.; Causin, V.; Maggini, M. Conjugated polymers in cages: Templating poly(3-hexylthiophene) nanocrystals by inert gel matrices. Adv. Mater. 2012, 24, 5636–5641. [Google Scholar] [CrossRef]
- Aronggaowa, B.; Toda, Y.; Ito, N.; Shikinaka, K.; Shimomura, T. Transparent conductive films fabricated from polythiophene nanofibers composited with conventional polymers. Polymers 2013, 5, 1325–1338. [Google Scholar] [CrossRef] [Green Version]
- Yagi, M.; Ito, N.; Kawasaki, M.; Shimomura, T. Semiconducting properties of p- and n-type organic nanofiber/poly (methyl methacrylate) composite films for film rectifier. Synth. Met. 2016, 213, 1–6. [Google Scholar] [CrossRef]
- Lu, G.; Tang, H.; Huan, Y.; Li, S.; Li, L.; Wang, Y.; Yang, X. Enhanced charge transportation in semiconducting polymer/insulating polymer composites: The role of an interpenetrating bulk interface. Adv. Funct. Mater. 2010, 20, 1714–1720. [Google Scholar] [CrossRef]
- Stauffer, D.; Aharony, A. Introduction to Percolation Theory; Taylor and Francis: London, UK, 1992. [Google Scholar]
- Scher, H.; Zallen, R. Critical density in percolation processes. J. Chem. Phys. 1970, 53, 3759–3761. [Google Scholar] [CrossRef] [Green Version]
- Bauhofer, W.; Kovacs, J.Z. A review and analysis of electrical percolation in carbon nanotube polymer composites. Compos. Sci. Technol. 2009, 69, 1486–1498. [Google Scholar] [CrossRef]
- Menon, M.; Yoon, C.O.; Moses, D.; Heeger, A.J. Metal-insulator transition in doped conducting polymer. In Handbook of Conducting Polymer, 2nd ed.; Skotheim, T.A., Elsenbaumer, R.L., Reynolds, J.R., Eds.; Marcel Decker: New York, NY, USA, 1998; pp. 27–121. [Google Scholar]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Morita, J.; Goto, T.; Kanehashi, S.; Shimomura, T. Electrical Double Percolation of Polybutadiene/Polyethylene Glycol Blends Loaded with Conducting Polymer Nanofibers. Polymers 2020, 12, 2658. https://doi.org/10.3390/polym12112658
Morita J, Goto T, Kanehashi S, Shimomura T. Electrical Double Percolation of Polybutadiene/Polyethylene Glycol Blends Loaded with Conducting Polymer Nanofibers. Polymers. 2020; 12(11):2658. https://doi.org/10.3390/polym12112658
Chicago/Turabian StyleMorita, Jun, Takanori Goto, Shinji Kanehashi, and Takeshi Shimomura. 2020. "Electrical Double Percolation of Polybutadiene/Polyethylene Glycol Blends Loaded with Conducting Polymer Nanofibers" Polymers 12, no. 11: 2658. https://doi.org/10.3390/polym12112658
APA StyleMorita, J., Goto, T., Kanehashi, S., & Shimomura, T. (2020). Electrical Double Percolation of Polybutadiene/Polyethylene Glycol Blends Loaded with Conducting Polymer Nanofibers. Polymers, 12(11), 2658. https://doi.org/10.3390/polym12112658