Durability and Service Life Prediction of Fluorocarbon Elastomer under Thermal Environments
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Aging Test
2.3. Analysis
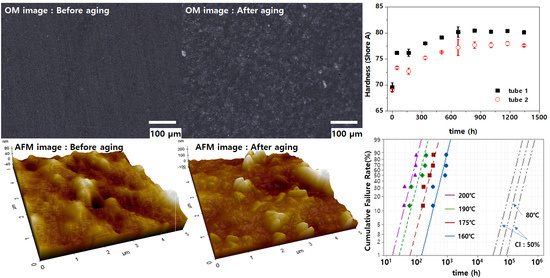
3. Results and Discussion
3.1. TGA-FTIR Analysis
3.2. Surface Morphology
3.3. Mechanical Properties
3.4. Service Life Prediction
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Drobny, J.G. Fluoroelastomers Handbook; Elsevier: Cambridge, MA, USA, 2016. [Google Scholar]
- Drobny, J.G. Fluoroelastomers. In Introduction to Fluoropolymers; Ebnesajjad, S., Ed.; Elsevier: Boston, MA, USA, 2013; pp. 150–193. [Google Scholar]
- Lee, P.-C.; Kim, S.Y.; Jeoung, S.K.; Wei, S.-H.; Ko, Y.K.; Ha, J.U.; Lee, J.-Y.; Kim, M. Lifetime Prediction of Fluoroelastomer Using Accelerated Aging Test. Polym. Korea 2021, 45, 910–914. [Google Scholar] [CrossRef]
- Starkova, O.; Gagani, A.I.; Karl, C.W.; Rocha, I.B.C.M.; Burlakovs, J.; Krauklis, A.E. Modelling of Environmental Ageing of Polymers and Polymer Composites—Durability Prediction Methods. Polymers 2022, 14, 907. [Google Scholar] [CrossRef] [PubMed]
- Plota, A.; Masek, A. Lifetime Prediction Methods for Degradable Polymeric Materials—A Short Review. Materials 2020, 13, 4507. [Google Scholar] [CrossRef] [PubMed]
- Lee, P.-C.; Um, C.; Jeoung, S.K.; Ko, Y.K.; Ha, J.U.; Lee, J.-Y.; Lee, J.Y.; Kim, K.S.; Kim, D.H. Accelerated Thermal Aging of Natural Fiber/Polyproylene Composites for Automotive Interior Parts. Polym. Korea 2021, 45, 922–926. [Google Scholar] [CrossRef]
- Kong, E.; Yoon, B.; Nam, J.-D.; Suhr, J. Accelerated Aging and Lifetime Prediction of Graphene-Reinforced Natural Rubber Composites. Macromol. Res. 2018, 26, 998–1003. [Google Scholar] [CrossRef]
- Awaja, F.; Zhang, S.; Tripathi, M.; Nikiforov, A.; Pugno, N. Cracks, microcracks and fracture in polymer structures: Formation, detection, autonomic repair. Prog. Mater. Sci. 2016, 83, 536–573. [Google Scholar] [CrossRef]
- ISO, the International Organization for Standardization. Rubber, Vulcanized or Thermoplastic—Estimation of Life-Time and Maximum Temperature of Use (Standard No. ISO 11346:2014); International Organization for Standardization: Geneva, Switzerland, 2014; pp. 1–10. [Google Scholar]
- Escobar, L.A.; Meeker, W.Q. A Review of Accelerated Test Models. Stat. Sci. 2006, 21, 552–577. [Google Scholar] [CrossRef] [Green Version]
- Zhang, W.; Zang, Y.; Lu, Y.; Lin, W.; Zhao, S.; Xiong, J. Thermal Decomposition of Brominated Butyl Rubber. Materials 2021, 14, 6767. [Google Scholar] [CrossRef] [PubMed]
- Bouaziz, R.; Truffault, L.; Borisov, R.; Ovalle, C.; Laiarinandrasana, L.; Miquelard-Garnier, G.; Fayolle, B. Elastic Properties of Polychloroprene Rubbers in Tension and Compression during Ageing. Polymers 2020, 12, 2354. [Google Scholar] [CrossRef] [PubMed]
- Liu, Q.; Shi, W.; Chen, Z.; Li, K.; Liu, H.; Li, S. Rubber accelerated ageing life prediction by Peck model considering initial hardness influence. Polym. Test. 2019, 80, 106132. [Google Scholar] [CrossRef]
- Daley, J.R. Assessment of Life Prediction Methods for Elastomeric Seals-A Review. In Elastomers and Components: Service Life Prediction-Progress and Challenges; Coveney, V.A., Ed.; Woodhead Publishing Ltd.: Cambridge, UK, 2006. [Google Scholar]
- Maanaoui, H.E.; Meier, J. Lifetime prediction with temperature dependence for EPDM and NR elastomers based on fatigue crack growth mechanical measurements and filler distribution. Polymer 2021, 228, 123909. [Google Scholar] [CrossRef]
- Lee, P.-C.; Kim, S.Y.; Jeoung, S.K.; Ko, Y.K.; Ha, J.U.; Lee, J.-Y.; Kim, M. Life Time Prediction of elastomer for Automotive Vapor Fuel Hose. Polym. Korea 2022, 46, 397–401. [Google Scholar]
- Ford, A.R.; Burns, W.A.; Reeve, S.W. Rotational Analysis of FTIR Spectra from Cigarette Smoke. J. Chem. Educ. 2004, 81, 865. [Google Scholar] [CrossRef]
- Susanta, M.; Afshin, G.-S.; Peter, K.; Søren, H.; Kristoffer, A. Chemical Degradation of an Uncrosslinked Pure Fluororubber in an Alkaline Environment. J. Polym. Sci. Part A Polym. Chem. 2004, 42, 6216–6229. [Google Scholar]
- Sugama, T.; Pyatina, T.; Redline, E.M.; McElhanon, J.R.; Blankenship, D.A. Evaluation of the Performance of Orings Made with Different Elastomeric Polymers in Simulated Geothermal Environments at 300 °C; Brookhaven National Lab. (BNL): New York, NY, USA, 2014. [Google Scholar] [CrossRef]
- Jagdish, P.; Rajesh, N.P.; Natarajan, S. Growth and Characterization of Urea Adduct with m-Nitrobenzoic Acid, m-Nitroaniline, and p-Xylene Mixtures. J. Miner. Mater. Charact. Eng. 2010, 9, 471–481. [Google Scholar] [CrossRef]
- Nguyen-Tri, P.; Triki, E.; Nguyen, T.A. Butyl Rubber-Based Composite: Thermal Degradation and Prediction of Service Lifetime. J. Compos. Sci. 2019, 3, 48. [Google Scholar] [CrossRef] [Green Version]
- Lellinger, D.; Alig, I.; Oehler, H.; Rode, K.; Malz, F.; Herkenrath, L.M.; Youn, J.Y. Accelerated thermal aging of thermoplastic materials for the motor compartment: Characterization, degradation model and lifetime prediction. In Service Life Prediction of Polymers and Coatings; White, C.C., Nichols, M.E., Pickett, J.E., Eds.; William Andrew: Cambridge, MA, USA, 2020; pp. 117–160. [Google Scholar]






| Physical Parameter | Bx | Service Time (×103·h) | |
|---|---|---|---|
| Tube 1 | Tube 2 | ||
| Elongation | B0.1 | 7.60 | 35.0 |
| B1 | 11.6 | 63.7 | |
| B10 | 17.8 | 117 | |
| CS | B0.1 | 24.8 | 6.47 |
| B1 | 58.4 | 12.7 | |
| B10 | 140 | 25.1 | |
| Hardness | B0.1 | 23.7 | 40.7 |
| B1 | 53.9 | 73.5 | |
| B10 | 124 | 134 | |
| Method | Physical Parameter | Ea (kJ mol−1) | |
|---|---|---|---|
| Tube 1 | Tube 2 | ||
| Thermal | Elongation | 74.91 ± 4.331 | 91.69 ± 8.942 |
| CS | 159.6 ± 28.84 | 120.3 ± 21.86 | |
| Hardness | 93.93 ± 8.879 | 86.70 ± 6.436 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, P.-C.; Kim, S.Y.; Ko, Y.K.; Ha, J.U.; Jeoung, S.K.; Lee, J.-Y.; Kim, M. Durability and Service Life Prediction of Fluorocarbon Elastomer under Thermal Environments. Polymers 2022, 14, 2047. https://doi.org/10.3390/polym14102047
Lee P-C, Kim SY, Ko YK, Ha JU, Jeoung SK, Lee J-Y, Kim M. Durability and Service Life Prediction of Fluorocarbon Elastomer under Thermal Environments. Polymers. 2022; 14(10):2047. https://doi.org/10.3390/polym14102047
Chicago/Turabian StyleLee, Pyoung-Chan, Su Young Kim, Youn Ki Ko, Jin Uk Ha, Sun Kyoung Jeoung, Ju-Yub Lee, and Minsu Kim. 2022. "Durability and Service Life Prediction of Fluorocarbon Elastomer under Thermal Environments" Polymers 14, no. 10: 2047. https://doi.org/10.3390/polym14102047
APA StyleLee, P.-C., Kim, S. Y., Ko, Y. K., Ha, J. U., Jeoung, S. K., Lee, J.-Y., & Kim, M. (2022). Durability and Service Life Prediction of Fluorocarbon Elastomer under Thermal Environments. Polymers, 14(10), 2047. https://doi.org/10.3390/polym14102047