Steady-State Creep of Asphalt Concrete
Abstract
:1. Introduction
2. Materials and Methods
2.1. Bitumen
2.2. Asphalt Concrete
2.3. Sample Preparation
2.4. Test
3. Results and Discussion
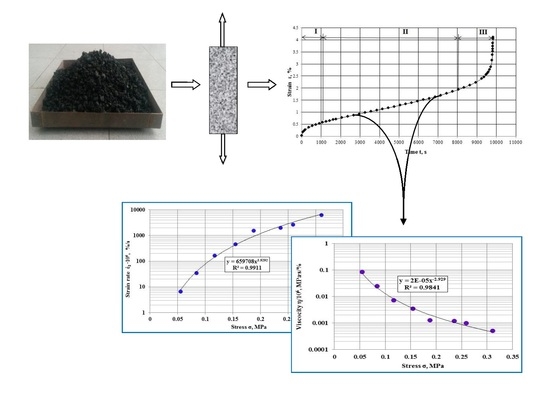
3.1. Creep Curve
3.2. Steady-State Creep
3.3. Defining Relations for Steady-State Creep of Asphalt Concrete at Complex Stressed Condition
4. Conclusions
- -
- For creep curve stage II, the asphalt concrete deformation occurred at a constant rate. The strain rate for this stage is dependent on the stress, and this dependence is satisfactorily described by a power function. The stress has a great influence on the strain rate where the increase of stress by one order increases the strain rate approximately by four orders;
- -
- The dependences were constructed for the start point, end point, and the duration of the stage of steady-state creep on the stress. The stress also impacts greatly on the specified time characteristics, where the increase of stress by one order increases these characteristics for 4.3–4.5 orders;
- -
- The values of viscosity for asphalt concrete were determined at various stresses. The dependence was defined for viscosity on the stress and can also be satisfactorily described by a power function. In particular, the increase of stress by one order reduces the viscosity by three orders;
- -
- Assuming that asphalt concrete is an incompressible material, then the stress deviator is proportional to the strain rate deviator. Hence, there is a functional relationship between the stress intensity and the strain rate intensity, which does not depend on the type of stress condition. The defining relations were formulated for the steady-state creep of asphalt concrete under complex stressed conditions.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- The Asphalt Handbook (MS-4), 7th ed.; Asphalt Institute: Lexington, MA, USA, 2008.
- Papagiannakis, A.T.; Masad, E.A. Pavement Design and Materials; John Wiley & Sons, Inc.: New Jersey, NJ, USA, 2008. [Google Scholar]
- Yoder, E.J.; Witczak, M.W. Principles of Pavement Design; John Wiley & Sons, Inc.: New Jersey, NJ, USA, 1975. [Google Scholar]
- Cristensen, R.M. Theory of Viscoelasticity: An Introduction; Academic Press: New York, NY, USA, 1971. [Google Scholar]
- Ferry, J.D. Viscoelastic Properties of Polymers; John Wiley & Sons, Inc.: New York, NY, USA, 1980. [Google Scholar]
- Tschoegl, N.W. The Phenomenological Theory of Linear Viscoelastic Behavior. An Introduction; Springer: Berlin, Germany, 1989. [Google Scholar]
- Hopkins, I.L.; Hamming, R.W. On creep and relaxation. J. Appl. Phys. 1957, 28, 906–909. [Google Scholar] [CrossRef]
- Taherkhani, H. Compressive creep behavior of asphalt mixtures. Eng. Procedia 2011, 10, 583–588. [Google Scholar] [CrossRef]
- Hassan, M.M. Relationship between creep time dependent index and Paris low parameters for bituminous mixtures. J. S. Afr. Inst. Civ. Eng. 2013, 2, 8–11. [Google Scholar]
- Soleimanbeigi, A.; Edil, T.B.; Benson, C.H. Creep response of recycled asphalt shingles. Can. Geotech. J. 2013, 51, 103–114. [Google Scholar] [CrossRef]
- Mahan, H.M. Behavior of permanent deformation in asphalt concrete pavements under temperature variation. Al-Qadisiya J. Eng. Sci. 2013, 1, 62–73. [Google Scholar]
- Zhou, Z.G.; Feng, L.; Yuan, X.X.; Xiong, H. Study of the creep damage properties of asphalt mixture under static load. In Proceedings of the 13th International Conference on Fracture, Beijing, China, 16–21 June 2013.
- Jaczewski, M.; Judycki, J. Effects of deviations from thermo-rheologically simple behavior of asphalt mixtures in creep on developing of master curves of their stiffness modulus. In Proceedings of the 9th International Conference on Environmental Engineering, Vilnius, Lithuania, 22–23 May 2014.
- Iskakbayev, A.; Teltayev, B.; Alexandrov, S. Determination of the creep parameters of linear viscoelastic materials. J. Appl. Math. 2016, 2016, 6568347. [Google Scholar] [CrossRef]
- Kachanov, L. Introduction to Continuum Damage Mechanics; Martinus Nijhoff Publishers: Dordrecht, The Netherlands, 1986. [Google Scholar]
- Rabotnov, Y.N. Introduction to Fracture Mechanics; Nauka: Moscow, Russia, 1987. [Google Scholar]
- Bitumens and Bitumen Binders. Oil Road Viscous Bitumens (ST RK 1373); Technical Specifications: Astana, Kazakhstan, 2013.
- Performance Graded Asphalt Binder Specification and Testing (Superpave Series No. 1); Asphalt Institute: Lexington, MA, USA, 2003.
- Hot Mix Asphalt for Roads and Airfields (ST RK 1225); Technical Specifications: Astana, Kazakhstan, 2013.
- Crushed Stone and Gravel of Dense Rock for Construction Works (SТ RК 1284); Technical Specifications: Astana, Kazakhstan, 2004.
- Bituminous Mixtures. Test Methods for Hot Mix Asphalt. Part 33: Specimen Prepared by Roller Compactor (EN 12697-33); European Committee for Standardization: Brussels, Belgium, 2003.
- Iskakbayev, A.; Teltayev, B.; Andriadi, F.; Estayev, K.; Suppes, E.; Iskakbayeva, A. Experimental research of creep, recovery and fracture processes of asphalt concrete under tension. In Proceedings of the 24th International Congress on Theoretical and Applied Mechanics, Montreal, QC, Canada, 21–26 August 2016.
- Teltayev, B.B.; Iskakbayev, A.I.; Rossi, C.O. Regularities of Creep and Long-Term Strength of Hot Asphalt Concrete under Tensile. In Proceedings of the 4th Chinese-European Workshop “Functional Pavement Design”, Delft, The Netherlands, 29 June–1 July 2016.
- Nadai, A. Theory of Flow and Fracture of Solids; MeGraw-Hill: New York, NY, USA, 1963. [Google Scholar]
- Huang, Y.H. Pavement Analysis and Design, 2nd ed.; Pearson Education, Inc.: Upper Saddle River, NJ, USA, 2004. [Google Scholar]
- Talreja, R.; Sing, C.V. Damage and Failure of Composite Materials; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]















| Indicator | Measurement Unit | Requirements of ST RK 1373 | Value |
|---|---|---|---|
| Penetration, 25 °С, 100 gr, 5 s | 0.1 mm | 101–130 | 104 |
| Penetration Index PI | - | −1.0, … ,+1.0 | −0.34 |
| Tensility at temperature: | cm | - | - |
| 25 °С | - | ≥90 | 140 |
| 0 °С | - | ≥4.0 | 5.7 |
| Softening point | °С | ≥43 | 46.0 |
| Fraas point | °С | ≤−22 | −25.9 |
| Dynamic viscosity, 60 °С | Pa·s | ≥120 | 175.0 |
| Kinematic viscosity | mm2/s | ≥180 | 398.0 |
| Indicator | Measurement Unit | Requirements of ST RK 1284 [20] | Value | |
|---|---|---|---|---|
| Fraction 5–10 mm | Fraction 10–20 mm | |||
| Average density | g/cm3 | - | 2.55 | 2.62 |
| Elongated particle content | % | ≤25 | 13 | 9 |
| Clay particle content | % | ≤1.0 | 0.3 | 0.2 |
| Bitumen adhesion | - | - | satisf. | satisf. |
| Water absorption | % | - | 1.93 | 0.90 |
| Indicator | Measurement Unit | Requirements of ST RK 1225 | Value |
|---|---|---|---|
| Average density | g/cm3 | - | 2.39 |
| Water saturation | % | 1.5–4.0 | 2.3 |
| Voids in mineral aggregate | % | ≤19 | 14 |
| Air void content in asphalt concrete | % | 2.5–5.0 | 3.8 |
| Compression strength at temperature | MPa | - | - |
| 0 °С | - | ≤13.0 | 7.0 |
| 20 °С | - | - | 3.4 |
| 50 °С | - | ≥1.3 | 1.4 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Iskakbayev, A.; Teltayev, B.; Oliviero Rossi, C. Steady-State Creep of Asphalt Concrete. Appl. Sci. 2017, 7, 142. https://doi.org/10.3390/app7020142
Iskakbayev A, Teltayev B, Oliviero Rossi C. Steady-State Creep of Asphalt Concrete. Applied Sciences. 2017; 7(2):142. https://doi.org/10.3390/app7020142
Chicago/Turabian StyleIskakbayev, Alibai, Bagdat Teltayev, and Cesare Oliviero Rossi. 2017. "Steady-State Creep of Asphalt Concrete" Applied Sciences 7, no. 2: 142. https://doi.org/10.3390/app7020142
APA StyleIskakbayev, A., Teltayev, B., & Oliviero Rossi, C. (2017). Steady-State Creep of Asphalt Concrete. Applied Sciences, 7(2), 142. https://doi.org/10.3390/app7020142