3.1. Composition and Crystal Structure
The atomic content data of the (Ti, Zr)N
ternary films are listed in
Table 1. Only Ti, Zr, and N are included in the measurement, for SiO
substrates were used and the measurement of oxygen is difficult. The N content was above 50% for all the (Ti, Zr)N
films. The nitrogen-overstoichiometry of the films reflects that it is not difficult for ionized nitrogen atoms to combine with metal atoms in a vacuum. It is in the expectation that the N-content of the films increases with
increasing, which can be explained by the reaction of the nitrogen atom and metal atoms. In the experiments, metal targets were used, and the nitrogen atoms reacting with metal atoms were from the nitrogen flow. Higher
brought more nitrogen atoms to react with deposited metal atoms and increased the nitrogen content of the films.
Table 1 shows that the Ti and Zr content are also influenced by
. The Ti content is lower than the Zr content in the films deposited with lower (10% and 15%)
s, but it is just on the contrary for the films of higher (20%, 30% and 50%)
s. This result is different from that of the co-sputtered (Ti, Zr)N
films with nitride targets in our previous work [
21], in which Ti atoms prefer lower
s and Zr atoms prefer higher ones. The content of the films is affected mainly by two processes, namely, sputter and absorption. Different
means a different proportion of nitrogen and argon ions sputtering targets. Argon ions usually sputter metal targets with a higher rate than nitrogen ions, especially for the zirconium target, because zirconium has a higher atomic mass than titanium. So the influence of
on the sputtering rate of the zirconium target is great than that on titanium target. As
increasing, the sputtering rate of both the Ti and Zr targets decreases. However, the decrease of the sputtering rate of the Ti target is not as great as that of the Zr target. This is one possible reason why Ti content decrease more slowly than Zr content with
increasing as shown in
Table 1. The absorption and growth process of nitride films in the experiments include the reaction between metal and nitrogen. It is easier for zirconium atoms to react with nitrogen atoms, because their higher electronegativity makes them easier to lose electrons. So the Zr content in the films of lower
is higher. It is possible that the reaction speed of zirconium with nitrogen tends to saturation when
is above 15%. The reaction of the titanium gets more benefit from the increase of
, and the reaction speed is accelerated significantly, which also leads to the higher Ti content than Zr content in the films of higher
.
The variation of the content of the film is also reflected by its crystal structure.
Figure 1a shows the evolution of XRD
–2
patterns of the films deposited with different
. The analysis of the XRD data was based on JCPDS cards (No. 65-0965 and No. 65-0972). It can be seen for all the films, there is a strong (111) diffraction peak located between the fcc-TiN(111) peak and the fcc-ZrN(111) one. There is also a weak (200) characteristic peak only in the pattern of the films deposited with
of 5%, which is also between the fcc-TiN(200) peak and the fcc-ZrN(200) one. No other characteristic diffraction peak exists in the patterns. It can be concluded that the films are B1-structured and the preferred orientation is (111), which has growth advantages [
10]. The results show that all the (Ti, Zr)N
films exist as a kind of solid solution, in which the Ti and Zr atoms are distributed in a rocksalt crystal structure with a certain degree of uniformity. Because the Zr and Ti are of the same group and ZrN and TiN are of the same crystal structure, according to the Hume-Rothery rule [
24], it is easy to form a solid solution for ZrN and TiN.
As the nitrogen flow ratio
gradually increases, the (111) diffraction peak moves toward the fcc-TiN (111) peak. The shift of diffraction peak reflects the change of lattice constants.
Figure 1b shows the lattice constants
a of the films calculated by the Bragg equation. It can be easily seen that the lattice constants are in the range of 4.38–4.42 Å and monotonically decrease with
increasing. Because Ti–N bond is shorter than Zr–N bond, with
increasing, the increased Ti percent ratio (Ti/Zr), as shown in
Table 1, will decrease the lattice constant. Furthermore, the increase in nitrogen content also reduces the lattice constant, though the films are nitrogen-overstoichiometric. As Balasubramanian reported [
25], in nitrogen-overstoichiometric B1-structures nitrides, the main defects are cation vacancies, which exhibit negative formation energies, and are thermodynamically favored. While interstitials are difficult to form [
25] for their higher positive forming energy. So as
increases, more and more cation vacancies form, which is another main factor leading to a smaller lattice constant under higher
.
To further investigate the effects of
on the microstructure of the film, we studied the crystallite size
D calculated by Scherrer equation,
where
is the full width at half maximum (FWHM) of the (111) peaks value, and
is the diffraction peak position. The data of the crystallite size are shown in
Figure 1c, in which crystallite size increases with
increasing. This result is different from that of the ternary nitride films co-sputtered with nitride targets [
21], in which crystallite size decreases with nitrogen content increasing. For the growth process of films sputtered from metal targets, the chemical reaction is necessary to form nitride film, and nitrogen-rich atmosphere is favored by the nucleation and nuclei merging process of nitride, because nitrogen-rich atmosphere provide much more chance for metal atoms to combine with nitrogen atoms. So higher
leads to larger crystallite size in this work. However, for the growth process from nitride targets, many nitride particles with raw chemical bonds deposited on substrates, and too much nitrogen possibly leads to more defects in the films. Therefore higher
leads to small crystallite size in our previous work, in which nitride targets were sputtered.
The chemical states of the (Ti, Zr)N
films were obtained using X-ray photoelectron spectroscopy. All samples were surface-cleaned with argon ion etching of 2000 eV for 56 s before testing.
Figure 2a–c shows the XPS spectra of the Ti 2
p, Zr 3
d and N 1
s core energy levels of (Ti, Zr)N
films, respectively, with the measurement uncertainty below 0.1 eV. The dashed lines are multimodal spectra fitted with Gaussian multimodality, which are used to distinguish different contributions of different bonds. As shown by the dotted line in
Figure 2a,b, the characteristic Ti 2
and Zr 3
d peaks result mainly from the interaction of three kinds of bonds. Besides the expected signals of metal (Ti and Zr) and nitride (TiN
and ZrN
), there are characteristic peaks of metal oxides. The appearance of the Ti–O, Zr–O, O–Zr–N bonds indicates that oxygen atoms enter some vacancies in the B1-structured nitride lattice, for only the diffraction peaks of rocksalt nitride exist in the XRD results. We think these bonds are related to the residual oxygen or H
O in the vacuum chamber [
26]. The peaks near the binding energy of 461 eV in
Figure 2a and 183 eV in
Figure 2b are almost all contributed by dioxide TiO
and ZrO
, respectively. It is possible that the titanium dioxide bonds results mainly from the post-deposition oxidization of the films, for there is no diffraction peaks of titanium dioxide in the XRD results after all. Although the plasma etching was performed before the test, it was difficult to eliminate the intended effect of oxygen.
The solid line in
Figure 2c shows the N
core energy level spectra. Besides a small contribution of the N
O
, the peaks are mainly composed the peaks of TiN
and ZrN
. Quantitative analysis of integral proportion of the peaks indicates that the integral proportion TiN
peak gets to its maximum at
of 20%, while that of ZrN
peak gets to its minimum at the same
. The variation of the integral proportion reflects the nitrification extend of the metal atoms, which influences the electronic and optical properties of the nitride films.
3.2. Dielectric Function
Dielectric properties are fundamental for the study and applications of plasmonic materials. The dielectric functions of the films in this work were measured by incident elliptically polarized light at an angle of 70
to an ellipsometry spectrometer. The complex dielectric function
=
+
is determined by the elliptic angles
and
, and then fitted to the Drude–Lorentz dispersion model with two Lorentz oscillators. Due to the presence of both intraband and interband transitions in the film, the electronic response in the nitride is fitted using the following equation [
27]:
where
,
, and
represent the background dielectric function, the plasma frequency, and the Drude collision frequency (relaxation rate).
,
,
and
,
,
represent the energy position, intensity and broadening parameters of the first and second Lorentz oscillators, respectively. Series of fitting parameters of the film are shown in
Table 2.
Figure 3a,b depict the real part
and imaginary part
of the permittivities of the (Ti, Zr)N
films, respectively. As shown in
Figure 3a, as
increases, the
curve redshifts. For all the films, as the incidence wavelength increases,
decreases from positive to negative values. The positive and negative values of
are usually associated with interband and intraband transition respectively. A major practical importance is the crossover frequency
, which is defined as the frequency at which
= 0. The screened plasma frequency
is associated with the plasmonic resonances frequency
, which is proportional to the square root of the carrier concentration [
11]. So the variation of
directly reflects the behavior of carrier concentration. The free electron density
n can be obtain by Equation (
3)
For the metallic nitride, the effective mass
of electrons can be approximatively replaced by the mass
m. The calculated free electron density values were also listed in
Table 2.
As shown in
Table 2, both the plasma frequency
and the electron density
n of the (Ti, Zr)N
films decreases with
increasing. The screened plasma frequency
, indicated in
Figure 3a, is sharply reduced from 2.72 eV to 1.27 eV when
increases from 10% to 30%. For the films of
= 50%,
is positive in the whole range of the measurement. For ternary (Ti, Zr)N
film, many factors, such as the inhomogeneity of atomic distribution, point defects of alloying, grain boundaries, possibly trap electrons and reduces the plasma resonance frequency. In this work, it is mainly the increase of N content and the consequent reduction of metal content, as shown in
Table 1, that reduce free electron concentration, because the free electrons are gradually absorbed by increasing nitrogen atoms.
The imaginary part
of the complex permittivity of the (Ti, Zr)N
films is given in
Figure 3b. In visible range,
increases with wavelength increasing.
is recognized to be associated with dielectric loss, and the results indicate that in NIR range (Ti, Zr)N
films of lower
is more lossy than those of higher
. There are one or two peaks in the
spectra in the near-ultraviolet region as indicated in the inset, which is associated with interband transitions [
28]. As
increases, the peak moves towards lower energies. This shift reflects that increased nitrogen content causes the reduction of energy levels at which the interband transition is excited. The other peaks between 5 and 6 eV in the
curves of the films deposited with
of 30% and 50% possibly resulting from the deeper interband transition, which was elevated by increased nitrogen content.
Another parameter for evaluating the plasmonic characteristics of the films is energy loss function, which, are evaluated using equation [
29]:
where
and
values are the data in
Figure 3.
Figure 4 exhibits the energy loss function spectrum of (Ti, Zr)N
films deposited with different
. These spectra indicate the strong plasmon resonance in the films and the effects of
are significant. With
increasing, the energy loss peak, shown in the inset, exhibits a red shift. The results also reflect that as
increases, the metallicity of the film decreases. This experimental law of the energy peak is consistent with that of
in
Figure 3a and the peak of
in the inset of
Figure 3b.
3.3. Electronic Structure
The optical and dielectric properties of the films are determined by their electronic structure. For B1-structured metal nitrides, under standard conditions, the difference in the electronegativity between N and metal atoms will cause the metal compound with partial ionicity in the bond. The commingling of metallicity and ionicity is the reason for most of the special characteristics exhibited by metal nitrides. In a close-packed rocksalt structure of nitride, nitrogen atoms occupy octahedral interstitial sites in the (fcc) metal sublattice. In order to obtain the electronic characteristics of Ti–Zr ternary nitride, based on the CASTEP code of the Materials Studio software package, we performed a generalized gradient approximation using the Perdew Burke Ernzerhof function [
30,
31]. The calculation was based on a primitive cell of B1-structured Ti
Zr
N, the lattice constant of which is set as 4.40. The cut-off energy of the plane-wave basis set is ultra-fine. The
-point set was 10 × 10 × 10, and the band energy tolerance and SCF tolerance were 1.0 × 10
eV and 5.0 × 10
eV/atom, respectively.
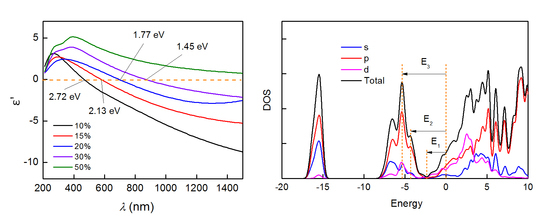
The calculated electronic band structure and the density of states are shown in
Figure 5, in which the Fermi level was set at zero energy. In
Figure 5, we identify three separate bundles of energy bands. As indicated in the total and projected density of states (DOS) shown in
Figure 5b, each band is composed of a predominant characteristic deriving from a single ionic contribution plus a minor covalent contribution [
32]. At higher binding energies around −16 eV, there is a single band with a primary N
characteristic. The energy range from −9 to −3 eV is characterized by a bundle of three dispersive bands, which mostly derive from N
states partially hybridized with Ti and Zr orbitals. Finally, a bundle of five overlapping bands from −3 to 6 eV around the Fermi level has a main metal characteristic with a weak contribution from N
states.
Metallic nitride is not a typical metal, and the presence of interband transition induces small positive
values above the crossover frequency
. The plasmon resonance excitation in the visible range is important for optoelectronic applications, and the origin of this resonance is the interaction between interband and intraband transitions. This can be explained by the electronic structure in
Figure 5, in which the band around the Fermi surface is responsible for the metallicity and the negative
values in the long-wavelength range. For energies lower than 3.0 eV, the only possible excitations of the valence electrons are due to intraband transitions in Zr
or Ti
bands crossing the Fermi level [
32]. In this range, the partial coupling with N
states gives a very low contribution to optical absorption, which leads to small
. As the excitation energy is increased, interband transitions from N
to Zr
and Ti
take place. This creates a dielectric screening, which results in zero crossover of
from negative to positive at
, at which
= 0 and
have a minimum.
is interpreted as a screened plasmon, which involves the collective oscillation of a reduced charge density, because only a fraction of the total valence electrons can be considered as free and the rest are effectively screened [
32].
The interband absorption originates from the bands of 3–8 eV below Fermi level. According to the selection rules for photonic excitation, the N
electrons in the bands transmit to the Fermi level and exhibit interband transition. As marked in
Figure 5, there are 3 characteristic energy levels in the DOS function.
, around 3 eV below
, is the energy level at which Zr
or Ti
DOS getting to its minimum, and N
states begin to dominate the total DOS function. In addition,
is the cut-off energy level of the N
DOS [
28], at which the N
bands begin to degenerate at
point. We found that
is approximatively equal to the
value of the films deposited with 10% N
, in
Figure 3b. So,
can be regarded as the threshold of the dielectric contribution to the optical response. The energy level of 4.2 and 5.3 eV below
, marked by
and
, correspond to a local maximum and the global maximum of the DOS of N
bands, respectively. It can also be found that
and
are approximatively equal to the positive maximum of
and
in the near-ultraviolet region in
Figure 3. The difference between the peculiar energy levels (
,
and
) in electronic structure and the characteristic frequencies in dielectric function possibly results from the non-stoichiometic defects. Increased nitrogen content enhance the DOS of N
band and reduce the DOS of metal, and consequently make the characteristic energy level (
,
and
in
Figure 5) shift towards fermi level. This shift reduced the frequency of interband transition, at which
crosses zero. The results of the calculation are consistent with the behavior of dielectric function tuned by
shown in
Figure 3.
3.4. Plasmonic Quality Factors
The plasmonic performance of a plasmonic materials may be evaluated by factors of merit for localized surface plasmon resonance (LSPR) and for surface plasmon polariton (SPP). The quality factor for LSPR (
) of nitride particles can be defined as [
33,
34]:
where
and
represent the real and imaginary part of
in the results above.
The calculated
values of all the films are shown in
Figure 6a. In the figure, All the films in this study have a relatively high
, and the strength and location of
peaks of the films are different. However, its dependence on
is not significant, and not monotonous either. This result is different from the
behavior of films sputtered with nitride targets, in which increased
can reduce the value of
significantly. The difference possibly results from different portions of the chemical bond in the films. The non-trend resonance peaks in the
-
plot indicate the optimal
region of the corresponding samples for the application in plasmonic devices.
To describe the potential of these nitride films for plasmonic application, we consider the interface of nitride films and air, which sustains SPP formation and propagation. The factor of merit
of the nitride layers interfaced with air for SPP mode can be given as [
33,
34]:
The
spectra of intraband region (
< 0) of the films are plotted in
Figure 6b. We can see that
decreases as
increases for the reduced metallicity of the films. The
of the films deposited with low
can be up to 2.5, which leads to a potential application for Ti–Zr ternary nitride as alternative plasmonic materials.
The effects of on the plasmonic quality are mainly due to the modification of free carrier concentration by nitrogen content. More and more defects caused by increased is another important factor. Nevertheless, the results indicate the feasibility of tuning the plasmonic factors by nitrogen flow ratio.
The plasmonic factors of materials can be affected by many experimental parameters. This work and our previous works [
20,
21] show that the
and
of the Ti–Zr ternary films are affected by the composition, the substrate bias and temperature during the deposition in magnetron co-sputtering. In these work the peak value of
is in the same order of magnitude. In addition, the composition has a greater influence on the shift of the
resonance peak. Other parameters have limited influence on the
resonance peak position. The peak position of
is not only related to the composition of the film, but also easily affected by deposition temperature. These works provide a comprehensive reference to select plasmonic materials in specific application scenarios.