Theoretical Analysis of Terahertz Dielectric–Loaded Graphene Waveguide
Abstract
:1. Introduction
2. Theory
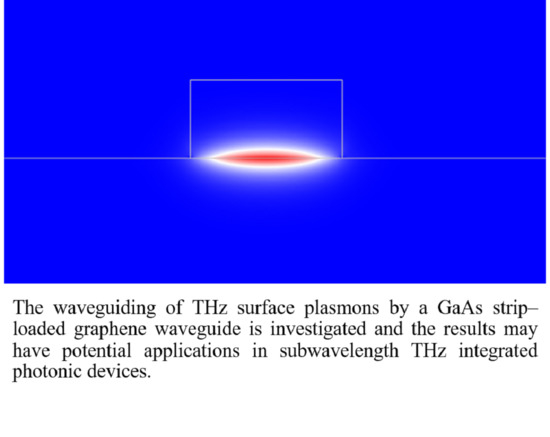
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ahmadivand, A.; Gerislioglu, B.; Ahuja, R.; Mishra, Y.K. Terahertz plasmonics: The rise of toroidal metadevices towards immunobiosensings. Mater. Today 2020, 32, 108–130. [Google Scholar] [CrossRef]
- Chan, W.L.; Deibel, J.; Mittleman, D.M. Imaging with terahertz radiation. Rep. Prog. Phys. 2007, 70, 1325–1379. [Google Scholar] [CrossRef]
- Ma, J.; Shrestha, R.; Adelberg, J.; Yeh, C.Y.; Hossain, Z.; Knightly, E.; Jornet, J.M.; Mittleman, D.M. Security and eavesdropping in terahertz wireless links. Nature 2018, 563, 89–93. [Google Scholar] [CrossRef] [PubMed]
- Koenig, S.; Lopez-Diaz, D.; Antes, J.; Boes, F.; Henneberger, R.; Leuther, A.; Tessmann, A.; Schmogrow, R.; Hillerkuss, D.; Palmer, R.; et al. Wireless sub-THz communication system with high data rate. Nat. Photonics 2013, 7, 977–981. [Google Scholar] [CrossRef]
- Salamin, Y.; Benea-Chelmus, I.C.; Fedoryshyn, Y.; Heni, W.; Elder, D.L.; Dalton, L.R.; Faist, J.; Leuthold, J. Compact and ultra-efficient broadband plasmonic terahertz field detector. Nat. Commun. 2019, 10, 5550. [Google Scholar] [CrossRef]
- Yang, Y.; Yamagami, Y.; Yu, X.; Pitchappa, P.; Webber, J.; Zhang, B.; Fujita, M.; Nagatsuma, T.; Singh, R. Terahertz topological photonics for on-chip communication. Nat. Photonics 2020, 14, 446–451. [Google Scholar] [CrossRef] [Green Version]
- Gramotnev, D.K.; Bozhevolnyi, S.I. Plasmonics beyond the diffraction limit. Nat. Photonics 2010, 4, 83–91. [Google Scholar] [CrossRef]
- Atakaramians, S.; Afshar, S.; Monro, T.M.; Abbott, D. Terahertz dielectric waveguides. Adv. Opt. Photonics 2013, 5, 169–215. [Google Scholar] [CrossRef]
- Ung, B.; Mazhorova, A.; Dupuis, A.; Rozé, M.; Skorobogatiy, M. Polymer microstructured optical fibers for terahertz wave guiding. Opt. Express 2011, 19, B848–B861. [Google Scholar] [CrossRef] [Green Version]
- Anthony, J.; Leonhardt, R.; Argyros, A. Hybrid hollow core fibers with embedded wires as THz waveguides. Opt. Express 2013, 21, 2903–2912. [Google Scholar] [CrossRef] [PubMed]
- Hassani, A.; Dupuis, A.; Skorobogatiy, M. Porous polymer fibers for low-loss Terahertz guiding. Opt. Express 2008, 16, 6340–6351. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, D.; Chen, L.; Wu, X.; Liu, F. Terahertz composite plasmonic slabs based on double-layer metallic gratings. Opt. Express 2020, 28, 18212–18223. [Google Scholar] [CrossRef]
- Wang, K.; Mittleman, D.M. Metal wires for terahertz wave guiding. Nature 2004, 432, 376–379. [Google Scholar] [CrossRef] [PubMed]
- Cao, Q.; Jahns, J. Azimuthally polarized surface plasmons as effective terahertz waveguides. Opt. Express 2005, 13, 511–518. [Google Scholar] [CrossRef] [PubMed]
- Gordon, R. Reflection of cylindrical surface waves. Opt. Express 2009, 17, 18621–18629. [Google Scholar] [CrossRef] [PubMed]
- Chen, S.H.; Chen, K.W.; Chu, K.R. A comparative study of single-wire and hollow metallic waveguides for terahertz waves. Aip Adv. 2018, 8, 115028. [Google Scholar] [CrossRef] [Green Version]
- Kang, J.H.; Kim, D.S.; Seo, M. Terahertz wave interaction with metallic nanostructures. Nanophotonics 2018, 7, 763–793. [Google Scholar] [CrossRef]
- He, X.Y.; Cao, J.C.; Feng, S.L. Simulation of the propagation property of metal wires terahertz waveguides. Chin. Phys. Lett. 2006, 23, 2066–2069. [Google Scholar]
- Liang, H.; Ruan, S.; Zhang, M. Terahertz surface wave propagation and focusing on conical metal wires. Opt. Express 2008, 16, 18241–18248. [Google Scholar] [CrossRef]
- Zheng, Z.; Kanda, N.; Konishi, K.; Kuwata-Gonokami, M. Efficient coupling of propagating broadband terahertz radial beams to metal wires. Opt. Express 2013, 21, 10642–10650. [Google Scholar] [CrossRef]
- Mbonye, M.; Mendis, R.; Mittleman, D.M. A terahertz two-wire waveguide with low bending loss. Appl. Phys. Lett. 2009, 95, 233506. [Google Scholar] [CrossRef] [Green Version]
- Pahlevaninezhad, H.; Darcie, T.E.; Heshmat, B. Two-wire waveguide for terahertz. Opt. Express 2010, 18, 7415–7420. [Google Scholar] [CrossRef] [PubMed]
- Teng, D.; Cao, Q.; Li, S.; Gao, H. Tapered dual elliptical plasmon waveguides as highly efficient terahertz connectors between approximate plate waveguides and two-wire waveguides. J. Opt. Soc. Am. A 2014, 31, 268–273. [Google Scholar] [CrossRef] [PubMed]
- Liang, H.; Ruan, S.; Zhang, M.; Su, H. Nanofocusing of terahertz wave on conical metal wire waveguides. Opt. Commun. 2010, 283, 262–264. [Google Scholar] [CrossRef]
- He, X.Y. Investigation of terahertz surface waves of a metallic nanowire. J. Opt. Soc. Am. B 2010, 27, 2298–2303. [Google Scholar] [CrossRef]
- Oulton, R.F.; Sorger, V.J.; Genov, D.A.; Pile, D.F.P.; Zhang, X. A hybrid plasmonic waveguide for subwavelength confinement and long-range propagation. Nat. Photonics 2008, 2, 496–500. [Google Scholar] [CrossRef] [Green Version]
- Eldlio, M.; Ma, Y.Q.; Maeda, H.; Cada, M. A long-range hybrid THz plasmonic waveguide with low attenuation loss. Infrared Phys. Technol. 2017, 80, 93–99. [Google Scholar] [CrossRef]
- Teng, D.; Cao, Q.; Wang, K. An extension of the generalized nonlocal theory for the mode analysis of plasmonic waveguides at telecommunication frequency. J. Opt. 2017, 19, 055003. [Google Scholar] [CrossRef] [Green Version]
- Chu, S.; Wang, Q.; Yu, L.; Gao, H.; Liang, Y.; Peng, W. Numerical investigation on multiple resonant modes of double-layer plasmonic grooves for sensing application. Nanomaterials 2020, 10, 308. [Google Scholar] [CrossRef] [Green Version]
- Huang, T.J.; Yin, L.Z.; Zhao, J.; Du, C.H.; Liu, P.K. Amplifying evanescent waves by dispersion-induced plasmons: Defying the materials limitation of superlens. ACS Photonics 2020, 7, 2173–2181. [Google Scholar] [CrossRef]
- Jablan, M.; Buljan, H.; Soljačić, M. Plasmonics in graphene at infrared frequencies. Phys. Rev. B 2009, 80, 245435. [Google Scholar] [CrossRef] [Green Version]
- Vakil, A.; Engheta, N. Transformation optics using graphene. Science 2011, 332, 1291–1294. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Teng, D.; Wang, K.; Huan, Q.S.; Chen, W.G.; Zhe, L. High-performance light transmission based on graphene plasmonic waveguides. J. Mater. Chem. C 2020, 8, 6832–6838. [Google Scholar] [CrossRef]
- Bao, Z.; Tang, Y.; Hu, Z.D.; Zhang, C.; Balmakou, A.; Khakhomov, S.; Semchenko, I.; Wang, J. Inversion method characterization of graphene-based coordination absorbers incorporating periodically patterned metal ring metasurfaces. Nanomaterials 2020, 10, 1102. [Google Scholar] [CrossRef] [PubMed]
- Zhu, X.L.; Yan, W.; Mortensen, N.A.; Xiao, S.S. Bends and splitters in graphene nanoribbon waveguides. Opt. Express 2013, 21, 3486–3491. [Google Scholar] [CrossRef] [Green Version]
- Ding, Y.; Guan, X.; Zhu, X.; Hu, H.; Bozhevolnyi, S.I.; Oxenløwe, L.K.; Jin, K.J.; Mortensen. N., A.; Xiao, S. Efficient electro-optic modulation in low-loss graphene-plasmonic slot waveguides. Nanoscale 2017, 9, 15576–15581. [Google Scholar] [CrossRef] [Green Version]
- Teng, D.; Wang, K.; Li, Z.; Cao, Q.; Tang, Y.N.; Zhao, Y.Z.; Liu, Z.Y.; Zhang, Y.W.; Guo, R.Z. Graphene gap plasmonic waveguide for deep-subwavelength transmission of mid-infrared waves. Acta Opt. Sin. 2020, 40, 0623002. [Google Scholar] [CrossRef]
- Gonçalves, P.A.D.; Dias, E.J.C.; Xiao, S.; Vasilevskiy, M.I.; Mortensen, N.A.; Peres, N.M.R. Graphene plasmons in triangular wedges and grooves. ACS Photonics 2016, 3, 2176–2183. [Google Scholar] [CrossRef] [Green Version]
- Xu, W.; Zhu, Z.H.; Liu, K.; Zhang, J.F.; Yuan, X.D.; Lu, Q.S.; Qin, S.Q. Dielectric loaded graphene plasmon waveguide. Opt. Express 2015, 23, 5147–5153. [Google Scholar] [CrossRef]
- Gao, Y.; Ren, G.; Zhu, B.; Liu, H.; Lian, Y.; Jian, S. Analytical model for plasmon modes in graphene-coated nanowire. Opt. Express 2014, 22, 24322–24331. [Google Scholar] [CrossRef]
- Teng, D.; Wang, K.; Li, Z.; Zhao, Y. Graphene-coated nanowire dimers for deep subwavelength waveguiding in mid-infrared range. Opt. Express 2019, 27, 12458–12469. [Google Scholar] [CrossRef] [PubMed]
- Wu, D.; Tian, J. Study on the plasmonic characteristics of bow-tie type graphene-coated nanowire pair. Optik 2018, 156, 689–695. [Google Scholar] [CrossRef]
- Teng, D.; Wang, K.; Li, Z. Graphene-coated nanowire waveguides and their applications. Nanomaterials 2020, 10, 229. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, D.; Tian, J.; Yang, R. Study of mode performances of graphene-coated nanowire integrated with triangle wedge substrate. J. Nonlinear Opt. Phys. 2018, 27, 1850013. [Google Scholar] [CrossRef]
- Teng, D.; Guo, J.K.; Yang, Y.D.; Ma, W.S.; Wang, K. Study of modal properties in graphene-coated nanowires integrated with substrates. Appl. Phys. B 2020, 126, 173. [Google Scholar] [CrossRef]
- Saeed, M.; Ghaffar, A.; Alkanhal, M.A.S.; Alqahtani, A.H.; Khan, Y.; Rehman, S. Plasmon modes supported by metamaterial-filled monolayer graphene cylindrical waveguides. J. Opt. Soc. Am. B 2020, 37, 3515–3520. [Google Scholar] [CrossRef]
- Hasan, K.B.M.R.; Islam, M.A.; Alam, M.S. Design of a broadband single mode hybrid plasmonic waveguide incorporating silicon nanowire. Opt. Mater. Express 2020, 10, 2783–2799. [Google Scholar] [CrossRef]
- Teng, D.; Yang, Y.D.; Guo, J.K.; Ma, W.S.; Tang, Y.N.; Wang, K. Efficient guiding mid-infrared waves with graphene-coated nanowire based plasmon waveguides. Results Phys. 2020, 17, 103169. [Google Scholar] [CrossRef]
- Huang, Y.; Zhang, L.; Yin, H.; Zhang, M.; Su, H.; Li, I.L.; Liang, H. Graphene-coated nanowires with a drop-shaped cross section for 10 nm confinement and 1 mm propagation. Opt. Lett. 2017, 42, 2078. [Google Scholar] [CrossRef]
- Teng, D.; Wang, K.; Li, Z.; Zhao, Y.; Zhao, G.; Li, H.; Wang, H. Graphene-coated elliptical nanowires for low loss subwavelength terahertz transmission. Appl. Sci. 2019, 9, 2351. [Google Scholar] [CrossRef] [Green Version]
- Zhou, X.; Zhang, T.; Chen, L.; Hong, W.; Li, X. A graphene-based hybrid plasmonic waveguide with ultra-deep subwavelength confinement. J. Lightwave Technol. 2014, 32, 4199–4203. [Google Scholar] [CrossRef]
- Chen, M.; Sheng, P.; Sun, W.; Cai, J. A symmetric terahertz graphene-based hybrid plasmonic waveguide. Opt. Commun. 2016, 376, 41–46. [Google Scholar] [CrossRef]
- Wan, P.; Yang, C.; Liu, Z. Channel hybrid plasmonic modes in dielectric-loaded graphene groove waveguides. Opt. Commun. 2018, 420, 72–77. [Google Scholar] [CrossRef]
- He, X.Q.; Ning, T.G.; Lu, S.; Li, J.; Li., R.; Pei, L. Ultralow loss graphene-based hybrid plasmonic waveguide with deep-subwavelength confinement. Opt. Express 2018, 26, 10109–10118. [Google Scholar] [CrossRef] [PubMed]
- He, X.Q.; Ning, T.G.; Pei, L.; Zheng, J.J.; Li, J.; Wen, X.D. Tunable hybridization of graphene plasmons and dielectric modes for highly confined light transmit at terahertz wavelength. Opt. Express 2019, 27, 5961–5972. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Yang, C.; Wan, P.; Ding, L.; Xu, W. Dielectric-loaded black phosphorus surface plasmon polariton waveguides. Opt. Express 2019, 27, 18005–18015. [Google Scholar] [CrossRef]
- Steinberger, B.; Hohenau, A.; Ditlbacher, H.; Stepanov, A.L.; Drezet, A.; Aussenegg, F.R.; Leitner, A.; Krenn, J.R. Dielectric stripes on gold as surface plasmon waveguides. Appl. Phys. Lett. 2006, 88, 094104. [Google Scholar] [CrossRef]
- Holmgaard, T.; Bozhevolnyi, S.I. Theoretical analysis of dielectric-loaded surface plasmon-polariton waveguides. Phys. Rev. B 2007, 75, 245405. [Google Scholar] [CrossRef]
- Hocker, G.B.; Burns, W.K. Mode dispersion in diffused channel waveguides by the effective index method. Appl. Opt. 1977, 16, 113–118. [Google Scholar] [CrossRef]
- Kogelnik, H.; Ramaswamy, V. Scaling rules for thin-film optical waveguides. Appl. Opt. 1974, 13, 1857–1862. [Google Scholar] [CrossRef]
- Xiao, S.Y.; Wang, T.; Liu, T.T.; Yan, X.; Li, Z.; Xu, C. Active modulation of electromagnetically induced transparency analogue in terahertz hybrid metal-graphene metamaterials. Carbon 2018, 126, 271–278. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.; Hong, Q.; Zou, J.; He, Y.; Yuan, X.; Zhu, Z.; Qin, S. Fano-resonance in hybrid metal-graphene metamaterial and its application as mid-infrared plasmonic sensor. Micromachines 2020, 11, 268. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhou, Y.; Zhu, Y.Y.; Zhang, K.; Wu, H.W.; Peng, R.W.; Fan, R.H.; Wang, M. Plasmonic band structures in doped graphene tubes. Opt. Express 2017, 25, 12081–12089. [Google Scholar] [CrossRef] [PubMed]
- Ahmadivand, A.; Gerislioglu, B.; Ramezani, Z. Gated graphene island-enabled tunable charge transfer plasmon terahertz metamodulator. Nanoscale 2019, 11, 8091–8095. [Google Scholar] [CrossRef] [PubMed]
- Efetov, D.K.; Kim, P. Controlling electron-phonon interactions in graphene at ultrahigh carrier densities. Phys. Rev. Lett. 2010, 105, 256805. [Google Scholar] [CrossRef] [Green Version]
- Kanahashi, K.; Tanaka, N.; Shoji, Y.; Maruyama, M.; Jeon, I.; Kawahara, K.; Ishihara, M.; Hasegawa, M.; Ohta, H.; Ago, H.; et al. Formation of environmentally stable hole-doped graphene films with instantaneous and high-density carrier doping via a boron-based oxidant. npj 2d Mater. Appl. 2019, 3, 44. [Google Scholar] [CrossRef] [Green Version]
- Jabbarzadeh, F.; Heydari, M.; Habibzadeh-Sharif, A. A comparative analysis of the accuracy of Kubo formulations for graphene plasmonics. Mater. Res. Express 2019, 6, 086209. [Google Scholar] [CrossRef]
- Ahmadivand, A.; Gerislioglu, B.; Ahuja, R.; Mishra, Y.K. Toroidal Metaphotonics and Metadevices. Laser Photonics Rev. 2020, 14, 1900326. [Google Scholar] [CrossRef]
- Veronis, G.; Fan, S. Crosstalk between three-dimensional plasmonic slot waveguides. Opt. Express 2008, 16, 2129–2140. [Google Scholar] [CrossRef] [Green Version]








Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Teng, D.; Wang, K. Theoretical Analysis of Terahertz Dielectric–Loaded Graphene Waveguide. Nanomaterials 2021, 11, 210. https://doi.org/10.3390/nano11010210
Teng D, Wang K. Theoretical Analysis of Terahertz Dielectric–Loaded Graphene Waveguide. Nanomaterials. 2021; 11(1):210. https://doi.org/10.3390/nano11010210
Chicago/Turabian StyleTeng, Da, and Kai Wang. 2021. "Theoretical Analysis of Terahertz Dielectric–Loaded Graphene Waveguide" Nanomaterials 11, no. 1: 210. https://doi.org/10.3390/nano11010210
APA StyleTeng, D., & Wang, K. (2021). Theoretical Analysis of Terahertz Dielectric–Loaded Graphene Waveguide. Nanomaterials, 11(1), 210. https://doi.org/10.3390/nano11010210