Fabrication and Conductive Mechanism Analysis of Stretchable Electrodes Based on PDMS-Ag Nanosheet Composite with Low Resistance, Stability, and Durability
Abstract
:1. Introduction
2. Experimental Section
2.1. Materials
2.2. Fabrication of the Stretchable Electrode
2.3. Characterization and Measurement of the Stretchable Electrode
2.4. Simulation Methods
3. Results and Discussion
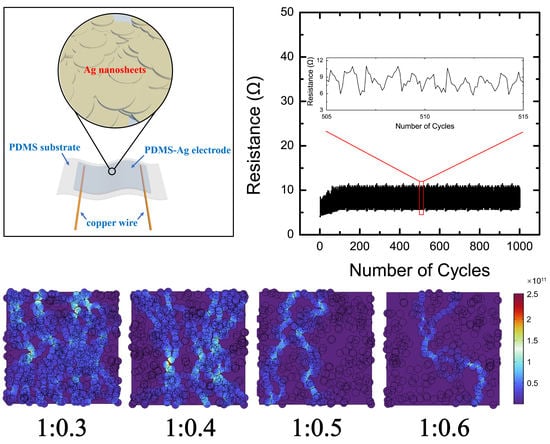
3.1. Morphology Characterization and Basic Electrical Properties of the Stretchable Electrodes
3.2. Conductive Performance Test of PDMS-Ag Electrodes during Stretching
3.3. Conductive Mechanism Analysis of PDMS-Ag Electrodes
3.4. Finite Element Analysis of the PDMS-Ag Electrodes
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Wang, C.Y.; Xia, K.L.; Wang, H.M.; Liang, X.P.; Yin, Z.; Zhang, Y.Y. Advanced Carbon for Flexible and Wearable Electronics. Adv. Mater. 2019, 31, 1801072. [Google Scholar] [CrossRef]
- Zhao, J.; Li, N.; Yu, H.; Wei, Z.; Liao, M.Z.; Chen, P.; Wang, S.P.; Shi, D.X.; Sun, Q.J.; Zhang, G.Y. Highly Sensitive MoS2 Humidity Sensors Array for Noncontact Sensation. Adv. Mater. 2017, 29, 1702076. [Google Scholar] [CrossRef]
- Li, C.W.; Zhang, Y.F.; Yang, S.T.; Zhao, H.T.; Guo, Y.; Cong, T.Z.; Huang, H.; Fan, Z.; Liang, H.W.; Pan, L.J. A flexible tissue-carbon nanocoil-carbon nanotube-based humidity sensor with high performance and durability. Nanoscale 2022, 14, 7025–7038. [Google Scholar] [CrossRef]
- Li, C.W.; Yang, S.T.; Guo, Y.; Huang, H.; Chen, H.; Zuo, X.Q.; Fan, Z.; Liang, H.W.; Pan, L.J. Flexible, multi-functional sensor based on all-carbon sensing medium with low coupling for ultrahigh-performance strain, temperature and humidity sensing. Chem. Eng. J. 2021, 426, 130364. [Google Scholar] [CrossRef]
- Liao, M.; Ye, L.; Zhang, Y.; Chen, T.Q.; Peng, H.S. The Recent Advance in Fiber-Shaped Energy Storage Devices. Adv. Electron. Mater. 2019, 5, 1800456. [Google Scholar] [CrossRef] [Green Version]
- Lv, T.; Yao, Y.; Li, N.; Chen, T. Wearable fiber-shaped energy conversion and storage devices based on aligned carbon nanotubes. Nano. Today 2016, 11, 644–660. [Google Scholar] [CrossRef]
- Tadesse, M.G.; Mengistie, D.A.; Chen, Y.; Wang, L.C.; Loghin, C.; Nierstrasz, V. Electrically conductive highly elastic polyamide/lycra fabric treated with PEDOT:PSS and polyurethane. J. Mater. Sci. 2019, 54, 9591–9602. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.P.; Wang, Y.S.; Chen, Y.J.; Yousaf, M.; Wu, H.S.; Cao, A.Y.; Han, R.P.S. Reticulate Dual-Nanowire Aerogel for Multifunctional Applications: A High-Performance Strain Sensor and a High Areal Capacity Rechargeable Anode. Adv. Funct. Mater. 2019, 29, 1807467. [Google Scholar] [CrossRef]
- Zhang, C.; Liu, S.Y.; Huang, X.; Guo, W.; Li, Y.Y.; Wu, H. A stretchable dual-mode sensor array for multifunctional robotic electronic skin. Nano. Energy 2019, 62, 164–170. [Google Scholar] [CrossRef]
- Yu, L.T.; Yeo, J.C.; Soon, R.H.; Yeo, T.; Lee, H.H.; Lim, C.T. Highly Stretchable, Weavable, and Washable Piezoresistive Microfiber Sensors. Acs. Appl. Mater. Inter. 2018, 10, 12773–12780. [Google Scholar] [CrossRef] [PubMed]
- Lv, Z.S.; Luo, Y.F.; Tang, Y.X.; Wei, J.Q.; Zhu, Z.Q.; Zhou, X.R.; Li, W.L.; Zeng, Y.; Zhang, W.; Zhang, Y.Y.; et al. Editable Supercapacitors with Customizable Stretchability Based on Mechanically Strengthened Ultralong MnO2 Nanowire Composite. Adv. Mater. 2018, 30, 1704531. [Google Scholar] [CrossRef] [PubMed]
- Trung, T.Q.; Kim, C.; Lee, H.B.; Cho, S.M.; Lee, N.E. Toward a Stretchable Organic Light-Emitting Diode on 3D Microstructured Elastomeric Substrate and Transparent Hybrid Anode. Adv. Mater. Technol. 2020, 5, 1900995. [Google Scholar] [CrossRef]
- An, B.W.; Gwak, E.J.; Kim, K.; Kim, Y.C.; Jang, J.; Kim, J.Y.; Park, J.U. Stretchable, Transparent Electrodes as Wearable Heaters Using Nanotrough Networks of Metallic Glasses with Superior Mechanical Properties and Thermal Stability. Nano. Lett. 2016, 16, 471–478. [Google Scholar] [CrossRef] [PubMed]
- Hwang, H.; Kim, D.G.; Jang, N.S.; Kong, J.H.; Kim, J.M. Simple method for high-performance stretchable composite conductors with entrapped air bubbles. Nanoscale Res. Lett. 2016, 11, 14. [Google Scholar] [CrossRef] [Green Version]
- Hong, H.C.; Chen, C.M. Design, Fabrication and Failure Analysis of Stretchable Electrical Routings. Sens. Basel 2014, 14, 11855–11877. [Google Scholar] [CrossRef]
- Qaiser, N.; Damdam, A.N.; Khan, S.M.; Bunaiyan, S.; Hussain, M.M. Design Criteria for Horseshoe and Spiral-Based Interconnects for Highly Stretchable Electronic Devices. Adv. Funct. Mater. 2021, 31, 2007445. [Google Scholar] [CrossRef]
- Webb, R.C.; Bonifas, A.P.; Behnaz, A.; Zhang, Y.H.; Yu, K.J.; Cheng, H.Y.; Shi, M.X.; Bian, Z.G.; Liu, Z.J.; Kim, Y.S.; et al. Ultrathin conformal devices for precise and continuous thermal characterization of human skin. Nat. Mater. 2013, 12, 938–944. [Google Scholar] [CrossRef]
- Tang, J.; Guo, H.; Zhao, M.M.; Yang, J.T.; Tsoukalas, D.; Zhang, B.Z.; Liu, J.; Xue, C.Y.; Zhang, W.D. Highly Stretchable Electrodes on Wrinkled Polydimethylsiloxane Substrates. Sci. Rep. 2015, 5, 16527. [Google Scholar] [CrossRef] [Green Version]
- Fan, J.A.; Yeo, W.H.; Su, Y.W.; Hattori, Y.; Lee, W.; Jung, S.Y.; Zhang, Y.H.; Liu, Z.J.; Cheng, H.Y.; Falgout, L.; et al. Fractal design concepts for stretchable electronics. Nat. Commun. 2014, 5, 3266. [Google Scholar] [CrossRef] [Green Version]
- Jeong, G.S.; Baek, D.H.; Jung, H.C.; Song, J.H.; Moon, J.H.; Hong, S.W.; Kim, I.Y.; Lee, S.H. Solderable and electroplatable flexible electronic circuit on a porous stretchable elastomer. Nat. Commun. 2012, 3, 977. [Google Scholar] [CrossRef]
- Chen, W.; Li, Y.D.; Li, R.Q.; Thean, A.V.Y.; Guo, Y.X. Bendable and Stretchable Microfluidic Liquid Metal-Based Filter. IEEE Microw. Wirel. Co. 2018, 28, 203–205. [Google Scholar] [CrossRef]
- Cao, Y.; Morrissey, T.G.; Acome, E.; Allec, S.I.; Wong, B.M.; Keplinger, C.; Wang, C. A Transparent, Self-Healing, Highly Stretchable Ionic Conductor. Adv. Mater. 2017, 29, 1605099. [Google Scholar] [CrossRef] [PubMed]
- Park, M.; Park, J.; Jeong, U. Design of conductive composite elastomers for stretchable electronics. Nano. Today 2014, 9, 244–260. [Google Scholar] [CrossRef]
- Ladd, C.; So, J.H.; Muth, J.; Dickey, M.D. 3D Printing of Free Standing Liquid Metal Microstructures. Adv. Mater. 2013, 25, 5081–5085. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Zhu, C.X.; Pfattner, R.; Yan, H.P.; Jin, L.H.; Chen, S.C.; Molina-Lopez, F.; Lissel, F.; Liu, J.; Rabiah, N.I.; et al. A highly stretchable, transparent, and conductive polymer. Sci. Adv. 2017, 3, e1602076. [Google Scholar] [CrossRef] [Green Version]
- Zhu, S.; So, J.H.; Mays, R.; Desai, S.; Barnes, W.R.; Pourdeyhimi, B.; Dickey, M.D. Ultrastretchable Fibers with Metallic Conductivity Using a Liquid Metal Alloy Core. Adv. Funct. Mater. 2013, 23, 2308–2314. [Google Scholar] [CrossRef]
- Hong, G.B.; Luo, Y.H.; Chuang, K.J.; Cheng, H.Y.; Chang, K.C.; Ma, C.M. Facile Synthesis of Silver Nanoparticles and Preparation of Conductive Ink. Nanomaterials-Basel 2022, 12, 171. [Google Scholar] [CrossRef]
- Kim, K.K.; Choi, J.; Kim, J.H.; Nam, S.; Ko, S.H. Evolvable Skin Electronics by In Situ and In Operando Adaptation. Adv. Funct. Mater. 2022, 32, 2106329. [Google Scholar] [CrossRef]
- Nguyen, H.L.; Jo, Y.K.; Cha, M.; Cha, Y.J.; Yoon, D.K.; Sanandiya, N.D.; Prajatelistia, E.; Oh, D.X.; Hwang, D.S. Mussel-Inspired Anisotropic Nanocellulose and Silver Nanoparticle Composite with Improved Mechanical Properties, Electrical Conductivity and Antibacterial Activity. Polymers-Basel 2016, 8, 102. [Google Scholar] [CrossRef] [Green Version]
- Yeo, J.; Kim, G.; Hong, S.; Kim, M.S.; Kim, D.; Lee, J.; Lee, H.B.; Kwon, J.; Suh, Y.D.; Kang, H.W.; et al. Flexible supercapacitor fabrication by room temperature rapid laser processing of roll-to-roll printed metal nanoparticle ink for wearable electronics application. J. Power Sources. 2014, 246, 562–568. [Google Scholar] [CrossRef]
- Wu, X.L.; Wang, S.Y.; Luo, Z.W.; Lu, J.X.; Lin, K.W.; Xie, H.; Wang, Y.H.; Li, J.Z. Inkjet Printing of Flexible Transparent Conductive Films with Silver Nanowires Ink. Nanomaterials-Basel 2021, 11, 1571. [Google Scholar] [CrossRef] [PubMed]
- Lee, P.; Lee, J.; Lee, H.; Yeo, J.; Hong, S.; Nam, K.H.; Lee, D.; Lee, S.S.; Ko, S.H. Highly Stretchable and Highly Conductive Metal Electrode by Very Long Metal Nanowire Percolation Network. Adv. Mater. 2012, 24, 3326–3332. [Google Scholar] [CrossRef] [PubMed]
- Vo, T.T.; Lee, H.J.; Kim, S.Y.; Suk, J.W. Synergistic Effect of Graphene/Silver Nanowire Hybrid Fillers on Highly Stretchable Strain Sensors Based on Spandex Composites. Nanomaterials-Basel 2020, 10, 2063. [Google Scholar] [CrossRef] [PubMed]
- Won, P.; Park, J.J.; Lee, T.; Ha, I.; Han, S.; Choi, M.; Lee, J.; Hong, S.; Cho, K.J.; Ko, S.H. Stretchable and Transparent Kirigami Conductor of Nanowire Percolation Network for Electronic Skin Applications. Nano. Lett. 2019, 19, 6087–6096. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jung, J.; Cho, H.; Yuksel, R.; Kim, D.; Lee, H.; Kwon, J.; Lee, P.; Yeo, J.; Hong, S.; Unalan, H.E.; et al. Stretchable/flexible silver nanowire electrodes for energy device applications. Nanoscale 2019, 11, 20356–20378. [Google Scholar] [CrossRef]
- Cho, J.H.; Kang, D.J.; Jang, N.S.; Kim, K.H.; Won, P.; Ko, S.H.; Kim, J.M. Metal Nanowire-Coated Metal Woven Mesh for High-Performance Stretchable Transparent Electrodes. Acs. Appl. Mater. Inter. 2017, 9, 40905–40913. [Google Scholar] [CrossRef]
- Ge, Y.J.; Duan, X.D.; Zhang, M.; Mei, L.; Hu, J.W.; Hu, W.; Duan, X.F. Direct Room Temperature Welding and Chemical Protection of Silver Nanowire Thin Films for High Performance Transparent Conductors. J. Am. Chem. Soc. 2018, 140, 193–199. [Google Scholar] [CrossRef]
- Song, T.B.; Chen, Y.; Chung, C.H.; Yang, Y.; Bob, B.; Duan, H.S.; Li, G.; Tu, K.N.; Huang, Y.; Yang, Y. Nanoscale Joule Heating and Electromigration Enhanced Ripening of Silver Nanowire Contacts. Acs. Nano. 2014, 8, 2804–2811. [Google Scholar] [CrossRef]
- Tokuno, T.; Nogi, M.; Karakawa, M.; Jiu, J.T.; Nge, T.T.; Aso, Y.; Suganuma, K. Fabrication of silver nanowire transparent electrodes at room temperature. Nano. Res. 2011, 4, 1215–1222. [Google Scholar] [CrossRef]
- Celano, T.A.; Hill, D.J.; Zhang, X.; Pinion, C.W.; Christesen, J.D.; Flynn, C.J.; McBride, J.R.; Cahoon, J.F. Capillarity-Driven Welding of Semiconductor Nanowires for Crystalline and Electrically Ohmic Junctions. Nano. Lett. 2016, 16, 5241–5246. [Google Scholar] [CrossRef] [PubMed]
- Lu, H.F.; Zhang, D.; Cheng, J.Q.; Liu, J.; Mao, J.; Choy, W.C.H. Locally Welded Silver Nano-Network Transparent Electrodes with High Operational Stability by a Simple Alcohol-Based Chemical Approach. Adv. Funct. Mater. 2015, 25, 4211–4218. [Google Scholar] [CrossRef]
- Lee, J.; Lee, P.; Lee, H.; Lee, D.; Lee, S.S.; Ko, S.H. Very long Ag nanowire synthesis and its application in a highly transparent, conductive and flexible metal electrode touch panel. Nanoscale 2012, 4, 6408–6414. [Google Scholar] [CrossRef]
- Lee, J.; Lee, P.; Lee, H.B.; Hong, S.; Lee, I.; Yeo, J.; Lee, S.S.; Kim, T.S.; Lee, D.; Ko, S.H. Room-Temperature Nanosoldering of a Very Long Metal Nanowire Network by Conducting-Polymer-Assisted Joining for a Flexible Touch-Panel Application. Adv. Funct. Mater. 2013, 23, 4171–4176. [Google Scholar] [CrossRef]
- Li, C.W.; Pan, L.J.; Deng, C.H.; Wang, P.; Huang, Y.Y.; Nasir, H. A flexible, ultra-sensitive strain sensor based on carbon nanocoil network fabricated by an electrophoretic method. Nanoscale 2017, 9, 9872–9878. [Google Scholar] [CrossRef] [PubMed]
- Li, C.W.; Pan, L.J.; Deng, C.H.; Cong, T.Z.; Yin, P.H.; Wu, Z.L. A highly sensitive and wide-range pressure sensor based on a carbon nanocoil network fabricated by an electrophoretic method. J. Mater. Chem. C 2017, 5, 11892–11900. [Google Scholar] [CrossRef]
- Xu, S.; Rezvanian, O.; Peters, K.; Zikry, M.A. The viability and limitations of percolation theory in modeling the electrical behavior of carbon nanotube-polymer composites. Nanotechnology 2013, 24, 155706. [Google Scholar] [CrossRef]
- Dang, Z.M.; Jiang, M.J.; Xie, D.; Yao, S.H.; Zhang, L.Q.; Bai, J.B. Supersensitive linear piezoresistive property in carbon nanotubes/silicone rubber nanocomposites. J. Appl. Phys. 2008, 104, 024114. [Google Scholar] [CrossRef]
- Chen, L.; Chen, G.H.; Lu, L. Piezoresistive behavior study on finger-sensing silicone rubber/graphite nanosheet nanocomposites. Adv. Funct. Mater. 2007, 17, 898–904. [Google Scholar] [CrossRef]
- Li, C.W.; Pan, L.J.; Deng, C.H.; Wang, P. CNC-Al2O3-Ti: A new unit for micro scale strain sensing. Rsc. Adv. 2016, 6, 107683–107688. [Google Scholar] [CrossRef]









Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Huang, K.; Yuan, T.; Cong, T.; Fan, Z.; Pan, L. Fabrication and Conductive Mechanism Analysis of Stretchable Electrodes Based on PDMS-Ag Nanosheet Composite with Low Resistance, Stability, and Durability. Nanomaterials 2022, 12, 2628. https://doi.org/10.3390/nano12152628
Li C, Huang K, Yuan T, Cong T, Fan Z, Pan L. Fabrication and Conductive Mechanism Analysis of Stretchable Electrodes Based on PDMS-Ag Nanosheet Composite with Low Resistance, Stability, and Durability. Nanomaterials. 2022; 12(15):2628. https://doi.org/10.3390/nano12152628
Chicago/Turabian StyleLi, Chengwei, Kai Huang, Tingkang Yuan, Tianze Cong, Zeng Fan, and Lujun Pan. 2022. "Fabrication and Conductive Mechanism Analysis of Stretchable Electrodes Based on PDMS-Ag Nanosheet Composite with Low Resistance, Stability, and Durability" Nanomaterials 12, no. 15: 2628. https://doi.org/10.3390/nano12152628
APA StyleLi, C., Huang, K., Yuan, T., Cong, T., Fan, Z., & Pan, L. (2022). Fabrication and Conductive Mechanism Analysis of Stretchable Electrodes Based on PDMS-Ag Nanosheet Composite with Low Resistance, Stability, and Durability. Nanomaterials, 12(15), 2628. https://doi.org/10.3390/nano12152628