Modeling the Additive Effects of Nanoparticles and Polymers on Hydrogel Mechanical Properties Using Multifactor Analysis
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Polymerization Reaction
2.3. Rheological Measurements
2.4. Factorial Design
2.5. Multivariate Analysis of Variance
2.6. Discriminant Analysis Using Han Plots
3. Results
3.1. Saturation in Nanoparticle Mediated Enhancement of Hydrogel Mechanical Properties
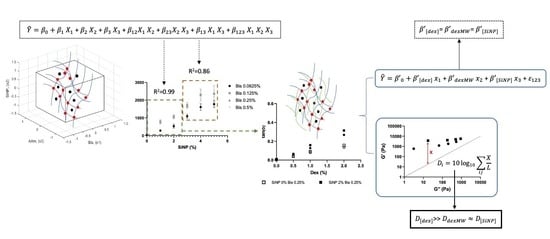
3.2. Factorial Design Models Identify the Significance of Hydrogel Nanocomposite Parameters
3.3. Design of the Interpenetrating Network Hydrogel Nanocomposite Studies
3.4. Effect of Dextran on the Elastic and Viscous Properties of Hydrogel Nanocomposites
3.5. Statistical Analyses of the Interpenetrating Network Hydrogel Nanocomposites
4. Discussion and Future Work
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dragan, E. Design and Applications of Interpenetrating Polymer Network Hydrogels. A Review. Chem. Eng. J. 2014, 243, 572–590. [Google Scholar] [CrossRef]
- Yu, Z.; Zhang, Y.; Gao, Z.J.; Ren, X.Y.; Gao, G.H. Enhancing Mechanical Strength of Hydrogels via IPN Structure. J. Appl. Polym. Sci. 2017, 134, 44503. [Google Scholar] [CrossRef]
- Naseri, N.; Deepa, B.; Mathew, A.P.; Oksman, K.; Girandon, L. Nanocellulose-Based Interpenetrating Polymer Network (IPN) Hydrogels for Cartilage Applications. Biomac 2016, 17, 3714–3723. [Google Scholar] [CrossRef] [PubMed]
- Dinu, M.V.; Schwarz, S.; Dinu, I.A.; Drăgan, E.S. Comparative Rheological Study of Ionic Semi-IPN Composite Hydrogels Based on Polyacrylamide and Dextran Sulphate and of Polyacrylamide Hydrogels. Colloid Polym. Sci. 2012, 290, 1647–1657. [Google Scholar] [CrossRef]
- Dinu, M.V.; Perju, M.M.; Drăgan, E.S. Composite IPN Ionic Hydrogels Based on Polyacrylamide and Dextran Sulfate. React. Funct. Polym. 2011, 71, 881–890. [Google Scholar] [CrossRef]
- Wang, J.; Hu, H.; Yang, Z.; Wei, J.; Li, J. IPN Hydrogel Nanocomposites Based on Agarose and ZnO with Antifouling and Bactericidal Properties. Mater. Sci. Eng. C Mater. Biol. Appl. 2016, 61, 376–386. [Google Scholar] [CrossRef]
- Kheirabadi, M.; Bagheri, R.; Kabiri, K. Swelling and Mechanical Behavior of Nanoclay Reinforced Hydrogel: Single Network vs. Full Interpenetrating Polymer Network. Polym. Bull. 2015, 72, 1663–1681. [Google Scholar] [CrossRef]
- Haraguchi, K.; Murata, K.; Takehisa, T. Stimuli-Responsive Nanocomposite Gels and Soft Nanocomposites Consisting of Inorganic Clays and Copolymers with Different Chemical Affinities. Macromolecules 2012, 45, 385–391. [Google Scholar] [CrossRef]
- Haraguchi, K.; Farnworth, R.; Ohbayashi, A.; Takehisa, T. Compositional Effects on Mechanical Properties of Nanocomposite Hydrogels Composed of Poly(N,N-Dimethylacrylamide) and Clay. Macromolecules 2003, 36, 5732–5741. [Google Scholar] [CrossRef]
- Haraguchi, K.; Li, H.-J. Mechanical Properties and Structure of Polymer-Clay Nanocomposite Gels with High Clay Content. Macromolecules 2006, 39, 1898–1905. [Google Scholar] [CrossRef]
- Haraguchi, K.; Song, L. Microstructures Formed in Co-Cross-Linked Networks and Their Relationships to the Optical and Mechanical Properties of PNIPA/Clay Nanocomposite Gels. Macromolecules 2007, 40, 5526–5536. [Google Scholar] [CrossRef]
- Liu, R.; Liang, S.; Tang, X.-Z.; Yan, D.; Li, X.; Yu, Z.-Z. Tough and Highly Stretchable Graphene Oxide/Polyacrylamide Nanocomposite Hydrogels. J. Mater. Chem. 2012, 22, 14160–14167. [Google Scholar] [CrossRef]
- Zoratto, N.; Matricardi, P. 4-Semi-IPNs and IPN-Based Hydrogels. In Polymeric Gels; Pal, K., Banerjee, I., Eds.; Woodhead Publishing Series in Biomaterials; Woodhead Publishing: Sawston, UK, 2018; pp. 91–124. ISBN 978-0-08-102179-8. [Google Scholar]
- Myung, D.; Koh, W.; Ko, J.; Hu, Y.; Carrasco, M.; Noolandi, J.; Ta, C.N.; Frank, C.W. Biomimetic Strain Hardening in Interpenetrating Polymer Network Hydrogels. Polymer 2007, 48, 5376–5387. [Google Scholar] [CrossRef]
- Myung, D.; Waters, D.; Wiseman, M.; Duhamel, P.-E.; Noolandi, J.; Ta, C.N.; Frank, C.W. Progress in the Development of Interpenetrating Polymer Network Hydrogels. Polym. Adv. Technol. 2008, 19, 647–657. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gu, Z.; Huang, K.; Luo, Y.; Zhang, L.; Kuang, T.; Chen, Z.; Liao, G. Double Network Hydrogel for Tissue Engineering. WIREs Nanomed. Nanobiotechnol. 2018, 10, e1520. [Google Scholar] [CrossRef]
- Haque, M.A.; Kurokawa, T.; Gong, J.P. Super Tough Double Network Hydrogels and Their Application as Biomaterials. Polymer 2012, 53, 1805–1822. [Google Scholar] [CrossRef]
- Nonoyama, T.; Gong, J.P. Double-Network Hydrogel and Its Potential Biomedical Application:A Review. J. Eng. Med. 2015, 229, 853–863. [Google Scholar] [CrossRef]
- Panteli, P.; Patrickios, C. Multiply Interpenetrating Polymer Networks: Preparation, Mechanical Properties, and Applications. Gels 2019, 5, 36. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Levin, A.; Sharma, V.; Hook, L.; García-Gareta, E. The Importance of Factorial Design in Tissue Engineering and Biomaterials Science: Optimisation of Cell Seeding Efficiency on Dermal Scaffolds as a Case Study. J. Tissue Eng. 2018, 9, 2041731418781696. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.; Li, J. One-Pot Synthesis of IPN Hydrogels with Enhanced Mechanical Strength for Synergistic Adsorption of Basic Dyes. Soft Mater. 2015, 13, 160–166. [Google Scholar] [CrossRef]
- Zhang, Q.; Fang, Z.; Cao, Y.; Du, H.; Wu, H.; Beuerman, R.; Chan-Park, M.; Duan, H.; Xu, R. High Refractive Index Inorganic−Organic Interpenetrating Polymer Network (IPN) Hydrogel Nanocomposite toward Artificial Cornea Implants. ACS Macro Lett. 2012, 1, 876–881. [Google Scholar] [CrossRef] [PubMed]
- Olad, A.; Doustdar, F.; Gharekhani, H. Starch-Based Semi-IPN Hydrogel Nanocomposite Integrated with Clinoptilolite: Preparation and Swelling Kinetic Study. Carbohydr. Polym. 2018, 200, 516–528. [Google Scholar] [CrossRef] [PubMed]
- Chang, A.; Babhadiashar, N.; Barrett-Catton, E.; Asuri, P. Role of Nanoparticle–Polymer Interactions on the Development of Double-Network Hydrogel Nanocomposites with High Mechanical Strength. Polymer 2020, 12, 470. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lu, X.; Mi, Y. Characterization of the Interfacial Interaction between Polyacrylamide and Silicon Substrate by Fourier Transform Infrared Spectroscopy. Macromolecules 2005, 38, 839–843. [Google Scholar] [CrossRef]
- Wu, L.; Zeng, L.; Chen, H.; Zhang, C. Effects of Silica Sol Content on the Properties of Poly(Acrylamide)/Silica Composite Hydrogel. Polym. Bull. 2012, 68, 309–316. [Google Scholar] [CrossRef]
- Zaragoza, J.; Babhadiashar, N.; O’Brien, V.; Chang, A.; Blanco, M.; Zabalegui, A.; Lee, H.; Asuri, P. Experimental Investigation of Mechanical and Thermal Properties of Silica Nanoparticle-Reinforced Poly(Acrylamide) Nanocomposite Hydrogels. PLoS ONE 2015, 10, e0136293. [Google Scholar] [CrossRef]
- Zaragoza, J.; Chang, A.; Asuri, P. Effect of Crosslinker Length on the Elastic and Compression Modulus of Poly(Acrylamide) Nanocomposite Hydrogels. J. Phys. Conf. Ser. 2017, 790, 012037. [Google Scholar] [CrossRef] [Green Version]
- Zaragoza, J.; Fukuoka, S.; Kraus, M.; Thomin, J.; Asuri, P. Exploring the Role of Nanoparticles in Enhancing Mechanical Properties of Hydrogel Nanocomposites. Nanomaterials 2018, 8, 882. [Google Scholar] [CrossRef] [Green Version]
- Lam, J.; Kim, K.; Lu, S.; Tabata, Y.; Scott, D.W.; Mikos, A.G.; Kasper, F.K. A Factorial Analysis of the Combined Effects of Hydrogel Fabrication Parameters on the in Vitro Swelling and Degradation of Oligo(Poly(Ethylene Glycol) Fumarate) Hydrogels. J. Biomed. Mater. Res. A 2014, 102, 3477–3487. [Google Scholar] [CrossRef] [Green Version]
- Gajra, B.; Pandya, S.S.; Singh, S.; Rabari, H.A. Mucoadhesive Hydrogel Films of Econazole Nitrate: Formulation and Optimization Using Factorial Design. J. Drug Deliv. 2014, 2014, e305863. [Google Scholar] [CrossRef]
- Stanojević, M.; Krušić, M.K.; Filipović, J.; Parojčić, J.; Stupar, M. An Investigation into the Influence of Hydrogel Composition on Swelling Behavior and Drug Release from Poly(Acrylamide-Co-Itaconic Acid) Hydrogels in Various Media. Drug Deliv. 2006, 13, 34. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jin, X.; Wei, C.; Wu, C.; Zhang, W. Gastric Fluid-Induced Double Network Hydrogel with High Swelling Ratio and Long-Term Mechanical Stability. Compos. Part B Eng. 2022, 236, 109816. [Google Scholar] [CrossRef]
- Rodell, C.B.; Dusaj, N.N.; Highley, C.B.; Burdick, J.A. Injectable and Cytocompatible Tough Double-Network Hydrogels through Tandem Supramolecular and Covalent Crosslinking. Adv. Mater. 2016, 28, 8419–8424. [Google Scholar] [CrossRef] [PubMed]
- Shahriari-Khalaji, M.; Hong, S.; Hu, G.; Ji, Y.; Hong, F.F. Bacterial Nanocellulose-Enhanced Alginate Double-Network Hydrogels Cross-Linked with Six Metal Cations for Antibacterial Wound Dressing. Polymers 2020, 12, 2683. [Google Scholar] [CrossRef] [PubMed]
- Cai, L.; Dewi, R.E.; Heilshorn, S.C. Injectable Hydrogels with In Situ Double Network Formation Enhance Retention of Transplanted Stem Cells. Adv. Funct. Mater. 2015, 25, 1344–1351. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fung, T.-M.; Lazo, C.G.; Smith, A.M. Elasticity and Energy Dissipation in the Double Network Hydrogel Adhesive of the Slug Arion Subfuscus. Philos. Trans. R. Soc. B Biol. Sci. 2019, 374, 20190201. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Li, G.; Huang, K.; Deng, J.; Guo, M.; Cai, M.; Zhang, Y.; Guo, C.F. Highly Conducting and Stretchable Double-Network Hydrogel for Soft Bioelectronics. Adv. Mater. 2022, 34, 2200261. [Google Scholar] [CrossRef]
- Netsopa, S.; Niamsanit, S.; Sakloetsakun, D.; Milintawisamai, N. Characterization and Rheological Behavior of Dextran from Weissella Confusa R003. Int. J. Polym. Sci. 2018, 5790526, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Ahmad, N.H.; Ahmed, J.; Hashim, D.M.; Manap, Y.A.; Mustafa, S. Oscillatory and Steady Shear Rheology of Gellan/Dextran Blends. J. Food Sci. Technol. 2015, 52, 2902–2909. [Google Scholar] [CrossRef] [Green Version]
- McCann, J.; Behrendt, J.M.; Yan, J.; Halacheva, S.; Saunders, B.R. Poly(Vinylamine) Microgel–Dextran Composite Hydrogels: Characterisation; Properties and PH-Triggered Degradation. J. Colloid Interface Sci. 2015, 449, 21–30. [Google Scholar] [CrossRef]
- Pescosolido, L.; Schuurman, W.; Malda, J.; Matricardi, P.; Alhaique, F.; Coviello, T.; van Weeren, P.R.; Dhert, W.J.A.; Hennink, W.E.; Vermonden, T. Hyaluronic Acid and Dextran-Based Semi-IPN Hydrogels as Biomaterials for Bioprinting. Biomacromolecules 2011, 12, 1831–1838. [Google Scholar] [CrossRef] [PubMed]
- Box, G.E.P.; Hunter, J.S.; Hunter, W.G. Statistics for Experimenters: Design, Innovation, and Discovery, 2nd ed.; Wiley: Hoboken, NJ, USA, 2005; ISBN 978-0-471-71813-0. [Google Scholar]
- Tayefi, M.; Razavi-Nouri, M.; Sabet, A. Influence of Ordering and Disordering of Organoclay on Rheological Properties of Uncured and Cured Ethylene-Octene Copolymer Nanocomposites. Appl. Clay Sci. 2017, 135, 206–214. [Google Scholar] [CrossRef]
- Mohammadi, M.; Yousefi, A.A.; Ehsani, M. Thermorheological Analysis of Blend of High- and Low-Density Polyethylenes. J. Polym. Res. 2012, 19, 9798. [Google Scholar] [CrossRef]
- Gao, G.; Xiao, Y.; Wang, Q.; Fu, J. Synergistic Toughening of Nanocomposite Double Network Hydrogels by Physical Adsorption and Chemical Bonding of Polymer Chains to Inorganic Nanospheres and Nanorods: A Comparative Study. RSC Adv. 2016, 6, 37974–37981. [Google Scholar] [CrossRef]
- Wang, Q.; Hou, R.; Cheng, Y.; Fu, J. Super-Tough Double-Network Hydrogels Reinforced by Covalently Compositing with Silica-Nanoparticles. Soft Matter. 2012, 8, 6048–6056. [Google Scholar] [CrossRef]
- Domínguez, J.C.; Oliet, M.; Alonso, M.V.; Rojo, E.; Rodríguez, F. Structural, Thermal and Rheological Behavior of a Bio-Based Phenolic Resin in Relation to a Commercial Resol Resin. Ind. Crops Prod. 2013, 42, 308–314. [Google Scholar] [CrossRef]
- Zare, Y.; Park, S.P.; Rhee, K.Y. Analysis of Complex Viscosity and Shear Thinning Behavior in Poly (Lactic Acid)/Poly (Ethylene Oxide)/Carbon Nanotubes Biosensor Based on Carreau–Yasuda Model. Results Phys. 2019, 13, 102245. [Google Scholar] [CrossRef]
- Zhang, H.; Gao, T.; Zhang, S.; Zhang, P.; Li, R.; Ma, N.; Wei, H.; Zhang, X. Conductive and Tough Smart Poly(N-Isopropylacrylamide) Hydrogels Hybridized by Green Deep Eutectic Solvent. Macromol. Chem. Phys. 2021, 222, 2000301. [Google Scholar] [CrossRef]
- Yan, C.; Pochan, D.J. Rheological Properties of Peptide-Based Hydrogels for Biomedical and Other Applications. Chem. Soc. Rev. 2010, 39, 3528–3540. [Google Scholar] [CrossRef] [Green Version]
- Uman, S.; Dhand, A.; Burdick, J.A. Recent Advances in Shear-Thinning and Self-Healing Hydrogels for Biomedical Applications. J. Appl. Polym. Sci. 2020, 137, 48668. [Google Scholar] [CrossRef]
- Zandi, N.; Sani, E.S.; Mostafavi, E.; Ibrahim, D.M.; Saleh, B.; Shokrgozar, M.A.; Tamjid, E.; Weiss, P.S.; Simchi, A.; Annabi, N. Nanoengineered Shear-Thinning and Bioprintable Hydrogel as a Versatile Platform for Biomedical Applications. Biomaterials 2021, 267, 120476. [Google Scholar] [CrossRef] [PubMed]





| Level | Bis Low | Bis High | SiNP Low | SiNP High | AAm |
|---|---|---|---|---|---|
| −1 | 0.0625 | 0.125 | 0 | 3 | 2.5 |
| 0 | 0.125 | 0.25 | 1 | 4 | 5 |
| 1 | 0.25 | 0.5 | 2 | 5 | 10 |
| Variable | Level (s) |
|---|---|
| Bis (x1) | 0.0625%, 0.125% |
| SiNP (x2) | 0%, 2% |
| Dextran MW (x3) | 100 kDa, 500 kDa |
| Dextran Concentration (x4) | 0%, 0.5%, 1%, 2% |
| Estimate | Std Error | t Ratio | Prob > |t| | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Term | 2a | 2b | 2c | 2a | 2b | 2c | 2a | 2b | 2c | 2a | 2b | 2c |
| β0 | 7300.06 | 7758.30 | 6041.55 | 1318.13 | 1137.70 | 125.14 | 5.54 | 6.82 | 48.28 | <0.0001 | <0.0001 | <0.0001 |
| β1 | 5964.70 | 5356.50 | 4757.83 | 1571.32 | 1356.22 | 149.18 | 3.8 | 3.95 | 31.89 | 0.0012 | 0.0009 | <0.0001 |
| β2 | 1825.21 | 1530.29 | 2010.72 | 1571.32 | 516.50 | 149.18 | 1.16 | 2.96 | 13.48 | 0.2598 | 0.0080 | <0.0001 |
| β3 | 1231.53 | 883.41 | 1189.84 | 610.18 | 526.65 | 96.93 | 2.02 | 1.68 | 12.27 | 0.0579 | 0.1098 | <0.0001 |
| β12 | 580.62 | 1771.09 | 1665.38 | 1873.13 | 615.71 | 177.83 | 0.31 | 2.88 | 9.36 | 0.76 | 0.0097 | <0.0001 |
| β13 | 73.40 | 280.22 | 599.80 | 727.38 | 627.81 | 115.55 | 0.1 | 0.45 | 5.19 | 0.9207 | 0.6604 | <0.0001 |
| β23 | −178.42 | 36.75 | 169.53 | 727.38 | 239.09 | 115.55 | −0.25 | 0.15 | 1.47 | 0.8089 | 0.8795 | 0.1587 |
| β123 | 295.50 | 66.60 | 155.33 | 867.09 | 285.02 | 137.75 | 0.34 | 0.23 | 1.13 | 0.737 | 0.8178 | 0.2735 |
| Bis (%) | Dex (%) | Dex 100 kDa | Dex 500 kDa | ||
|---|---|---|---|---|---|
| SiNP 0% | SiNP 2% | SiNP 0% | SiNP 2% | ||
| 0.0625 | 0 | 6.77 | 3.39 | 8.34 | 5.3 |
| 0.0625 | 0.5 | 4.81 | 2.21 | 6.78 | 3.88 |
| 0.0625 | 1 | 3.1 | 1.21 | 4.45 | 2.28 |
| 0.0625 | 2 | 0 | 0 | 0 | 0 |
| 0.125 | 0 | 4.68 | 4.82 | 5.05 | 5.89 |
| 0.125 | 0.5 | 4.05 | 3.66 | 4.61 | 5.11 |
| 0.125 | 1 | 3.23 | 2.52 | 3.81 | 4.05 |
| 0.125 | 2 | 2.75 | 1.53 | 2.75 | 1.53 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barrett-Catton, E.; Pedersen, K.; Mobed-Miremadi, M.; Asuri, P. Modeling the Additive Effects of Nanoparticles and Polymers on Hydrogel Mechanical Properties Using Multifactor Analysis. Nanomaterials 2022, 12, 4461. https://doi.org/10.3390/nano12244461
Barrett-Catton E, Pedersen K, Mobed-Miremadi M, Asuri P. Modeling the Additive Effects of Nanoparticles and Polymers on Hydrogel Mechanical Properties Using Multifactor Analysis. Nanomaterials. 2022; 12(24):4461. https://doi.org/10.3390/nano12244461
Chicago/Turabian StyleBarrett-Catton, Emma, Kyle Pedersen, Maryam Mobed-Miremadi, and Prashanth Asuri. 2022. "Modeling the Additive Effects of Nanoparticles and Polymers on Hydrogel Mechanical Properties Using Multifactor Analysis" Nanomaterials 12, no. 24: 4461. https://doi.org/10.3390/nano12244461
APA StyleBarrett-Catton, E., Pedersen, K., Mobed-Miremadi, M., & Asuri, P. (2022). Modeling the Additive Effects of Nanoparticles and Polymers on Hydrogel Mechanical Properties Using Multifactor Analysis. Nanomaterials, 12(24), 4461. https://doi.org/10.3390/nano12244461