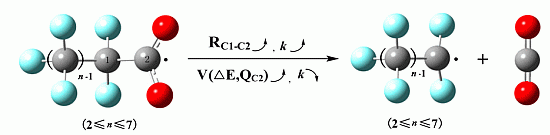Next Article in Journal
Modulation of Nitrosative Stress via Glutathione-Dependent Formaldehyde Dehydrogenase and S-Nitrosoglutathione Reductase
Next Article in Special Issue
A Combined Experimental and Computational Study of Vam3, a Derivative of Resveratrol, and Syk Interaction
Previous Article in Journal
Genetic Breeding and Diversity of the Genus Passiflora: Progress and Perspectives in Molecular and Genetic Studies
Previous Article in Special Issue
Computational Study on Substrate Specificity of a Novel Cysteine Protease 1 Precursor from Zea mays
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
IJMS
Volume 15
Issue 8
10.3390/ijms150814153
ijms-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Kinetics and Quantitative Structure—Activity Relationship Study on the Degradation Reaction from Perfluorooctanoic Acid to Trifluoroacetic Acid

by
Chen Gong
1,
Xiaomin Sun
1,*,
Chenxi Zhang
1,
Xue Zhang
1 and
Junfeng Niu
2,*
1
Environment Research Institute, Shandong University, Jinan 250100, China
2
State Key Laboratory of Water Environment Simulation, School of Environment, Beijing Normal University, Beijing 100875, China
*
Authors to whom correspondence should be addressed.
Int. J. Mol. Sci. 2014, 15(8), 14153-14165; https://doi.org/10.3390/ijms150814153
Submission received: 1 March 2014 / Revised: 5 June 2014 / Accepted: 19 July 2014 / Published: 14 August 2014
(This article belongs to the Collection Computational, Structural and Spectroscopic Studies of Enzyme Mechanisms, Inhibition and Dynamics)
Browse Figures
Versions Notes

Abstract

:
Investigation of the degradation kinetics of perfluorooctanoic acid (PFOA) has been carried out to calculate rate constants of the main elementary reactions using the multichannel Rice-Ramsperger-Kassel-Marcus theory and canonical variational transition state theory with small-curvature tunneling correction over a temperature range of 200~500 K. The Arrhenius equations of rate constants of elementary reactions are fitted. The decarboxylation is role step in the degradation mechanism of PFOA. For the perfluorinated carboxylic acids from perfluorooctanoic acid to trifluoroacetic acid, the quantitative structure–activity relationship of the decarboxylation was analyzed with the genetic function approximation method and the structure–activity model was constructed. The main parameters governing rate constants of the decarboxylation reaction from the eight-carbon chain to the two-carbon chain were obtained. As the structure–activity model shows, the bond length and energy of C1–C2 (RC1–C2 and EC1–C2) are positively correlated to rate constants, while the volume (V), the energy difference between EHOMO and ELUMO (ΔE), and the net atomic charges on atom C2 (QC2) are negatively correlated.

Graphical Abstract

1. Introduction

Perfluorinated carboxylic acids (PFCAs) have been widely used in industry as surfactants, surface treatment agents, metal coating, fire retardants and carpet cleaners for many years [1,2,3,4]. As a typical perfluorinated acid, the production of perfluorooctanoic acid (PFOA) worldwide exceeded 1000 metric tons in 2004 [5]. PFOA is a new persistent organic pollutant and difficult to decompose in the environment [6]. Due to its long-range oceanic and atmospheric transportation ability, PFOA has been detected in various environmental matrices such as water, dust, sediments and living tissues [7,8,9]. Research shows that levels of PFOA in wildlife range from 0.05 ng/mL in the blood of cod collected from European waters to 8.14 ng/mL in the plasma of loggerhead sea turtles from North America [10,11]. It can be also accumulated in creatures through water, food and atmosphere, causing the decrease in fertility rate, birth weight and other immune system diseases [12,13,14,15,16,17,18]. Although the environmental protection agency of the United States (US-EPA) and the European environment agency (EEA) have adopted an industrial program in order to reduce the global emissions of PFOA [19,20], the remained PFOA in the environment still has potential risk on humans. So, it is important and urgent to find an effective degradation method.
Recently, electrochemical treatment of PFOA has been considered one of the most promising methods due to its strong oxidation and environmental compatibility [6]. What is more, Hoffman et al. found that PFOA-contaminated drinking water is a significant contributor to PFOA levels in serum [21]. Researchers have found that PFOA could be degraded over boron-doped diamond (BDD) film electrode and Ti/SnO2-Sb-Bi electrode [22,23,24]. The reaction mechanism is proposed as the direct electrochemical oxidation cleaves the C-C bond between the C7F15 and COOH in PFOA to generate a C7F15 radical and CO2 firstly [24]. Zhuo et al. studied the electrochemical oxidation of PFOA using Ti/SnO2-Sb-Bi anode, and they found that after 2 h electrolysis, over 99% of PFOA was degraded with a first-order kinetic constant of 1.93 h−1. Then, the degradation mechanism was revealed according to the intermediate products detected [23]. Lin et al. studied the electrochemical degradation efficiency of PFOA using different anode, current density, pH value, plate distance and concentration. They also put forward a reaction mechanism in accordance with experiment results [6]. Although much work has been done to study the degradation effect of electrochemistry, the degradation mechanism has been partly revealed, and the rate constants of elementary reactions have not been reported so far. The reaction rate is helpful to find an optimal reaction way, then a kinetics study is needed. Furthermore, the quantitative structure–activity relationship (QSAR) analysis is helpful to understand the reaction mechanism.
In this study, the geometrical parameters are optimized at the MPWB1K/6-31 + G(d, p) level. On the basis of the quantum chemical information, the rate constants are calculated using the multichannel Rice-Ramsperger-Kassel-Marcus (RRKM) theory and canonical variational transition state theory (CVT) with small-curvature tunneling (SCT) correction over a wide temperature range of 200-500 K. Then, the kinetics study has been performed to calculate the rate constants of elementary reactions, and the quantitative structure–activity relationship from perfluorooctanoic acid to trifluoroacetic acid with rate constants is analyzed in order to explore the main factors affecting the rate constant of decarboxylation reaction.

2. Results and Discussion

The main possible reaction paths of electrochemical degradation of PFOA are drawn in Figure 1. Figure 2 shows two reaction circles in the electrochemical degradation pathways. The chemical structures of transition states in electrochemical degradation reactions are shown in Figure S1.

2.1. Reaction Mechanism

The detailed electrochemical mineralization mechanism of PFOA has been analyzed in previous study [25]. The first step of electrochemical degradation is the electron transfer process from carboxylic acid to anode in which the intermediate IM1 is generated [24]. Then, perfluoroheptyl radical IM2 and CO2 are produced from IM1 via the transition state TS1, which is a decarboxylation reaction. In the subsequent reactions, IM2 may continue to react with OH, O2 and H2O in the electrolytic cell. Channel A shows the reactions initiated by OH. The addition reaction of IM2 with OH generates adduct IM3, which is a barrierless process. Perfluoroheptanoyl fluoride IM4 is formed through a HF desorption process in the four-membered ring of IM3 via the transition state TS2. Obviously, IM4 can be hydrolyzed to perfluoroheptanoic acid (PFHA) P1 and hydrofluoric acid HF. What is more, IM4 also can continue to react with OH to generate adduct IM5 via the transition state TS3. Then, the fluorine atom will be removed to form P1 via the transition state TS4. A HF desorption process in the four-membered ring of IM5 can also occur to generate PFHA radical P2 via the transition state TS5. Obviously, the HF desorption process of IM3 is challenged due to its high potential barrier of 53.43 kcal·mol−1. An easier way lies in the hydrogen atom of the IM3 being abstracted by OH to form intermediate IM6 via the transition state TS6, and the potential barrier is 6.62 kcal·mol−1. Then, IM6 can remove a fluorine atom to produce the IM4 via the transition state TS7, or be decomposed into perfluorohexyl radical IM7 and difluorophosgene via the transition state TS8. The above reaction barriers are 33.02 and 8.32 kcal·mol−1, respectively. Difluorophosgene can be hydrolyzed to the final products HF and CO2. Obviously, the latter path occurs much more easily thermodynamically.
Channel B depicts the reactions initiated by oxygen. IM2 reacts with O2 to generate adduct IM8, which is a barrierless reaction. The oxygen atom of IM8 can be abstracted by OH and O2 to form IM9 and IM6 via the transition state TS9 and TS10, respectively. IM9 can also be decomposed into IM6 and OH, but considering the high potential barrier of TS9, it is a difficult reaction.
The reaction of IM2 and H2O is shown in Channel C. The hydrogen atom of H2O is abstracted by IM2 and IM10 is generated via the transition state TS11. The potential barrier and endothermic energy are 13.23 and 3.62 kcal·mol−1, respectively.
As shown in Figure 2, the electrochemical degradation of PFOA has three circles. The first one is the degradation from PFOA radical to PFHpA radical, the subsequent degradation process is from PFHpA to PFHxA radical, until the perfluorinated acetic acid finally decomposes into CO2 and HF. The second one is the degradation from PFOA to PFHpA, and the final products are same as for the first one. The last one is the degradation from C7F15 radical to C6F13 radical, and C6F13 radical to C5F11 radical, until it decomposes to CF3 radical, which represents complete degradation.
Ijms 15 14153 g001 1024
Figure 1. The main possible reaction paths of electrochemical degradation of PFOA embedded with the potential barriers ΔEb (kcal·mol−1) and reaction heats ΔEr (kcal·mol−1).
Figure 1. The main possible reaction paths of electrochemical degradation of PFOA embedded with the potential barriers ΔEb (kcal·mol−1) and reaction heats ΔEr (kcal·mol−1).
Ijms 15 14153 g001
Ijms 15 14153 g002 1024
Figure 2. The three reaction circles in the electrochemical degradation pathways.
Figure 2. The three reaction circles in the electrochemical degradation pathways.
Ijms 15 14153 g002

2.2. Rate Constants

The RRKM, TST and the CVT with SCT correction method are used to calculate the rate constants. The RRKM method is employed to calculate the rate constants of elementary reactions without barriers, such as elementary reactions (3), (11), and (13). Except for the above elementary reactions of PFOA, the rate constants of decarboxylation reactions of PFCAs radicals (~C8–C2) are also calculated for PFOA when it is degraded successfully after decarboxylation reaction in experiments [25,26]. The rate constants of elementary reactions (2)–(15) and decarboxylation reactions (~C8–C2) at the temperature range of 200–500 K are reported and listed in Table 1 and Table 2, respectively. The reaction pressure is adopted at atmospheric pressure.
Table
Table 1. The rate constants of decarboxylation reactions (~C8–C2) at the temperature range of 200–500 K (CVT/SCT).
Table 1. The rate constants of decarboxylation reactions (~C8–C2) at the temperature range of 200–500 K (CVT/SCT).
T (K)k8C-7C ak7C-6C ak6C-5Cak5C-4Cak4C-3Cak3C-2Cak2C-1Ca
2001.13 × 10124.21 × 10101.34 × 10112.05 × 10113.81 × 10115.17 × 10117.62 × 1011
2201.26 × 10125.01 × 10101.54 × 10112.50 × 10114.25 × 10115.22 × 10117.58 × 1011
2401.39 × 10125.80 × 10101.74 × 10112.95 × 10114.67 × 10115.28 × 10117.55 × 1011
2601.52 × 10126.57 × 10101.92 × 10113.40 × 10115.07 × 10115.33 × 10117.17 × 1011
2801.64 × 10127.32 × 10102.10 × 10113.85 × 10115.45 × 10115.38 × 10117.19 × 1011
298.151.75 × 10127.97 × 10102.25 × 10114.25 × 10115.79 × 10115.42 × 10117.21 × 1011
3201.87 × 10128.72 × 10102.43 × 10114.73 × 10116.17 × 10115.48 × 10117.25 × 1011
3401.97 × 10129.38 × 10102.58 × 10115.16 × 10116.50 × 10115.52 × 10117.29 × 1011
3602.08 × 10121.00 × 10112.73 × 10115.58 × 10116.82 × 10115.56 × 10117.33 × 1011
3802.17 × 10121.06 × 10112.86 × 10115.98 × 10117.13 × 10115.60 × 10117.37 × 1011
4002.27 × 10121.12 × 10112.99 × 10116.38 × 10117.42 × 10115.64 × 10117.41 × 1011
4502.48 × 10121.25 × 10113.29 × 10117.32 × 10113.19 × 10115.73 × 10117.52 × 1011
5002.68 × 10121.36 × 10113.55 × 10118.19 × 10113.38 × 10115.82 × 10117.82 × 1011
a The unit of the rate constant is s−1.
Table
Table 2. The rate constants of elementary reactions (2)–(15) at the temperature range of 200–500 K (CVT/SCT).
Table 2. The rate constants of elementary reactions (2)–(15) at the temperature range of 200–500 K (CVT/SCT).
T (K)k(2) ak(3) bk(4) ak(5) bk(6) ak(7) ak(8) bk(9) ak(10) ak(11) bk(12) bk(13) ak(14) bk(15) b
2001.13 × 10123.21 × 10−122.80 × 10−421.06 × 10−221.64 × 10−201.54 × 10−321.15 × 10−244.21 × 10−249.70 × 1069.92 × 10−181.21 × 10−584.75 × 10−212.60 × 10−703.73 × 10−38
2201.26 × 10124.33 × 10−121.98 × 10−373.71 × 10−224.83 × 10−181.18 × 10−286.70 × 10−247.50 × 10−212.99 × 1071.75 × 10−173.50 × 10−561.91 × 10−201.80 × 10−653.63 × 10−36
2401.39 × 10125.58 × 10−122.18 × 10−331.06 × 10−211.12 × 10−152.04 × 10−252.96 × 10−233.86 × 10−187.70 × 1072.82 × 10−173.85 × 10−546.17 × 10−201.98 × 10−611.67 × 10−34
2601.52 × 10126.93 × 10−125.74 × 10−302.59 × 10−211.13 × 10−131.12 × 10−221.05 × 10−227.59 × 10−161.72 × 1084.28 × 10−172.03 × 10−521.69 × 10−195.27 × 10−584.33 × 10−33
2801.64 × 10128.35 × 10−124.90 × 10−275.62 × 10−215.89 × 10−122.49 × 10−203.14 × 10−227.02 × 10−143.46 × 1086.18 × 10−176.00 × 10−514.07 × 10−194.59 × 10−557.15 × 10−32
298.151.75 × 10129.69 × 10−121.02 × 10−241.04 × 10−201.35 × 10−101.79 × 10−187.52 × 10−222.52 × 10−126.01 × 1088.28 × 10−178.70 × 10−508.24 × 10−199.84 × 10−536.64 × 10−31
3201.87 × 10121.13 × 10−112.84 × 10−222.01 × 10−203.63 × 10−91.62 × 10−161.91 × 10−211.09 × 10−101.08 × 1091.14 × 10−161.45 × 10−481.75 × 10−182.84 × 10−507.02 × 10−30
3401.97 × 10121.29 × 10−115.84 × 10−203.44 × 10−205.12 × 10−86.01 × 10−154.05 × 10−212.24 × 10−91.73 × 1091.48 × 10−161.37 × 10−473.25 × 10−182.70 × 10−484.71 × 10−29
3602.08 × 10121.44 × 10−113.25 × 10−185.56 × 10−205.37 × 10−71.49 × 10−137.95 × 10−213.29 × 10−82.65 × 1091.87 × 10−161.01 × 10−465.66 × 10−181.56 × 10−462.58 × 10−28
3802.17 × 10121.60 × 10−111.18 × 10−168.60 × 10−204.40 × 10−62.64 × 10−121.46 × 10−203.62 × 10−73.87 × 1092.32 × 10−166.05 × 10−469.40 × 10−185.93 × 10−451.19 × 10−27
4002.27 × 10121.75 × 10−113.01 × 10−151.06 × 10−192.93 × 10−53.50 × 10−112.55 × 10−203.14 × 10−65.45 × 1092.84 × 10−163.01 × 10−451.49 × 10−171.57 × 10−434.77 × 10−27
4502.48 × 10122.05 × 10−112.79 × 10−122.44 × 10−191.59 × 10−38.21 × 10−98.39 × 10−202.95 × 10−41.13 × 10104.42 × 10−168.93 × 10−444.08 × 10−171.64 × 10−409.11 × 10−26
5002.68 × 10122.30 × 10−116.58 × 10−104.89 × 10−193.87 × 10−26.45 × 10−72.23 × 10−191.15 × 10−22.03 × 10106.42 × 10−161.35 × 10−429.42 × 10−174.37 × 10−381.00 × 10−24
a The unit of the rate constant is s−1; b The unit of the rate constant is cm3·molecule−1·s−1.
For the purpose of comparison, the elementary reaction (2) is taken as an example, and the TST rate constants, the CVT rate constants with the ZCT and the SCT correction are listed in Table S1. The TST rate constant at 200 K is 3.10 × 1014 s−l, which is 284 times that of the CVT rate constant at the same temperature, 1.09 × 1012 s−l. It suggests that the variational effect is significant in this reaction, and the higher the temperature is, the weaker the variational effect is. What is more, the tunneling effects are also taken into account to compute the rate constants. It is clear that the CVT constants have no significant difference with the CVT/ZCT and CVT/SCT ones over the temperature range of 200–500 K. It can be seen that the tunneling effect plays a less important role in this rate constant calculation. According to the previous study, the CVT/SCT rate constants are in good agreement with experimental values in a large temperature range [27,28,29]. Then, the results of the CVT/SCT method at 298.15 K are chosen for discussion in this paper. The TST, CVT with the ZCT or the SCT correction rate constants of the main elementary reactions (3)–(15) are listed in Tables S2–S11. Due to the absence of the available experimental values, it is difficult to make a direct comparison of the calculated CVT/SCT rate constants with the experimental values for all the elementary reactions. We hope that our CVT/SCT calculations may provide a good estimation.
The rate constant of the decarboxylation reaction (2) is 1.75 × 1012 s−l. Obviously, the decarboxylation reaction occurs quite easily after the electron transfer process due to the low potential barrier. The rate constants of elementary reactions (3), (11) and (15) are 9.69 × 10−12, 8.28 × 10−17 and 6.64 × 10−31 cm3·molecule−1·s−1, respectively, which means that Channel A is the main reaction pathway since the rate constant is much higher than those of the other two pathways. As Figure 1 shows, the subsequent reactions of the IM3 in Channel A are divided into two paths, (4) and (8), the rate constant of reaction (4) is 1.02 × 10−24 s−1, while the rate constant of reaction (8) is 7.52 × 10−22 cm3·molecule−1·s−1. The latter is 737 times higher than that of the reaction (4), so the IM3 is easier to react with OH to form the IM6. Then, reaction (10) occurs readily with the rate constant of 6.01 × 108 s−1, which demonstrates that the circle of CnF2n+1 → Cn−1F2n−1 is the optimal reaction pathway in the degradation process due to the high rate constants. The circle of CnF2n+1COO → Cn−1F2n−1COO is achieved by the reaction (5) and (7), and the rate constants are 1.04 × 10−20 cm3·molecule−1·s−1 and 1.79 × 10−18 s−1, respectively. In Channel B, the rate constants of the abstraction reactions (12) and (14) are 8.70 × 10−50 and 9.84 × 10−53 cm3·molecule−1·s−1, which are difficult to achieve because the reaction barrier is high.
For the rate constants over the temperature range of 200–500 K, the Arrhenius equations, i.e., k(T) = A exp(−Ea/RT), are shown in Table 3. The pre-exponential factor and the activation energy can be obtained from Arrhenius equations. The correlative coefficient R2 is above 0.997.
Table
Table 3. The Arrhenius equations for the rate constants k(2)–k(15) over the temperature range of 200–500 K.
Table 3. The Arrhenius equations for the rate constants k(2)–k(15) over the temperature range of 200–500 K.
ReactionAEa (kJ/mol)Arrhenius EquationR2
(2)4.66 × 10122398.76k = 4.66 × 1012 exp(−288.52/T)0.997
(3)9.13 × 10−115564.81k = 9.13 × 10−11 exp(−669.33/T)0.999
(4)3.22 × 1012207,450.90k = 3.22 × 1012 exp(−24,952/T)0.999
(5)1.30 × 10−1623,352.36k = 1.30 × 10−16 exp(−2808.80/T)0.999
(6)8.05 × 1010118,399.70k = 8.05 × 1010 exp(−14,241/T)0.999
(7)7.89 × 1010163,511.40k = 7.89 × 1010 exp(−19,667/T)0.999
(8)6.35 × 10−1633,661.72k = 6.35 × 10−16 exp(−4048.80/T)0.999
(9)2.30 × 1012136,798.60k = 2.30 × 1012 exp(−16,454/T)0.999
(10)3.18 × 101221,194.88k = 3.18 × 1012 exp(−2549.30/T)0.999
(11)8.98 × 10−1511,478.31k = 8.98 × 10−15 exp(−1380.6/T)0.998
(12)7.04 × 10−32102,328.70k = 7.04 × 10−32 exp(−12,308/T)0.998
(13)5.49 × 10−1427,305.67k = 5.49 × 10−14 exp(−3284.3/T)0.999
(14)1.09 × 10−16205,547.02k = 1.09 × 10−16 exp(−24,723/T)0.999
(15)7.12 × 10−1685,567.69k = 7.12 × 10−16 exp(−10,292/T)0.999
k(T) = A exp(−Ea/RT), where A, pre-exponential factor; Ea, activation energy; R, ideal gas constant (R = 8.314); T, temperature (K); R2, correlation coefficient.

2.3. QSAR Models

The quantitative structure–activity relationship is performed to reveal the relationship between the structures of PFCAs radicals (~C8–C2) and the rate constants of decarboxylation reactions at 298.15 K. The atom number of PFCAs (2 ≤ n ≤ 7) radicals is drawn in Figure 3. The obtained parameters, such as the bond length, molecular mass, volume, dipole, bond energy and net atomic charge, are listed in Table 4. Table 5 shows the actual values, predicted values and residual values of the model. The comparison of actual values and predicted ones is shown in Figure S2.
Ijms 15 14153 g003 1024
Figure 3. The atom number of PFCAs (2 ≤ n ≤ 7) radicals.
Figure 3. The atom number of PFCAs (2 ≤ n ≤ 7) radicals.
Ijms 15 14153 g003
The independent variables are chosen as follows: the bond length of C1–C2 (RC1–C2), the molecular mass (M), the volume (V), the difference ΔE between EHOMO and ELUMO, the dipole of molecule, the bond energy of C1–C2 (EC1–C2), the net atomic charges on atom C1 and C2 (QC1 and QC2). The dependent variable is the logarithmic form of rate constants (log k). Then, the genetic function approximation (GFA) is adopted to filter the optimum combination of parameters. A five-parameter combination is obtained, which includes sufficient information and high reliability in predicting the rate constants of decarboxylation reactions. The structure–activity model obtained from the GFA calculation is given as follows:
log k = 361.39 × RC1–C2 − 0.03 × V − 2.87 × ΔE + 0.22 × EC1–C2 − 27.73 × QC2 − 534.97
The correlative coefficient R2 and the cross validated R2 (CV) are above 0.999. It can be seen from Table 5 that the relativity between actual and predicted values is excellent.
As the structure–activity model shows, RC1–C2 and EC1–C2 are positively correlated to rate constants, while V, ΔE and QC2 are negatively correlated. It is obvious that the rate constant is largely affected by RC1–C2 due to the high factor. When the bond of RC1–C2 is elongated, the stereo effect is reduced, and the rate constant is increased correspondingly.
Table
Table 4. The parameters used to make quantitative structure–activity relationship analysis.
Table 4. The parameters used to make quantitative structure–activity relationship analysis.
PFCAs RadicalsRC1–C2 (Å)MV (cm3/mol)ΔE (a.u.)Dipole (Debye)EC1–C2 (kcal/mol)QC1 (C)QC2 (C)
C7F15COO1.5433412.9659166.9720.36341.159424.120.6590.365
C6F13COO1.5386362.9691152.6340.36161.138224.220.6630.367
C5F11COO1.5388312.9723141.1610.27281.243624.150.6000.374
C4F9COO1.5441262.9755118.2180.25331.209424.100.4550.457
C3F7COO1.5394212.978783.1140.36651.201924.260.4720.417
C2F5COO1.5380162.981962.9980.28631.135223.030.3220.418
CF3COO1.5392112.985046.7590.28941.182819.690.9360.419
Table
Table 5. The actual values, predicted values and residual values of the model (T = 298.15 K).
Table 5. The actual values, predicted values and residual values of the model (T = 298.15 K).
PFCAs RadicalsLg k (Actual Values)Lg k (Predicted Values)Residual Values
C7F15COO12.243012.2439−8.34 × 10−4
C6F13COO10.901510.9029−1.42 × 10−3
C5F11COO11.352211.34972.43 × 10−3
C4F9COO11.628411.6286−2.02 × 10−4
C3F7COO11.762711.76032.43 × 10−3
C2F5COO11.734011.7373−3.28 × 10−3
CF3COO11.857911.85718.72 × 10−4

3. Experimental Section

3.1. Geometry Optimization

The geometrical parameters of reactants, transition states, intermediates, and products are optimized at the MPWB1K/6-31 + G(d, p) level. The vibrational frequencies have been calculated at the same level in order to determine the nature of stationary points. The MPWB1K method is a hybrid density functional theory (HDFT) model developed by Truhlar et al. Study shows that MPWB1K gives the best results for a combination of thermochemistry, thermochemical kinetics, hydrogen bonding and weak interactions, especially for thermochemical kinetics and non-covalent interaction [30]. Compared with other conventional methods such as B3LYP and MP2, MPWB1K is more accurate and less time-consuming. The 6-31+G(d, p) basis sets are chosen to perform the geometry optimization and the 6-311 + G(3df, 2p) basis sets are adopted to calculate the potential energy for this medium-scale system after overall consideration of the computational accuracy and time cost [31,32]. Each transition state is verified to connect the specific reactants with products by performing an intrinsic reaction coordinate (IRC) analysis. All the calculations are performed using the GAUSSIAN 03 programs [33]. In this study, TS, IM and P represent the transition state, the intermediate and product, respectively.

3.2. Kinetic Calculation

Among the minimum energy path, about 40 points near the transition state are selected to perform the vibrational frequency calculation, 20 points on the reactant side and 20 points on the product side which should represent the shape of the minimum energy path. Based on the information from ab initio calculations, including coordinates, gradients, and force constants or Hessian matrix, the rate constants with tunneling effects are calculated by the POLYRATE 9.7 program [34]. The canonical variational transition state theory (CVT) with small-curvature tunneling (SCT) effect correction is a useful method to calculate rate constants [35], which has been successfully applied to lots of research [36,37,38]. The Rice-Ramsperger-Kassel-Marcus (RRKM) theory is used to calculate the rate constants of the reactions that have no transition states. Study shows that the rate constants of barrierless reactions calculated by RRKM are in good agreement with the experimental observation [39].

3.3. QSAR Analysis

The genetic function approximation (GFA) [40] in the Materials studio package is adopted to describe the relationship between the rate constants of decarboxylation reactions and the structures of PFCAs radicals (~C8–C2). The parameters obtained from geometry optimization and frequency calculation, such as the bond length, molecular mass, volume, atomic net charge and frontier orbital energies (EHOMO, ELUMO, ΔE), are chosen as the independent variables. The dependent variable is the logarithmic form of rate constants, log k. The optimal parameter combination can be obtained after analysis.

4. Conclusions

In this paper, the rate constants of electrochemical reactions are calculated using RRKM theory and the CVT with SCT correction. The structure–activity relationship is analyzed in order to find out the relationship between the structures of PFCA radicals (~C2–C8) and the rate constants of decarboxylation reactions.
(1) The circle of CnF2n+1 → Cn−1F2n−1 is the optimal reaction pathway in the degradation process due to the high rate constants.
(2) The quantitative structure–activity relationship is investigated using the GFA method. The structure–activity model has been constructed: RC1–C2 and EC1–C2 are positively correlated to the rate constants of decarboxylation reactions.

Supplementary Files

Supplementary File 1

Acknowledgments

This work is supported by National Natural Science Foundation of China (No. 21277082, 21337001), Marie Curie International Research Staff Exchange Scheme Fellowship within the 7th European Community Framework Programme (No. 295132), Taishan Scholar Grant (ts20120552), National High Technology Research and Development Program 863 (No. 2012AA06A301), Program for New Century Excellent Talents in University(NCET-13-0349) and Open Project from special fund of State Key Joint Laboratory of Environment Simulation and Pollution Control (No. 13K05ESPCP).

Author Contributions

C.G. and X.S. designed and performed the mechanism calculations, then wrote the manuscript; C.Z. and X.Z. analyzed the data; J.N. was responsible for experimental verification. All authors provided final approval of the manuscript.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Hori, H.; Hayakawa, E.; Einaga, H.; Kutsuna, S.; Koike, K.; Ibusuki, T.; Kiatagawa, H.; Arakawa, R. Decomposition of environmentally persistent perfluorooctanoic acid in water by photochemical approaches. Environ. Sci. Technol. 2004, 38, 6118–6124. [Google Scholar] [CrossRef]
  2. Renner, R. Growing concern over perfluorinated chemicals. Environ. Sci. Technol. 2001, 35, 154A–160A. [Google Scholar] [CrossRef]
  3. Giesy, J.P.; Kannan, K. Global distribution of perfluorooctane sulfonate in wildlife. Environ. Sci. Technol. 2001, 35, 1339–1342. [Google Scholar] [CrossRef]
  4. Schultz, M.M.; Barofsky, D.F.; Field, J.A. Fluorinated alkyl surfactants. Environ. Eng. Sci. 2003, 20, 487–501. [Google Scholar] [CrossRef]
  5. DeWitt, J.C.; Copeland, C.B.; Strynar, M.J.; Luebke, R.W. Perfluorooctanoic acid-induced immunomodulation in adult C57BL/6J or C57BL/6N female mice. Environ. Health Perspect. 2008, 116, 644–650. [Google Scholar] [CrossRef]
  6. Lin, H.; Niu, J.; Ding, S.; Zhang, L. Electrochemical degradation of perfluorooctanoic acid (PFOA) by Ti/SnO2-Sb, Ti/SnO2-Sb/PbO2 and Ti/SnO2-Sb/MnO2 anodes. Water Res. 2012, 46, 2281–2289. [Google Scholar] [CrossRef]
  7. Loganathan, B.G.; Sajwan, K.S.; Sinclair, E.; Senthil Kumar, K.; Kannan, K. Perfluoroalkyl sulfonates and perfluorocarboxylates in two wastewater treatment facilities in Kentucky and Georgia. Water Res. 2007, 41, 4611–4620. [Google Scholar] [CrossRef]
  8. Guo, R.; Sim, W.J.; Lee, E.S.; Lee, J.H.; Oh, J.E. Evaluation of the fate of perfluoroalkyl compounds in wastewater treatment plants. Water Res. 2010, 44, 3476–3486. [Google Scholar] [CrossRef]
  9. Loos, R.; Locoro, G.; Comero, S.; Contini, S.; Schwesig, D.; Werres, F.; Balsaa, P.; Gans, O.; Weiss, S.; Blaha, L.; et al. Pan-European survey on the occurrence of selected polar organic persistent pollutants in ground water. Water Res. 2010, 44, 4115–4126. [Google Scholar] [CrossRef]
  10. Falandysz, J.; Taniyasu, S.; Gulkowska, A.; Yamashita, N.; Schulte-Oehlmann, U. Is fish a major source of fluorinated surfactants and repellents in humans living on the Baltic Coast? Environ. Sci. Technol. 2006, 40, 748–751. [Google Scholar] [CrossRef]
  11. Keller, J.M.; Kannan, K.; Taniyasu, S.; Yamashita, N.; Day, R.D.; Arendt, M.D.; Segars, A.L.; Kucklick, J.R. Perfluorinated compounds in the plasma of loggerhead and Kemp’s ridley sea turtles from the southeastern coast of the United States. Environ. Sci. Technol. 2005, 39, 9101–9108. [Google Scholar] [CrossRef]
  12. Moriwaki, H.; Takagi, Y.; Tanaka, M.; Tsuruho, K.; Okitsu, K.; Maeda, Y. Sonochemical decomposition of perfluorooctane sulfonate and perfluorooctanoic acid. Environ. Sci. Technol. 2005, 39, 3388–3392. [Google Scholar] [CrossRef]
  13. Berthiaume, J.; Wallace, K.B. Perfluorooctanoate, perflourooctanesulfonate, and N-ethyl perfluorooctanesulfonamido ethanol; peroxisome proliferation and mitochondrial biogenesis. Toxicol. Lett. 2002, 129, 23–32. [Google Scholar] [CrossRef]
  14. Olsen, G.W.; Logan, P.W.; Hansen, K.J.; Simpson, C.A.; Burris, J.M.; Burlew, M.M.; Vorarath, P.P.; Venkateswarlu, P.; Schumpert, J.C.; Mandel, J.H. An occupational exposure assessment of a perfluorooctanesulfonyl fluoride production site: Biomonitoring. AIHA J. 2003, 64, 651–659. [Google Scholar] [CrossRef]
  15. Sanderson, H.; Boudreau, T.M.; Mabury, S.A.; Cheong, W.J.; Solomon, K.R. Ecological impact and environmental fate of perfluorooctane sulfonate on the zooplankton community in indoor microcosms. Environ. Toxicol. Chem. 2002, 21, 1490–1496. [Google Scholar] [CrossRef]
  16. Hines, E.P.; White, S.S.; Stanko, J.P.; Gibbs-Flournoy, E.A.; Lau, C.; Fenton, S.E. Phenotypic dichotomy following developmental exposure to perfluorooctanoic acid (PFOA) in female CD-1 mice: Low doses induce elevated serum leptin and insulin, and overweight in mid-life. Mol. Cell. Endocrinol. 2009, 304, 97–105. [Google Scholar] [CrossRef]
  17. Kennedy, G.L.; Butenhoff, J.L.; Olsen, G.W.; O’Connor, J.C.; Seacat, A.M.; Perkins, R.G.; Biegel, L.B.; Murphy, S.R.; Farrar, D.G. The toxicology of perfluorooctanoate. Crit. Rev. Toxicol. 2004, 34, 351–384. [Google Scholar] [CrossRef]
  18. Lau, C.; Anitole, K.; Hodes, C.; Lai, D.; Pfahles-Hutchens, A.; Seed, J. Perfluoroalkyl acids: A review of monitoring and toxicological findings. J. Toxicol. Sci. 2007, 99, 366–394. [Google Scholar] [CrossRef]
  19. US EPA. Revised Draft Hazard Assessment of Perfluorooctanoic Acid and Its Salts. Available online: http://www.fluoridealert.org/wp-content/pesticides/pfoa.epa.nov.4.2002.pdf (accessed on 4 November 2002).
  20. Järnberg, U.; Holmström, K.; van Bavel, B. Perfluoroalkylated Acids and Related Compounds (PFAS) in the Swedish Environment-Chemistry. Available online: http://www.diva-portal.org/smash/get/diva2:657980/FULLTEXT01.pdf (accessed on 1 May 2007).
  21. Hoffman, K.; Webster, T.F.; Bartell, S.M.; Weisskopf, M.G.; Fletcher, T.; Vieira, V.M. Private drinking water wells as a source of exposure to perfluorooctanoic acid (PFOA) in communities surrounding a fluoropolymer production facility. Environ. Health Perspect. 2011, 119, 92–97. [Google Scholar]
  22. Ochiai, T.; Moriyama, H.; Nakata, K.; Murakami, T.; Koide, Y.; Fujishima, A. Electrochemical and photocatalytic decomposition of perfluorooctanoic acid with a hybrid reactor using a boron-doped diamond electrode and TiO2 photocatalyst. Chem. Lett. 2011, 40, 682–683. [Google Scholar] [CrossRef]
  23. Zhuo, Q.; Deng, S.; Yang, B.; Huang, J.; Yu, G. Efficient electrochemical oxidation of perfluorooctanoate using a Ti/SnO2-Sb-Bi anode. Environ. Sci. Technol. 2011, 45, 2973–2979. [Google Scholar] [CrossRef]
  24. Ochiai, T.; Lizuka, Y.; Nakata, K.; Murakami, T.; Tryk, D.A.; Fujishima, A.; Koide, Y.; Morito, Y. Efficient electrochemical decomposition of perfluorocarboxylic acids by the use of a boron-doped diamond electrode. Diam. Relat. Mater. 2011, 20, 64–67. [Google Scholar] [CrossRef]
  25. Niu, J.; Lin, H.; Gong, C.; Sun, X. Theoretical and experimental insights into the electrochemical mineralization mechanism of perfluorooctanoic acid. Environ. Sci. Technol. 2013, 47, 14341–14349. [Google Scholar] [CrossRef]
  26. Niu, J.; Lin, H.; Xu, J.; Wu, H.; Li, Y. Electrochemical mineralization of perfluorocarboxylic acids (PFCAs) by Ce-doped modified porous nanocrystalline PbO2 film electrode. Environ. Sci. Technol. 2012, 46, 10191–10198. [Google Scholar]
  27. Zhang, Q.; Zhang, R.; Gu, Y. Kinetics and mechanism of O (3P) reaction with CH3CHF2: A theoretical study. J. Phys. Chem. A 2004, 108, 1064–1068. [Google Scholar]
  28. Zhang, Q.; Zhang, R.; Chan, K.; Bello, I. Ab initio and variational transition state approach to β-C3N4 formation: Kinetics for the reaction of CH3NH2 with H. J. Phys. Chem. A 2005, 109, 9112–9117. [Google Scholar]
  29. Sun, T.; Zhang, Q.; Qu, X.; Wang, W. Mechanism and direct dynamics studies for the reaction of monoethylsilane EtSiH3 with atomic O (3P). Chem. Phys. Lett. 2005, 407, 527–532. [Google Scholar]
  30. Zhao, Y.; Truhlar, D.G. Hybrid meta density functional theory methods for thermochemistry, thermochemical kinetics, and noncovalent interactions: The MPW1B95 and MPWB1K models and comparative assessments for hydrogen bonding and van der Waals interactions. J. Phys. Chem. A 2004, 108, 6908–6918. [Google Scholar]
  31. Hehre, W.; Ditchfield, R.; Pople, J. Theoretical investigations on the solvation process. J. Chem. Phys. 1972, 56, 2557–2562. [Google Scholar]
  32. Zheng, J.J.; Zhao, Y.; Truhlar, D.G. The DBH24/08 database and its use to assess electronic structure model chemistries for chemical reaction barrier heights. J. Chem. Theory Comput. 2009, 5, 808–821. [Google Scholar]
  33. Frisch, M.; Trucks, G.; Schlegel, H.; Scuseria, G.; Robb, M.; Cheeseman, J.; Zakrzewski, V.; Montgomery, J., Jr.; Stratmann, R.; Burant, J.; et al. Gaussian 03,Revision E.01. Available online: http://www.gaussian.com/g_misc/g03/citation_g03.htm (accessed on 11 June 2003).
  34. Corchado, J.C.; Chuang, Y.Y.; Fast, P.L.; Hu, W.P.; Liu, Y.P.; Lynch, G.C.; Nguyen, K.A.; Jackels, C.F.; Fernandez Ramos, A.; Ellingson, B.A.; et al. POLYRATE Version 9.7; University of Minnesota: Minneapolis, MN, USA, 2007. [Google Scholar]
  35. Miller, W.H. Tunneling corrections to unimolecular rate constants, with application to formaldehyde. J. Am. Chem. Soc. 1979, 101, 6810–6814. [Google Scholar] [CrossRef]
  36. Zhang, Q.; Yu, W.; Zhang, R.; Zhou, Q.; Gao, R.; Wang, W. Quantum chemical and kinetic study on dioxin formation from the 2, 4, 6-TCP and 2, 4-DCP precursors. Environ. Sci. Technol. 2010, 44, 3395–3403. [Google Scholar] [CrossRef]
  37. Qu, X.; Wang, H.; Zhang, Q.; Shi, X.; Xu, F.; Wang, W. Mechanistic and kinetic studies on the homogeneous gas-phase formation of PCDD/Fs from 2, 4, 5-trichlorophenol. Environ. Sci. Technol. 2009, 43, 4068–4075. [Google Scholar] [CrossRef]
  38. Gong, C.; Sun, X.; Zhang, C. The atmospheric chemical reaction of 4-tert-butylphenol initiated by OH radicals. Environ. Chem. 2013, 10, 111–119. [Google Scholar] [CrossRef]
  39. Hou, H.; Wang, B. Ab initio study of the reaction of propionyl (C2H5CO) radical with oxygen (O2). J. Chem. Phys. 2007, 127, 054306. [Google Scholar]
  40. Rogers, D.; Hopfinger, A.J. Application of genetic function approximation to quantitative structure-activity relationships and quantitative structure-property relationships. J. Chem. Inf. Comput. Sci. 1994, 34, 854–866. [Google Scholar]

Share and Cite

MDPI and ACS Style

Gong, C.; Sun, X.; Zhang, C.; Zhang, X.; Niu, J. Kinetics and Quantitative Structure—Activity Relationship Study on the Degradation Reaction from Perfluorooctanoic Acid to Trifluoroacetic Acid. Int. J. Mol. Sci. 2014, 15, 14153-14165. https://doi.org/10.3390/ijms150814153

AMA Style

Gong C, Sun X, Zhang C, Zhang X, Niu J. Kinetics and Quantitative Structure—Activity Relationship Study on the Degradation Reaction from Perfluorooctanoic Acid to Trifluoroacetic Acid. International Journal of Molecular Sciences. 2014; 15(8):14153-14165. https://doi.org/10.3390/ijms150814153

Chicago/Turabian Style

Gong, Chen, Xiaomin Sun, Chenxi Zhang, Xue Zhang, and Junfeng Niu. 2014. "Kinetics and Quantitative Structure—Activity Relationship Study on the Degradation Reaction from Perfluorooctanoic Acid to Trifluoroacetic Acid" International Journal of Molecular Sciences 15, no. 8: 14153-14165. https://doi.org/10.3390/ijms150814153

APA Style

Gong, C., Sun, X., Zhang, C., Zhang, X., & Niu, J. (2014). Kinetics and Quantitative Structure—Activity Relationship Study on the Degradation Reaction from Perfluorooctanoic Acid to Trifluoroacetic Acid. International Journal of Molecular Sciences, 15(8), 14153-14165. https://doi.org/10.3390/ijms150814153

Article Metrics

Back to TopTop