Non-Dimensional Groups, Film Thickness Equations and Correction Factors for Elastohydrodynamic Lubrication: A Review

Abstract

Apart from complex numerical models to predict the tribological behavior of elastohydrodynamically lubricated contacts, non-dimensional similarity groups and analytically solvable proximity equations can be used to estimate integral fluid film parameters. Based upon the pioneering work presented by Dowson and Higginson as well as Blok and Moes, these approaches have been continuously improved over the years by modifications or correction factors to capture different contact geometries (line-, point- or elliptical contacts) as well as to include fluid compression, thermal, non-Newtonian, starvation or roughness effects. Consequently, this review article aims at systematically reviewing these modifications/corrections and discussing their applicability as well as limitations before presenting some recommendations for future research activities.

1. Introduction

Power transmission in machine elements or engine components such as rolling bearings, gears or cam/followers is generally achieved by concentrated rolling and rolling-sliding contacts, in which the rubbing bodies are locally elastically deformed due to the acting contact forces [1]. For dry contacts, the involved deformations, pressures and stresses can be approximated by the Hertzian theory [2]. In the presence of sufficient lubricant, a hydrodynamic pressure is built up and the contacting surfaces are at least partially separated by a thin fluid film [3,4]. If the local elastic deformation and the lubricant film thickness are on a similar order of magnitude, this condition is usually referred to as elastohydrodynamic lubrication (EHL) or, thermo-elastohydrodynamic lubrication (TEHL), when considering thermal effects as well.

For numerical modeling, Computational Fluid Dynamics (CFD)- or Navier-Stokes-based approaches are generally associated with high computational effort and numerical instabilities [5]. Therefore, the simulation of EHL contacts is usually done by applying the Reynolds equation [6] for hydrodynamics and solving the system of equations coupled with elastic deformation. For this purpose, multigrid and multilevel integration methods based upon finite difference approaches and iterative (weak) coupling with an elastic half-space theory [7,8] or fully (strong) coupled finite element approaches [9,10] have been utilized. A schematic representation of an infinite EHL line-contact with common input variables is illustrated in Figure 1.

In addition to these rather complex and time-consuming simulation programs that allow for the investigation of local and time-resolved phenomena, there are also some analytically solvable proximity equations for the simplified, integral calculation of important EHL film parameters such as the minimum film height h_min or central lubricant gap h_c. These equations enable a fast and, in many cases, sufficiently accurate approximation as well as the incorporation into superordinate multi-body or system simulations for friction calculation or the identification of critical operating conditions of aggregates, machines or facilities. Most of these approaches, however, were established several decades ago and incrementally enhanced through modifications or correction factors by numerous authors based upon novel experimental/theoretical insights as well as advances in simulation and measurement technology. Therefore, this contribution aims to systematically present the evolution and most recent progress in film thickness estimation approaches, thus helping the interested reader to select the most suitable approach in terms of applicability and validity. For that purpose, this article follows a logical and chronological order. At first, non-dimensional groups are introduced in Section 2, which represent similarity indices for EHL contacts. Based upon that, the available proximity equations for the calculation of the minimum and central film thickness in infinite 2D line-, 3D point- or elliptical contacts are summarized in Section 3. 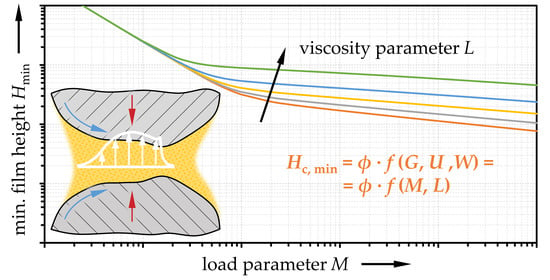Since these approaches may be subject to deviations under certain circumstances, correction factors for fluid compressibility (Section 4.1), thermal effects (4.2), non-Newtonian fluid behavior (4.3), starvation (4.4) as well as rough surfaces and asperity contact (4.5) are subsequently introduced. Finally, the applicability and limitations of the film thickness equations are discussed before presenting some recommendations for future research activities.

2. Non-Dimensional Groups

Based upon early numerical solutions [11], certain similarities were recognized and dimensionless groups were introduced to generalize calculations and results [12]. For an infinite 2D line-contact as well as a 3D point-contact, using the reduced Young’s modulus E’, the pressure-viscosity coefficient α_p, the base viscosity η₀, the effective velocity u_m, the effective radius R, the contact length l and the normal load F, Dowson and Higginson [13,14] defined the material parameter

$$G={\alpha }_{\mathrm{p}}·E^{\prime },$$

(1)

the velocity parameter

$$U=\frac{{\eta }_0·u_{\mathrm{m}}}{E^{\prime }·R_{\mathrm{x}}},$$

(2)

the load parameter

$$W_{2\mathrm{D}}=\frac{F}{l·E^{\prime }·R_{\mathrm{x}}},W_{3\mathrm{D}}=\frac{F}{E^{\prime }·R_{\mathrm{x}}^2},$$

(3)

as well as the fluid film parameters

$$H_{\mathrm{c}}=\frac{h_{\mathrm{c}}}{R_{\mathrm{x}}}, H_{\min }=\frac{h_{\min }}{R_{\mathrm{x}}}$$

(4)

and developed correlations between them (see Section 3). Typical ranges of these EHL parameters are summarized in

Table 1. Typical ranges for the dimensionless parameters in extension to [4,15].

Parameter	Typical Range
G	1500–6000
U	10−13–10−8
W	10−6–10−3
Hc	1.5 × 10−5–25 × 10−5
Hmin	10−5–2 × 10−4

.

Blok and Moes [16,17] showed that the four parameters of Dowson and Higginson can be unidirectionally transformed (i.e., re-transformation is not possible [1]) into three parameters thus introducing the modified load parameter

$$M_{2\mathrm{D}}{=W}_{2\mathrm{D}}·{\left(2·U\right)}^{-\frac{1}{2}}{,M}_{2\mathrm{D}}{=W}_{3\mathrm{D}}·{\left(2·U\right)}^{-\frac{3}{4}}$$

(5)

and the viscosity parameter

$$L=G·{\left(2·U\right)}^{\frac{1}{4}}$$

(6)

in addition to the lubricant film parameter

$$H_{\min }=h_{\min }·U^{-\frac{1}{2}}.$$

(7)

Another set of dimensionless groups were used by Greenwood [18], which can also be shortened and expressed in the Dowson & Higginson notation:

$$g_1=\frac{{\alpha }_{\mathrm{p}}·F^{\frac{2}{3}}}{{\left({\eta }_0·u_{\mathrm{m}}\right)}^{\frac{1}{2}}·R_{\mathrm{x}}}=G·W^{\frac{3}{2}}·U^{-1},$$

(8)

$$g_2={\alpha }_{\mathrm{p}}·{\left(\frac{F·E^{\prime }}{\pi ·R_{\mathrm{x}}}\right)}^{\frac{1}{2}}=0.4·G·W^{-1},$$

(9)

$$H^{*}=\frac{h·F}{{\eta }_0·u_{\mathrm{m}}·R_{\mathrm{x}}}=H·W·U^{-1}.$$

(10)

Later, Johnson [19] proposed parameters for the elasticity

$$P_{\mathrm{E}}={\left(\frac{F^2}{{\eta }_0·u_{\mathrm{m}}·l^2·R_{\mathrm{x}}}\right)}^{\frac{1}{2}},$$

(11)

the pressure-viscosity-relationship

$$P_{\mathsf{\alpha }}={\left(\frac{{\alpha }^2·F^3}{{\eta }_0·u_{\mathrm{m}}·l^3·R_{\mathrm{x}}^2}\right)}^{\frac{1}{2}},$$

(12)

and the film thickness

$$H^{*}=\frac{h_{\min }·F}{{\eta }_0·u_{\mathrm{m}}·l·R_{\mathrm{x}}},$$

(13)

which can be transformed to the Blok/Moes notation. For completeness, it should also be noted that Habchi et al. [20] suggested the use of the Weissenberg number Γ [21], which characterizes the shear stress of inlet flow in relation to the Newtonian limit by means of the relaxation time λ_Γ, the shear rate $\dot{\gamma }$, the shear stress τ and the shear modulus G [22]:

$$\Gamma ={\lambda }_{\Gamma }·\dot{\gamma }=\frac{\tau }{G}\approx \frac{{\eta }_0·u_{\mathrm{m}}}{h_{\mathrm{n}}·G}.$$

(14)

the Nahme-Griffith number [23], which describes the ratio of viscous heating to temperature-dependent viscosity changes using the temperature T and the thermal conductivity λ:

$$Na=\frac{-\frac{\partial \ln \left({\eta }_0\right)}{\partial T}·{\tau }^2·h^2}{\lambda ·{\eta }_0}=\frac{-\frac{\partial \ln \left({\eta }_0\right)}{\partial T}·{\eta }_0·{\left(u_1{-u}_2\right)}^2}{\lambda },$$

(15)

the limiting-shear-stress number with the limiting τ_l and unbound shear stress τ_u as well as the limiting stress-pressure coefficient λ_τ:

$$Li=\frac{{\tau }_{\mathrm{u}}}{{\tau }_{\mathrm{l}}}=\frac{{\tau }_{\mathrm{u}}}{{\lambda }_{\mathsf{\tau }}·p}$$

(16)

and the thermoviscous regime indicator

$$\mathit{Ti}=\frac{\mathit{Na}·\mathit{Wi}}{\mathit{Li}}$$

(17)

to distinguish between different friction regimes. These regimes were the linear (Wi < 1), non-linear viscous (Wi > 1, Li < 1, Ti < 100), plateau (Li > 2, Ti < 100) and thermoviscous (Ti > 100) traction regime. It is important to mention that the dimensionless parameters from Dowson/Higginson and Blok/Moes are the most frequently used in literature [15,24].

3. Film Thickness Equations

3.1. Line-Contacts (2D)

Dowson and Higginson [13,14] proposed an analytically solvable regression equation for the approximation of the minimum lubricant gap in an infinite 2D line-contact as a function of the dimensionless material, velocity and load parameters:

$$H_{\min }=f\left(G,U,W\right)\approx 1.6·G^{0.6}·U^{0.7}·W_{2\mathrm{D}}^{-0.13}.$$

(18)

It can be seen that the load has only a rather small influence, while the material and velocity parameters, especially the product of velocity and viscosity, strongly affect the film thickness. This equation has been modified by Moes [16]:

$$H_{\min }\approx 2.53·G^{0.55}·U^{0.7}·W_{2\mathrm{D}}^{-0.125},$$

(19)

whereas the parameters M and L can be utilized due to the transformability:

$$H_{\min }=f\left(M,L\right)\approx 1.56·M_{2\mathrm{D}}^{-0.125}·L^{0.55}.$$

(20)

Consequently, the relation between film height, load and viscosity is illustrated in Figure 2. The equations for the minimum lubricant gap H_min were further modified and extended based upon new insights and/or novel data from EHL simulations. For the 2D line-contact, the approaches from Dowson [25]:

$$H_{\min }\approx 2.65·G^{0.54}·U^{0.7}·W_{2\mathrm{D}}^{-0.13}$$

(21)

as well as Jacobson and Hamrock [26]:

$$H_{\min }\approx 3.07·G^{0.57}·U^{0.71}·W_{2\mathrm{D}}^{-0.11}$$

(22)

are prominent examples. An approximation for the central film thickness was introduced by Dowson and Toyoda [27]:

$$H_c\approx 3.06·G^{0.56}·U^{0.69}·W_{2\mathrm{D}}^{-0.1}.$$

(23)

It is important to emphasize that the aforementioned approaches represent only approximations with a limited validity (see blue-shaded area in Figure 2, which shows the domain of the Dowson-Higginson [14] equation). If the respective validity range does not hold true, e.g., below the Martin-Gümbel asymptote in Figure 2, lubricant film heights tend to be underestimated. Thus, Johnson [19] distinguished four regimes depending on the fluid (iso-/piezoviscous) and material (rigid/elastic) properties [1,15] (Figure 3). Therefore, proximity equations for the isoviscous/rigid (IR) or Martin-Gümbel regime with hydrodynamic lubrication (small M, L = 0):

$$H_{\mathrm{IR}}^{*}\approx 4.09\to H_{\min }\approx 2.45·M_{2\mathrm{D}}^{-1},$$

(24)

the isoviscous/elastic (IE) or Herrebrugh regime with easily deformable contacting bodies (soft EHL; large M and L = 0):

$$H_{\mathrm{IE}}^{*}\approx 3.1·P_{\mathrm{E}}^{0.8}\to H_{\min }\approx 2.05·M_{2\mathrm{D}}^{-1},$$

(25)

the piezoviscous/rigid (VR) or Blok regime (small M, large L):

$$H_{\mathrm{VR}}^{*}\approx 1.66·P_{\mathsf{\alpha }}^{0.667}\to H_{\min }\approx 1.05·L^{\frac{2}{3}},$$

(26)

and the piezoviscous/elastic (VE) or Dowson and Higginson regime with hard EHL contacts (large M, large L):

$$H_{\mathrm{VE}}^{*}\approx 2.53·P_{\mathrm{E}}^{0.55}·P_{\mathsf{\alpha }}^{0.55}\to H_{\min }\approx 1.56·M_{2\mathrm{D}}^{-0.125}·L^{0.55}$$

(27)

were defined [1].

Furthermore, Myers et al. [28] introduced a transition regime (TR in Figure 3), for which the following film thickness equations were proposed:

$$H_{\min }\approx \{\begin{array}{c}0383·P_{\mathrm{E}}^{-0.370}·P_{\mathsf{\alpha }}+4.465·P_{\mathrm{E}}^{0.667}\mathrm{for}1<P_{\mathrm{E}}\le 15\\ 0.853·P_{\mathrm{E}}^{-0.664}·P_{\mathsf{\alpha }}+3.410·P_{\mathrm{E}}^{0.770}\mathrm{for}15<P_{\mathrm{E}}\le 100\end{array}.$$

(28)

Based upon updated EHL simulations performed by Lubrecht [29] and Venner [30], Moes [31] presented a new approximation formula for the entire range of concentrated, lubricated 2D line-contacts (Figure 2), which utilizes asymptotic values for the lubricant film height:

$$H_{\min }\approx {\left\{{\left[{\left(0.99·M_{2\mathrm{D}}^{-0.125}·L^{0.75}·t\right)}^r+{\left(2.05·M_{2\mathrm{D}}^{-0.2}\right)}^r\right]}^{\frac{s}{r}}+{\left(2.45·M_{2\mathrm{D}}^{-1}\right)}^s\right\}}^{s^{-1}},$$

(29)

with

$${s=3-\mathrm{e}}^{-2·M_{2\mathrm{D}}^{-1}},$$

(30)

$${r=\mathrm{e}}^{1-\frac{4}{L+5}},$$

(31)

$${t=1-\mathrm{e}}^{-3.5·M_{2\mathrm{D}}^{-0.125}·{\mathrm{L}}^{-0.25}}.$$

(32)

Similar to this concept, Moes [32] suggested the following function fit for the central film height:

$$H_{\mathrm{c}}\approx {\left[{\left(H_{\mathrm{c},\mathrm{IR}}^{\frac{7}{3}}{+H}_{\mathrm{c},\mathrm{IE}}^{-\frac{7}{3}}\right)}^{\frac{3}{7}·s}+{\left(H_{\mathrm{c},\mathrm{VR}}^{-\frac{7}{2}}{+H}_{\mathrm{c},\mathrm{VE}}^{-\frac{7}{2}}\right)}^{-\frac{2}{7}·\mathrm{s}}\right]}^{{\mathrm{s}}^{-1}},$$

(33)

with

$$s=\frac{1}{5}·\left(7+8·{\mathrm{e}}^{-2·\frac{H_{\mathrm{c},\mathrm{IE}}}{H_{\mathrm{c},\mathrm{IR}}}}\right)$$

(34)

and the asymptotic approximations for the IR, IE, VR and VE regimes:

$$H_{\mathrm{c},\mathrm{IR}}\approx 3·M^{-1},$$

(35)

$$H_{\mathrm{c},\mathrm{IE}}\approx 2.62105·M^{-\frac{1}{5}},$$

(36)

$$H_{\mathrm{c},\mathrm{VR}}\approx 1.28666·L^{\frac{2}{3}},$$

(37)

$$H_{\mathrm{c},\mathrm{VE}}\approx 1.31106·M_{2\mathrm{D}}^{-\frac{1}{8}}·L^{\frac{3}{4}}.$$

(38)

3.2. Point- and Elliptical Contacts (3D)

Furthermore, a relation between the non-dimensional groups and the minimum film thickness for 3D point-contacts was suggested by Evans and Snidle [33]:

$$H_{\min }\approx 1.9·M_{3\mathrm{D}}^{-0.17}·L^{0.34}$$

(39)

as well as by Hamrock and Dowson [34] for elliptical contacts:

$$H_{\min }\approx 3.63·G^{0.49}·U^{0.67}·W_{3\mathrm{D}}^{-0.073}\left({1-\mathrm{e}}^{-0.68·k}\right)$$

(40)

with the ellipticity parameter

$$k=1.03·{\left(\frac{R_{\mathrm{y}}}{R_{\mathrm{x}}}\right)}^{0.64}.$$

(41)

and a flow velocity along the smaller half-axis. Chittenden et al. [35] extended this approach by varying flow direction and introduced a term considering the dependence on the ratio of the radii transversal to and in flow direction of the lubricant:

$$H_{\min }\approx 3.68·G^{0.49}·U^{0.68}·W_{3\mathrm{D}}^{-0.073}\left({1-\mathrm{e}}^{-0.67·{\left(\frac{R_{\mathrm{s}}}{R_{\mathrm{e}}}\right)}^{\frac{2}{3}}}\right),$$

(42)

with:

$$\frac{R_{\mathrm{s}}}{R_{\mathrm{e}}}=\frac{\frac{R_{\mathrm{y}}}{R_{\mathrm{x}}}·{\cos }^2{\xi +\sin }^2\xi }{{\cos }^2\xi +\frac{R_{\mathrm{y}}}{R_{\mathrm{x}}}·{\sin }^2\xi }.$$

(43)

Similar to the concept for 2D line-contacts from Moes [32], respective function fits for a 3D point-contact are given as:

$$H_{\min }\approx {\left\{{\left[{\left(1.7·M^{-\frac{1}{9}}·L^{\frac{3}{4}}·t\right)}^r+{\left(1.96·M^{-\frac{1}{9}}\right)}^r\right]}^{\frac{s}{r}}+{\left(47.3·M^{-2}\right)}^s\right\}}^{\frac{t}{s}},$$

(44)

with

$$s=12-10·{\mathrm{e}}^{{-M}^{-2}},$$

(45)

$${r=\mathrm{e}}^{1-\frac{6}{L+8}},$$

(46)

$${t=1-\mathrm{e}}^{-0.9·M^{\frac{1}{6}}·L^{-\frac{1}{6}}}.$$

(47)

For the central film thickness, respective formulas were suggested by Hamrock and Dowson [34]:

$$H_{\mathrm{c}}\approx 2.69·G^{0.53}·U^{0.67}·W_{3\mathrm{D}}^{-0.067}\left(1-0.61·{\mathrm{e}}^{-0.73·k}\right),$$

(48)

Chittenden et al. [35]:

$$H_{\mathrm{c}}\approx 4.31·G^{0.49}·U^{0.68}·W_{3\mathrm{D}}^{-0.073}\left({1-\mathrm{e}}^{-1.23·{\left(\frac{R_{\mathrm{s}}}{R_{\mathrm{e}}}\right)}^{\frac{2}{3}}}\right)$$

(49)

as well as Evans and Snidle [33]:

$$H_{\mathrm{c}}\approx 1.7·M_{3\mathrm{D}}^{-0.026}·L^{0.4}.$$

(50)

Furthermore, Nijenbanning et al. [36] suggested relations (Figure 4) for the central film thickness of 3D elliptical contacts:

$$H_{\mathrm{c}}\approx {\left\{{\left[H_{\mathrm{c},\mathrm{IR}}^{\frac{3}{2}}+{\left(H_{\mathrm{c},\mathrm{IE}}^{-4}+H_{00}^{-4}\right)}^{-\frac{3}{8}}\right]}^{\frac{2}{3}s}+{\left(H_{\mathrm{c},\mathrm{VR}}^{-8}+H_{\mathrm{c},\mathrm{VE}}^{-8}\right)}^{-\frac{s}{8}}\right\}}^{s^{-1}},$$

(51)

with

$$s=1.5·\left({1+\mathrm{e}}^{-1.2·\frac{H_{\mathrm{IE}}}{H_{\mathrm{IR}}}}\right),$$

(52)

$$H_{00}=1.8·{\left(\frac{R_{\mathrm{x}}}{R_{\mathrm{y}}}\right)}^{-1},$$

(53)

$$H_{\mathrm{c},\mathrm{IR}}\approx 145·{\left[1+0.796·{\left(\frac{R_{\mathrm{x}}}{R_{\mathrm{y}}}\right)}^{\frac{14}{15}}\right]}^{-\frac{15}{7}}·{\left(\frac{R_{\mathrm{x}}}{R_{\mathrm{y}}}\right)}^{-1}·M_{3\mathrm{D}}^{-2}$$

(54)

$$H_{\mathrm{c},\mathrm{IE}}\approx 3.18·{\left[1+0.006·\ln \left(\frac{R_{\mathrm{x}}}{R_{\mathrm{y}}}\right)+0.63·{\left(\frac{R_{\mathrm{x}}}{R_{\mathrm{y}}}\right)}^{\frac{4}{7}}\right]}^{-\frac{14}{25}}·{\left(\frac{R_{\mathrm{x}}}{R_{\mathrm{y}}}\right)}^{-\frac{1}{15}}·M_{3\mathrm{D}}^{-\frac{2}{15}},$$

(55)

$$H_{\mathrm{c},\mathrm{VR}}\approx 1.29·{\left[1+0.691·\left(\frac{R_{\mathrm{x}}}{R_{\mathrm{y}}}\right)\right]}^{-\frac{2}{3}}·L^{\frac{2}{3}},$$

(56)

$$H_{\mathrm{c},\mathrm{VE}}\approx 1.48·{\left[1+0.006·\ln \left(\frac{R_{\mathrm{x}}}{R_{\mathrm{y}}}\right)+0.63·{\left(\frac{R_{\mathrm{x}}}{R_{\mathrm{y}}}\right)}^{\frac{4}{7}}\right]}^{-\frac{7}{20}}·{\left(\frac{R_{\mathrm{x}}}{R_{\mathrm{y}}}\right)}^{-\frac{1}{24}}·M_{3\mathrm{D}}^{-\frac{1}{12}}·L^{\frac{3}{4}}.$$

(57)

Most of these approaches were developed based upon numerical data and give an almost constant ratio of central and minimum film thickness. However, this ratio may vary between 1 and 3 depending on M and L. Therefore, Sperka et al. [38] derived from experimental data an equation to predict this relation dependent on the pressure-viscosity coefficient α_p:

$$\frac{H_{\mathrm{c}}}{H_{\min }}=1+0.1·{\alpha }_{\mathrm{p}}^{0.128}·M_{3\mathrm{D}}^{0.38}{-M}_{3\mathrm{D}}^{\frac{1}{2}}·{\left[\frac{{\alpha }_{\mathrm{p}}^{0.2}·\ln \left(L\right)-3}{22.7}\right]}^2.$$

(58)

4. Correction Factors

The described non-dimensional groups and film thickness equations deliver reasonable agreement with many lubricants as experimentally verified via interferometric film thickness measurements [37,39]. However, deviations can be observed under certain conditions due to fluid compressibility, thermal effects, shear-thinning, insufficient lubricant supply or surface roughness. All these aspects are not fully considered in the original numerical solutions, based upon which the equations in Section 3 were derived. These effects can be taken into account using corrections factors ϕ_x, which are multiplied with the film height parameter to obtain the corrected gap height:

$$H_{\min ,\mathrm{cor}}={\varphi }_{\mathrm{x}}·H_{\min },$$

(59)

$$H_{\mathrm{c},\mathrm{cor}}={\varphi }_{\mathrm{x}}·H_{\mathrm{c}},$$

(60)

Since the different correction factors feature specific applicability and limitations, they will be addressed in more detail hereinafter.

4.1. Fluid Compressibility

In the original numerical solutions [14], the density-pressure-relationship according to Dowson and Higginson [40] was applied. Yet, the density was not considered as an input variable in the non-dimensional groups and film thickness equations. Although lubricant compressibility plays no major role in the minimum film formation, the central film thickness particularly can be influenced under certain conditions [41]. According to Venner and Bos [42] and later verified by Habchi and Bair [43], predictions for compressible fluids can be adjusted from the incompressible approximations with the base density ρ₀ using the following correction factor

$${\varphi }_{\mathrm{comp}}=\frac{{\rho }_0}{\rho \left(p_{\mathrm{H}}\right)},$$

(61)

whereby any model could be utilized for calculating the density for the respective Hertzian pressure ρ(p_H) [44].

4.2. Thermal Effects

The deduction of non-dimensional parameters and film thickness equations was based upon the isothermal solution of the EHL problem. However, for high speeds and velocity differences of the rubbing surfaces (slip), local temperatures in the fluid can significantly exceed the mass temperatures of the contacting bodies due to lubricant compression at the contact inlet and shearing in the contact center, thus reducing viscosity and, hence, film thickness [45]. To approximate these thermal aspects, a first theoretical approach to capture the effects coming from inlet compression and shearing was suggested by Greenwood and Kauzlarich [46]. The proposed correction factor was given as:

$${\varphi }_{\mathrm{th}}\approx {\left[1-0.24·\frac{n·{\eta }_0·u_{\mathrm{m}}^2}{\lambda ·\left(T_0{+T}_{\mathrm{s}}\right)}\right]}^{\frac{3}{4}},$$

(62)

using the constant T_s and exponent n from the Slotte equation [47] to describe the viscosity-temperature behavior as well as thermal conductivity λ of the given lubricant. However, this correlation tends to overestimate the influence of temperature at high speeds due to the application of the Crook approximation [48,49] of the film shape, which only holds true for a rolling speed of a few m/s [46]. Further, Murch and Wilson [50] introduced a thermal stress parameter, which was subsequently simplified by Jackson [51]:

$$Q=\frac{{\eta }_0·u_{\mathrm{m}}^2·\beta }{\lambda },$$

(63)

with the viscosity-temperature coefficient β to determine the thermal film thickness reduction:

$${\varphi }_{\mathrm{th}}\approx \frac{3.94}{3.74+Q^{0.66}}.$$

(64)

Since the fluid film height is mostly influenced by the viscosity at the contact inlet, thermal effects due to shearing in the center are less relevant. Therefore, these approaches were designed for pure rolling conditions. To extend their applicability, Wilson and Sheu [52] introduced a dependence on the slide-to-roll ratio (see Figure 5):

$${\varphi }_{\mathrm{th}}\approx {\left\{1+0.241·\left[1+14.8·{\left(\frac{SRR}{2}\right)}^{0.83}\right]·Q^{0.64}\right\}}^{-1},$$

(65)

which corresponds to the Murch and Wilson formulation for pure rolling conditions. These approaches were further modified by e.g., Pandey and Gosh [53]:

$${\varphi }_{\mathrm{th}}\approx {\left\{1+0.133·\left[1+5.65·{\left(\frac{SRR}{2}\right)}^{0.96}\right]·Q^{0.71}\right\}}^{-1},$$

(66)

adapted for specific lubricants [54] or modified for a combination with non-Newtonian fluid behavior [55].

4.3. Non-Newtonian Fluid Behavior

Similar to thermal effects, shear thinning of the lubricant at high speeds and velocity differences can also affect the minimum film height and change the film profile in the contact center [56]. Therefore, Bair [22] suggested a correction factor

$${\varphi }_{\mathrm{nn}}\approx {\left\{1+0.79·{\left[\left(1+SRR\right)·\Gamma \right]}^{\frac{1}{1+0.2·SRR}}\right\}}^{3.6·{\left(1-n\right)}^{1.7}}$$

(67)

for a lubricant with Carreau viscosity behavior [57] depending on the Carreau exponent n and the Weissenberg number. This approach was extended and distinguished for minimum and central lubrication film height by Khonsari et al. for line-contacts [58]

$${\varphi }_{\mathrm{nn},\min }\approx {\left\{1+0.83·{\left[\left(1+SRR\right)·\Gamma \right]}^{\frac{1}{1+0.24·SRR}}\right\}}^{3.4·{\left(1-n\right)}^{1.6}},$$

(68)

$${\varphi }_{\mathrm{nn},\mathrm{c}}\approx {\left\{1+0.75·{\left[\left(1+SRR\right)·\Gamma \right]}^{\frac{1}{1+0.22·SRR}}\right\}}^{3.1·{\left(1-n\right)}^{1.6}},$$

(69)

and for point-contacts [59]

$${\varphi }_{\mathrm{nn},\min }\approx {\left(1+\Gamma \right)}^{1.07·{\left(1-n\right)}^{1.36}},$$

(70)

$${\varphi }_{\mathrm{nn},\mathrm{c}}\approx {\left(1+\Gamma \right)}^{1.16·{\left(1-n\right)}^{1.23}}$$

(71)

respectively, or by Habchi et al. [60]:

$${\varphi }_{\mathrm{nn},\min }\approx {\left\{{\left(\frac{{\eta }_{\infty }}{{\eta }_0}\right)}^{0.893}+\left[1-{\left(\frac{{\eta }_{\infty }}{{\eta }_0}\right)}^{0.893}\right]·{\left(1+1.543·\Gamma \right)}^{n-1}\right\}}^{-1},$$

(72)

$${\varphi }_{\mathrm{nn},\mathrm{c}}\approx {\left\{{\left(\frac{{\eta }_{\infty }}{{\eta }_0}\right)}^{0.7469}+\left[1-{\left(\frac{{\eta }_{\infty }}{{\eta }_0}\right)}^{0.7469}\right]·{\left(1+1.678·\Gamma \right)}^{n-1}\right\}}^{-1}.$$

(73)

The latter were derived for fluids with Carreau viscosity and two Newtonian plateaus.

4.4. Starvation

Assuming an unlimited oil supply, the EHL pressure build-up can start at any distance from the contact center. However, a lubricant contact may suffer from starvation due to high speeds, highly viscous lubricants, or insufficient lubricant supply. The lubricant supply is such that the starting point of the pressure generation is closer to the contact and the generated film thickness is significantly smaller compared to the fully flooded case [61]. The latter is reached when the distance between contact and inlet, the so-called inlet meniscus, ceases to affect the minimum film thickness to any significant extent. Therefore, Hamrock and Dowson [62] suggested taking the fully flooded-starved boundary:

$$m_{\min }^{*}\approx 1+3.34·{\left[{\left(\frac{R_{\mathrm{x}}}{b_{\mathrm{H}}}\right)}^2·H_{\min }\right]}^{0.56},$$

(74)

$$m_{\mathrm{c}}^{*}\approx 1+3.06·{\left[{\left(\frac{R_{\mathrm{x}}}{b_{\mathrm{H}}}\right)}^2·H_{\mathrm{c}}\right]}^{0.58}$$

(75)

and the inlet location m into account to calculate correction factors for the minimum lubricant gap

$${\varphi }_{\mathrm{s},\min }\approx {\left(\frac{m-1}{m^{*}-1}\right)}^{0.25}$$

(76)

and the central film thickness:

$${\varphi }_{\mathrm{s},\mathrm{c}}\approx {\left(\frac{m-1}{m^{*}-1}\right)}^{0.29}.$$

(77)

Instead, Wedeven et al. [63] proposed:

$$m^{*}\approx 1+3.52·{\left[{\left(\frac{R_{\mathrm{x}}}{b_{\mathrm{H}}}\right)}^2·H_{\mathrm{c}}\right]}^{\frac{2}{3}}$$

(78)

for the fully flooded-starved boundary and Wisniewski [64]

$${\varphi }_{\mathrm{s},\min }\approx 0.6·\log \left({\theta }_{\mathrm{m}}\right)+0.535$$

(79)

as a function of the ratio of the fractional film content at the contact inlet

$${\theta }_{\mathrm{m}}=\frac{h_{\mathrm{liq}}\left(m^{*}\right)}{h\left(m^{*}\right)}$$

(80)

for the correction factor [15].

4.5. Surface Roughness and Asperity Contact

It is well accepted that the surface topography in terms of stochastic, manufacturing-related deviations from the smooth, ideal flat surface or intentionally produced surface features such as surface textures has a decisive impact on the film formation in EHL contacts [65,66,67,68,69]. While waviness or roughness oriented transversely to the direction of motion can have a positive effect on the global lubricant film height under certain circumstances [70,71], structures oriented in the direction of motion typically induce negative effects [72,73]. In addition, the consideration of rough surfaces is often accompanied by locally strongly increased pressures [74,75,76]. With decreasing lubricant film heights, simultaneous solid asperity contact and hydrodynamic pressure build-up can occur, thus sharing the normal load, which is referred to as mixed lubrication [77]. A first approach to considering the influence of surface topography was introduced by Zhu and Cheng [78] based upon the statistical consideration of surface asperities in terms micro-hydrodynamics [79] and load sharing [80,81]. Similar to the flow factor method from Patir and Cheng [82], they derived corresponding correction factors to adjust the minimum and central film height depending on the orientation of the surface topography and the ratio of the smooth lubricant gap to the quadratic mean surface roughness (Figure 6). To quantify the spatial orientation, the so-called Peklenik factor γ can be used as a quotient of the characteristic correlation lengths of two mutually orthogonal measurement directions [83]. Using deterministic calculations assuming full film lubrication, Kumar et al. [84] derived a correction factor for 2D line-contacts depending on the normalized amplitude A of the surface waviness or roughness:

$${\varphi }_{\mathrm{r}}\approx 1-0.7823·A^{0.8213}=1-1.04·{\left(\frac{\sigma ·R_{\mathrm{x}}}{b_{\mathrm{H}}^2}\right)}^{0.8213}.$$

(81)

However, it is important to mention that the wavelength was assumed to be constant for the analysis and only pure rolling conditions were considered, thus neglecting the impact of velocities and slip. Using an stochastic approach similar to Zhu and Cheng, Masjedi and Khonsari [85] developed correction factors for adjusting the minimum film thickness:

$${\varphi }_{\mathrm{r},\min }\approx 1+0.141·{\left(\frac{\sigma }{R_{\mathrm{x}}}\right)}^{1.073}·V^{0.149}·W^{-0.044}·U^{-0.828}·G^{-0.954}·{\kappa }^{-0.395}$$

(82)

and central film thickness

$${\varphi }_{\mathrm{r},\mathrm{c}}\approx 1+0.025·{\left(\frac{\sigma }{R_{\mathrm{x}}}\right)}^{1.248}·V^{0.119}·W^{-0.133}·U^{-0.884}·G^{-0.977}·{\kappa }^{-0.081}.$$

(83)

5. Applicability, Limitations and Future Directions

An overview of the applicability for different contact forms and conditions of the presented film thickness approximations is displayed in

Table 2. Overview of film thickness equations and correction factors.

		Line-Contact	Point-Contact	Elliptical Contact
	minimum film thickness	Dowson and Higginson [14], Moes [16], Dowson [25], Jacobson and Hamrock [26], Johnson [19], Myers et al. [28], Moes [31]	Evans and Snidle [33], Hamrock and Dowson [34], Chittenden et al. [35],	Hamrock and Dowson [34], Chittenden et al. [35]
	central film thickness	Dowson and Toyoda [27], Moes [32]	Evans and Snidle [33], Hamrock and Dowson [34], Chittenden et al. [35], Moes [32], Nijenbanning et al. [36]	Hamrock and Dowson [34], Chittenden et al. [35], Nijenbanning et al. [36]
correction factor for	fluid compressibility	Canzi et al. [44]	Canzi et al. [44]	Canzi et al. [44]
	thermal effects	Greenwood and Kauzlarich [46], Murch and Wilson [50], Jackson [51], Wilson and Sheu [52], Pandey and Gosh [53]
	non-Newtonian fluid behavior	Bair [22], Jang et al. [58]	Kumar et al. [59], Habchi et al. [60]
	starvation	Hamrock and Dowson [62] Wisniewski [64]
	surface roughness and asperity contact	Kumar et al. [84]	Zhu and Cheng [78], Masjedi and Khonsari [85]	Masjedi and Khonsari [85]

and Figure 7.

As can be seen in Table 2 and Figure 7, there are many approaches for film thickness equations, ranging from the infinite line-contact to point- or elliptical contacts. Yet, these equations were originally derived from different numerical data and, therefore, have individual ranges of validity. While comparisons of the approaches for 2D line-contacts are practically not possible, this possibility exists for point contacts. The experimental studies from Chaomleffel et al. [37] using interferometric film thickness measurements demonstrated reasonable agreement for the central film thickness even when using lubricants with shear thinning characteristics and applying the equations outside their stated ranges. However, the minimum film thickness outside the proposed domain of the equations was greatly influenced and could not be predicted for all conditions. In this context, the usage of other rheological models, e.g., from Eyring [87] or Lee-Hamrock [88], seems reasonable (see Section 4.5). Wheeler et al. [86] came to similar conclusions regarding the accuracy of the equations when predicting the central film height and comparing these values with numerical predictions. They also reported on some shortcomings for heavily loaded contacts with slow entrainment and recommended the use of the Chittenden formulation [35] for the central film height and the Nijenbanning model [36] for the minimum lubricant gap.

When analyzing the film thickness equations in detail, it becomes evident that they only apply for stationary load cases. However, real operating conditions of machine elements or engine components are never completely stationary, and time dependencies are usually present or even dominant. For the extreme scenario of pure impact EHL, Wang et al. [89]

$$H_{\mathrm{c},\min }\approx 2.3·M_{2\mathrm{D}}^{0.2}·L^{0.55}$$

(84)

as well as Venner et al. [90]

$$H_{\mathrm{c},\min }\approx 0.73·M_{3\mathrm{D}}^{\frac{1}{6}}·L^{0.55}$$

(85)

suggested equations for the minimum central film thickness in a piezoviscous line- and point-contact, respectively, which show similarities to the classical EHL contact. Nevertheless, there is still more potential for future enhancement and extension of the film thickness equations towards transient effects. In this regard, the influence of surface properties and topographies can be also seen, which becomes even more important in the context of start-stop-operations, low viscosity lubricants, as well as the trend to operate under mixed lubrication [91]. This applies for manufacturing-related surface roughnesses and designed surface textures or even thin coatings [10,92]. Even though they can already be deterministically modelled by advanced numerical approaches across different scales [93], apart from [78,84,85], surface properties have not yet found their way into analytically solvable film thickness equations. This could be done based upon the development of further correction factors and/or by using the existing non-dimensional groups. In addition, modern machine learning (ML) or artificial intelligence (AI) algorithms, such as artificial neural networks (ANN), could play a decisive role in an efficient and accurate way, see

Table 3. Comparison of different methods for film thickness prediction according to [94].

	Numerical EHL Simulation	Analytically Solvable Proximity Equation	Machine Learning Algorithm
software resources	High	very low	low
calculation time	minutes to hours	few minutes	few seconds
accuracy	very high	medium to good	good

. Properly trained models can have an excellent predictive power with a high level of correlation between calculations and simulation or experimental data [94]. Thereby, ML algorithms could be interpreted as a kind of black-box, which determines relevant EHL result variables depending on the input parameters (see Figure 1). In this regard, the prediction can go far beyond the minimum or central lubricant gap calculated in the analytical film thickness equations and also include the maximum pressure, shear rate or temperature rise. Furthermore, locally and time-resolved prediction models are conceivable.

To summarize, although the equations describing the film thickness were developed several decades ago, their use is still widespread and reasonable due to the fact that good predictions of film thickness are possible with negligible computing time. However, it is highly recommended to consider the validity range of the equations applied. Furthermore, the available correction factors should be evaluated to estimate a possible influence of thermal or non-Newtonian effects as well as the surface topography at least in a first approximation. In summary, there is still potential for extending the fluid film equations, especially regarding transient effects (time-varying stress collectives as well as surface properties and topography).