Removal of Different Dye Solutions: A Comparison Study Using a Polyamide NF Membrane
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.1.1. Membrane
2.1.2. Reagents
- Acid Brown-83 (AB83), C18H13N6NaO8S and its molecular weight is 496.39 g/mol. Supplied by Alfa Industries (Spain).
- Allura Red (AR), C18H14N2Na2O8S2. Its molecular weight is 496.44 g/mol, 80% of purity. Supplied by Sigma-Aldrich INC (Germany).
- Basic Fuchsin (BF), C20H20ClN3. Its molecular weight is 337.86 g/mol. Supplied by Sigma-Aldrich (Germany).
- Crystal Violet (CV), C20H11N2Na3O10S3. Its molecular weight is 407.98 g/mol and ≥90% of purity. Supplied by Sigma-Aldrich INC (Germany).
- Methyl Orange (MO), C14H14N3O3NaS. Its molecular weight is 327.33 g/mol. Supplied by Probus (Spain).
- Sunset Yellow (SY), C16H10N2Na2O7S2. Its molecular weight is 452.37 g/mol and 80% of purity. Supplied by Sigma (Germany).
- Sodium Chloride (NaCl). Its molecular weight is 58.4 g/mol. Supplied by Panreac (Spain).
- Hydrous magnesium chloride, MgCl·6H2O. Its molecular weight is 203.30 g/mol. Supplied by Panreac (Spain).
2.2. Equipment
2.2.1. Membrane Module
2.2.2. Spectrophotometer
2.2.3. Variable Pressure Scanning Electron Microscope
- Resolution: 3 nm (high vacuum mode) or 4.5 nm (low vacuum mode);
- Zoom: 15–300,000;
- Accelerating voltage: 0.3–30 kv;
- Variable pressure range: 1–270 Pa;
- Secondary electrons detector;
- Robinson’s backscattered electron detector;
- Secondary electrons in variable pressure detector;
- X-ray detector;
- Eucentric plate with computer control and motorized movements in X, Y, Z, R and T;
- Crio-SEM cooling plate (−190 ± 60 °C);
- Peltier’s cooling sample holder (−15 ± 50 °C).
2.3. Experimental Series
2.3.1. Distilled Water Assays
2.3.2. Salts Assays
2.3.3. Dyes Assays
- Firstly, while the pH was maintained constant at 7 and the flow at 150 L/h, the operating pressure was varied: 10 and 15 bar.
- With the aim of finding out the influence of the pH, the previous experimental series were repeated, changing the pH: first at 8 and afterwards at 3.
- Finally, once all of these assays were carried out, the distilled water assay and the salts assay were repeated to check if the membrane had lost permeability after its use.
3. Results and Discussion
3.1. Membrane Characterization
Permeability Coefficient Determination
3.2. Determination of Selectivity and Performance of the Membrane against Salt Solutions
3.3. Influence of the Chemical Structure of Different Dyes
3.4. Influence of pH: Comparison of Electrostatic Interaction and Membrane Performance
3.5. Fouling Phenomenon after Treatment of Dyes Solutions
3.6. Morphologic Study of the Membrane
3.7. Application of the Spiegler–Kedem–Katchalsky Model
- Reflection coefficient (σ). This indicates the capacity of the membrane to be permeated by the solute. A σ = 0 value indicates that the membrane is completely permeable for the solute, whereas a σ = 1 value indicates that the solute is unable to go through the membrane, as it is completely impermeable (total reflection).
- Solute permeability coefficient (Ps). It is defined as the speed at which the solute passes through the membrane. It is unique for each compound and membrane. It is measured in m/s.
3.8. Comparative Study of the Results
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| C0 | solute concentration in the feed (kg/m3) |
| Cm | solute concentration in the membrane (kg/m3) |
| Cp | solute concentration in the permeate (kg/m3) |
| Cs | logarithmic average solute concentration between feeding and permeate (kg/m3) |
| FF | fouling factor (%) |
| Js | solute flux density (kg/m2s) |
| Jv | solvent flux density (kg/m2s) |
| Lp | solvent permeability coefficient (m/s) |
| Lp0 | initial solvent permeability coefficient (m/s) |
| Lpf | final solvent permeability coefficient (m/s) |
| Ps | solute permeability coefficient (m/s) |
| r | rejection (dimensionless) |
| R | rejection coefficient (%) |
| Robs | observed rejection coefficient (%) |
| ΔP | operating pressure (Pa) |
| ΔП | osmotic pressure (Pa) |
| σ | reflection coefficient |
References
- Dasgupta, J.; Sikder, J.; Chakraborty, S.; Curcio, S.; Drioli, E. Remediation of textile effluents by membrane based treatment techniques: A state of the art review. J. Environ. Manag. 2015, 147, 55–72. [Google Scholar] [CrossRef]
- Donkadokula, N.Y.; Kola, A.K.; Naz, I.; Saroj, D. A review on advanced physico-chemical and biological textile dye wastewater treatment techniques. Rev. Environ. Sci. Biotechnol. 2020, 19, 543–560. [Google Scholar] [CrossRef]
- De Jager, D.; Sheldon, M.S.; Edwards, W. Colour removal from textile wastewater using a pilot-scale dual-stage MBR and subsequent RO system. Sep. Purif. Technol. 2014, 135, 135–144. [Google Scholar] [CrossRef]
- Verma, A.K.; Dash, R.R.; Bhunia, P. A review on chemical coagulation/flocculation technologies for removal of colour from textile wastewater. J. Environ. Manag. 2012, 93, 154–168. [Google Scholar] [CrossRef] [PubMed]
- Liang, C.Z.; Sun, S.P.; Li, F.Y.; Ong, Y.K.; Chung, T.S. Treatment of highly concentrated wastewater containing multiple synthetic dyes by a combined process of coagulation/flocculation and nanofiltration. J. Membr. Sci. 2014, 469, 306–315. [Google Scholar] [CrossRef]
- Lau, W.J.; Ismail, A.F. Polymeric nanofiltration membranes for textile dye wastewater treatment: Preparation, performance evaluation, transport modelling, and fouling control-a review. Desalination 2009, 245, 321–348. [Google Scholar] [CrossRef]
- Wang, Z.; Wu, A.; Ciacchi, L.C.; Wei, G. Recent Advances in Nanoporous Membranes for Water Purification. Nanomaterials 2018, 8, 65. [Google Scholar] [CrossRef] [Green Version]
- Ghadhban, M.Y.; Majdi, H.S.; Rashid, K.T.; Alsalhy, Q.F.; Lakshmi, D.S.; Salih, I.K.; Figoli, A. Removal of dye from a leather tanning factory by flat-sheet ultrafiltration (UF) membrane. Membranes 2020, 10, 47. [Google Scholar] [CrossRef] [Green Version]
- Guo, D.; Xiao, Y.; Li, T.; Zhou, Q.; Shen, L.; Li, R.; Xu, Y.; Lin, H. Fabrication of high-performance composite nanofiltration membranes for dye wastewater treatment: Mussel-inspired layer-by-layer self-assembly. J. Colloid Interface Sci. 2020, 560, 273–283. [Google Scholar] [CrossRef]
- Jun, B.M.; Yoon, Y.; Park, C.M. Post-treatment of nanofiltration polyamide membrane through alkali-catalyzed hydrolysis to treat dyes in model wastewater. Water 2019, 11, 1645. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Wong, E.; Mai, Z.; Der Bruggen, B.V. Fabrication of composite polyamide/Kevlar aramid nanofiber nanofiltration membranes with high permselectivity in water desalination. J. Membr. Sci. 2019, 592, 117396–117407. [Google Scholar] [CrossRef]
- Benkhaya, S.; M´rabet, S.; Hsissou, R.; El Harfi, A. Synthesis of new low-cost organic ultrafiltration membrane made from Polysulfone/Polyetherimide blends and its application from soluble azoic dyes removal. J. Mater. Res. Technol. 2020, 9, 4763–4772. [Google Scholar]
- Hidalgo, A.M.; Leon, G.; Gómez, M.; Murcia, M.D.; Gómez, E.; Penalva, P. Removal of 4-chloro-2-methyl-phenol from aqueous solutions by nanofiltration and reverse osmosis. Desalin. Water Treat. 2015, 53, 1499–1505. [Google Scholar] [CrossRef]
- Hidalgo, A.M.; Murcia, M.D.; Gómez, E.; Leon, G.; Cascales, E. Influence of physicochemical parameters of organic solutes on the retention and flux in nanofiltration process. Chem. Eng. Technol. 2016, 39, 1177–1184. [Google Scholar] [CrossRef]
- Cheng, L.; Zhu, L.P.; Zhang, P.B.; Sun, J.; Zhu, B.K.; Xu, Y.Y. Molecular separation by poly (N-vinyl imidazole) gel-filled membranes. J. Membr. Sci. 2016, 497, 472–484. [Google Scholar] [CrossRef]
- Chidambaram, T.; Oren, Y.; Noel, M. Fouling of nanofiltration membranes by dyes during brine recovery from textile dye bath wastewater. Chem. Eng. J. 2015, 262, 156–168. [Google Scholar] [CrossRef]
- Li, C.; Yang, Y.; Liu, Y.; Hou, L. Removal of PhACs and their impacts on membrane fouling in NF/RO membrane filtration of various matrices. J. Membr. Sci. 2018, 548, 439–448. [Google Scholar] [CrossRef]
- Zhang, N.; Huang, Z.; Yang, N.; Zhang, L.; Jiang, B.; Sun, Y.; Ma, J. Nanofiltration membrane via EGCG-PEI co-deposition followed by crosslinking on microporous PTFE substrates for desalination. Sep. Purif. Technol. 2020, 232, 115964–115974. [Google Scholar] [CrossRef]
- Restolho, J.A.; Prates, A.; Pinho, M.N.; Afonso, M.D. Sugars and lignosulphonates recovery from eucalyptus spent sulphite liquor by membrane processes. Biomass Bioenergy 2009, 33, 1558–1566. [Google Scholar] [CrossRef]
- Catarino, M.; Mendes, A. Dealcoholizing wine by membrane separation processes. Innov. Food Sci. Emerg. Technol. 2011, 12, 330–337. [Google Scholar] [CrossRef]
- Labarca, F.; Bórquez, R. Comparative study of nanofiltration and ion exchange for nitrate reduction in the presence of chloride and iron in groundwater. Sci. Total Environ. 2020, 723, 137809–137821. [Google Scholar] [CrossRef] [PubMed]
- Wijmans, J.G.; Baker, R.W. The solution-diffusion model: A review. J. Membr. Sci. 1995, 107, 1–21. [Google Scholar] [CrossRef]
- Revchuk, A.D.; Suffet, I.N. Ultrafiltration separation of aquatic natural organic matter: Chemical probes for quality assurance. Water Res. 2009, 43, 3685–3692. [Google Scholar] [CrossRef] [PubMed]
- Hidalgo, A.M.; Gómez, M.; Murcia, M.D.; Serrano, M.; Rodríguez-Schmidt, R.; Escudero, P.A. Behaviour of polysulfone ultrafiltration membrane for dyes removal. Water Sci. Technol. 2018, 77, 2093–2100. [Google Scholar] [CrossRef]
- Lee, A.; Elamb, J.W.; Darling, S.B. Membrane materials for water purification: Design, development, and application. Environ. Sci. Water Res. Technol. 2016, 2, 17–42. [Google Scholar] [CrossRef]
- Abdel-Fatah, M.A. Nanofiltration systems and applications in wastewater treatment: Review article. Ain Shams Eng. J. 2018, 9, 3077–3092. [Google Scholar] [CrossRef]
- Yang, Z.; Zhou, Y.; Feng, Z.; Rui, X.; Zhang, T.; Zhang, Z. A review on reverse osmosis and nanofiltration membranes for water purification. Polymers 2019, 11, 1252. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kedem, O.; Katchalsky, A. Thermodynamics of flow processes in biological systems. Biophys. J. 1962, 2, 53–78. [Google Scholar]
- Spiegler, K.S.; Kedem, O. Thermodynamics of hyperfiltration (reverse osmosis): Criteria for efficient membranes. Desalination 1966, 1, 311–326. [Google Scholar] [CrossRef]
- Ben-David, A.; Bason, S.; Jopp, J.; Oren, Y.; Freger, V. Partitioning of organic solutes between water and polyamide layer of RO and NF membranes: Correlation of rejection. J. Membr. Sci. 2006, 281, 480–490. [Google Scholar] [CrossRef]
- Hidalgo, A.M.; León, G.; Gómez, M.; Murcia, M.D.; Gómez, E.; Gómez, J.L. Application of the Spiegler-Kedem-Kachalsky model to the removal of 4-chlorophenol by different nanofiltration membranes. Desalination 2013, 315, 70–75. [Google Scholar] [CrossRef]
- Yaroshchuk, A.E. Rejection of singles salts versus transmembrane volume flow in RO/NF: Thermodynamic properties, model of constant coefficients, and its modification. J. Membr. Sci. 2002, 198, 285–297. [Google Scholar] [CrossRef]
- Pontie, M.; Buisson, H.; Diawara, C.K.; Essis-Tome, H. Studies of halide ions mass transfer in nanofiltration application to selective defluorination of brackish drinking water. Desalination 2003, 157, 127–134. [Google Scholar] [CrossRef]
- Nora’aini, A.; Abdul Wahab, M. Regional Symposium on Membrane Science and Technology. In The Development of Predictive Modeling of Nanofiltration Membrane Properties: A Review; Kolej Universiti Sains & Teknologi: Johor, Malaysia; Universiti Kebangsaan: Johor, Malaysia, 2004. [Google Scholar]







| Manufacturer | Alfa Laval (Denmark) |
| Product denomination | NF99 |
| Type | Thin-film composite on polyester |
| Composition | Polyamide |
| Membrane surface area (m2) | 0.003 |
| Maximum pressure (N m−2) | 55 × 105 |
| MgSO4 rejection (%) (2 kg m−3, 9·105 N m−2) | ≥98 |
| NaCl rejection (%) | >90 |
| pH range | 3−10 |
| Temperature range (°C) | 5–50 |
| Dyes | Log Kow | pKa | Water Solubility (g L−1) |
|---|---|---|---|
| AB83 | - | - | >100 |
| AR | −0.55 | - | 225 |
| BF | 1.632 (*) | - | 1–5 |
| CV | 0.96 0.51 | pKa1 = 5.31 pKa2 = 8.64 | 4 |
| MO | −0.66 (*) | - | 0.2 5.0 (*) |
| SY | −1.18 | pKa1 = 0.82 pKa2 = 1.46 | 190 |
| Membrane | Water Permeability Lp (m s−1) | Solute Permeability Ps (m·s−1) | |
|---|---|---|---|
| NaCl | MgCl2 | ||
| NF99 | 1.665 × 10−8 | 6.705 × 10−6 | 1.632 × 10−7 |
| References [13,19,20] | 1.5 × 10−8 | - | - |
| Dyes | Allura Red | Basic Fuchsin | Crystal Violet | Methyl Orange | Sunset Yellow |
|---|---|---|---|---|---|
| Dreiding energy (kcal/mol) | 318.39 | 186.09 | 294.85 | 237.39 | 311.43 |
| MMFF94 energy (kcal/mol) | 196.18 | 69.17 | 121.74 | 93.12 | 184.7 |
| Minimal projection area (Å2) | 51.15 | 55.59 | 71.18 | 30 | 46.88 |
| Maximal projection area (Å2) | 117.52 | 81.35 | 105.67 | 93.1 | 105.50 |
| Minimal projection radius (Å) | 5.33 | 6.28 | 7.49 | 3.96 | 5.78 |
| Maximal projection radius (Å) | 8.57 | 6.76 | 8.06 | 8.58 | 8.40 |
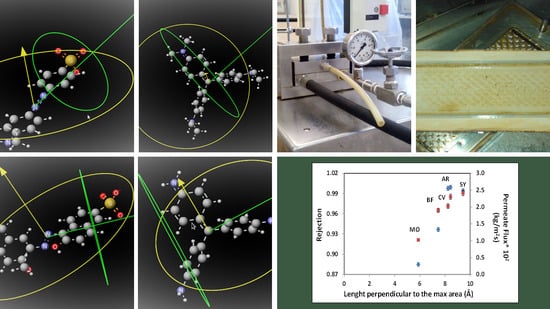
| Length perpendicular to the max area (Å) | 8.44 | 7.46 | 8.24 | 5.88 | 9.43 |
| Length perpendicular to the min area (Å) | 16.78 | 12.60 | 14.84 | 17.33 | 16.22 |
| Van der Waals volume (Å3) | 338.50 | 270.57 | 378.31 | 254.85 | 295.56 |
| Dyes | Permeate Flux (kg/m2 s) × 103 | Rejection Coefficients (%) | ||||
|---|---|---|---|---|---|---|
| pH = 3 | pH = 7 | pH = 8 | pH = 3 | pH = 7 | pH = 8 | |
| AB83 | 35.44 | 33.50 | 33.33 | 99.40 | 99.48 | 99.56 |
| AR | 39.20 | 41.11 | 38.40 | 99.99 | 99.93 | 99.95 |
| BF | - | 30.86 | - | - | 98.78 | - |
| CV | 38.66 | 32.96 | 16.75 | 99.91 | 99.78 | 99.98 |
| MO | 37.87 | 31.72 | 19.17 | 97.27 | 87.47 | 99.02 |
| SY | 34.67 | 40.00 | 38.67 | 99.78 | 99.22 | 99.88 |
| Dyes | AB83 | AR | BF | CV | MO | SY |
|---|---|---|---|---|---|---|
| FF (%) | 10.65 | 0.6 | 87.23 | 24.13 | 35.29 | 3.44 |
| Dyes | Ps (m s−1) | σ | Standard Deviation |
|---|---|---|---|
| AB83 | 1.6418 × 10−7 | 0.9954 | 0.3605 |
| AR | 2.6624 × 10−8 | 0.9994 | 3.8356 |
| BF | 2.021 × 10−7 | 0.9887 | 0.1692 |
| CV | 1.0198 × 10−7 | 0.9974 | 1.0023 |
| MO | 7.8114 × 10−7 | 0.9563 | 0.2147 |
| SY | 2.5221 × 10−7 | 0.9942 | 0.3382 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hidalgo, A.M.; León, G.; Gómez, M.; Murcia, M.D.; Gómez, E.; Macario, J.A. Removal of Different Dye Solutions: A Comparison Study Using a Polyamide NF Membrane. Membranes 2020, 10, 408. https://doi.org/10.3390/membranes10120408
Hidalgo AM, León G, Gómez M, Murcia MD, Gómez E, Macario JA. Removal of Different Dye Solutions: A Comparison Study Using a Polyamide NF Membrane. Membranes. 2020; 10(12):408. https://doi.org/10.3390/membranes10120408
Chicago/Turabian StyleHidalgo, Asunción María, Gerardo León, María Gómez, María Dolores Murcia, Elisa Gómez, and José Antonio Macario. 2020. "Removal of Different Dye Solutions: A Comparison Study Using a Polyamide NF Membrane" Membranes 10, no. 12: 408. https://doi.org/10.3390/membranes10120408
APA StyleHidalgo, A. M., León, G., Gómez, M., Murcia, M. D., Gómez, E., & Macario, J. A. (2020). Removal of Different Dye Solutions: A Comparison Study Using a Polyamide NF Membrane. Membranes, 10(12), 408. https://doi.org/10.3390/membranes10120408