Droplet Characteristics of Rotating Packed Bed in H2S Absorption: A Computational Fluid Dynamics Analysis
Abstract
:1. Introduction
2. Simulation
2.1. Physical Model and Grid Refinement of RPB
2.2. Mathematical Modelling
2.2.1. Governing Equations
2.2.2. Turbulence Model
2.2.3. Droplet Force Balance
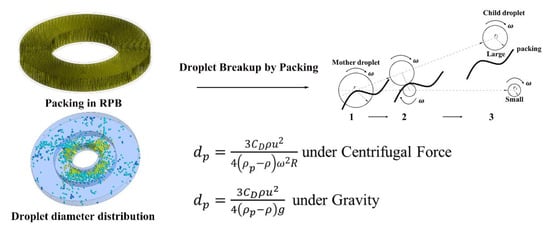
2.2.4. Droplet Coalescence and Breakup Model
2.3. Fluid Properties
2.4. Solution Procedure
2.5. Grid Independence
3. Results and Discussions
3.1. Droplet Velocity in RPB
3.1.1. Effect of Initial Droplet Velocity on Droplet Velocity
3.1.2. Effect of Rotating Speed on Droplet Velocity
3.2. Average Residence Time Distribution in RPB
3.2.1. Effect of Initial Droplet Velocity on Average Residence Time
3.2.2. Effect of Rotating Speed on Average Residence Time
3.3. Droplet Diameter Distribution in RPB
3.3.1. Effect of Initial Droplet Diameter on Droplet Diameter Distribution
3.3.2. Effect of Initial Droplet Velocity and Rotating Speed on Droplet Diameter
3.4. Principle of Processing Intensification in RPB
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclatures
| CFD | Computational fluid dynamics |
| HTU | Height of mass transfer unit |
| IS-RPB | Impinging stream RPB |
| RPB | Rotating packed bed |
| RSR | Rotor-stator reactor |
| SP-RPB | Split packing RPB |
| TAB | Taylor analogy breakup |
| Latin symbols | |
| Drag coefficient | |
| Aerodynamic force of droplet (N) | |
| External body force (N) | |
| Drag force (N) | |
| Force in rotating reference frame in x direction (N) | |
| Force in rotating reference frame in y direction (N) | |
| Virtual mass force (N) | |
| Additional acceleration term | |
| Influence of the buoyancy force | |
| Influence of the mean velocity gradients | |
| Turbulent Prandtl number for energy | |
| R | Radial position (m) |
| Actual collision parameter | |
| Critical offset of collision | |
| Initial droplet diameter (mm) | |
| Arithmetic mean diameter of two droplet (m) | |
| Gravitational vector (9.8 m/s2) | |
| Turbulence kinetic energy | |
| Mass of droplet (kg) | |
| Mass of large droplet (kg) | |
| Mass of small droplet (kg) | |
| Static pressure (Pa) | |
| Radius of large droplet (m) | |
| Radius of small droplet (m) | |
| Fluid velocity (m/s) | |
| Initial droplet velocity (m/s) | |
| Velocity of large droplet (m/s) | |
| Droplet velocity (m/s) | |
| Whirl velocity (m/s) | |
| Velocity of small droplet (m/s) | |
| Relative velocity (m/s) | |
| Displacement of droplet (m) | |
| Greek symbols | |
| Fluid density (kg/m3) | |
| Gas density (kg/m3) | |
| Liquid density (kg/m3) | |
| Droplet density (kg/m3) | |
| Rotating speed (rpm) | |
| Centrifugal acceleration (rad/s) | |
| Gas viscosity (mPa·s) | |
| Liquid viscosity (mPa·s) | |
| Molecular viscosity (mPa·s) | |
| Turbulent viscosity (mPa·s) | |
| Liquid surface tension (N/m) | |
| Dissipation rate | |
| Dimensionless groups | |
| Reynolds number | |
| Weber number | |
References
- Chen, Y.-S.; Lin, F.-Y.; Lin, C.-C.; Tai, C.Y.-D.; Liu, H.-S. Packing Characteristics for Mass Transfer in a Rotating Packed Bed. Ind. Eng. Chem. Res. 2006, 45, 6846–6853. [Google Scholar] [CrossRef]
- Neumann, K.; Gladyszewski, K.; Gross, K.; Qammar, H.; Wenzel, D.; Gorak, A.; Skiborowski, M. A guide on the industrial application of rotating packed beds. Chem. Eng. Res. Des. 2018, 134, 443–462. [Google Scholar] [CrossRef]
- Burns, J.R.; Ramshaw, C. Process intensification: Visual study of liquid maldistribution in rotating packed beds. Chem. Eng. Sci. 1996, 51, 1347–1352. [Google Scholar] [CrossRef]
- Guo, K.; Guo, F.; Feng, Y.; Chen, J.; Zheng, C.; Gardner, N.C. Synchronous visual and RTD study on liquid flow in rotating packed-bed contactor. Chem. Eng. Sci. 2000, 55, 1699–1706. [Google Scholar] [CrossRef]
- Li, Y.; Wang, S.; Sun, B.; Arowo, M.; Zou, H.; Chen, J.; Shao, L. Visual study of liquid flow in a rotor-stator reactor. Chem. Eng. Sci. 2015, 134, 521–530. [Google Scholar] [CrossRef]
- Sang, L.; Luo, Y.; Chu, G.-W.; Zhang, J.-P.; Xiang, Y.; Chen, J.-F. Liquid flow pattern transition, droplet diameter and size distribution in the cavity zone of a rotating packed bed: A visual study. Chem. Eng. Sci. 2017, 158, 429–438. [Google Scholar] [CrossRef]
- Shi, X.; Xiang, Y.; Wen, L.-X.; Chen, J.-F. CFD analysis of liquid phase flow in a rotating packed bed reactor. Chem. Eng. J. 2013, 228, 1040–1049. [Google Scholar] [CrossRef]
- Ouyang, Y.; Zou, H.-K.; Gao, X.-Y.; Chu, G.-W.; Xiang, Y.; Chen, J.-F. Computational fluid dynamics modeling of viscous liquid flow characteristics and end effect in rotating packed bed. Chem. Eng. Process. Process Intensif. 2018, 123, 185–194. [Google Scholar] [CrossRef]
- Ouyang, Y.; Wang, S.; Xiang, Y.; Zhao, Z.; Wang, J.; Shao, L. CFD analyses of liquid flow characteristics in a rotor-stator reactor. Chem. Eng. Res. Des. 2018, 134, 186–197. [Google Scholar] [CrossRef]
- Xie, P.; Lu, X.; Yang, X.; Ingham, D.; Ma, L.; Pourkashanian, M. Characteristics of liquid flow in a rotating packed bed for CO2 capture: A CFD analysis. Chem. Eng. Sci. 2017, 172, 216–229. [Google Scholar] [CrossRef]
- Ko, G.H.; Ryou, H.S. Modeling of droplet collision-induced breakup process. Int. J. Multiph. Flow 2005, 31, 723–738. [Google Scholar] [CrossRef]
- Wang, Z.; Yang, T.; Liu, Z.; Wang, S.; Gao, Y.; Wu, M. Mass Transfer in a Rotating Packed Bed: A Critical Review. Chem. Eng. Process. Process Intensif. 2019, 139, 78–94. [Google Scholar] [CrossRef]
- Liu, Y.; Luo, Y.; Chu, G.-W.; Luo, J.-Z.; Arowo, M.; Chen, J.-F. 3D numerical simulation of a rotating packed bed with structured stainless steel wire mesh packing. Chem. Eng. Sci. 2017, 170, 365–377. [Google Scholar] [CrossRef]
- Sang, L.; Luo, Y.; Chu, G.W.; Liu, Y.Z.; Liu, X.Z.; Chen, J.F. Modeling and experimental studies of mass transfer in the cavity zone of a rotating packed bed. Chem. Eng. Sci. 2017, 170, 355–364. [Google Scholar] [CrossRef]
- Qian, Z.; Li, Z.-H.; Guo, K. Industrial Applied and Modeling Research on Selective H2S Removal Using a Rotating Packed Bed. Ind. Eng. Chem. Res. 2012, 51, 8108–8116. [Google Scholar] [CrossRef]
- Qian, Z.; Xu, L.-B.; Li, Z.-H.; Li, H.; Guo, K. Selective Absorption of H2S from a Gas Mixture with CO2 by Aqueous N-Methyldiethanolamine in a Rotating Packed Bed. Ind. Eng. Chem. Res. 2010, 49, 6196–6203. [Google Scholar] [CrossRef]
- Guo, F.; Zheng, C.; Guo, K.; Feng, Y.; Gardner, N.C. Hydrodynamics and mass transfer in cross-flow rotating packed bed. Chem. Eng. Sci. 1997, 52, 3853–3859. [Google Scholar] [CrossRef]
- Yang, P.-F.; Luo, S.; Zhang, D.-S.; Yang, P.-Z.; Liu, Y.-Z.; Jiao, W.-Z. Extraction of nitrobenzene from aqueous solution in impinging stream rotating packed bed. Chem. Eng. Process. Process Intensif. 2018, 124, 255–260. [Google Scholar] [CrossRef]
- Rajan, S.; Kumar, M.; Ansari, M.J.; Rao, D.P.; Kaistha, N. Limiting Gas Liquid Flows and Mass Transfer in a Novel Rotating Packed Bed (HiGee). Ind. Eng. Chem. Res. 2011, 50, 986–997. [Google Scholar] [CrossRef]
- Shivhare, M.K.; Rao, D.P.; Kaistha, N. Mass transfer studies on split-packing and single-block packing rotating packed beds. Chem. Eng. Process. Process Intensif. 2013, 71, 115–124. [Google Scholar] [CrossRef]






















| Inner Diameter (mm) | Outer Diameter (mm) | Height (mm) | |
|---|---|---|---|
| RPB | 45 | 160 | 24 |
| Packing | 48 | 92 | 20 |
| CH4 | C2H6 | C3H8 | C4H10 | C5H12 | CO2 | H2S | N2 | |
|---|---|---|---|---|---|---|---|---|
| Gas (mol %) | 85.71 | 2.30 | 0.73 | 0.47 | 0.24 | 4.25 | 5.04 | 1.27 |
| Liquid (m %) | An MDEA aqueous solution with a mass fraction of 35% | |||||||
| Rotating Speed (rpm) | 300 | 600 | 900 | 900 | 900 |
|---|---|---|---|---|---|
| Initial droplet velocity (m/s) | 0.5 | 0.5 | 0.5 | 1.5 | 2.5 |
| Number of droplets | 58 | 111 | 183 | 360 | 391 |
| Total residence time (s) | 7.7 | 12.7 | 15.5 | 17.2 | 15.0 |
| Average residence time (s) | 0.132 | 0.114 | 0.085 | 0.048 | 0.039 |
| Force Field | Droplet Diameter |
|---|---|
| Rotating packed bed | |
| deposition process under gravity |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Wu, X.; Yang, T.; Wang, S.; Liu, Z.; Dan, X. Droplet Characteristics of Rotating Packed Bed in H2S Absorption: A Computational Fluid Dynamics Analysis. Processes 2019, 7, 724. https://doi.org/10.3390/pr7100724
Wang Z, Wu X, Yang T, Wang S, Liu Z, Dan X. Droplet Characteristics of Rotating Packed Bed in H2S Absorption: A Computational Fluid Dynamics Analysis. Processes. 2019; 7(10):724. https://doi.org/10.3390/pr7100724
Chicago/Turabian StyleWang, Zhihong, Xuxiang Wu, Tao Yang, Shicheng Wang, Zhixi Liu, and Xiaodong Dan. 2019. "Droplet Characteristics of Rotating Packed Bed in H2S Absorption: A Computational Fluid Dynamics Analysis" Processes 7, no. 10: 724. https://doi.org/10.3390/pr7100724
APA StyleWang, Z., Wu, X., Yang, T., Wang, S., Liu, Z., & Dan, X. (2019). Droplet Characteristics of Rotating Packed Bed in H2S Absorption: A Computational Fluid Dynamics Analysis. Processes, 7(10), 724. https://doi.org/10.3390/pr7100724