Single and Binary Equilibrium Studies for Ni2+ and Zn2+ Biosorption onto Lemna gibba from Aqueous Solutions
Abstract
:1. Introduction
2. Materials and Methods
2.1. Ni2+ and Zn2+ Stock and Test Solutions
2.2. Preparation of Biosorbent
2.3. Proximate Chemical Analysis of Biosorbent
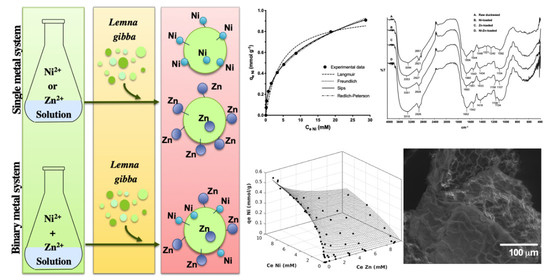
2.4. Biosorption Studies
2.5. Modeling of Single and Binary Equilibrium Biosorption Isotherms of Ni2+ and Zn2+ Ions
2.5.1. Mono-Component Isotherm Models
Freundlich Isotherm Model
Langmuir Isotherm Model
Sips Isotherm Model
Redlich–Peterson Isotherm Model
2.5.2. Multi-Component Isotherm Models
Non-Modified Competitive Langmuir Isotherm Model
Modified Competitive Langmuir Isotherm
Modified Langmuir Isotherm with an Interaction Factor η
Extended Freundlich Isotherm
Modified Sips Isotherm
Non-Modified Competitive Redlich-Peterson Isotherm
Modified Redlich-Peterson Isotherm with Interaction Factor η
2.6. Determination of Isotherm Parameters and Statistical Analysis
2.7. FTIR Analysis
2.8. SEM and SEM-EDX Analysis
2.9. Analytical Techniques
3. Results and Discussion
3.1. Proximate Composition of L. gibba
3.2. Influences of Metal Solution pH on the Individual and Simultaneous Biosorption of Ni2+ and Zn2+ onto L. gibba
3.3. Influence of Initial Metal Concentration in the Single and Binary Biosorption Systems of Ni2+ and Zn2+ onto L. gibba
3.4. Isotherms and Their Modeling
3.4.1. Single-Metal Biosorption Systems
3.4.2. Binary-Metal Biosorption Systems
3.5. FTIR Analysis
3.6. SEM-EDX Analysis
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Nys, C.; Asselman, J.; Hochmuth, J.D.; Janssen, C.R.; Blust, R.; Smolders, E.; De Schamphelaere, K.A. Mixture toxicity of nickel and zinc to Daphnia magna is noninteractive at low effect sizes but becomes synergistic at high effect sizes. Environ. Toxicol. Chem. 2015, 34, 1091–1102. [Google Scholar] [CrossRef] [PubMed]
- Meng, L.; Lin, D.; Wang, J.; Zeng, Y.; Liu, Y.; Lu, X. Electrochemically Activated Nickel–Carbon Composite as Ultrastable Cathodes for Rechargeable Nickel–Zinc Batteries. ACS Appl. Mater. Interfaces 2019, 11, 14854–14861. [Google Scholar] [CrossRef]
- Yang, S.; Bo, M.; Peng, C.; Li, Y.; Li, Y. Three-electrode flexible zinc-nickel battery with black phosphorus modified polymer electrolyte. Mater. Lett. 2018, 233, 118–121. [Google Scholar] [CrossRef]
- Malamis, S.; Katsou, E. A review on zinc and nickel adsorption on natural and modified zeolite, bentonite and vermiculite: Examination of process parameters, kinetics and isotherms. J. Hazard. Mater. 2013, 252, 428–461. [Google Scholar] [CrossRef] [PubMed]
- Bashmakova, E.B.; Pashkovskiy, P.P.; Radyukina, N.L.; Kuznetsov, V.V. Possible mechanism of iron deficit development in Mimulus guttatus plants exposed to joint action of nickel and zinc salts. Russ. J. Plant Physiol. 2015, 62, 761–771. [Google Scholar] [CrossRef]
- Brocato, J.; Costa, M. 10th NTES Conference: Nickel and arsenic compounds alter the epigenome of peripheral blood mononuclear cells. J. Trace Elements Med. Biol. 2015, 31, 209–213. [Google Scholar] [CrossRef] [Green Version]
- Maroney, M.J.; Ciurli, S. Nonredox Nickel Enzymes. Chem. Rev. 2013, 114, 4206–4228. [Google Scholar] [CrossRef] [Green Version]
- Nriagu, J. Zinc Toxicity in Humans. In Encyclopedia of Environmental Health; Elsevier BV: Amsterdam, The Netherlands, 2011; Volume 5, pp. 801–807. [Google Scholar]
- Martinez, R.S.; Sáenz, M.E.; Alberdi, J.L.; Di Marzio, W.D. Comparative ecotoxicity of single and binary mixtures exposures of nickel and zinc on growth and biomarkers of Lemna gibba. Ecotoxicology 2019, 28, 686–697. [Google Scholar] [CrossRef]
- De Figuerêdo, L.P.; Nilin, J.; Da Silva, A.Q.; Damasceno, É.P.; Loureiro, S.; Costa-Lotufo, L.V. Zinc and nickel binary mixtures act additively on the tropical mysid Mysidopsis juniae. Mar. Freshw. Res. 2016, 67, 301–308. [Google Scholar] [CrossRef]
- Aranda-García, E.; Cristiani-Urbina, E. Kinetic, Equilibrium, and Thermodynamic Analyses of Ni(II) Biosorption from Aqueous Solution by Acorn Shell of Quercus crassipes. Water Air Soil Pollut. 2018, 229, 119. [Google Scholar] [CrossRef]
- Aranda-García, E.; Cristiani-Urbina, E. Effect of pH on hexavalent and total chromium removal from aqueous solutions by avocado shell using batch and continuous systems. Environ. Sci. Pollut. Res. 2019, 26, 3157–3173. [Google Scholar] [CrossRef] [PubMed]
- Arim, A.L.; Guzzo, G.; Quina, M.J.; Ferreira, L.M.G. Single and binary sorption of Cr(III) and Ni(II) onto modified pine bark. Environ. Sci. Pollut. Res. 2018, 25, 28039–28049. [Google Scholar] [CrossRef] [PubMed]
- Thangaraj, V.; Aravamudan, K.; Lingam, R.; Subramanian, S. Individual and simultaneous adsorption of Ni (II), Cd (II), and Zn (II) ions over polyamide resin: Equilibrium, kinetic and thermodynamic studies. Environ. Prog. Sustain. Energy 2019, 38, S340–S351. [Google Scholar] [CrossRef]
- León, M.D.C.C.-D.; Flores-Alamo, N.; Solache-Ríos, M.J.; De La Rosa-Gómez, I.; Díaz-Campos, G. Lead and Copper Adsorption Behaviour by Lemna gibba: Kinetic and Equilibrium Studies. CLEAN—Soil Air Water 2017, 45, 1600357. [Google Scholar] [CrossRef]
- Babativa, D.F.R.; Ramírez, A.J.E. Comparación de las características poblacionales de Lemna minuta (ARACEAE: LEMNOIDEAE) en tres medios de cultivo. Rev. Colomb. Biotecnol. 2018, 20, 84–96. [Google Scholar] [CrossRef]
- Topal, M.; Obek, E.; Şenel, G.U.; Topal, E.I.A. Removal of tetracycline antibiotic by Lemna gibba L. from aqueous solutions. Water Environ. J. 2020, 34, 37–44. [Google Scholar] [CrossRef]
- Mkandawire, M.; Dudel, E.G. Are Lemna spp. effective phytoremediation agents? Bioremediat. Biodivers. Bioavail. 2007, 1, 56–71. [Google Scholar]
- Azer, S. Taxonomic revision of genus Lemna L. (Lemnaceae Gray) in Egypt. Ann. Agric. Sci. 2013, 58, 257–263. [Google Scholar] [CrossRef] [Green Version]
- Tang, Y.; Chen, L.; Wei, X.; Yao, Q.; Li, T. Removal of lead ions from aqueous solution by the dried aquatic plant, Lemna perpusilla Torr. J. Hazard. Mater. 2013, 244, 603–612. [Google Scholar] [CrossRef]
- Reyes-Ledezma, J.L.; Uribe-Ramírez, D.; Cristiani-Urbina, E.; Morales-Barrera, L. Biosorptive removal of acid orange 74 dye by HCl-pretreated Lemna sp. PLoS ONE 2020, 15, e0228595. [Google Scholar] [CrossRef] [PubMed]
- Horwitz, W.; Latimer, G.W., Jr. Official Methods of Analysis of AOAC International, 18th ed.; AOAC International: Gaithersburg, MD, USA, 2005. [Google Scholar]
- Filipović-Kovačević, Ž.; Sipos, L.; Briški, F. Biosorption of chromium, copper, nickel and zinc ions onto fungal pellets of Aspergillus niger 405 from aqueous solutions. Food Technol. Biotechnol. 2000, 38, 211–216. [Google Scholar]
- Gupta, V.K.; Suhas; Nayak, A.; Agarwal, S.; Chaudhary, M.; Tyagi, I. Removal of Ni (II) ions from water using scrap tire. J. Mol. Liq. 2014, 190, 215–222. [Google Scholar] [CrossRef]
- Netzahuatl-Muñoz, A.R.; Cristiani-Urbina, M.D.C.; Cristiani-Urbina, E. Chromium Biosorption from Cr(VI) Aqueous Solutions by Cupressus lusitanica Bark: Kinetics, Equilibrium and Thermodynamic Studies. PLoS ONE 2015, 10, e0137086. [Google Scholar] [CrossRef] [Green Version]
- Oliveira, E.; Montanher, S.; Andrade, A.; Nóbrega, J.D.A.; Rollemberg, M. Equilibrium studies for the sorption of chromium and nickel from aqueous solutions using raw rice bran. Process. Biochem. 2005, 40, 3485–3490. [Google Scholar] [CrossRef]
- Febrianto, J.; Kosasih, A.N.; Sunarso, J.; Ju, Y.-H.; Indraswati, N.; Ismadji, S. Equilibrium and kinetic studies in adsorption of heavy metals using biosorbent: A summary of recent studies. J. Hazard. Mater. 2009, 162, 616–645. [Google Scholar] [CrossRef]
- Vijayaraghavan, K.; Padmesh, T.; Palanivelu, K.; Manikam, V. Biosorption of nickel(II) ions onto Sargassum wightii: Application of two-parameter and three-parameter isotherm models. J. Hazard. Mater. 2006, 133, 304–308. [Google Scholar] [CrossRef] [PubMed]
- Aksu, Z.; Açikel, Ü.; Kabasakal, E.; Tezer, S. Equilibrium modelling of individual and simultaneous biosorption of chromium(VI) and nickel(II) onto dried activated sludge. Water Res. 2002, 36, 3063–3073. [Google Scholar] [CrossRef]
- Srivastava, V.C.; Mall, I.D.; Mishra, I.M. Equilibrium modelling of single and binary adsorption of cadmium and nickel onto bagasse fly ash. Chem. Eng. J. 2006, 117, 79–91. [Google Scholar] [CrossRef]
- McKay, G.; Porter, J. A Comparison of Langmuir Based Models for Predicting Multicomponent Metal Ion Equilibrium Sorption Isotherms on Peat. Process. Saf. Environ. Prot. 1997, 75, 171–180. [Google Scholar] [CrossRef]
- Luna, A.S.; Costa, A.L.H.; Da Costa, A.C.A.; Henriques, C.A. Competitive biosorption of cadmium(II) and zinc(II) ions from binary systems by Sargassum filipendula. Bioresour. Technol. 2010, 101, 5104–5111. [Google Scholar] [CrossRef]
- Aksu, Z.; Akpinar, D. Competitive biosorption of phenol and chromium(VI) from binary mixtures onto dried anaerobic activated sludge. Biochem. Eng. J. 2001, 7, 183–193. [Google Scholar] [CrossRef]
- Muhammad; Chuah, T.; Robiah, Y.; Suraya, A.; Choong, T. Single and binary adsorptions isotherms of Cd(II) and Zn(II) on palm kernel shell based activated carbon. Desalin. Water Treat. 2011, 29, 140–148. [Google Scholar] [CrossRef]
- Guerrero-Coronilla, I.; Aranda-Garcia, E.; Cristiani-Urbina, E. Biosorption of Metanil Yellow Dye from Aqueous Solutions by the Entire Water Hyacinth Plant (Eichhornia crassipes) and Its Vegetative Organs. Environ. Eng. Manag. J. 2019, 18, 1671–1682. [Google Scholar] [CrossRef]
- Hach Company. Hach Water Analysis Handbook; Hach Company: Hach, Loveland, 2008. [Google Scholar]
- Schmid, R.; Landolt, E. Biosystematic Investigations in the Family of Duckweeds (Lemnaceae). TAXON 1987, 36, 781. [Google Scholar] [CrossRef]
- Landesman, L. Effects of herbivory and competition of growth of Lemnaceae in systems for wastewater treatment and livestock feed production. Ph.D. Thesis, University of Louisiana, Lafayette, LA, USA, 2000; p. 150. [Google Scholar]
- Salam, O.E.A.; Reiad, N.A.; Elshafei, M.M. A study of the removal characteristics of heavy metals from wastewater by low-cost adsorbents. J. Adv. Res. 2011, 2, 297–303. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.; Fan, T.; Zeng, G.-M.; Li, X.; Tong, Q.; Ye, F.; Zhou, M.; Xu, W.-H.; Huang, Y.-E. Removal of cadmium and zinc ions from aqueous solution by living Aspergillus niger. Trans. Nonferrous Met. Soc. China 2006, 16, 681–686. [Google Scholar] [CrossRef]
- Saygideger, S.; Gülnaz, O.; Istifli, E.S.; Yucel, N. Adsorption of Cd(II), Cu(II) and Ni(II) ions by Lemna minor L.: Effect of physicochemical environment. J. Hazard. Mater. 2005, 126, 96–104. [Google Scholar] [CrossRef]
- Quintelas, C.; Rocha, Z.; Silva, B.; Fonseca, B.; Figueiredo, H.; Tavares, M. Removal of Cd(II), Cr(VI), Fe(III) and Ni(II) from aqueous solutions by an E. coli biofilm supported on kaolin. Chem. Eng. J. 2009, 149, 319–324. [Google Scholar] [CrossRef] [Green Version]
- Şengil, I.A.; Ozacar, M. Competitive biosorption of Pb2+, Cu2+ and Zn2+ ions from aqueous solutions onto valonia tannin resin. J. Hazard. Mater. 2009, 166, 1488–1494. [Google Scholar] [CrossRef]
- Sağ, Y.; Akçael, B.; Kutsal, T. Evaluation, interpretation, and representation of three-metal biosorption equilibria using a fungal biosorbent. Process. Biochem. 2001, 37, 35–50. [Google Scholar] [CrossRef]
- Sedlakova-Kadukova, J.; Horváthová, H. Biosorption of Copper, Zinc and Nickel From Multi-Ion Solutions. Nova Biotechnol. Chim. 2012, 11, 125–132. [Google Scholar] [CrossRef] [Green Version]
- Li, C.; Li, D.; Feng, J.; Fan, X.; Chen, S.; Zhang, D.; He, R. Duckweed (Lemna minor) is a novel natural inducer of cellulase production in Trichoderma reesei. J. Biosci. Bioeng. 2019, 127, 486–491. [Google Scholar] [CrossRef] [PubMed]
- Zhang, S.; Chen, T.; Li, W.; Dong, Q.; Xiong, Y. Physicochemical properties and combustion behavior of duckweed during wet torrefaction. Bioresour. Technol. 2016, 218, 1157–1162. [Google Scholar] [CrossRef] [PubMed]
- Blanes, P.S.; Bordoni, M.E.; Gonzalez, J.C.; García, S.I.; Atria, A.M.; Sala, L.F.; Bellú, S.E. Application of soy hull biomass in removal of Cr(VI) from contaminated waters. Kinetic, thermodynamic and continuous sorption studies. J. Environ. Chem. Eng. 2016, 4, 516–526. [Google Scholar] [CrossRef]
- Kuppusamy, S.; Thavamani, P.; Megharaj, M.; Venkateswarlu, K.; Lee, Y.B.; Naidu, R. Oak (Quercus robur) Acorn Peel as a Low-Cost Adsorbent for Hexavalent Chromium Removal from Aquatic Ecosystems and Industrial Effluents. Water Air Soil Pollut. 2016, 227, 62. [Google Scholar] [CrossRef]








| Initial Zn2+ Concentration (mM) | ξNi (%) |
|---|---|
| 0.2 | −2.096 |
| 0.4 | −8.474 |
| 1 | −19.157 |
| 2 | −30.772 |
| 4 | −43.319 |
| 6 | −52.371 |
| 10 | −60.335 |
| C0Zn (mM) | C0Ni (mM) | ||||||
|---|---|---|---|---|---|---|---|
| 0.2 | 0.4 | 1 | 2 | 4 | 6 | 10 | |
| 0.2 | 0.69 | 0.68 | 0.79 | 0.98 | 0.99 | 0.99 | 0.98 |
| 0.4 | 0.52 | 0.42 | 0.67 | 0.90 | 0.95 | 0.92 | 0.94 |
| 1 | 0.43 | 0.31 | 0.61 | 0.76 | 0.86 | 0.84 | 0.79 |
| 2 | 0.26 | 0.24 | 0.49 | 0.63 | 0.74 | 0.74 | 0.68 |
| 4 | 0.12 | 0.10 | 0.36 | 0.54 | 0.61 | 0.59 | 0.55 |
| 6 | 0.02 | 0.07 | 0.24 | 0.40 | 0.55 | 0.50 | 0.47 |
| 10 | 0.01 | 0.05 | 0.16 | 0.30 | 0.44 | 0.44 | 0.39 |
| Initial Ni2+ Concentration (mM) | ξZn (%) |
|---|---|
| 0.2 | −0.127 |
| 0.4 | −0.374 |
| 1 | −2.171 |
| 2 | −2.507 |
| 4 | −8.548 |
| 6 | −12.079 |
| 10 | −16.536 |
| C0Ni (mM) | C0Zn (mM) | ||||||
|---|---|---|---|---|---|---|---|
| 0.2 | 0.4 | 1 | 2 | 4 | 6 | 10 | |
| 0.2 | 0.93 | 0.96 | 0.96 | 1.00 | 1.00 | 1.00 | 1.00 |
| 0.4 | 0.50 | 1.00 | 0.98 | 0.97 | 1.00 | 1.00 | 0.99 |
| 1 | 0.32 | 0.88 | 0.96 | 0.98 | 0.95 | 1.00 | 1.00 |
| 2 | 0.24 | 0.68 | 0.94 | 0.99 | 0.94 | 1.00 | 1.00 |
| 4 | 0.19 | 0.61 | 0.74 | 0.80 | 0.91 | 0.97 | 0.99 |
| 6 | 0.04 | 0.48 | 0.54 | 0.71 | 0.89 | 0.95 | 1.00 |
| 10 | 0.02 | 0.33 | 0.44 | 0.61 | 0.80 | 0.94 | 1.00 |
| Model | Ni2+ | Zn2+ |
|---|---|---|
| Langmuir | ||
| qmax (mmol g−1) | 0.982 ± 0.074 | 0.852 ± 0.022 |
| BL (L mmol−1) | 0.231 ± 0.053 | 0.875 ± 0.112 |
| R2 | 0.965 | 0.9885 |
| SSE | 0.029 | 0.011 |
| Sy.x | 0.060 | 0.0352 |
| RMSE | 0.057 | 0.0333 |
| Freundlich | ||
| KF (mmol g−1) (mmol L−1)−1/nF | 0.253 ± 0.007 | 0.382 ± 0.028 |
| nF | 2.587 ± 0.069 | 3.705 ± 0.388 |
| R2 | 0.997 | 0.961 |
| SSE | 0.002 | 0.037 |
| Sy.x | 0.017 | 0.064 |
| RMSE | 0.016 | 0.061 |
| Sips | ||
| qmax (mmol g−1) | 2.849 ± 0.744 | 0.974 ± 0.040 |
| Bs (mmol L−1)-nS | 0.095 ± 0.026 | 0.677 ± 0.061 |
| nS | 0.476 ± 0.03 | 0.710 ± 0.051 |
| R2 | 0.999 | 0.997 |
| SSE | 0.001 | 0.003 |
| Sy.x | 0.011 | 0.019 |
| RMSE | 0.010 | 0.017 |
| Redlich–Peterson | ||
| KRP (L g−1) | 1.886 ± 0.523 | 1.234 ± 0.098 |
| ARP (mmol L−1)-BRP | 6.456 ± 2.038 | 2.028 ± 0.222 |
| bRP | 0.655 ± 0.012 | 0.885 ± 0.011 |
| R2 | 0.999 | 0.999 |
| SSE | 0.001 | 0.001 |
| Sy.x | 0.010 | 0.011 |
| RMSE | 0.009 | 0.010 |
| Ni2+ | Zn2+ | Ni2+ | Zn2+ | ||
|---|---|---|---|---|---|
| Non-modified competitive Langmuir | Modified Sips | ||||
| qmaxNi | 0.982 ± 0.074 | qmax Ni | 0.750 ± 0.048 | ||
| qmaxZn | 0.852 ± 0.022 | BS Ni | 0.488 ± 0.063 | 0.306 ± 0.115 | |
| BLNi | 0.231 ± 0.053 | 0.231 ± 0.053 | nS Ni | 0.903 ± 0.048 | 0.970 ± 0.165 |
| BLZn | 0.875 ± 0.112 | 0.875 ± 0.112 | qmax Zn | 0.764 ± 0.018 | |
| R2 | 0.935 | 0.985 | BS Zn | 0.941 ± 0.151 | 1.202 ± 0.123 |
| SSE | 0.122 | 0.067 | nS Zn | 0.785 ± 0.050 | 1.195 ± 0.059 |
| Sy.x | 0.045 | 0.033 | R2 | 0.988 | 0.989 |
| RMSE | 0.044 | 0.032 | SSE | 0.022 | 0.050 |
| Sy.x | 0.019 | 0.029 | |||
| Modified Langmuir with interaction factor η | RMSE | 0.018 | 0.028 | ||
| qmaxNi | 0.982 ± 0.074 | ||||
| qmaxZn | 0.852 ± 0.022 | Non-modified competitive Redlich-Peterson | |||
| BLNi | 0.231 ± 0.053 | 0.231 ± 0.053 | KRP Ni | 1.886 ± 0.523 | |
| BLZn | 0.875 ± 0.112 | 0.875 ± 0.112 | ARP Ni | 6.456 ± 2.038 | 6.456 ± 2.038 |
| η Ni | 1.076 ± 0.049 | 1.237 ± 0.161 | bRP Ni | 0.655 ± 0.012 | 0.655 ± 0.012 |
| η Zn | 1.809 ± 0.206 | 1.041 ± 0.046 | KRP Zn | 1.234 ± 0.098 | |
| R2 | 0.960 | 0.986 | ARP Zn | 2.028 ± 0.222 | 2.028 ± 0.222 |
| SSE | 0.075 | 0.063 | bRP Zn | 0.885 ± 0.011 | 0.885 ± 0.011 |
| Sy.x | 0.035 | 0.032 | R2 | 0.903 | 0.440 |
| RMSE | 0.034 | 0.031 | SSE | 0.182 | 2.526 |
| Sy.x | 0.055 | 0.207 | |||
| Modified competitive Langmuir | RMSE | 0.054 | 0.200 | ||
| qmax Ni′ | 0.675 ± 0.02 | ||||
| qmax Zn′ | 0.832 ± 0.015 | Modified Redlich–Peterson with interaction | |||
| BNi′ | 0.523 ± 0.045 | 0.194 ± 0.026 | factor η | ||
| BZn′ | 0.74 ± 0.066 | 0.908 ± 0.063 | KRP Ni | 1.886 ± 0.523 | |
| R2 | 0.985 | 0.986 | ARP Ni | 6.456 ± 2.038 | 6.456 ± 2.038 |
| SSE | 0.028 | 0.061 | bRP Ni | 0.655 ± 0.012 | 0.655 ± 0.012 |
| Sy.x | 0.021 | 0.032 | KRP Zn | 1.234 ± 0.098 | |
| RMSE | 0.021 | 0.031 | ARP Zn | 2.028 ± 0.222 | 2.028 ± 0.222 |
| bRP Zn | 0.885 ± 0.011 | 0.885 ± 0.011 | |||
| Extended Freundlich | η Ni | 1.019 ± 0.04 | 28.292 ± 6.56 | ||
| KF Ni | 0.253 ± 0.007 | η Zn | 0.382 ± 0.036 | 0.868 ± 0.054 | |
| nF Ni | 2.587 ± 0.069 | R2 | 0.984 | 0.983 | |
| KF Zn | 0.382 ± 0.028 | SSE | 0.031 | 0.077 | |
| nF Zn | 3.705 ± 0.388 | Sy.x | 0.022 | 0.035 | |
| xNi | 0.687 ± 0.067 | RMSE | 0.022 | 0.034 | |
| yNi | 0.759 ± 0.075 | ||||
| zNi | 0.823 ± 0.055 | ||||
| xZn | 2.142 ± 0.285 | ||||
| yZn | 0.074 ± 0.027 | ||||
| zZn | 1.249 ± 0.211 | ||||
| R2 | 0.980 | 0.978 | |||
| SSE | 0.037 | 0.095 | |||
| Sy.x | 0.025 | 0.040 | |||
| RMSE | 0.024 | 0.039 | |||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Morales-Barrera, L.; Flores-Ortiz, C.M.; Cristiani-Urbina, E. Single and Binary Equilibrium Studies for Ni2+ and Zn2+ Biosorption onto Lemna gibba from Aqueous Solutions. Processes 2020, 8, 1089. https://doi.org/10.3390/pr8091089
Morales-Barrera L, Flores-Ortiz CM, Cristiani-Urbina E. Single and Binary Equilibrium Studies for Ni2+ and Zn2+ Biosorption onto Lemna gibba from Aqueous Solutions. Processes. 2020; 8(9):1089. https://doi.org/10.3390/pr8091089
Chicago/Turabian StyleMorales-Barrera, Liliana, César Mateo Flores-Ortiz, and Eliseo Cristiani-Urbina. 2020. "Single and Binary Equilibrium Studies for Ni2+ and Zn2+ Biosorption onto Lemna gibba from Aqueous Solutions" Processes 8, no. 9: 1089. https://doi.org/10.3390/pr8091089
APA StyleMorales-Barrera, L., Flores-Ortiz, C. M., & Cristiani-Urbina, E. (2020). Single and Binary Equilibrium Studies for Ni2+ and Zn2+ Biosorption onto Lemna gibba from Aqueous Solutions. Processes, 8(9), 1089. https://doi.org/10.3390/pr8091089