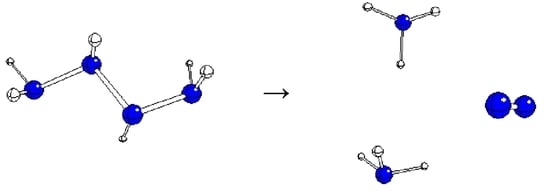
Hydrazine Oxidation in Aqueous Solutions I: N4H6 Decomposition

Breza, Martin; Manova, Alena

doi:10.3390/inorganics11100413

Open AccessArticle

Hydrazine Oxidation in Aqueous Solutions I: N₄H₆ Decomposition

by

Martin Breza

^1,*

and

Alena Manova

²

¹

Department of Physical Chemistry, Slovak Technical University, Radlinskeho 9, SK-81237 Bratislava, Slovakia

²

Department of Analytical Chemistry, Slovak Technical University, Radlinskeho 9, SK-81237 Bratislava, Slovakia

^*

Author to whom correspondence should be addressed.

Inorganics 2023, 11(10), 413; https://doi.org/10.3390/inorganics11100413

Submission received: 29 September 2023 / Revised: 14 October 2023 / Accepted: 17 October 2023 / Published: 18 October 2023

(This article belongs to the Special Issue 10th Anniversary of Inorganics: Inorganic Materials)

Download

Browse Figures

Versions Notes

Abstract

:

A mixture of nonlabeled (¹⁴N₂H₄) and ¹⁵N labeled hydrazine (¹⁵N₂H₄) in an aqueous solution is oxidized to ¹⁵N₂, ¹⁴N₂, and ¹⁴N¹⁵N molecules, indicating the intermediate existence of the ¹⁴NH₂-¹⁴NH-¹⁵NH-¹⁵NH₂ with subsequent hydrogen transfers and splitting of side N-N bonds. The structures, thermodynamics and electron characteristics of various N₄H₆ molecules in aqueous solutions are investigated using theoretical treatment at the CCSD/cc-pVTZ level of theory to explain the crucial part of the hydrazine oxidation reaction. Most N₄H₆ structures in aqueous solutions are decomposed during geometry optimization. Splitting the bond between central nitrogen atoms is the most frequent method, but the breakaway of the side nitrogen is energetically the most preferred one. The N-N fissions are enabled by suitable hydrogen rearrangements. Gibbs free energy data indicate the dominant abundance of NH₃... N₂... NH₃ species. The side N atoms have very high negative charges, which should support hydrogen transfers in aqueous solutions. The only stable cyclo-(NH)₄…H₂ structure has a Gibbs energy that is too high and breaks the H₂ molecule. The remaining initial cyclic structures are split into hydrazine and HN≡NH or H₂N≡N species, and their relative abundance in aqueous solutions is vanishing.

Keywords:

Coupled Cluster; geometry optimization; N-N bond splitting; QTAIM analysis; electron structure

Graphical Abstract

1. Introduction

Hydrazine N₂H₄ is a colorless flammable liquid that is used in industry and agriculture due to its reducing properties. It is used as a corrosion inhibitor in boilers, as a rocket propellant, antioxidant, catalyst, and pesticide precursor. In boiler water, it serves as an oxygen scavenger that reacts with oxygen into nitrogen and water only, which does not cause corrosion of ferrous metals. Unreacted hydrazine can be decomposed into ammonia, which can be corrosive to copper and copper-containing alloys [1]. Thus, the knowledge of the exact mechanism of its oxidation is of practical importance so far.

Higginson and Sutton [2] studied the oxidation of ¹⁵N-enriched hydrazine by an excess of various oxidizing agents in aqueous solutions. Mass spectroscopic analysis of the evolved nitrogen for 28, 29 and 30 mass-number abundance (i.e., incidence of ¹⁴N₂, ¹⁵N¹⁴N and ¹⁵N₂ molecules, respectively) has shown that the proportion of ¹⁵N₂ molecules decreased while that of ¹⁵N¹⁴N molecules increased depending on the oxidizing agent used. If the nitrogen produced by the reaction

2 N₂H₄ → N₂ + 2 NH₃

(1)

involves no N-N fission, the evolved N₂ molecule originates in the same N₂H₄ molecule, and therefore it must have the same distribution of ¹⁵N isotopes as the hydrazine reactant. This implies that some of the nitrogen molecules are formed by a mechanism involving a N-N fission and the formation of nitrogen-containing radicals from two different hydrazine molecules as follows:

¹⁵NH₂-¹⁵NH₂ + ¹⁴NH₂-¹⁴NH₂ → ¹⁵NH₂-¹⁵NH• + •¹⁴NH-¹⁴NH₂ → ¹⁵NH₂-¹⁵NH-¹⁴NH-¹⁴NH₂

(2)

¹⁵NH₂-¹⁵NH-¹⁴NH-¹⁴NH₂ → ¹⁵NH₃ + ¹⁵NH = ¹⁴N-¹⁴NH₂ → ¹⁵NH₃ + ¹⁵N¹⁴N + ¹⁴NH₃

(3)

¹⁵NH₂-¹⁵NH-¹⁴NH-¹⁴NH₂ → ¹⁵NH₂-¹⁵N = ¹⁴NH + ¹⁴NH₃ → ¹⁵NH₃ + ¹⁵N¹⁴N + ¹⁴NH₃

(4)

Cahn and Powell [3] confirmed the randomized ¹⁵N¹⁴N composition obtained by one-electron oxidation of ¹⁵N enriched hydrazines with a number of oxidizing agents unlike exclusively four-electron oxidizing agents (acid iodate, alkaline ferricyanide) that produced unrandomized N₂ molecules (all four hydrogen atoms must be removed from a single hydrazine molecule). Petek and Bruckenstein [4] observed that the electrooxidation of ¹⁵N labelled hydrazine (96.7% enrichment) at the Pt electrode produced N₂ molecules with the ratio of ¹⁴N¹⁵N/¹⁵N¹⁵N = 0.07 ± 0.01 while in Ce(IV) solutions it was 0.9 ± 0.2. A ratio of both isotopic forms between these two limits was produced by simultaneous electrooxidation and homogeneous oxidation with electrogenerated Ce(IV).

A bright yellow substance, stable under −178 °C, is formed after thermal decomposition of hydrazine at high temperatures (~850 °F) and low pressures (~0.5 mm Hg) in a flowing system [5]. The authors suppose that it is tetrazane N₄H₆.

Based on polarographic and voltammetric studies of hydrazine in alkali solutions, Karp and Meites [6] suggested its two-electron oxidation to diimide with subsequent dimerization and decomposition as follows

2 N₂H₂ → N₄H₄ → NH₄⁺ + N₃⁻

(5)

The proposed mechanism is also capable of explaining the randomized ¹⁵N¹⁴N composition.

Ball [7] investigated the structure and some thermochemical properties of the cis- and trans-conformations of tetrazane NH₂-NH-NH-NH₂ using various-level ab initio methods. Unlike nearly planar trans-conformation, the cis-conformation should be denoted as a gauche structure (N-N-N-N dihedral angle of ca 90°).

The decomposition of hydrazine was studied at the CCSD(T)-F12a/aug-ccpVTZ//ωB97x-D3/6-311++G(3df,3pd) level of theory [8]. A comprehensive analysis of the N₄H₆ singlet potential energy surfaces was performed. Three stable isomers, NH₂-NH-NH-NH₂, NH₂-NH-NH₂=NH and NH₂-NH₂-N=NH_2, and the transition states for H transfers between them were obtained as well. Stabilized NH₂-NH-NH-NH₂ formation becomes significant only at relatively high pressures and low temperatures due to its decomposition into N₂H₃• + N₂H₃•. No direct reaction between NH₂-NH-NH-NH₂ and NH₂-NH₂-N=NH₂ was found. NH₃ eliminations from NH₂-NH-NH-NH₂ and NH₂-NH₂-N=NH₂ are energetically preferred, but only NH₂-NH₂-N=NH₂ has relatively small activation energy for this reaction (see Table 1).

It is evident that the decomposition of N₄H₆ is crucial for hydrazine oxidation with subsequent ¹⁵N¹⁴N molecule formation. It depends on the suitable N₄H₆ site of N-N bond splitting. At first, the NH₂-NH-NH-NH₂ isomer is formed by the reaction

N₂H₃• + N₂H₃• → NH₂-NH-NH-NH₂

(6)

In the next steps, H transfers and possible N-N bond splitting may proceed. The main aim of this study is a quantum-chemical study of N₄H₆ isomers in aqueous solutions solely at the Coupled Cluster level of theory and to determine the sites of the possible N-N fission within them. The thermodynamic properties of the decomposition reaction products enable us to predict the possible formation of ¹⁵N¹⁴N molecules in real systems. The electronic structure of the optimized structures will also be discussed.

2. Results and Discussion

We consider possible linear isomers of N₄H₆ with an N1-N2-N3-N4 backbone and the composition of N1H_m-N2H_n-N3H_p-N4H_q, where subscripts m, n, p and q denote the number of H atoms bonded to individual Ni; i = 1→ 4, atoms, and m + n + p + q = 6. We started geometry optimizations from anti- and syn-conformations of N1-N2-N3-N4. The optimized structures usually correspond to gauche conformers, or some N-N bonds are split (see Table 2). If N1 and N2 correspond to ¹⁵N atoms, while N3 and N4 correspond to the ¹⁴N ones, then N1-N2 and N3-N4 fissions would lead to ¹⁵N¹⁴N molecules, unlike the N2-N3 fissions.

In the case of cyclo-N₄H₆ isomers we can use the same notation, but any N-N fission can lead to ¹⁵N¹⁴N molecules because of suitable H transfers within the cycle.

We introduce the notation Xmnpq for the individual systems under study, where X = A, B and C, and D stands for anti-, syn-, cyclic and gauche-structures and the indices m, n, p and q are explained above. X = E denotes structures with N-N fissions, i.e., consisting of two or three molecules after geometry optimization. X = F stands for structures with N2-N3 fissions and subsequent N1-N4 bond formations. The N-N fissions in E and F systems are denoted by round brackets where the mutually bonded N atoms are included in the same bracket couple. The different structures with the same Xmnpq notation can be distinguished by additional letters a, b, c, etc. For example, E(22)(11)a and E(22)(11)b denote two different structures composed of H₂N-NH₂ and HN=NH molecules.

The N₄H₆ structures under study are shown in Table 2 and are divided into three groups according to the initial N1-N2-N3-N4 conformations. The H atom rearrangements during geometry optimizations are less frequent in the anti-conformations (starting A structures) than in the syn-conformations (starting B structures). In both groups the probability of N-N fissions is approximately 50%, and N2-N3 fissions prevail. On the other hand, the N1-N2 fissions lead to energetically preferred products such as E(3)(201), E(3)(102) and especially E(3)(00)(3). In the B1221 syn-conformation the mutual interaction of N1 and N4 causes the formation of the N1-N4 bond and N2-N3 fission leading to the structure of H₂N2-N1H-N4H-N3H₂, i.e., F12)(21, in gauche conformation or decomposition to more stable HN1=N4H and H₂N2-N3H₂ species denoted as the F1)(22)(1 system.

The relative Gibbs free energies in Table 2 are related to the structure D2112a obtained by the reaction (6) in the first step. According to these data, the system E(3)(00)(3), which corresponds to ¹⁵NH₃, ¹⁴NH₃ and ¹⁵N¹⁴N molecules, is dominant among all N₄H₆ structures in aqueous solutions under normal conditions and the relative abundance of the remaining systems vanishes. In general, the decomposed E systems are more stable than the remaining structures (see Table 2, Table 3 and Table 4, Figure 1 and Figure 2).

During the geometry optimization of the starting cyclic C structures (Table 2), only the least stable cyclo-(NH)₄ structure, denoted as E1111, preserves its tetraatomic ring after removing an H₂ molecule. The remaining C structures split into hydrazine and HN=NH in E(22)(11)d or H₂N=N in E(22)(02). The disadvantage of cyclic structure preservation is indicated by preferring the above-mentioned F structures after N1-N4 bonding within geometry optimization of the starting B1221 syn-conformation.

The bonding within N₄H₆ structures can be described by individual bond lengths d (Table 3 and Table 4) as well as by the corresponding electron density ρ (Table 5 and Table 6) and ellipticity ε (Table 7 and Table 8) at their bond-critical points (BCP) [9]. Bond strengths decrease with bond lengths d and increase with their BCP electron densities ρ_BCP. Their double bond character in acyclic structures increases with their BCP ellipticities ε_BCP.

The D1221 structure has an extremely long N2-N3 bond, and the remaining N-N bonds are shorter than the average N₄H₆ ones. The ρ_BCP(N2-N3)~0.1 e/Bohr³ corresponds to a very weak bond, and the remaining N-N bonds are approximately three times stronger. The ε_BCP(N2-N3)~0.1 is relatively high, and the remaining double N-N bonds have a ca. two times higher ellipticity.

The D2112a-c structures differ in N1-N2-N3-N4 dihedral angles, and their bond length alternation decreases with non-planarity of their backbone. Their ρ_BCP(N-N) values vary by about ~0.3 e/Bohr³, as in single N-N bonds. The ε_BCP(N2-N3) values decrease with non-planarity (~0.1 and less), while they are very small for the remaining N-N bonds, which correspond to single bonds.

Similarly, the D2121a-d structures differ in the N1-N2-N3-N4 dihedral angles, with the N2-N3 bond length being longer and weaker than the remaining ones’. The ρ_BCP(N-N) values that vary by about ~0.3 e/Bohr³ correspond to single N-N bonds. The ε_BCP(N2-N3) values decrease with non-planarity (~0.1 and less); ε_BCP(N3-N4)~0.2 is typical for double bonds.

The N-N bond properties in the A2202, D2202a-b and D2022 structures (aside from reverse numbering of N atoms) vary with the N1-N2-N3-N4 dihedral angles. The N2-N3 bonds are the shortest in all these systems. The ρ_BCP(N-N) values that vary by about ~0.3 e/Bohr³ are typical for single N-N bonds but the ε_BCP(N2-N3)~0.3 in all structures indicate the double-bond character of this bond.

In A3021 the N-N bond lengths decrease with the distance from N1, and the BCP ellipticity values indicate the same trend in decreasing double-bond character. However, the ρ_BCP(N-N) values of about 0.3 e/Bohr³ correspond to single N-N bonds.

Analogous trends are observed for D3012a-b structures.

In E1111 with N-N bond lengths of ca. 1.5 Å and ρ_BCP(N-N)~0.3 e/Bohr³ typical for single N-N bonds, the ε_BCP(N-N) values of 0.108 can be explained by mechanical strain in its four-membered ring rather than by its double-bond character.

The remaining E systems consist of two or three independent molecules, interacting through weak hydrogen bonds only, which can be treated independently of their parent E structures. The possible biradical character of E(32)(10) can be excluded on the basis of its atomic charges (see later) which indicate the existence of [NH₃-NH₂]⁺ and [HN≡N]⁻ charged species.

H₂N-NH₂ with an N-N distance of 1.45 Å, ρ_BCP(N-N) = 0.295 e/Bohr³ and ε_BCP(N-N) = 0.008 in all E systems is typical for a single N-N bond.

HN=NH with an N-N distance of 1.245 Å, ρ_BCP(N-N) = 0.486 e/Bohr³ and ε_BCP(N-N) = 0.189 in all E systems corresponds to the double N-N bond.

Its isomer H₂N=N has a N-N distance of 1.23 Å and ρ_BCP(N-N) = 0.497 e/Bohr³ which correspond to the double N-N bond in contradiction with ε_BCP(N-N) = 0.020, which corresponds to single or triple bonds.

On the other hand, NH₃-NH has a N-N distance of 1.47 Å and ρ_BCP(N-N) = 0.27 e/Bohr³, which corresponds to the single N-N bond in contrast to the high ε_BCP(N-N) value of 0.156.

The [NH₃-NH₂]⁺ cation with an N-N distance of 1.446 Å, ρ_BCP(N-N) = 0.293 e/Bohr³ and ε_BCP(N-N) = 0.089 corresponds to a single N-N bond.

Its counterpart [HN≡N]⁻ has a N-N distance of 1.242 Å and ρ_BCP(N-N) = 0.48 e/Bohr³ which correspond to the double N-N bond in contradiction with its too low ε_BCP(N-N) = 0.072.

N₂ has a N-N distance of 1.096 Å, ρ_BCP(N-N) = 0.714 e/Bohr³ and ε_BCP(N-N) = 0.000, which is typical for the triple bond.

Finally, H₂N-N=NH with N-N distances of 1.36 and 1.24 Å, ρ_BCP(N-N) values of 0.37 and 0.48 e/Bohr³ as well as ε_BCP(N-N) values of 0.23 and 0.13, respectively, probably correspond to nearly-double N-N bonds.

The F(11)(22) system is explained within the HN≡NH and H₂N-NH₂ structures above.

The F12)(21 structure H₂N2-N1H-N4H-N3H₂ (aside from different numbering of N atoms) corresponds to the D2112 structures explained above.

We have not discussed N-H bonding in the systems under study because the differences in their bond lengths and BCP electron densities are too small. However, their BCP electron densities are higher than those of N-N bonds except HN=NH, H₂N=N, [HN≡N]⁻ and N₂. Increased ε_BCP(N-H) values can mostly be ascribed to the double-bond character of neighboring N-N bonds, except ε_BCP(N-H) = 0.3 in NH₃ molecules within the E(3)(102) systems.

The nitrogen atomic charges in the A and D structures (Table 9) on the N1 and N4 atoms are more negative (−0.65 to −0.82) than on the central N2 and N3 atoms (−0.35 to −0.50). Positive hydrogen atomic charges bonded to side N1 and N4 atoms increase with the number of bonded H atoms. The same trend holds for H atoms bonded to central N2 and N3 atoms which are more positive than the side hydrogens.

In the decomposed E systems (Table 10), negative N charges increase with the number of bonded H atoms. An analogous trend for positive H charges cannot be confirmed. Atomic charges are only slightly affected by hydrogen bonding. In the E(32)(10) system, the charges of its [NH₃-NH₂]⁺ and [HN≡N]⁻ subsystems are +0.97 and −0.68, respectively (the ideal charges are +1.00 and −1.00, respectively). The errors can be ascribed to numerical integration of electron density up to 0.001 e/Bohr³ (instead of 0.000 e/Bohr³). A significantly higher error of [HN≡N]⁻ is caused by the higher diffusive character of the electron density of anionic species. When accounting for the errors in the electron density integration over atomic basins, the alternative biradical structure of the neutral E(32)(10) subsystems (the ideal charges of both species should be 0.00) seems to be less probable.

3. Method

Geometry optimizations for various isomers of neutral N₄H₆ molecules were performed at the CCSD (Coupled Cluster using Single and Double substitutions from the Hartree-Fock determinant) [10] level of theory and cc-pVTZ basis sets [11]. The effects of the aqueous solution were taken into account within the SMD (Solvation Model based on the solute electron Density) solvation model [12]. The optimized structures were tested by vibrational analysis for the absence of imaginary vibrations. Gaussian16 (Revision B.01) software [13] was used for all quantum-chemical calculations.

The electron structures of the systems under study were evaluated in terms of Quantum Theory of Atoms-in-Molecules (QTAIM) [9] using AIM2000 (Version 1.0) software [14]. The bond strengths were compared according to the electron densities ρ at the bond-critical points (BCP). The BCP bond ellipticities ε_BCP were evaluated as

ε_BCP = λ₁/λ₂ − 1

(7)

where λ_i are the eigenvalues of the Hessian of the BCP electron density within the sequence λ₁ < λ₂ < 0 < λ₃. Atomic charges were obtained by integration over atomic basins up to 0.001 e/Bohr³.

Visualization and geometry modification were performed using MOLDRAW (Release 2.0) software (https://www.moldraw.software.informer.com, accessed on 9 September 2019) [15].

4. Conclusions

We have shown that most N₄H₆ structures in aqueous solutions are decomposed during geometry optimization. Splitting the bond between central nitrogen atoms is the most frequent method, but the breakaway of the side nitrogen is energetically the most preferred one. The N-N fissions are enabled by suitable hydrogen rearrangements. The initial H₂N-NH-NH-NH₂ structure (D2112) has a very weak central N-N bond, which explains the high degree of reversibility for the reaction (6). The most stable system NH₃…N₂…NH₃ (E(3)(00)(3) system) might be obtained by transfers of both H atoms bonded with central nitrogens to the side N atoms. According to [8], such double H transfer was not found by quantum-chemical calculations in vacuo, and so must be decomposed into several steps and this instantaneous decomposition should be slowed down. Furthermore, our calculations show that the transfer of the third H atom to the side nitrogen is very energetically disadvantageous, as indicated by the Gibbs energies of the structures NH₃-N=NH₂-NH and NH₃-N=NH-NH₂ (A3021 and D3012, respectively, see Table 2). In aqueous solutions, H atom transfers can be mediated by H₂O, H₃O⁺ and/or OH⁻ species. We have shown that side N atoms have very high negative charges that should support such hydrogen transfers.

The experimentally observed formation of ¹⁵N¹⁴N molecules [1,2,3,4] is enabled by side N-N fissions. We have shown that the Gibbs free energy data (Table 2) indicate the dominant abundance of the NH₃... N₂... NH₃ species (E(3)(00)(3) system) in aqueous solutions, which explains the mentioned observations.

The ¹⁵N¹⁴N molecules can also be created by the decomposition of cyclic N₄H₆ structures. We have shown the high instability of such species. The only stable cyclo-(NH)₄…H₂ structure (E1111) has a too-high Gibbs energy and breaks the H₂ molecule instead. The remaining initial cyclic structures are split into hydrazine and HN≡NH (E(22)(11)d) or H₂N≡N species (E(22)(02), see Table 2), and their relative abundance in aqueous solutions vanishes.

We can deduce from the QTAIM analysis of our systems that single, double and triple N-N bonds exhibit BCP electron densities of ca. 0.2, 0.5 and 0.7 e/Bohr³ with BCP ellipticities of ca 0, 0.2 and 0, respectively. The bonds in the N₄H₆ structures often exhibit significant deviations from these values.

Our study did not solve all of the problems related to hydrazine oxidation in aqueous solutions. The role of various water forms and the corresponding transition states should also be investigated. The transition states can possibly be of extremely high-energy. Thus, the thermodynamic stability of the products means less if their formation is kinetically hindered. Moreover, directly accounting for the solvent molecules is required. An alternative reaction pathway through N₄H₄ [6] according to reaction (5) is worth studying as well. Further theoretical studies in these fields are desirable.

Author Contributions

Methodology, software, investigation, writing—original draft preparation, writing—review and editing, M.B.; conceptualization, supervision, project administration, funding acquisition, A.M. All authors have read and agreed to the published version of the manuscript.

Funding

This publication was supported by the Competence Center for SMART Technologies for Electronics and Informatics Systems and Services under the project no. ITMS 26240220072.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

All necessary research data are presented in the article.

Acknowledgments

M.B. thanks the HPC center at the Slovak University of Technology in Bratislava, which is a part of the Slovak Infrastructure of High Performance Computing (SIVVP Project No. 26230120002, funded by the European Region Development Funds), for computing facilities.

Conflicts of Interest

The authors declare no conflict of interest.

References

Lauko, L.; Hudec, R.; Lenghartova, K.; Manova, A.; Cacho, F.; Beinrohr, E. Simple Electrochemical Determination of Hydrazine in Water. Pol. J. Environ. Stud. 2015, 24, 1659–1666. [Google Scholar] [CrossRef] [PubMed]
Higginson, W.C.E.; Sutton, D. The Oxidation of Hydrazine in Aqueous Solution. Part II. The Use of ¹⁵N as a Tracer in the Oxidation of Hydrazine. J. Chem. Soc. 1953, 1402–1406. [Google Scholar] [CrossRef]
Cahn, J.W.; Powell, R.E. Oxidation of Hydrazine in Solution. J. Am. Chem. Soc. 1954, 76, 2568–2572. [Google Scholar] [CrossRef]
Petek, M.; Bruckenstein, S. An Isotopic Labeling Investigation of the Mechanism of the Electrooxidation of Hydrazine at Platinum. An Electrochemical Mass Spectrometric Study. Electroanal. Chem. Interrac. Electrochem. 1973, 47, 329–333. [Google Scholar] [CrossRef]
Rice, F.O.; Sherber, F. The Hydrazino Radical and Tetrazane. J. Am. Chem. Soc. 1955, 77, 291–293. [Google Scholar] [CrossRef]
Karp, S.; Meites, L. The Voltammetric Characteristics and Mechanism of Electrooxidation of Hydrazine. J. Am. Chem. Soc. 1962, 84, 906–912. [Google Scholar] [CrossRef]
Ball, D.W. Tetrazane: Hartree-Fock, Gaussian-2 and -3, and Complete Basis Set Predictions of Some Thermochemical Properties of N₄H₆. J. Phys. Chem. A 2001, 105, 465–470. [Google Scholar] [CrossRef]
Dana, A.G.; Moore, K.B., III; Jasper, A.W.; Green, W.H. Large Intermediates in Hydrazine Decomposition: A Theoretical Study of the N₃H₅ and N₄H₆ Potential Energy Surfaces. J. Phys. Chem. A 2019, 123, 4679–4692. [Google Scholar] [CrossRef] [PubMed]
Bader, R.F.W. Atoms in Molecules: A Quantum Theory; Clarendon Press: Oxford, UK, 1990; ISBN 9780198558651. [Google Scholar]
Scuseria, G.E.; Janssen, C.L.; Schaefer, H.F., III. An efficient reformulation of the closed-shell coupled cluster single and double excitation (CCSD) equations. J. Chem. Phys. 1988, 89, 7382–7387. [Google Scholar] [CrossRef]
Dunning, T.H., Jr. Gaussian basis sets for use in correlated molecular calculations. I. The atoms boron through neon and hydrogen. J. Chem. Phys. 1989, 90, 1007–1023. [Google Scholar] [CrossRef]
Marenich, A.V.; Cramer, C.J.; Truhlar, D.G. Universal solvation model based on solute electron density and a continuum model of the solvent defined by the bulk dielectric constant and atomic surface tensions. J. Phys. Chem. B 2009, 113, 6378–6396. [Google Scholar] [CrossRef] [PubMed]
Frisch, G.W.; Trucks, M.J.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision B.01; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
Biegler-König, F.; Schönbohm, J.; Bayles, D. AIM2000—A Program to Analyze and Visualize Atoms in Molecules. J. Comput. Chem. 2001, 22, 545–559. [Google Scholar]
Ugliengo, P. MOLDRAW: A Program to Display and Manipulate Molecular and Crystal Structures, University Torino, Torino. 2012. Available online: https://www.moldraw.software.informer.com (accessed on 9 September 2019).

Figure 1. Optimized geometries of stable A and D structures (N—blue, H—white).

Figure 2. Optimized geometries of stable E systems (N—blue, H—white).

Table 1. Reaction, ΔE_r, and activation, E_a, energy data from elementary reactions on the N₄H₆ potential energy surface [8].

Reaction	ΔE_r (kJ/mol)	E_a (kJ/mol
N₂H₄ + H₂N=N → NH₂-NH-NH-NH₂	−103.6	50.6
N₂H₄ + H₂N=N → NH₂NH₂N=NH₂	29.0	55.4
NH₂-NH-NH-NH₂ → NH₂NH=N + NH₃	7.5	178.7
NH₂-NH-NH-NH₂ → NH₂-N=NH + NH₃	−102.5	214.1
NH₂-NH₂-N=NH₂ → NH₂-N=NH + NH₃	−245.1	38.7
NH₂-NH-NH-NH₂ → NH₂-NH-NH₂=NH	151.1	158.6
NH₂-NH-NH₂=NH → NH₂-NH₂-N=NH₂	−18.5	74.4
N₂H₃• + N₂H₃• → NH₂-NH-NH-NH₂	−152.9	0.2
NH₂-NH=NH• + NH₂• → NH₂-NH-NH-NH₂	208.9	0.2
NH=NH₂-NH• + NH2• → NH₂-NH-NH₂=NH	682.7	0.2
NH₂-N=NH₂• + NH₂• → NH₂-NH₂-N=NH₂	37.9	2.8

Table 2. N1-N2-N3-N4 dihedral angles (Θ₁₂₃₄), absolute (G₂₉₈) and relative (ΔG₂₉₈) Gibbs free energies at 298.15 K for the optimized N₄H₆ structures obtained from the starting ones. The most stable structure is highlighted in bold. The different structures with the same notation are distinguished by additional letters a, b, c, or d.

Starting	Optimized	Θ₁₂₃₄ [^o]	G₂₉₈ [Hartree]	ΔG₂₉₈ [kJ/mol]	Remarks
A2112	D2112a	168.3	−222.09177	0.00
A2121	D2121a	−161.4	−222.04654	118.75
A2211	E(22)(11)a	−33.7	−222.10760	−41.56	H₂N-NH₂ + HN=NH
A2202	A2202	−179.9	−222.04618	119.71
A2220	E(22)(20)a	146.5	−222.07817	35.7	H₂N-NH₂ + H₂N=N
A1221	D1221	−168.5	−222.00357	231.58
A3210	E(32)(10)	14.3	−222.04629	119.42	H₃N-NH₂ + HN=N
A3201	E(22)(11)b	−142.8	−222.10755	−41.43	H₂N-NH₂ + HN=NH, 1→3 H rearrangement
A3201	E(3)(201)	−26.5	−222.14890	−150.04	NH₃ + H₂N-N=NH
A3111	D2112b	75.6	−222.09311	−3.52	1→4 H rearrangement
A3120	E(31)(20)	−21.4	−222.03229	156.16	H₃N-NH + H₂N=N
A3102	E(3)(102)a	−177.1	−222.14926	−150.94	NH₃ + HN=N-NH₂
A3012	D3012a	88.8	−222.04832	114.07
A3021	A3021	176.8	−221.99866	244.46
A3003	E(3)(00)(3)	60.4	−222.26295	−449.44	2NH₃ + N₂
B2112	D2112c	72.0	−222.09665	−12.80
B2121	D2121b	−65.0	−222.04530	122.02
B2121	D2121c	−44.8	−222.04982	110.13
B2211	E(22)(11)a	−33.7	−222.10760	−41.56	H₂N-NH₂ + HN=NH
B2202	D2202a	73.7	−222.05056	108.21
B2220	E(22)(20)b	−32.9	−222.07820	35.64	H₂N-NH₂ + H₂N=N
B1221	F12)(21	75.8	−222.09760	−15.30	N2-N3 fission, N1-N4 bonding
B1221	F1)(22)(1	−33.3	−222.10756	−41.45	H₂N-NH₂ + HN=NH, N1-N4 bonding
B3210	E(22)(11)c	−34.0	−222.10754	−41.41	H₂N-NH₂ + HN=NH, 1→4 H rearrangement
B3201	D2202b	80.1	−222.04758	116.02	1→4 H rearrangement
B3201	E(3)(201)	−26.5	−222.14892	−150.04	NH₃ + H₂N=N-NH
B3111	D2112b	75.6	−222.09311	−3.52	1→4 H rearrangement
B3120	D2121d	68.8	−222.04651	118.82	1→4 H rearrangement
B3102	E(3)(102)b	−20.6	−222.14678	−144.42	NH₃ + HN=N-NH₂
B3012	D3012b	−59.7	−222.04910	112.02
B3021	D2022	−73.8	−222.05056	108.21	1→4 H rearrangement
B3003	E(3)(00)(3)	60.4	−222.26235	−449.44	2 NH₃ + N₂
C2211	E(22)(11)d	12.3	−222.10759	−41.45	H₂N-NH₂ + HN=NH
C2202	E(22)(02)	29.6	−222.07822	35.58	H₂N-NH₂ + N=NH₂
C2121	E1111	23.1	−221.99631	250.64	Cyclo-N₄H₄ + H₂

Table 3. Interatomic distances (in Å) in the optimized Amnpq and Dmnpq structures. The different structures with the same notation are distinguished by additional letters a, b, c, or d.

Structure	N1-N2	N2-N3	N3-N4	N1-H	N2-H	N3-H	N4-H
D1221	1.341	1.840	1.338	1.017	1.019 1.016	1.015 1.021	1.018
D2112a	1.423	1.467	1.431	1.012 1.018	1.014	1.016	1.011 1.014
D2112b	1.432	1.419	1.440	1.012 1.015	1.018	1.013	1.011 1.015
D2112c	1.424	1.428	1.437	1.013 1.017	1.016	1.014	1.011 1.015
D2121a	1.413	1.480	1.412	1.011 1.017	1.015	1.020 1.021	1.018
D2121b	1.423	1.467	1.417	1.010 1.013	1.018	1.017 1.020	1.019
D2121c	1.413	1.504	1.409	1.013 1.024	1.017	1.017 1.020	1.020
D2121d	1.423	1.467	1.415	1.010 1.013	1.018	1.016 1.021	1.018
A2202	1.427	1.454	1.443	1.016(2×)	1.021(2×)	-	1.013(2×)
D2202a	1.459	1.422	1.446	1.017(2×)	1.016 1.022	-	1.012 1.013
D2202b	1.464	1.418	1.446	1.016 1.018	1.017 1.021	-	1.012 1.014
D2022	1.459	1.421	1.447	1.017(2×)	-	1.016 1.022	1.012 1.013
A3021	1.463	1.452	1.433	1.016 1.024(2×)	-	1.020 1.025	1.019
D3012a	1.463	1.418	1.463	1.016 1.021(2×)	-	1.013	1.015 1.017
D3012b	1.493	1.395	1.485	1.014 1.021(2×)	-	1.021	1.014 1.017

Table 4. Interatomic distances (in Å) in the optimized Emnpq and Fmnpq systems. The different structures with the same notation are distinguished by additional letters a, b, c, or d.

System	N1-N2	N2-N3	N3-N4	N1-H	N2-H	N3-H	N4-H
E1111 ^(a)	1.476	1.481	1.481	1.023	1.017	1.017	1.017
E(22)(11)a	1.446	3.118	1.245	1.012 1.014	1.011 1.014	1.030	1.027
E(22)(11)b	1.446	3.465	1.245	1.011 1.014	1.012 1.014	1.030	1.027
E(22)(11)c	1.445	3.292	1.245	1.011 1.014	1.012 1.014	1.027	1.030
E(22)(11)d	1.446	3.116	1.245	1.012 1.014	1.011 1.014	1.030	1.027
E(22)(20)a	1.446	3.271	1.225	1.011 1.014	1.013 1.014	1.028 1.034	-
E(22)(20)b	1.446	2.971	1.225	1.013 1.014	1.011 1.014	1.028 1.033	-
E(22)(02)	1.447	3.276	1.225	1.011 1.014	1.013 1.014	-	1.028 1.033
E(31)(20)	1.468	2.750	1.230	1.018(2×) 1.029	1.016	1.029 1.057	-
E(32)(10)	1.445	3.035	1.242	1.018(2×) 1.021	1.015 1.079	1.076	-
E(3)(201)	3.088	1.350	1.249	1.013(3×)	1.006 1.022	-	1.019
E(3)(102)a	3.117	1.243	1.365	1.013(3×)	1.026	-	1.008 1.014
E(3)(102)b	3.760	1.246	1.356	1.013(2×) 1.014	1.032	-	1.007 1.024
E(3)(00)(3)	3.636	1.096	3.711	1.014(3×)	-	-	1.013(3×)
F(11)(22)	1.245	3.291	1.446	1.030	1.027	1.012 1.014	1.011 1.014
F12)(21 ^(b)	1.430	3.017	1.424	1.012	1.012 1.018	1.012 1.017	1.017

Remarks: ^(a) N1-N4 bond length of 1.476 Å, ^(b) N1-N4 bond length of 1.432 Å.

Table 5. BCP electron density (in e/Bohr³) of N-N and N-H bonds in the optimized Amnpq and Dmnpq structures. The different structures with the same notation are distinguished by additional letters a, b, c, or d.

Structure	N1-N2	N2-N3	N3-N4	N1-H	N2-H	N3-H	N4-H
D1221	0.3705	0.1281	0.3734	0.3450	0.3483 0.3528	0.3467 0.3534	0.3448
D2112a	0.3156	0.2911	0.3092	0.3459 0.3515	0.3574	0.3551	0.3492 0.3518
D2112b	0.3092	0.3237	0.3036	0.3494 0.3508	0.3527	0.3561	0.3484 0.3514
D2112c	0.3149	0.3165	0.3050	0.3472 0.3513	0.3542	0.3552	0.3484 0.3515
D2121a	0.3212	0.2827	0.3080	0.3458 0.3516	0.3544	0.3520 0.3527	0.3415
D2121b	0.3149	0.2920	0.3074	0.3509 0.3517	0.3524	0.3521 0.3551	0.3404
D2121c	0.3224	0.2668	0.3133	0.3406 0.3505	0.3542	0.3508 0.3556	0.3404
D2121d	0.3149	0.2928	0.3086	0.3503 0.3521	0.3523	0.3509 0.3559	0.3417
A2202	0.3148	0.2864	0.2965	0.3478(2×)	0.3514(2×)	-	0.3504 0.3503
D2202a	0.2928	0.3098	0.2951	0.3469 0.3474	0.3499 0.3553	-	0.3506(2×)
D2202b	0.2898	0.3107	0.2956	0.3447 0.3478	0.3507 0.3549	-	0.3501 0.3510
D2022	0.2949	0.3102	0.2930	0.3505 0.3507	-	0.3498 0.3552	0.3470 0.3474
A3021	0.2759	0.2964	0.2956	0.3431 0.3437 0.3480	-	0.3499 0.3542	0.3397
D3012a	0.2761	0.3196	0.2874	0.3454 0.3473 0.3500	-	0.3564	0.3463 0.3476
D3012b	0.2562	0.3362	0.2736	0.3446 0.3450 0.3501	-	0.3488	0.3455 0.3497

Table 6. BCP electron density (in e/Bohr³) of N-N and N-H bonds in the optimized Emnpq and Fmnpq systems. The different structures with the same notation are distinguished by additional letters a, b, c, or d.

System	N1-N2	N2-N3	N3-N4	N1-H	N2-H	N3-H	N4-H
E1111 ^(a)	0.2858	0.2828	0.2824	0.3506	0.3545	0.3566	0.3546
E(22)(11)a	0.2953	-	0.4863	0.3502 0.3529	0.3500 0.3529	0.3463	0.3483
E(22)(11)b	0.2953	-	0.4826	0.3500 0.3529	0.3502 0.3529	0.3462	0.3482
E(22)(11)c	0.2954	-	0.4863	0.3500 0.3529	0.3503 0.3529	0.3482	0.3462
E(22)(11)d	0.2953	-	0.4863	0.3502 0.3529	0.3500 0.3529	0.3463	0.3482
E(22)(20)a	0.2947	-	0.4970	0.3499 0.3529	0.3501 0.3518	0.3367 0.3422	-
E(22)(20)b	0.2945	-	0.4967	0.3501 0.3517	0.3499 0.3529	0.3367 0.3423	-
E(22)(02)	0.2945	-	0.4967	0.3500 0.3529	0.3501 0.3517	-	0.3367 0.3423
E(31)(20)	0.2696	-	0.4927	0.3382 0.3486 0.3493	0.3426	0.3123 0.3412	-
E(32)(10)	0.2929	-	0.4831	0.3471 0.3453 0.3474	0.2909 0.3485	0.3032	-
E(3)(201)	-	0.3794	0.4825	0.3434 0.3435 0.3436	0.3531 0.3368	-	0.3503
E(3)(102)a	-	0.4891	0.3669	0.3435(2×) 0.3436	0.3448	-	0.3457 0.3521
E(3)(102)b	-	0.4833	0.3719	0.3432 0.3435 0.3436	0.3375	-	0.3345 0.3523
E(3)(00)(3)	-	0.7140	-	0.3432 0.3433 0.3433	-	-	0.3435 0.3437 0.3442
F(11)(22)	0.4863	-	0.2954	0.3463	0.3482	0.3502 0.3529	0.3500 0.3529
F12)(21 ^(b)	0.3096	-	0.3150	0.3573	0.3451 0.3515	0.3470 0.3515	0.3522

Remarks: ^(a) N1-N4 BCP electron density of 0.2858 e/Bohr³, ^(b) N1-N4 BCP electron density of 0.3134 e/Bohr³.

Table 7. BCP ellipticity of N-N and N-H bonds in the optimized Amnpq and Dmnpq structures. The different structures with the same notation are distinguished by additional letters a, b, c, or d.

Structure	N1-N2	N2-N3	N3-N4	N1-H	N2-H	N3-H	N4-H
D1221	0.230	0.107	0.231	0.048	0.015 0.017	0.015 0.016	0.047
D2112a	0.003	0.149	0.024	0.045 0.050	0.041	0.036	0.046 0.051
D2112b	0.040	0.039	0.012	0.046 0.051	0.043	0.051	0.047 0.051
D2112c	0.027	0.046	0.015	0.044 0.047	0.047	0.050	0.046 0.050
D2121a	0.027	0.123	0.198	0.048 0.051	0.046	0.007 0.009	0.073
D2121b	0.035	0.070	0.182	0.045 0.049	0.034	0.011 0.015	0.074
D2121c	0.026	0.074	0.192	0.039 0.048	0.038	0.013(2×)	0.071
D2121d	0.025	0.069	0.178	0.045 0.050	0.033	0.012 0.013	0.073
A2202	0.060	0.302	0.089	0.036(2×)	0.008(2×)	-	0.055(2×)
D2202a	0.045	0.288	0.084	0.034 0.035	0.012 0.013	-	0.053(2×)
D2202b	0.086	0.301	0.087	0.039 0.041	0.010 0.082	-	0.052 0.053
D2022	0.084	0.288	0.046	0.052 0.053	-	0.012 0.013	0.034 0.035
A3021	0.268	0.222	0.169	0.106 0.108 0.005	-	0.006 0.009	0.079
D3012a	0.267	0.124	0.064	0.006 0.007 0.008	-	0.049	0.044 0.048
D3012b	0.248	0.113	0.123	0.004 0.005(2×)	-	0.051	0.038(2×)

Table 8. BCP ellipticity of N-N and N-H bonds in the optimized Emnpq and Fmnpq systems. The different structures with the same notation are distinguished by additional letters a, b, c, or d.

System	N1-N2	N2-N3	N3-N4	N1-H	N2-H	N3-H	N4-H
E1111 ^(a)	0.103	0.108	0.108	0.029	0.030	0.027	0.030
E(22)(11)a	0.008	-	0.189	0.047 0.049	0.046 0.050	0.004	0.004
E(22)(11)b	0.008	-	0.189	0.046 0.050	0.047 0.049	0.004	0.004
E(22)(11)c	0.008	-	0.189	0.046 0.050	0.047 0.049	0.004	0.004
E(22)(11)d	0.008	-	0.189	0.047 0.049	0.046 0.049	0.004	0.004
E(22)(20)a	0.008	-	0.021	0.046 0.049	0.047(2×)	0.035 0.038	-
E(22)(20)b	0.007	-	0.020	0.047(2×)	0.046 0.049	0.035 0.038	-
E(22)(02)	0.007	-	0.020	0.046 0.049	0.047(2×)	-	0.035 0.039
E(31)(20)	0.156	-	0.005	0.006 0.011 0.012	0.080	0.029 0.035	-
E(32)(10)	0.089	-	0.072	0.009 0.010(2×)	0.027 0.045	0.005	-
E(3)(201)	-	0.138	0.229	0.033(3×)	0.043 0.053	-	0.008
E(3)(102)a	-	0.218	0.118	0.326 0.327(2×)	0.005	-	0.047 0.051
E(3)(102)b	-	0.238	0.133	0.324(2×) 0.329	0.001	-	0.041 0.052
E(3)(00)(3)	-	0.000	-	0.033 0.034(2×)	-	-	0.033(3×)
F(11)(22)	0.189	-	0.008	0.004	0.004	0.047 0.049	0.046 0.050
F12)(21 ^(b)	0.012	-	0.031	0.054	0.046 0.051	0.045 0.047	0.049

Remarks: ^(a) N1-N4 BCP ellipticity of 0.103; ^(b) N1-N4 BCP ellipticity of 0.041.

Table 9. Atomic charges of N and H (bonded to N in brackets) in the optimized Amnpq and Dmnpq structures. The asterisks denote the atoms also included in hydrogen bonds. The different structures with the same notation are distinguished by additional letters a, b, c, or d.

Structure	N1	N2	N3	N4	H(N1)	H(N2)	H(N3)	H(N4)
D1221	−0.657	−0.488	−0.485	−0.649	0.342	0.452 0.455	0.444 0.457	0.342
D2112a	−0.699	−0.347	−0.367	−0.706	0.379 0.392	0.391	0.382	0.391 0.404
D2112b	−0.691	−0.357	−0.354	−0.726	0.378 0.394	0.372	0.395	0.387 0.398
D2112c	−0.711	−0.354	−0.368	−0.729	0.377 0.389	0.382	0.396	0.389 0.401
D2121a	−0.700	−0.341	−0.398	−0.787	0.394 0.413	0.417	0.452(2×)	0.309
D2121b	−0.704	−0.361	−0.394	−0.811	0.400 0.416	0.405	0.470 0.560	0.302
D2121c	−0.709	−0.365	−0.412	−0.800 *	0.396 0.407 *	0.406	0.463 0.468	0.310
D2121d	−0.707	−0.361	−0.395	−0.809	0.402 0.420	0.408	0.458 0.471	0.304
A2202	−0.664	−0.388	−0.435	−0.750	0.418(2×)	0.450(2×)	-	0.362(2×)
D2202a	−0.712	−0.404	−0.430	−0.760	0.409 0.410	0.455 0.475	-	0.361 0.364
D2202b	−0.705	−0.397	−0.432	−0.737	0.407 0.411	0.459 0.465	-	0.357 0.367
D2022	−0.761	−0.430	−0.402	−0.711	0.361 0.365	-	0.455 0.475	0.409 0.410
A3021	−0.730	−0.368	−0.388	−0.824	0.460 0.461 0.496	-	0.403 0.423	0.286
D3012a	−0.732	−0.436	−0.390	−0.739	0.449(2×) 0.472	-	0.370	0.360 0.372
D3012b	−0.762	−0.417	−0.384	−0.754 *	0.444 0.466 * 0.473	-	0.345	0.376 0.378

Table 10. Atomic charges of N and H (bonded to N in bracket) in the optimized Emnpq and Fmnpq systems. Asterisks denote atoms also included in hydrogen bonds. The different structures with the same notation are distinguished by additional letters a, b, c, or d.

System	N1	N2	N3	N4	H(N1)	H(N2)	H(N3)	H(N4)
E1111	−0.345	−0.367 *	−0.373	−0.367	0.383	0.408	0.396 *	0.403
E(22)(11)a	−0.707	−0.727 *	−0.358	−0.348 *	0.380 0.392 *	0.385 0.393	0.409 *	0.380
E(22)(11)b	−0.727 *	−0.707	−0.360	−0.348 *	0.384 0.393	0.380 0.388 *	0.409 *	0.380
E(22)(11)c	−0.726 *	−0.706	−0.349h	−0.360	0.384 0.393	0.380 0.387 *	0.380	0.409h
E(22)(11)d	−0.706	−0.726 *	−0.359	−0.347 *	0.380 0.388 *	0.384 0.393	0.409 *	0.380
E(22)(20)a	−0.732 *	−0.714	−0.519	−0.271 *	0.380 0.393 *	0.387 0.395	0.417 0.460 *	-
E(22)(20)b	−0.713	−0.732 *	−0.517	−0.273 *	0.380 0.393 *	0.387 0.395	0.417 0.461 *	-
E(22)(02)	−0.714	−0.732 *	−0.272 *	−0.517	0.380 0.393 *	0.387 0.395	-	0.417 0.460 *
E(31)(20)	−0.751	−0.831 *	−0.543	−0.306 *	0.447 0.452 0.491 *	0.315	0.408 0.511 *	-
E(32)(10)	−0.718	−0.731	−0.426	−0.530 *	0.496 0.508(2×)	0.408 0.501 *	0.185	-
E(3)(201)	−1.079 *	−0.734	−0.035	−0.436	0.394(3×)	0.443 0.473 *	-	0.388
E(3)(102)a	−1.076 *	−0.454	−0.033	−0.686	0.394(3×)	0.428 *	-	0.429 0.445
E(3)(102)b	−1.084 *	−0.396	−0.030	−0.739	0.394 0.395 0.396	0.352	-	0.445 0.470 *
E(3)(00)(3)	−1.077 *	0.076 *	−0.049	−1.059	0.382 0.382 * 0.384	-	-	0.373 0.380 * 0.386
F(11)(22)	−0.359	−0.347 *	−0.706	−0.725 *	0.409 *	0.380	0.380 0.388 *	0.393 0.394
F12)(21	−0.356	−0.722	−0.702	−0.368	0.403	0.377 0.392	0.371 0.395	0.381

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).

Share and Cite

MDPI and ACS Style

Breza, M.; Manova, A. Hydrazine Oxidation in Aqueous Solutions I: N₄H₆ Decomposition. Inorganics 2023, 11, 413. https://doi.org/10.3390/inorganics11100413

AMA Style

Breza M, Manova A. Hydrazine Oxidation in Aqueous Solutions I: N₄H₆ Decomposition. Inorganics. 2023; 11(10):413. https://doi.org/10.3390/inorganics11100413

Chicago/Turabian Style

Breza, Martin, and Alena Manova. 2023. "Hydrazine Oxidation in Aqueous Solutions I: N₄H₆ Decomposition" Inorganics 11, no. 10: 413. https://doi.org/10.3390/inorganics11100413

APA Style

Breza, M., & Manova, A. (2023). Hydrazine Oxidation in Aqueous Solutions I: N₄H₆ Decomposition. Inorganics, 11(10), 413. https://doi.org/10.3390/inorganics11100413

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Menu