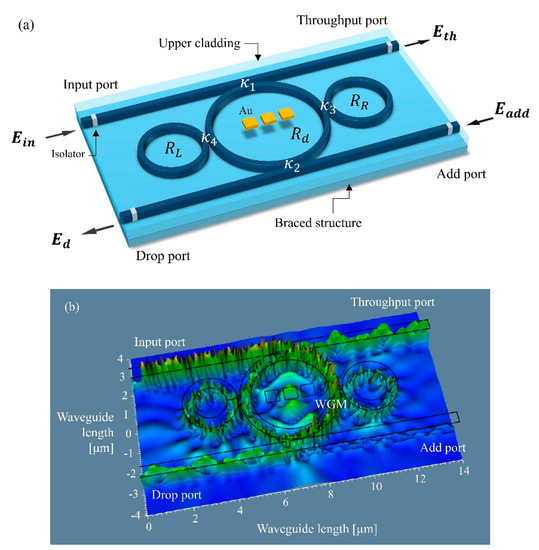
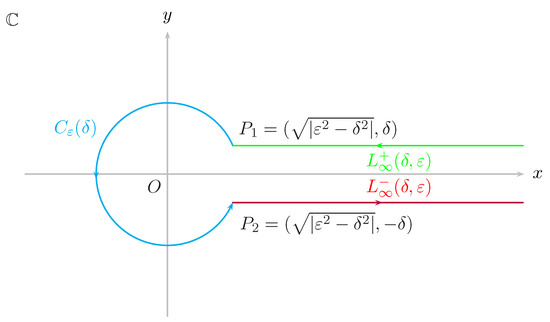
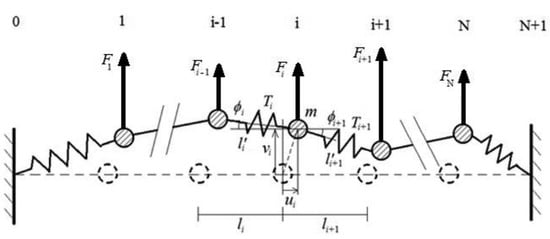
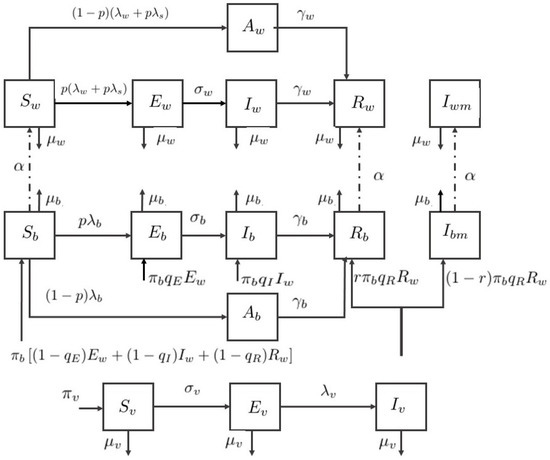
Mathematical Analysis and Applications (Closed)
A topical collection in Axioms (ISSN 2075-1680). This collection belongs to the section "Mathematical Analysis".
Viewed by 91595Editor
Interests: real and complex analysis; fractional calculus and its applications; integral equations and transforms; higher transcendental functions and their applications; q-series and q-polynomials; analytic number theory; analytic and geometric Inequalities; probability and statistics; inventory modeling and optimization
Special Issues, Collections and Topics in MDPI journals
Topical Collection Information
Dear Colleagues,
Investigations involving the theory and applications of the mathematical analytic tools and techniques are remarkably widespread in many diverse areas of the mathematical, physical, chemical, engineering, and statistical sciences. In this Collection, we invite and welcome review, expository, and original research articles dealing with the recent advances in mathematical analysis and its multidisciplinary applications.
Looking forward to your contribution to this Topical Collection,
Cordially yours,
Prof. Dr. H. M. Srivastava
Collection Editor
Manuscript Submission Information
Manuscripts should be submitted online at www.mdpi.com by registering and logging in to this website. Once you are registered, click here to go to the submission form. Manuscripts can be submitted until the deadline. All submissions that pass pre-check are peer-reviewed. Accepted papers will be published continuously in the journal (as soon as accepted) and will be listed together on the collection website. Research articles, review articles as well as short communications are invited. For planned papers, a title and short abstract (about 100 words) can be sent to the Editorial Office for announcement on this website.
Submitted manuscripts should not have been published previously, nor be under consideration for publication elsewhere (except conference proceedings papers). All manuscripts are thoroughly refereed through a single-blind peer-review process. A guide for authors and other relevant information for submission of manuscripts is available on the Instructions for Authors page. Axioms is an international peer-reviewed open access monthly journal published by MDPI.
Please visit the Instructions for Authors page before submitting a manuscript. The Article Processing Charge (APC) for publication in this open access journal is 2400 CHF (Swiss Francs). Submitted papers should be well formatted and use good English. Authors may use MDPI's English editing service prior to publication or during author revisions.
Keywords
- Mathematical (or higher transcendental) functions and their applications
- Fractional calculus and its applications
- q-series and q-polynomials
- Analytic number theory
- Special functions of mathematical physics and applied mathematics
- Geometric function theory of complex analysis
Related Special Issues
- Mathematical Analysis and Applications in Axioms (16 articles - displayed below)
- Mathematical Analysis and Applications II in Axioms (18 articles - displayed below)
- Mathematical Tools and Techniques Applicable to Probability Theory and Statistics in Axioms (13 articles - displayed below)
- Mathematical Analysis and Applications III in Axioms (14 articles)